Inscape
My entry for New Year's Day links to a paper by Robert T. Curtis*
from The Arabian Journal for Science and Engineering
(King Fahd University, Dhahran, Saudi Arabia),
Volume 27, Number 1A, January 2002.
From that paper:
"Combinatorially, an outer automorphism [of S6] can exist because the number of unordered pairs of 6 letters is equal to the number of ways in which 6 letters can be partitioned into three pairs. Which is to say that the two conjugacy classes of odd permutations of order 2 in S6 contain the same number of elements, namely 15. Sylvester… refers to the unordered pairs as duads and the partitions as synthemes. Certain collections of five synthemes… he refers to as synthematic totals or simply totals; each total is stabilized within S6 by a subgroup acting triply transitively on the 6 letters as PGL2(5) acts on the projective line. If we draw a bipartite graph on (15+15) vertices by joining each syntheme to the three duads it contains, we obtain the famous 8-cage (a graph of valence 3 with minimal cycles of length 8)…."
Here is a way of picturing the 8-cage and a related configuration of points and lines:
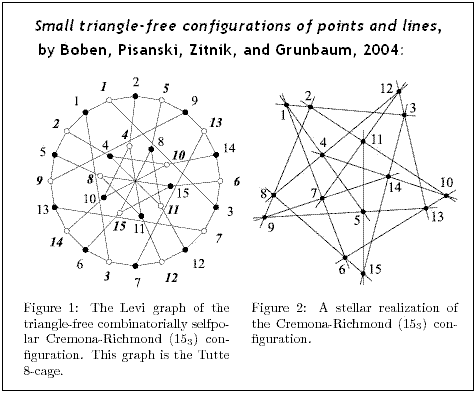
Diamond Theory shows that this structure
can also be modeled by an "inscape"
made up of subsets of a
4×4 square array:

The illustration below shows how the
points and lines of the inscape may
be identified with those of the
Cremona-Richmond configuration.
