Saturday, September 17, 2016
Thursday, September 15, 2016
The Smallest Perfect Number/Universe
The smallest perfect number,* six, meets
"the smallest perfect universe,"** PG(3,2).
* For the definition of "perfect number," see any introductory
number-theory text that deals with the history of the subject.
** The phrase "smallest perfect universe" as a name for PG(3,2),
the projective 3-space over the 2-element Galois field GF(2),
was coined by math writer Burkard Polster. Cullinane's square
model of PG(3,2) differs from the earlier tetrahedral model
discussed by Polster.
Comments Off on The Smallest Perfect Number/Universe
Tuesday, September 13, 2016
Parametrizing the 4×4 Array
The previous post discussed the parametrization of
the 4×4 array as a vector 4-space over the 2-element
Galois field GF(2).
The 4×4 array may also be parametrized by the symbol
0 along with the fifteen 2-subsets of a 6-set, as in Hudson's
1905 classic Kummer's Quartic Surface —
Hudson in 1905:
These two ways of parametrizing the 4×4 array — as a finite space
and as an array of 2-element sets — were related to one another
by Cullinane in 1986 in describing, in connection with the Curtis
"Miracle Octad Generator," what turned out to be 15 of Hudson's
1905 "Göpel tetrads":
A recap by Cullinane in 2013:
Click images for further details.
Comments Off on Parametrizing the 4×4 Array
Friday, August 26, 2016
Structure a Set, Set a Structure
A star figure and the Galois quaternion.
The square root of the former is the latter.
See also a search in this journal for "Set a Structure."
Comments Off on Structure a Set, Set a Structure
Wednesday, August 24, 2016
Core Statements
"That in which space itself is contained" — Wallace Stevens
An image by Steven H. Cullinane from April 1, 2013:
The large Desargues configuration of Euclidean 3-space can be
mapped canonically to the 4×4 square of Galois geometry —
On an Auckland University of Technology thesis by Kate Cullinane —
The thesis reportedly won an Art Directors Club award on April 5, 2013.
Comments Off on Core Statements
Tuesday, August 23, 2016
Puritan Contemplation:
For an authority on Japanese art —
Text Tiles*
Compare to and contrast with …
Remarks on art, contemplation. and Puritanism
from a recent Princeton University Press book —
“Lucy Lippard distinguished Asian art
(ego-less and contemplative)
from New York Minimalism
(moralistic and puritanical).”
— Mathematics and Art ,
Princeton U. Press, Fall 2015
* Update of Aug. 24, 2016 — See also Nov. 2, 2014.
Comments Off on Puritan Contemplation:
Sunday, August 21, 2016
Imperium Emporium
Comments Off on Imperium Emporium
Tuesday, August 16, 2016
Midnight Narrative
The images in the previous post do not lend themselves
to any straightforward narrative. Two portions of the
large image search are, however, suggestive —
Cross and Boolean lattice.
The improvised cross in the second pair of images
is perhaps being wielded to counteract the
Boole of the first pair of images. See the heading
of the webpage that is the source of the lattice
diagram toward which the cross is directed —
Update of 10 am on August 16, 2016 —
See also Atiyah on the theology of
(Boolean) algebra vs. (Galois) geometry:
Comments Off on Midnight Narrative
Monday, August 1, 2016
Cube
From this journal —
See (for instance) Sacred Order, July 18, 2006 —
From a novel published July 26, 2016, and reviewed
in yesterday's (print) New York Times Book Review —
|
The doors open slowly. I step into a hangar. From the rafters high above, lights blaze down, illuminating a twelve-foot cube the color of gunmetal. My pulse rate kicks up. I can’t believe what I’m looking at. Leighton must sense my awe, because he says, “Beautiful, isn’t it?” It is exquisitely beautiful. At first, I think the hum inside the hangar is coming from the lights, but it can’t be. It’s so deep I can feel it at the base of my spine, like the ultralow-frequency vibration of a massive engine. I drift toward the box, mesmerized.
— Crouch, Blake. Dark Matter: A Novel |
See also Log24 on the publication date of Dark Matter .
Comments Off on Cube
Saturday, July 30, 2016
Binary
"Benedict Cumberbatch Says a Journey
From Fact to Faith Is at the Heart of Doctor Strange"
— io9 yesterday
" 'This man comes from a binary universe where it’s all about logic,'
the actor told us at San Diego Comic-Con . . . .
'And there’s a lot of humor in the collision between Easter [sic ]
mysticism and Western scientific, sort of logical binary.' "
Related material — Strange Awards, April 14, 2016.
I prefer a different sort of journey. See Boole vs. Galois.
Comments Off on Binary
Thursday, July 28, 2016
The Giglmayr Foldings
Giglmayr's transformations (a), (c), and (e) convert
his starting pattern
1 2 5 6
3 4 7 8
9 10 13 14
11 12 15 16
to three length-16 sequences. Putting these resulting
sequences back into the 4×4 array in normal reading
order, we have
1 2 3 4 1 2 4 3 1 4 2 3
5 6 7 8 5 6 8 7 7 6 8 5
9 10 11 12 13 14 16 15 15 14 16 13
13 14 15 16 9 10 12 11 9 12 10 11
(a) (c) (e)
Four length-16 basis vectors for a Galois 4-space consisting
of the origin and 15 weight-8 vectors over GF(2):
0 0 0 0 0 0 0 0 0 0 1 1 0 1 0 1
0 0 0 0 1 1 1 1 0 0 1 1 0 1 0 1
1 1 1 1 0 0 0 0 0 0 1 1 0 1 0 1
1 1 1 1 1 1 1 1 0 0 1 1 0 1 0 1 .
(See "Finite Relativity" at finitegeometry.org/sc.)
The actions of Giglmayr's transformations on the above
four basis vectors indicate the transformations are part of
the affine group (of order 322,560) on the affine space
corresponding to the above vector space.
For a description of such transformations as "foldings,"
see a search for Zarin + Folded in this journal.
Comments Off on The Giglmayr Foldings
Sunday, July 24, 2016
Point Omega …
In this post, "Omega" denotes a generic 4-element set.
For instance … Cullinane's
![Logo for 'Elements of Finite Geometry'](http://m759.net/wordpress/?s="Elements-logo.gif"){kind=logo}
or Schmeikal's
.
The mathematics appropriate for describing
group actions on such a set is not Schmeikal's
Clifford algebra, but rather Galois's finite fields.
Comments Off on Point Omega …
Wednesday, July 20, 2016
Weather for Jews
"What I'm aiming for are moments of strong sensation —
moments of total physical experience of the landscape,
when weather just reaches out and sucks you in."
— The late Jane Wilson —
See also the previous post and, from the date of Wilson's death,
Geometry for Jews (Continued) —
Comments Off on Weather for Jews
Saturday, July 16, 2016
The Midnight Beginning
From the midnight beginning June 27, 2016 —
This review of the above post was suggested by the
Galois-related footnote in the previous post and by
an obituary in this morning's online New York Times .
See as well a July 6 obituary for the same person in
The Martha's Vineyard Times .
Comments Off on The Midnight Beginning
Binary Shema: The “O” and the “I”
The "I" of "I Am that I Am" has been described as a creation of
an "ur-unity" (see the Anderson passage below) and this ur-unity,
denoted by "O," has been described elsewhere as "a primary reality"
(see the Sullivan passage below). These descriptions are of course
much less clear than those usually given for the similar purely
mathematical * notations "0" and "1."
See also Quine's Shema in "Is Nothing Sacred?" —
0! = 1.
Quoted here on July 30, 2015 —
Linked to here on June 29, 2016 —
* Note for mathematicians: Here characteristic 0 is assumed .
Quine's Shema does not apply to Galois.
Comments Off on Binary Shema: The “O” and the “I”
Wednesday, June 29, 2016
Space Jews
For the Feast of SS. Peter and Paul —
In memory of Alvin Toffler and Simon Ramo,
a review of figures from the midnight that began
the date of their deaths, June 27, 2016 —
The 3×3×3 Galois Cube
See also Rubik in this journal.
Comments Off on Space Jews
Monday, June 27, 2016
Interplay
From a search in this journal for Euclid + Galois + Interplay —
The 3×3×3 Galois Cube
A tune suggested by the first image above —
Comments Off on Interplay
Saturday, June 18, 2016
Midnight in Herald Square
In memory of New Yorker artist Anatol Kovarsky,
who reportedly died at 97 on June 1.
Note the Santa, a figure associated with Macy's at Herald Square.
See also posts tagged Herald Square, as well as the following
figure from this journal on the day preceding Kovarsky's death.
A note related both to Galois space and to
the "Herald Square"-tagged posts —
"There is such a thing as a length-16 sequence."
— Saying adapted from a young-adult novel.
Comments Off on Midnight in Herald Square
Thursday, June 2, 2016
Bullshit Studies
"The allusion to 'the most precious square of sense' shows
Shakespeare doing an almost scholastic demonstration of
the need for a ratio and interplay among the senses as
the very constitution of rationality."
— Marshall McLuhan, The Gutenberg Galaxy ,
University of Toronto Press, 1962, page 13
"What Shakespeare refers to in Lear as the 'precious
square of sense' probably has reference to the traditional
'square of opposition' in logic and to that four-part analogy
of proportionality which is the interplay of sense and reason."
— McLuhan, ibid. , page 241
This is of course nonsense, and, in view of McLuhan's pose
as a defender of the Catholic faith, damned nonsense.
Epigraph by McLuhan —
"The Gutenberg Galaxy develops a mosaic or field
approach to its problems."
I prefer a different "mosaic or field" related to the movable
blocks of Fröbel, not the movable type of Gutenberg.
Comments Off on Bullshit Studies
Monday, May 30, 2016
Perfect Universe
(A sequel to the previous post, Perfect Number)
Since antiquity, six has been known as
"the smallest perfect number." The word "perfect"
here means that a number is the sum of its
proper divisors — in the case of six: 1, 2, and 3.
The properties of a six-element set (a "6-set")
divided into three 2-sets and divided into two 3-sets
are those of what Burkard Polster, using the same
adjective in a different sense, has called
"the smallest perfect universe" — PG(3,2), the projective
3-dimensional space over the 2-element Galois field.
A Google search for the phrase "smallest perfect universe"
suggests a turnaround in meaning , if not in finance,
that might please Yahoo CEO Marissa Mayer on her birthday —
The semantic turnaround here in the meaning of "perfect"
is accompanied by a model turnaround in the picture of PG(3,2) as
Polster's tetrahedral model is replaced by Cullinane's square model.
Further background from the previous post —
See also Kirkman's Schoolgirl Problem.
Comments Off on Perfect Universe
Tuesday, May 24, 2016
Rosenhain and Göpel Revisited
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above
Comments Off on Rosenhain and Göpel Revisited
Monday, May 9, 2016
Search for the Lost Theorem
The three Solomons of the previous post (LeWitt,
Marcus, and Golomb) suggest the three figures
-1, 0, and 1 … symbols for the three elements
of the Galois field GF(3). This in turn suggests a
Search for The Lost Theorem. Some cross-cultural
context: The First of May, 2010.
Comments Off on Search for the Lost Theorem
Sunday, May 8, 2016
The Three Solomons
Earlier posts have dealt with Solomon Marcus and Solomon Golomb,
both of whom died this year — Marcus on Saint Patrick's Day, and
Golomb on Orthodox Easter Sunday. This suggests a review of
Solomon LeWitt, who died on Catholic Easter Sunday, 2007.
A quote from LeWitt indicates the depth of the word "conceptual"
in his approach to "conceptual art."
|
From Sol LeWitt: A Retrospective , edited by Gary Garrels, Yale University Press, 2000, p. 376:
THE SQUARE AND THE CUBE "The best that can be said for either the square or the cube is that they are relatively uninteresting in themselves. Being basic representations of two- and three-dimensional form, they lack the expressive force of other more interesting forms and shapes. They are standard and universally recognized, no initiation being required of the viewer; it is immediately evident that a square is a square and a cube a cube. Released from the necessity of being significant in themselves, they can be better used as grammatical devices from which the work may proceed." "Reprinted from Lucy R. Lippard et al ., “Homage to the Square,” Art in America 55, No. 4 (July-August 1967): 54. (LeWitt’s contribution was originally untitled.)" |
See also the Cullinane models of some small Galois spaces —
Comments Off on The Three Solomons
Friday, May 6, 2016
Monday, April 25, 2016
Seven Seals
An old version of the Wikipedia article "Group theory"
(pictured in the previous post) —
"More poetically …"
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
The seven seals from the previous post, with some context —
These models of projective points are drawn from the underlying
structure described (in the 4×4 case) as part of the proof of the
Cullinane diamond theorem .
Comments Off on Seven Seals
Thursday, April 14, 2016
One Funeral at a Time
On this date in 2005, mathematician Saunders Mac Lane died at 95.
Related material —
Max Planck quotations:
Mac Lane on Boolean algebra:
Mac Lane’s summary chart (note the absence of Galois geometry ):
I disagree with Mac Lane’s assertion that “the finite models of
Boolean algebra are dull.” See Boole vs. Galois in this journal.
Comments Off on One Funeral at a Time
Strange Awards
From a review of a play by the late Anne Meara* —
"Meara, known primarily as an actress/comedian
(half of the team of Stiller & Meara, and mother of
Ben Stiller), is also an accomplished writer for the
stage; her After Play was much acclaimed….
This new, more ambitious piece starts off with a sly
send-up of awards dinners as the late benefactor of
a wealthy foundation–the comically pixilated scientist
Herschel Strange (Jerry Stiller)–is seen on videotape.
This tape sets a light tone that is hilariously
heightened when John Shea, as Arthur Garden,
accepts the award given in Strange's name."
Compare and contrast —
- A figure from yesterday's 1 PM post Black List:
-
The circular device of Doctor Strange on the cover of
Entertainment Weekly , Jan. 8/15 2016:
I of course prefer the Galois I Ching .
* See the May 25, 2015, post The Secret Life of the Public Mind.
Comments Off on Strange Awards
Monday, April 11, 2016
Like Decorations in a Cartoon Graveyard
From Sunday evening's In Memoriam post —
The "from Princeton" remark in the previous post came from
Princeton, but originated with a retired professor in Rochester,
NY, one Joseph Neisendorfer.
Another remark by Neisendorfer, from his weblog —
Those familiar with the chapter on Galois in the
Eric Temple Bell classic Men of Mathematics
will know that the words quoted above by
Neisendorfer are definitely not those of Albert Einstein.
Comments Off on Like Decorations in a Cartoon Graveyard
Combinatorial Spider
“Chaos is order yet undeciphered.”
— The novel The Double , by José Saramago,
on which the film "Enemy" was based
Some background for the 2012 Douglas Glover
"Attack of the Copula Spiders" book
mentioned in Sunday's Synchronicity Check —
-
"A vision of Toronto as Hell" — Douglas Glover in the
March 25, 2011, post Combinatorial Delight - For Louise Bourgeois — a post from the date of Galois's death—
Comments Off on Combinatorial Spider
Friday, April 8, 2016
Space Cross
For George Orwell
Illustration from a book on mathematics —
This illustrates the Galois space AG(4,2).
For some related spaces, see a note from 1984.
"There is such a thing as a space cross."
— Saying adapted from a young-adult novel
Comments Off on Space Cross
Monday, April 4, 2016
The Bauersfeld Structure*
"If you would be a poet, create works capable of
answering the challenge of apocalyptic times,
even if this meaning sounds apocalyptic."
"It's a trap!"
— Ferlinghetti's friend Erik Bauersfeld,
who reportedly died yesterday at 93
* See also, in this journal, Galois Cube and Deathtrap.
Comments Off on The Bauersfeld Structure*
Tuesday, February 23, 2016
Both Sides Now
(Continued from the link in the previous post to
a Feb. 20 NY Times essay on the brain's two sides)
From a webpage on Galois geometry —
|
Postscript From a 2002 review by Stacy G. Langton of Sherman Stein's book on mathematics, How the Other Half Thinks : "The title of Stein's book (perhaps chosen by the publisher?) seems to refer to the popular left brain/right brain dichotomy. As Stein writes (p. ix): 'I hope this book will help bridge that notorious gap that separates the two cultures: the humanities and the sciences, or should I say the right brain (intuitive, holistic) and the left brain (analytical, numerical). As the chapters will illustrate, mathematics is not restricted to the analytical and numerical; intuition plays a significant role.' Stein does well to avoid identifying mathematics with the activity of just one side of the brain. He would have done better, however, not to have endorsed the left brain/right brain ideology. While it does indeed appear to be the case that the two sides of our brain act in rather different ways, the idea that the right brain is 'intuitive, holistic,' while the left brain is 'analytical, numerical,' is a vast oversimplification, and goes far beyond the actual evidence." |
Comments Off on Both Sides Now
Wednesday, February 17, 2016
“Blank Space” Accolades
A post in memory of British theatre director Peter Wood,
who reportedly died on February 11, 2016.
From the date of the director's death —
"Leave a space." — Tom Stoppard
Comments Off on “Blank Space” Accolades
Friday, February 12, 2016
The Game
"Right through hell
there is a path…."
— Malcolm Lowry,
Under the Volcano
Comments Off on The Game
Sunday, January 31, 2016
Forgotten Lore
Continued from Sunday, January 24, 2016
Wikipedia on Io in Greek mythology
(a precursor to Marvel Comics) —
"Walter Burkert [18] notes that the story of Io was told
in the ancient epic tradition at least four times….
18. Burkert, Homo Necans (1974) 1983:
164 note 14, giving bibliography."
An "io" story I prefer — m24.io.
Comments Off on Forgotten Lore
Friday, January 29, 2016
Friday, January 22, 2016
Easter Footnote
A search from Easter 2013 for "Cremona synthemes" * —
For some strictly mathematical background, see
Classical Geometry in Light of Galois Geometry.
* For more about Cremona and synthemes,
see a 1975 paper by W. L. Edge,
"A Footnote on the Mystic Hexagram."
Comments Off on Easter Footnote
Thursday, January 21, 2016
Dividing the Indivisible
My statement yesterday morning that the 15 points
of the finite projective space PG(3,2) are indivisible
was wrong. I was misled by quoting the powerful
rhetoric of Lincoln Barnett (LIFE magazine, 1949).
Points of Euclidean space are of course indivisible:
"A point is that which has no parts" (in some translations).
And the 15 points of PG(3,2) may be pictured as 15
Euclidean points in a square array (with one point removed)
or tetrahedral array (with 11 points added).
The geometry of PG(3,2) becomes more interesting,
however, when the 15 points are each divided into
several parts. For one approach to such a division,
see Mere Geometry. For another approach, click on the
image below.
Comments Off on Dividing the Indivisible
Wednesday, January 13, 2016
Geometry for Jews
(Continued from previous episodes)
Boole and Galois also figure in the mathematics of space —
i.e. , geometry. See Boole + Galois in this journal.
Related material, according to Jung’s notion of synchronicity —
- This journal on the date, August 6, 2007, of the
above paper, and the following day —
posts now tagged Metamorphosis 2007 - This journal on two of the dates of the 2003 Haifa workshop
that the paper mentions in a footnote —
posts now tagged Solomon’s Mental Health Month
Comments Off on Geometry for Jews
Monday, January 11, 2016
Space Oddity
It is an odd fact that the close relationship between some
small Galois spaces and small Boolean spaces has gone
unremarked by mathematicians.
A Google search today for “Galois spaces” + “Boolean spaces”
yielded, apart from merely terminological sources, only some
introductory material I have put on the Web myself.
Some more sophisticated searches, however led to a few
documents from the years 1971 – 1981 …
“Harmonic Analysis of Switching Functions” ,
by Robert J. Lechner, Ch. 5 in A. Mukhopadhyay, editor,
Recent Developments in Switching Theory , Academic Press, 1971.
“Galois Switching Functions and Their Applications,”
by B. Benjauthrit and I. S. Reed,
JPL Deep Space Network Progress Report 42-27 , 1975
D.K. Pradhan, “A Theory of Galois Switching Functions,”
IEEE Trans. Computers , vol. 27, no. 3, pp. 239-249, Mar. 1978
“Switching functions constructed by Galois extension fields,”
by Iwaro Takahashi, Information and Control ,
Volume 48, Issue 2, pp. 95–108, February 1981
An illustration from the Lechner paper above —
“There is such a thing as harmonic analysis of switching functions.”
— Saying adapted from a young-adult novel
Comments Off on Space Oddity
Sunday, January 10, 2016
Sermons
This journal last Sunday —
For a more traditional sermon from last Sunday
at Nassau Presbyterian Church, Princeton NJ,
see "God for Dummies."
Comments Off on Sermons
Thursday, January 7, 2016
Point Omega…
… Continues. See previous episodes.
See as well …
-
Types of Ambiguity : Galois Meets Doctor Faustus,
from December 14, 2010.
-
From Jan. 5, the date of Pierre Boulez's death,
a post on Galois geometry.
- 2016 Joint Mathematics Meetings
The above image is from April 7, 2003.
Comments Off on Point Omega…
Tuesday, January 5, 2016
Sunday, January 3, 2016
Focus and Clarity in the New Year
A new finite-geometry site will, I hope,
display the above attributes. It is intended
to have a more discursive approach than
my current files at finitegeometry.org/sc.
Comments Off on Focus and Clarity in the New Year
Monday, December 28, 2015
ART WARS Continues
Combining two headlines from this morning’s
New York Times and Washington Post , we have…
Deceptively Simple Geometries
on a Bold Scale
Voilà —
Click image for details.
More generally, see
Boole vs. Galois.
Comments Off on ART WARS Continues
Friday, December 25, 2015
Dark Symbol
Related material:
The previous post (Bright Symbol) and
a post from Wednesday,
December 23, 2015, that links to posts
on Boolean algebra vs. Galois geometry.
"An analogy between mathematics and religion is apposite."
— Harvard Magazine review by Avner Ash of
Mathematics without Apologies
(Princeton University Press, January 18, 2015)
Comments Off on Dark Symbol
Wednesday, December 23, 2015
Splitting Apart
Comments Off on Splitting Apart
Sunday, December 13, 2015
The Monster as Big as the Ritz
"The colorful story of this undertaking begins with a bang."
— Martin Gardner on the death of Évariste Galois
Comments Off on The Monster as Big as the Ritz
Monday, December 7, 2015
Wittgenstein Illustrated
From "AMNESIA: VARIOUS, LUMINOUS, FIXED,"
An exhibition by Joseph Kosuth at
Sprüth Magers Gallery London,
NOVEMBER 26 2014 – FEBRUARY 14 2015 —
This journal, NOVEMBER 26 2014 –
|
Wednesday, November 26, 2014
Mathematics and Narrative
|
This journal, FEBRUARY 14 2015 —
Comments Off on Wittgenstein Illustrated
Tuesday, December 1, 2015
The Nutshell
See a search for Nocciolo in this journal.
An image from that search —
Recall also Hamlet's
"O God… bad dreams."
Comments Off on The Nutshell
Pascal’s Finite Geometry
See a search for "large Desargues configuration" in this journal.
The 6 Jan. 2015 preprint "Danzer's Configuration Revisited,"
by Boben, Gévay, and Pisanski, places this configuration,
which they call the Cayley-Salmon configuration , in the
interesting context of Pascal's Hexagrammum Mysticum .
They show how the Cayley-Salmon configuration is, in a sense,
dual to something they call the Steiner-Plücker configuration .
This duality appears implicitly in my note of April 26, 1986,
"Picturing the smallest projective 3-space." The six-sets at
the bottom of that note, together with Figures 3 and 4
of Boben et. al. , indicate how this works.
The duality was, as they note, previously described in 1898.
Related material on six-set geometry from the classical literature—
Baker, H. F., "Note II: On the Hexagrammum Mysticum of Pascal,"
in Principles of Geometry , Vol. II, Camb. U. Press, 1930, pp. 219-236
Richmond, H. W., "The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen (1900), Volume 53, Issue 1-2, pp 161-176
Richmond, H. W., "On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics , Vol. 31 (1900), pp. 125-160
Related material on six-set geometry from a more recent source —
Cullinane, Steven H., "Classical Geometry in Light of Galois Geometry," webpage
Comments Off on Pascal’s Finite Geometry
Friday, November 20, 2015
Anticommuting Dirac Matrices as Skew Lines
(Continued from November 13)
The work of Ron Shaw in this area, ca. 1994-1995, does not
display explicitly the correspondence between anticommutativity
in the set of Dirac matrices and skewness in a line complex of
PG(3,2), the projective 3-space over the 2-element Galois field.
Here is an explicit picture —
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Shaw, Ron, "Finite Geometry, Dirac Groups, and the Table of
Real Clifford Algebras," undated article at ResearchGate.net
Update of November 23:
See my post of Nov. 23 on publications by E. M. Bruins
in 1949 and 1959 on Dirac matrices and line geometry,
and on another author who gives some historical background
going back to Eddington.
Some more-recent related material from the Slovak school of
finite geometry and quantum theory —
The matrices underlying the Saniga paper are those of Pauli, not
those of Dirac, but these two sorts of matrices are closely related.
Comments Off on Anticommuting Dirac Matrices as Skew Lines
Monday, November 2, 2015
The Devil’s Offer
This is a sequel to the previous post and to the Oct. 24 post
Two Views of Finite Space. From the latter —
” ‘All you need to do is give me your soul:
give up geometry and you will have this
marvellous machine.’ (Nowadays you
can think of it as a computer!) “
Comments Off on The Devil’s Offer
Colorful Story
"The office of color in the color line
is a very plain and subordinate one.
It simply advertises the objects of
oppression, insult, and persecution.
It is not the maddening liquor, but
the black letters on the sign
telling the world where it may be had."
— Frederick Douglass, "The Color Line,"
The North American Review , Vol. 132,
No. 295, June 1881, page 575
Or gold letters.
From a search for Seagram in this journal —
"The colorful story of this undertaking begins with a bang."
— Martin Gardner on the death of Évariste Galois
Comments Off on Colorful Story
Saturday, October 31, 2015
Raiders of the Lost Crucible
Stanford Encyclopedia of Philosophy
on the date Friday, April 5, 2013 —
“First published Tue Sep 24, 1996;
substantive revision Fri Apr 5, 2013”
This journal on the date Friday, April 5, 2013 —
The object most closely resembling a “philosophers’ stone”
that I know of is the eightfold cube .
For some related philosophical remarks that may appeal
to a general Internet audience, see (for instance) a website
by I Ching enthusiast Andreas Schöter that displays a labeled
eightfold cube in the form of a lattice diagram —
Related material by Schöter —
A 20-page PDF, “Boolean Algebra and the Yi Jing.”
(First published in The Oracle: The Journal of Yijing Studies ,
Vol 2, No 7, Summer 1998, pp. 19–34.)
I differ with Schöter’s emphasis on Boolean algebra.
The appropriate mathematics for I Ching studies is,
I maintain, not Boolean algebra but rather Galois geometry.
See last Saturday’s post Two Views of Finite Space.
Unfortunately, that post is, unlike Schöter’s work, not
suitable for a general Internet audience.
Comments Off on Raiders of the Lost Crucible
Monday, October 26, 2015
Art and Theology
Yesterday's nonsense from The New York Times suggests
a better example of cultural criticism is needed. Try this …
The opening paragraph of "The many faces of Pablo Picasso,"
by Peter Conrad, at theguardian.com on Saturday,
7 February, 2009, 19.01 EST* —
"Picasso," the surrealist poet Paul Eluard said,
"paints like God or the devil." Picasso favoured
the first option. "I am God," he was once heard
telling himself. He muttered the mantra three
times, boasting of his power to animate and
enliven the visible world. Any line drawn by
his hand pulsed with vitality; when he looked
at it, a bicycle seat and its handlebar could
suddenly turn into the horned head of a bull.
But he also took a diabolical pleasure in
warping appearances, deforming faces and
twisting bodies, subjecting reality to a
tormenting inquisition.
As noted here, yesterday was the birth date (in 1811) of Galois.
It was also the birth date (in 1881) of Picasso.
Related material from the 2009 date* of the Conrad article —
The Log24 post "Childish Things." For those who deeply
dislike Picasso, there is also an 1880 opening illustration
to Hans Christian Andersen's The Snow Queen —
Story the First,
Which Describes a Looking-Glass
and the Broken Fragments
"You must attend to the commencement
of this story, for when we get to the end
we shall know more than we do now about
a very wicked hobgoblin; he was one of the
very worst, for he was a real demon."
Houghton Mifflin edition of 1880, Riverside Press, Cambridge
Click the above illustration for related posts in this journal.
* Also dated the following day, to correspond to the 00.01 GMT publication
time of The Guardian 's Sunday version, The Observer , in which it appeared.
Comments Off on Art and Theology
Sunday, October 25, 2015
Celtic Cross
The above illustrations are
from posts tagged
"Universe of Discourse."
Happy birthday to Évariste Galois, who may
prefer a mathematical, not religious,
interpretation of the above Celtic cross.
Comments Off on Celtic Cross
Saturday, October 24, 2015
Two Views of Finite Space
The following slides are from lectures on “Advanced Boolean Algebra” —
The small Boolean spaces above correspond exactly to some small
Galois spaces. These two names indicate approaches to the spaces
via Boolean algebra and via Galois geometry .
A reading from Atiyah that seems relevant to this sort of algebra
and this sort of geometry —
” ‘All you need to do is give me your soul: give up geometry
and you will have this marvellous machine.’ (Nowadays you
can think of it as a computer!) “
Related material — The article “Diamond Theory” in the journal
Computer Graphics and Art , Vol. 2 No. 1, February 1977. That
article, despite the word “computer” in the journal’s title, was
much less about Boolean algebra than about Galois geometry .
For later remarks on diamond theory, see finitegeometry.org/sc.
Comments Off on Two Views of Finite Space
Wednesday, October 21, 2015
Algebra and Space
"Perhaps an insane conceit …." Perhaps.
Related remarks on algebra and space —
"The Quality Without a Name" (Log24, August 26, 2015).
Comments Off on Algebra and Space
Sunday, October 18, 2015
Sunday School
A Unified Field —
Click the above image for further details.
See also a search in this journal for Jorie Graham.
Related dramatic dialogue for Emma Stone and
Joaquin Phoenix, actors in "Irrational Man" —
"Are you aware of what's going on at that table?"
Philosophical backstory by Hans Christian Andersen —
"He was quite frightened, and he tried to repeat the Lord's Prayer;
but all he could do, he was only able to remember the multiplication table."
Comments Off on Sunday School
Sunday, September 6, 2015
Elementally, My Dear Watson
Sarah Larson in the online New Yorker on Sept. 3, 2015,
discussed Google’s new parent company, “Alphabet”—
“… Alphabet takes our most elementally wonderful
general-use word—the name of the components of
language itself*—and reassigns it, like the words
tweet, twitter, vine, facebook, friend, and so on,
into a branded realm.”
Emma Watson in “The Bling Ring”
This journal, also on September 3 —
| Thursday, September 3, 2015
Filed under: Uncategorized — m759 @ 7:20 AM For the title, see posts from August 2007 Related theological remarks: Boolean spaces (old) vs. Galois spaces (new) in |
* Actually, Sarah, that would be “phonemes.”
Comments Off on Elementally, My Dear Watson
Friday, September 4, 2015
Thursday, September 3, 2015
Rings of August
For the title, see posts from August 2007 tagged Gyges.
Related theological remarks:
Boolean spaces (old) vs. Galois spaces (new) in
“The Quality Without a Name”
(a post from August 26, 2015) and the…
Related literature: A search for Borogoves in this journal will yield
remarks on the 1943 tale underlying the above film.
Comments Off on Rings of August
Wednesday, August 26, 2015
“The Quality Without a Name”
The title phrase, paraphrased without quotes in
the previous post, is from Christopher Alexander's book
The Timeless Way of Building (Oxford University Press, 1979).
A quote from the publisher:
"Now, at last, there is a coherent theory
which describes in modern terms
an architecture as ancient as
human society itself."
Three paragraphs from the book (pp. xiii-xiv):
19. Within this process, every individual act
of building is a process in which space gets
differentiated. It is not a process of addition,
in which preformed parts are combined to
create a whole, but a process of unfolding,
like the evolution of an embryo, in which
the whole precedes the parts, and actualy
gives birth to then, by splitting.
20. The process of unfolding goes step by step,
one pattern at a time. Each step brings just one
pattern to life; and the intensity of the result
depends on the intensity of each one of these
individual steps.
21. From a sequence of these individual patterns,
whole buildings with the character of nature
will form themselves within your thoughts,
as easily as sentences.
Compare to, and contrast with, these illustrations of "Boolean space":
(See also similar illustrations from Berkeley and Purdue.)
Detail of the above image —
Note the "unfolding," as Christopher Alexander would have it.
These "Boolean" spaces of 1, 2, 4, 8, and 16 points
are also Galois spaces. See the diamond theorem —
Comments Off on “The Quality Without a Name”
Friday, August 21, 2015
Finite Geometry at GitHub
(This post was updated on Jan. 10, 2016.)
See galois.io .
(That URL forwards to http://finite-geometry.github.io/galois/ .)
Recommended for editing: c9.io .
Comments Off on Finite Geometry at GitHub
Saturday, August 15, 2015
Schoolboy Problem
Sir Laurence Olivier, in "Term of Trial" (1962), dangles
a participle in front of schoolboy Terence Stamp:
"Walking to school today
my arithmetic book
fell into the gutter"
Were Stamp a Galois, the reply might be "Try this one, sir."
Comments Off on Schoolboy Problem
Friday, August 14, 2015
Discrete Space
(A review)
Galois space:
Counting symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
Comments Off on Discrete Space
Thursday, August 13, 2015
Anniversary
Today is reportedly the anniversary of the death,
in Paris in 1822, of Jean Robert Argand.
Some related material …
"Wessel's fame as a mathematician rests solely
on this paper, which was published in 1799,
giving for the first time a geometrical interpretation
of complex numbers. Today we call this geometric
interpretation the Argand diagram but Wessel's
work came first. It was rediscovered by Argand
in 1806 and again by Gauss in 1831. ….
Of course it is not unreasonable to call the
geometrical interpretation of complex numbers
the Argand diagram since it was Argand's work
which was influential. It was so named before
the world of mathematics learnt of Wessel's prior
publication. In fact Wessel's paper was not
noticed by the mathematical community until 1895…."
See also Tilting at Whirligigs (Log24 on March 8, 2008)
and The Galois Quaternion.
Comments Off on Anniversary
Sunday, July 19, 2015
Lying Rhyme
|
Tom Stoppard, Jumpers —
“Heaven, how can I believe in Heaven?”
“To begin at the beginning: Is God?…” “Leave a space.” |
See as well a search for "Heaven.gif" in this journal.
For the more literate among us —
… and the modulation from algebra to space.
Comments Off on Lying Rhyme
Thursday, July 9, 2015
Man and His Symbols
A post of July 7, Haiku for DeLillo, had a link to posts tagged "Holy Field GF(3)."
As the smallest Galois field based on an odd prime, this structure
clearly is of fundamental importance.
It is, however, perhaps too small to be visually impressive.
A larger, closely related, field, GF(9), may be pictured as a 3×3 array…
|
… hence as the traditional Chinese Holy Field.
Marketing the Holy Field
The above illustration of China's Holy Field occurred in the context of
Log24 posts on Child Buyers. For more on child buyers, see an excellent
condemnation today by Diane Ravitch of the U. S. Secretary of Education.
Comments Off on Man and His Symbols
Friday, June 19, 2015
Footnote
There is such a thing as geometry.*
* Proposition adapted from A Wrinkle in Time , by Madeleine L'Engle.
Comments Off on Footnote
Wednesday, June 17, 2015
Slow Art, Continued
The title of the previous post, "Slow Art," is a phrase
of the late art critic Robert Hughes.
Example from mathematics:
-
Göpel tetrads as subsets of a 4×4 square in the classic
1905 book Kummer's Quartic Surface by R. W. H. T. Hudson.
These subsets were constructed as helpful schematic diagrams,
without any reference to the concept of finite geometry they
were later to embody.
-
Göpel tetrads (not named as such), again as subsets of
a 4×4 square, that form the 15 isotropic projective lines of the
finite projective 3-space PG(3,2) in a note on finite geometry
from 1986 —
-
Göpel tetrads as these figures of finite geometry in a 1990
foreword to the reissued 1905 book of Hudson:
Click the Barth passage to see it with its surrounding text.
Related material:
Comments Off on Slow Art, Continued
Monday, June 15, 2015
Omega Matrix
See that phrase in this journal.
See also last night's post.
The Greek letter Ω is customarily used to
denote a set that is acted upon by a group.
If the group is the affine group of 322,560
transformations of the four-dimensional
affine space over the two-element Galois
field, the appropriate Ω is the 4×4 grid above.
Comments Off on Omega Matrix
Thursday, June 11, 2015
Omega
Omega is a Greek letter, Ω , used in mathematics to denote
a set on which a group acts.
For instance, the affine group AGL(3,2) is a group of 1,344
actions on the eight elements of the vector 3-space over the
two-element Galois field GF(2), or, if you prefer, on the Galois
field Ω = GF(8).
Related fiction: The Eight , by Katherine Neville.
Related non-fiction: A remark by Werner Heisenberg
in this journal on Saturday, June 6, 2015, the eightfold cube ,
and the illustrations below —
|
Mathematics
The Fano plane block design |
Magic
The Deathly Hallows symbol— |
Comments Off on Omega
Tuesday, June 9, 2015
Colorful Song
For geeks* —
" Domain, Domain on the Range , "
where Domain = the Galois tesseract and
Range = the four-element Galois field.
This post was suggested by the previous post,
by a Log24 search for Knight + Move, and by
the phrase "discouraging words" found in that search.
* A term from the 1947 film "Nightmare Alley."
Comments Off on Colorful Song
Tuesday, June 2, 2015
Miniature Lowry
A review describes the main character in the 1981 novel
The Knife in My Hands —
"His other peculiar characteristic is a fascination, developed during
his university years, with the Cabala and, like a miniature Lowry,*
he spends much of his time wandering drunkenly through its
labyrinthine mysteries."
AA motto: "Principles before personalities."
* Related material: Back to the Real.
Comments Off on Miniature Lowry
Sunday, May 31, 2015
Wednesday, May 13, 2015
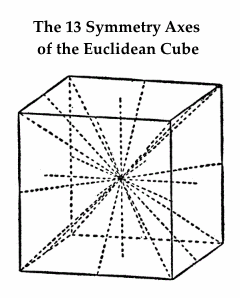
Space
Notes on space for day 13 of May, 2015 —
The 13 symmetry axes of the cube may be viewed as
the 13 points of the Galois projective space PG(2,3).
This space (a plane) may also be viewed as the nine points
of the Galois affine space AG(2,3) plus the four points on
an added "line at infinity."
Related poetic material:
The ninefold square and Apollo, as well as …
Comments Off on Space
Thursday, April 16, 2015
National Library Week
"Celebrate National Library Week 2015 (April 12-18, 2015)
with the theme "Unlimited possibilities @ your library®."
See also Library of Hell.
A page from Princeton University Press on March 18, 2012:
… "mathematics and narrative…." (top of page xvii).
I prefer the interplay of Euclidean and Galois mathematics.
Comments Off on National Library Week
Thursday, March 26, 2015
The Möbius Hypercube
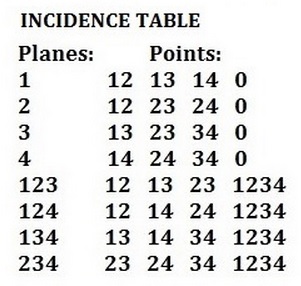
The incidences of points and planes in the
Möbius 84 configuration (8 points and 8 planes,
with 4 points on each plane and 4 planes on each point),
were described by Coxeter in a 1950 paper.*
A table from Monday's post summarizes Coxeter's
remarks, which described the incidences in
spatial terms, with the points and planes as the vertices
and face-planes of two mutually inscribed tetrahedra —
Monday's post, "Gallucci's Möbius Configuration,"
may not be completely intelligible unless one notices
that Coxeter has drawn some of the intersections in his
Fig. 24, a schematic representation of the point-plane
incidences, as dotless, and some as hollow dots. The figure,
"Gallucci's version of Möbius's 84," is shown below.
The hollow dots, representing the 8 points (as opposed
to the 8 planes ) of the configuration, are highlighted in blue.
Here a plane (represented by a dotless intersection) contains
the four points that are represented in the square array as lying
in the same row or same column as the plane.
The above Möbius incidences appear also much earlier in
Coxeter's paper, in figures 6 and 5, where they are shown
as describing the structure of a hypercube.
In figures 6 and 5, the dotless intersections representing
planes have been replaced by solid dots. The hollow dots
have again been highlighted in blue.
Figures 6 and 5 demonstrate the fact that adjacency in the set of
16 vertices of a hypercube is isomorphic to adjacency in the set
of 16 subsquares of a square 4×4 array, provided that opposite
sides of the array are identified, as in Fig. 6. The digits in
Coxeter's labels above may be viewed as naming the positions
of the 1's in (0,1) vectors (x4, x3, x2, x1) over the two-element
Galois field.† In that context, the 4×4 array may be called, instead
of a Möbius hypercube , a Galois tesseract .
* "Self-Dual Configurations and Regular Graphs,"
Bulletin of the American Mathematical Society,
Vol. 56 (1950), pp. 413-455
† The subscripts' usual 1-2-3-4 order is reversed as a reminder
that such a vector may be viewed as labeling a binary number
from 0 through 15, or alternately as labeling a polynomial in
the 16-element Galois field GF(24). See the Log24 post
Vector Addition in a Finite Field (Jan. 5, 2013).
Comments Off on The Möbius Hypercube
Tuesday, March 24, 2015
Hirzebruch
(Continued from July 16, 2014.)
Some background from Wikipedia:
"Friedrich Ernst Peter Hirzebruch ForMemRS[2]
(17 October 1927 – 27 May 2012)
was a German mathematician, working in the fields of topology,
complex manifolds and algebraic geometry, and a leading figure
in his generation. He has been described as 'the most important
mathematician in Germany of the postwar period.'
[3][4][5][6][7][8][9][10][11]"
A search for citations of the A. E. Brouwer paper in
the previous post yields a quotation from the preface
to the third ("2013") edition of Wolfgang Ebeling's
Lattices and Codes: A Course Partially Based
on Lectures by Friedrich Hirzebruch , a book
reportedly published on September 19, 2012 —
|
"Sadly, on May 27 this year, Friedrich Hirzebruch, Hannover, July 2012 Wolfgang Ebeling "
(Prof. Dr. Wolfgang Ebeling, Institute of Algebraic Geometry, |
Also sadly …
Comments Off on Hirzebruch
Monday, March 23, 2015
Gallucci’s Möbius Configuration
From H. S. M. Coxeter's 1950 paper
"Self-Dual Configurations and Regular Graphs,"
a 4×4 array and a more perspicuous rearrangement—
(Click image to enlarge.)
The above rearrangement brings Coxeter's remarks into accord
with the webpage The Galois Tesseract.
Update of Thursday, March 26, 2015 —
For an explanation of Coxeter's Fig. 24, see Thursday's later
post titled "The Möbius Hypercube."
Comments Off on Gallucci’s Möbius Configuration
Monday, February 16, 2015
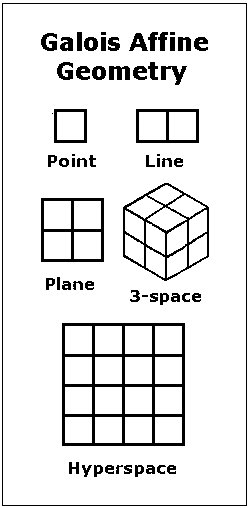
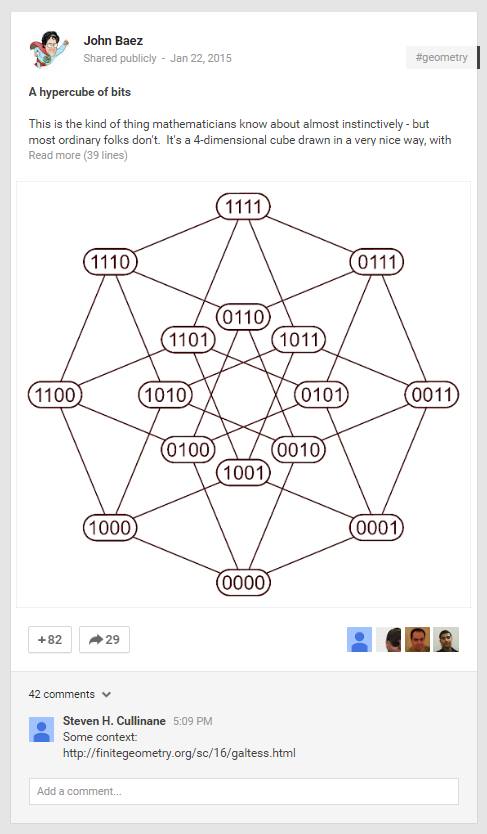
Monday, January 26, 2015
Savior for Atheists…
Continued from June 17, 2013
(John Baez as a savior for atheists):
As an atheists-savior, I prefer Galois…
The geometry underlying a figure that John Baez
posted four days ago, "A Hypercube of Bits," is
Galois geometry —
See The Galois Tesseract and an earlier
figure from Log24 on May 21, 2007:
For the genesis of the figure,
see The Geometry of Logic.
Comments Off on Savior for Atheists…
Monday, January 5, 2015
Gitterkrieg*
Wednesday, March 13, 2013
|
"I pondered deeply, then, over the
adventures of the jungle. And after
some work with a colored pencil
I succeeded in making my first drawing.
My Drawing Number One.
It looked something like this:
I showed my masterpiece to the
grown-ups, and asked them whether
the drawing frightened them.
But they answered: 'Why should
anyone be frightened by a hat?'"
* For the title, see Plato Thanks the Academy (Jan. 3).
Comments Off on Gitterkrieg*
Thursday, December 18, 2014
Platonic Analogy
(Five by Five continued)
As the 3×3 grid underlies the order-3 finite projective plane,
whose 13 points may be modeled by
the 13 symmetry axes of the cube,
so the 5×5 grid underlies the order-5 finite projective plane,
whose 31 points may be modeled by
the 31 symmetry axes of the dodecahedron.
See posts tagged Galois-Plane Models.
Comments Off on Platonic Analogy
Friday, December 5, 2014
Wittgenstein’s Picture
From Zettel (repunctuated for clarity):
249. « Nichts leichter, als sich einen 4-dimensionalen Würfel
vorstellen! Er schaut so aus… »
"Nothing easier than to imagine a 4-dimensional cube!
It looks like this…
[Here the editor supplied a picture of a 4-dimensional cube
that was omitted by Wittgenstein in the original.]
« Aber das meine ich nicht, ich meine etwas wie…
"But I don't mean that, I mean something like…
…nur mit 4 Ausdehnungen! »
but with four dimensions!
« Aber das ist nicht, was ich dir gezeigt habe,
eben etwas wie…
"But isn't what I showed you like…
…nur mit 4 Ausdehnungen? »
…only with four dimensions?"
« Nein; das meine ich nicht! »
"No, I don't mean that!"
« Was aber meine ich? Was ist mein Bild?
Nun der 4-dimensionale Würfel, wie du ihn gezeichnet hast,
ist es nicht ! Ich habe jetzt als Bild nur die Worte und
die Ablehnung alles dessen, was du mir zeigen kanst. »
"But what do I mean? What is my picture?
Well, it is not the four-dimensional cube
as you drew it. I have now for a picture only
the words and my rejection of anything
you can show me."
"Here's your damn Bild , Ludwig —"
Context: The Galois Tesseract.
Comments Off on Wittgenstein’s Picture
Wednesday, November 26, 2014
Mathematics and Narrative
Mathematics: Galois-Plane Models.
Narrative: "The Dreaming Jewels."
Comments Off on Mathematics and Narrative
A Tetrahedral Fano-Plane Model
Update of Nov. 30, 2014 —
It turns out that the following construction appears on
pages 16-17 of A Geometrical Picture Book , by
Burkard Polster (Springer, 1998).
"Experienced mathematicians know that often the hardest
part of researching a problem is understanding precisely
what that problem says. They often follow Polya's wise
advice: 'If you can't solve a problem, then there is an
easier problem you can't solve: find it.'"
—John H. Conway, foreword to the 2004 Princeton
Science Library edition of How to Solve It , by G. Polya
For a similar but more difficult problem involving the
31-point projective plane, see yesterday's post
"Euclidean-Galois Interplay."
The above new [see update above] Fano-plane model was
suggested by some 1998 remarks of the late Stephen Eberhart.
See this morning's followup to "Euclidean-Galois Interplay"
quoting Eberhart on the topic of how some of the smallest finite
projective planes relate to the symmetries of the five Platonic solids.
Update of Nov. 27, 2014: The seventh "line" of the tetrahedral
Fano model was redefined for greater symmetry.
Comments Off on A Tetrahedral Fano-Plane Model
Class Act
Update of Nov. 30, 2014 —
For further information on the geometry in
the remarks by Eberhart below, see
pp. 16-17 of A Geometrical Picture Book ,
by Burkard Polster (Springer, 1998). Polster
cites a different article by Lemay.
A search for background to the exercise in the previous post
yields a passage from the late Stephen Eberhart:
|
The first three primes p = 2, 3, and 5 therefore yield finite projective planes with 7, 13, and 31 points and lines, respectively. But these are just the numbers of symmetry axes of the five regular solids, as described in Plato's Timaeus : The tetrahedron has 4 pairs of face planes and corner points + 3 pairs of opposite edges, totalling 7 axes; the cube has 3 pairs of faces + 6 pairs of edges + 4 pairs of corners, totalling 13 axes (the octahedron simply interchanges the roles of faces and corners); and the pentagon dodecahedron has 6 pairs of faces + 15 pairs of edges + 10 pairs of corners, totalling 31 axes (the icosahedron again interchanging roles of faces and corners). This is such a suggestive result, one would expect to find it dealt with in most texts on related subjects; instead, while "well known to those who well know such things" (as Richard Guy likes to quip), it is scarcely to be found in the formal literature [9]. The reason for the common numbers, it turns out, is that the groups of symmetry motions of the regular solids are subgroups of the groups of collineations of the respective finite planes, a face axis being different from an edge axis of a regular solid but all points of a projective plane being alike, so the latter has more symmetries than the former. [9] I am aware only of a series of in-house publications by Fernand Lemay of the Laboratoire de Didactique, Faculté des Sciences de I 'Éducation, Univ. Laval, Québec, in particular those collectively titled Genèse de la géométrie I-X.
— Stephen Eberhart, Dept. of Mathematics, |
Eberhart died of bone cancer in 2003. A memorial by his
high school class includes an Aug. 7, 2003, transcribed
letter from Eberhart to a classmate that ends…
|
… I earned MA’s in math (UW, Seattle) and history (UM, Missoula) where a math/history PhD program had been announced but canceled. So 1984 to 2002 I taught math (esp. non-Euclidean geometry) at C.S.U. Northridge. It’s been a rich life. I’m grateful. Steve |
See also another informative BRIDGES paper by Eberhart
on mathematics and the seven traditional liberal arts.
Comments Off on Class Act
Friday, November 14, 2014
Another Opening, Another Show
“What happens when you mix the brilliant wit of Noel Coward
with the intricate plotting of Agatha Christie? Set during a
weekend in an English country manor in 1932, Death by Design
is a delightful and mysterious ‘mash-up’ of two of the greatest
English writers of all time. Edward Bennett, a playwright, and
his wife Sorel Bennett, an actress, flee London and head to
Cookham after a disastrous opening night. But various guests
arrive unexpectedly….”
— Samuel French (theatrical publisher) on a play that
opened in Houston on September 9, 2011.
Related material:
- This journal on May 28, 2014,
- on October 7, 2014,
- on November 13, 2014,
- and on the above opening night, September 9, 2011.
Comments Off on Another Opening, Another Show
Friday, October 31, 2014
Structure
Introducing a group of 322,560 affine transformations of Dürer’s ‘Magic’ Square
The four vector-space substructures of digits in 1st, 2nd, 3rd, 4th place,
together with the diamond theorem, indicate that Dürer’s square “minus one”
can be transformed by permutations of rows, columns, and quadrants to a
square with (decimal) digits in the usual numerical order, increasing from
top left to bottom right. Such permutations form a group of order 322,560.
(Continued from Vector Addition in a Finite Field, Twelfth Night, 2013.)
Comments Off on Structure
Monday, October 27, 2014
Revolutions in Geometry
A post in honor of Évariste Galois (25 October 1811 – 31 May 1832)
From a book by Richard J. Trudeau titled The Non-Euclidean Revolution —
See also “non-Euclidean” in this journal.
One might argue that Galois geometry, a field ignored by Trudeau,
is also “non-Euclidean,” and (for those who like rhetoric) revolutionary.
Comments Off on Revolutions in Geometry
Sunday, October 26, 2014
Centennial
The "Chern" of today's previous post is mathematician
Shiing-Shen Chern (b. Oct. 26, 1911, d. Dec. 3, 2004).
For an observance of the 2011 centennial of his birth,
see a website in China.
See also this journal on the centennial date —
Erlanger and Galois, a post of Oct. 26, 2011.
Comments Off on Centennial
Saturday, October 25, 2014
Foundation Square
In the above illustration of the 3-4-5 Pythagorean triangle,
the grids on each side may be regarded as figures of
Euclidean geometry or of Galois geometry.
In Euclidean geometry, these grids illustrate a property of
the inner triangle.
In elementary Galois geometry, ignoring the connection with
the inner triangle, the grids may be regarded instead as
illustrating vector spaces over finite (i.e., Galois) fields.
Previous posts in this journal have dealt with properties of
the 3×3 and 4×4 grids. This suggests a look at properties of
the next larger grid, the 5×5 array, viewed as a picture of the
two-dimensional vector space (or affine plane) over the finite
Galois field GF(5) (also known as ℤ5).
The 5×5 array may be coordinatized in a natural way, as illustrated
in (for instance) Matters Mathematical , by I.N. Herstein and
Irving Kaplansky, 2nd ed., Chelsea Publishing, 1978, p. 171:
See Herstein and Kaplansky for the elementary Galois geometry of
the 5×5 array.
For 5×5 geometry that is not so elementary, see…
-
"The Hoffman-Singleton Graph and its Automorphisms," by
Paul R. Hafner, Journal of Algebraic Combinatorics , 18 (2003), 7–12, and -
the Web pages "Hoffman-Singleton Graph" and "Higman-Sims Graph"
of A. E. Brouwer.
Hafner's abstract:
We describe the Hoffman-Singleton graph geometrically, showing that
it is closely related to the incidence graph of the affine plane over ℤ5.
This allows us to construct all automorphisms of the graph.
The remarks of Brouwer on graphs connect the 5×5-related geometry discussed
by Hafner with the 4×4 geometry related to the Steiner system S(5,8,24).
(See the Miracle Octad Generator of R. T. Curtis and the related coordinatization
by Cullinane of the 4×4 array as a four-dimensional vector space over GF(2).)
Comments Off on Foundation Square
Tuesday, October 21, 2014
Eerie Twist
(Continued from Nov. 15, 2011)
Ben Bradlee, legendary Washington Post editor, dies at 93
See also a post of Jan. 20, 2011, and an earlier post on Twelfth Night, 2010.
A star figure and the Galois quaternion.
The square root of the former is the latter.
Comments Off on Eerie Twist
Tools
(Night at the Museum continues.)
"Strategies for making or acquiring tools
While the creation of new tools marked the route to developing the social sciences,
the question remained: how best to acquire or produce those tools?"
— Jamie Cohen-Cole, “Instituting the Science of Mind: Intellectual Economies
and Disciplinary Exchange at Harvard’s Center for Cognitive Studies,”
British Journal for the History of Science vol. 40, no. 4 (2007): 567-597.
Obituary of a co-founder, in 1960, of the Center for Cognitive Studies at Harvard:
"Disciplinary Exchange" —
In exchange for the free Web tools of HTML and JavaScript,
some free tools for illustrating elementary Galois geometry —
The Kaleidoscope Puzzle, The Diamond 16 Puzzle,
The 2x2x2 Cube, and The 4x4x4 Cube
"Intellectual Economies" —
In exchange for a $10 per month subscription, an excellent
"Quilt Design Tool" —
This illustrates not geometry, but rather creative capitalism.
Related material from the date of the above Harvard death: Art Wars.
Comments Off on Tools
Saturday, October 18, 2014
Educational Series
Barron's Educational Series (click to enlarge):
The Tablet of Ahkmenrah:
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Another educational series (this journal):
Art theorist Rosalind Krauss and The Ninefold Square
Comments Off on Educational Series
Wednesday, October 1, 2014
Big Time
The following post suggests the Spiders and Snakes of Fritz Leiber’s
Changewar , a mythology inspired by the hallucinations of delirium tremens .
Comments Off on Big Time
Monday, September 22, 2014
Sunday, September 21, 2014
Uncommon Noncore
This post was suggested by Greg Gutfeld’s Sept. 4 remarks on Common Core math.
Problem: What is 9 + 6 ?
Here are two approaches suggested by illustrations of Desargues’s theorem.
Solution 1:
9 + 6 = 10 + 5,
as in Common Core (or, more simply, as in common sense), and
10 + 5 = 5 + 10 = 15 as in Veblen and Young:
Solution 2:
In the figure below,
9 + 6 = no. of V’s + no. of A’s + no. of C’s =
no. of nonempty squares = 16 – 1 = 15.
(Illustration from Feb. 10, 2014.)
The silly educationists’ “partner, anchor, decompose” jargon
discussed by Gutfeld was their attempt to explain “9 + 6 = 10 + 5.”
As he said of the jargon, “That’s not math, that’s the plot from ‘Silence of the Lambs.'”
Or from Richard, Frank, and Marcus in last night’s “Intruders”
(BBC America, 10 PM).
Comments Off on Uncommon Noncore
Sunday, September 14, 2014
Sensibility
Structured gray matter:
Graphic symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
Comments Off on Sensibility
Sunday, August 31, 2014
Sunday School
The Folding
Cynthia Zarin in The New Yorker , issue dated April 12, 2004—
“Time, for L’Engle, is accordion-pleated. She elaborated,
‘When you bring a sheet off the line, you can’t handle it
until it’s folded, and in a sense, I think, the universe can’t
exist until it’s folded — or it’s a story without a book.’”
The geometry of the 4×4 square array is that of the
3-dimensional projective Galois space PG(3,2).
This space occurs, notably, in the Miracle Octad Generator (MOG)
of R. T. Curtis (submitted to Math. Proc. Camb. Phil. Soc. on
15 June 1974). Curtis did not, however, describe its geometric
properties. For these, see the Cullinane diamond theorem.
Some history:
Curtis seems to have obtained the 4×4 space by permuting,
then “folding” 1×8 binary sequences into 4×2 binary arrays.
The original 1×8 sequences came from the method of Turyn
(1967) described by van Lint in his book Coding Theory
(Springer Lecture Notes in Mathematics, No. 201 , first edition
published in 1971). Two 4×2 arrays form each 4×4 square array
within the MOG. This construction did not suggest any discussion
of the geometric properties of the square arrays.
[Rewritten for clarity on Sept. 3, 2014.]
Comments Off on Sunday School
Saturday, August 30, 2014
Physics and Theology
The titles of the previous three posts refer to
Hermann Weyl’s 1918 book Raum, Zeit, Materie
(Space, Time, Matter).
This suggests a look at a poetically parallel 1950 title —
The Lion, the Witch and the Wardrobe —
and at its underlying philosophy:
I am among “those who do not know that this great myth became Fact.”
I do, however, note that some other odd things have become fact.
Those who wish more on this topic may consult:
- The Metaphysics of the Incarnation , from
Oxford University Press on Epiphany 2011. - Epiphany Riddle, from that same date.
- Incarnation in this journal.
Comments Off on Physics and Theology
Thursday, July 31, 2014
Zero System
The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and
"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."
See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.
An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):
See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.
Comments Off on Zero System
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Comments Off on Sunday School
Thursday, July 17, 2014
Paradigm Shift:
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Comments Off on Paradigm Shift:
Wednesday, July 16, 2014
Saturday, July 12, 2014
Sequel
A sequel to the 1974 film
Thunderbolt and Lightfoot :
Contingent and Fluky
Some variations on a thunderbolt theme:
These variations also exemplify the larger
Verbum theme:
A search today for Verbum in this journal yielded
a Georgetown University Chomskyite, Professor
David W. Lightfoot.
"Dr. Lightfoot writes mainly on syntactic theory,
language acquisition and historical change, which
he views as intimately related. He argues that
internal language change is contingent and fluky,
takes place in a sequence of bursts, and is best
viewed as the cumulative effect of changes in
individual grammars, where a grammar is a
'language organ' represented in a person's
mind/brain and embodying his/her language
faculty."
Some syntactic work by another contingent and fluky author
is related to the visual patterns illustrated above.
See Tecumseh Fitch in this journal.
For other material related to the large Verbum cube,
see posts for the 18th birthday of Harry Potter.
That birthday was also the upload date for the following:
See esp. the comments section.
Comments Off on Sequel
Sunday, July 6, 2014
Sticks and Stones
The title is from this morning's previous post.
From a theater review in that post—
… "all flying edges and angles, a perpetually moving and hungry soul"
… "a formidably centered presence, the still counterpoint"
A more abstract perspective:
See also Desargues via Galois (August 6, 2013).
Comments Off on Sticks and Stones
Sunday, June 8, 2014
Vide
"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Comments Off on Vide
Tuesday, June 3, 2014
Robert Steinberg, 1922-2014
Galois matrices, the subject of the previous post,
are of course not new. See, for instance, Steinberg in 1951:
The American Mathematical Society reports that Steinberg died
on May 25, 2014.
As the above 1951 paper indicates, Steinberg was well acquainted with
what Weyl called "the devil of abstract algebra." In this journal, however,
Steinberg himself appears rather as an angel of geometry.
Comments Off on Robert Steinberg, 1922-2014
Friday, May 30, 2014
Combinatorial Matrix Classes
A book by this title, Richard A. Brualdi’s Combinatorial Matrix Classes ,
was published by Cambridge University Press in 2006:
For some related remarks, see The Counter (March 13, 2011).
My own work deals with combinatorial properties of matrices
of 0’s and 1’s, but in the context of Galois (i.e., finite) fields,
not the real or complex fields. Despite the generality of
their titles, Combinatorial Matrix Theory and Combinatorial
Matrix Classes do not deal with Galois matrices.
Comments Off on Combinatorial Matrix Classes
Thursday, May 22, 2014
Visual Structure
“Chaos is order yet undeciphered.”
— The novel The Double , by José Saramago,
on which the recent film "Enemy" was based
For Louise Bourgeois — a post from the date of Galois's death—
For Toronto — Scene from a film that premiered there on Sept. 8, 2013:
Related material: This journal on that date, Sept. 8, 2013:
"I still haven't found what I'm looking for." — Bono
"In fact Surrealism found what it had been looking for
from the first in the 1920 collages [by Max Ernst],
which introduced an entirely original scheme of
visual structure…."
— Rosalind Krauss quoting André Breton*
in "The Master's Bedroom"
* "Artistic Genesis and Perspective of Surrealism"
(1941), in Surrealism and Painting (New York,
Harper & Row, 1972, p. 64).
See also Damnation Morning in this journal.
Comments Off on Visual Structure
Wednesday, May 21, 2014
The Tetrahedral Model of PG(3,2)
The page of Whitehead linked to this morning
suggests a review of Polster's tetrahedral model
of the finite projective 3-space PG(3,2) over the
two-element Galois field GF(2).
|
The above passage from Whitehead's 1906 book suggests
that the tetrahedral model may be older than Polster thinks.
Shown at right below is a correspondence between Whitehead's
version of the tetrahedral model and my own square model,
based on the 4×4 array I call the Galois tesseract (at left below).
(Click to enlarge.)
Comments Off on The Tetrahedral Model of PG(3,2)
Through the Vanishing Point*
Marshall McLuhan in "Annie Hall" —
"You know nothing of my work."
Related material —
"I need a photo opportunity
I want a shot at redemption
Don't want to end up a cartoon
In a cartoon graveyard"
— Paul Simon
It was a dark and stormy night…
— Page 180, Logicomix
A photo opportunity for Whitehead
(from Romancing the Cube, April 20, 2011)—
See also Absolute Ambition (Nov. 19, 2010).
* For the title, see Vanishing Point in this journal.
Comments Off on Through the Vanishing Point*
Tuesday, May 20, 2014
Play
From a recreational-mathematics weblog yesterday:
"This appears to be the arts section of the post,
so I’ll leave Martin Probert’s page on
The Survival, Origin and Mathematics of String Figures
here. I’ll be back to pick it up at the end. Maybe it’d like
to play with Steven H. Cullinane’s pages on the
Finite Geometry of the Square and Cube."
I doubt they would play well together.
Perhaps the offensive linking of the purely recreational topic
of string figures to my own work was suggested by the
string figures' resemblance to figures of projective geometry.
A pairing I prefer: Desargues and Galois —
For further details, see posts on Desargues and Galois.
Comments Off on Play
Sunday, April 27, 2014
Sunday School
Galois and Abel vs. Rubik
"Abel was done to death by poverty, Galois by stupidity.
In all the history of science there is no completer example
of the triumph of crass stupidity…."
— Eric Temple Bell, Men of Mathematics
Gray Space (Continued)
… For The Church of Plan 9.
Comments Off on Sunday School
Thursday, March 27, 2014
Diamond Space
Definition: A diamond space — informal phrase denoting
a subspace of AG(6, 2), the six-dimensional affine space
over the two-element Galois field.
The reason for the name:
Click to enlarge.
Comments Off on Diamond Space
Friday, March 21, 2014
Three Constructions of the Miracle Octad Generator
See also a Log24 post on this subject from Dec. 14, 2013,
especially (scroll down) the update of March 9, 2014.
Related material on the Turyn-Curtis construction
from the University of Cambridge —
— Slide by "Dr. Parker" — Apparently Richard A. Parker —
Lecture 4, "Discovering M24," in slides for lectures 1-8 from lectures
at Cambridge in 2010-2011 on "Sporadic and Related Groups."
See also the Parker lectures of 2012-2013 on the same topic.
A third construction of Curtis's 35 4×6 1976 MOG arrays would use
Cullinane's analysis of the 4×4 subarrays' affine and projective structure,
and point out the fact that Conwell's 1910 correspondence of the 35
4+4-partitions of an 8-set with the 35 lines of the projective 3-space
over the 2-element field, PG(3, 2), is essentially the same correspondence
as that constituting Curtis's 1976 MOG.
See The Diamond Theorem, Finite Relativity, Galois Space,
Generating the Octad Generator, and The Klein Correspondence.
Update of March 22-March 23 —
Adding together as (0,1)-matrices over GF(2) the black parts (black
squares as 1's, all other squares as 0's) of the 35 4×6 arrays of the 1976
Curtis MOG would then reveal* the symmetric role played in octads
by what Curtis called the heavy brick , and so reveal also the action of
S3 on the three Curtis bricks that leaves invariant the set of all 759
octads of the S(5, 8, 24) constructed from the 35 MOG arrays. For more
details of this "by-hand" construction, see Geometry of the 4×4 Square.
For the mathematical properties of the S(5, 8, 24), it is convenient to
have a separate construction (such as Turyn's), not by hand, of the
extended binary Golay code. See the Brouwer preprint quoted above.
* "Then a miracle occurs," as in the classic 1977 Sidney Harris cartoon.
Illustration of array addition from March 23 —
Comments Off on Three Constructions of the Miracle Octad Generator