Monday, July 21, 2008
Knight Moves:
The Relativity Theory
of Kindergarten Blocks
(Continued from
January 16, 2008)
Something:
From Friedrich Froebel,
who invented kindergarten:

Click on image for details.
An Unusually
Complicated Theory:
From Christmas 2005:

Click on image for details.
For the eightfold cube
as it relates to Klein's
simple group, see
"A Reflection Group
of Order 168."
For an even more
complicated theory of
Klein's simple group, see

Click on image for details.
Comments Off on Monday July 21, 2008
Saturday, July 19, 2008
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in this week's New Yorker:
"
A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Hermann Weyl on the hard core of objectivity:
"Perhaps the philosophically most relevant feature of modern science is the emergence of abstract symbolic structures as the hard core of objectivity behind– as Eddington puts it– the colorful tale of the subjective storyteller mind." (Philosophy of Mathematics and Natural Science, Princeton, 1949, p. 237)
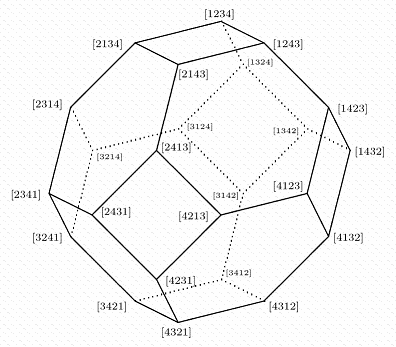
Steven H. Cullinane on the symmetries of a 4×4 array of points:
|
A Structure-Endowed Entity
"A guiding principle in modern mathematics is this lesson: Whenever you have to do with a structure-endowed entity S, try to determine its group of automorphisms, the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of S in this way."
— Hermann Weyl in Symmetry
Let us apply Weyl's lesson to the following "structure-endowed entity."

What is the order of the resulting group of automorphisms?
|
The above group of
automorphisms plays
a role in what Weyl,
following Eddington,
called a "colorful tale"–
Comments Off on Saturday July 19, 2008
Friday, July 18, 2008
Hard Core
David Corfield quotes Weyl in a weblog entry, "Hierarchy and Emergence," at the n-Category Cafe this morning:
"Perhaps the philosophically most relevant feature of modern science is the emergence of abstract symbolic structures as the hard core of objectivity behind– as Eddington puts it– the colorful tale of the subjective storyteller mind." (Philosophy of Mathematics and Natural Science [Princeton, 1949], p. 237)
For the same quotation in a combinatorial context, see the foreword by A. W. Tucker, "Combinatorial Problems," to a special issue of the IBM Journal of Research and Development, November 1960 (1-page pdf).
See also yesterday's Log24 entry.
Comments Off on Friday July 18, 2008
Thursday, July 17, 2008
CHANGE
FEW CAN BELIEVE IN
|
Continued from June 18.
Jungian Symbols
of the Self —

Compare and contrast:
Jung's four-diamond figure from
Aion — a symbol of the self —

Jung's Map of the Soul,
by Murray Stein:
"… Jung thinks of the self as undergoing continual transformation during the course of a lifetime…. At the end of his late work
Aion, Jung presents a diagram to illustrate the dynamic movements of the self…."
Comments Off on Thursday July 17, 2008
Wednesday, July 9, 2008
God, Time, Epiphany
8:28:32 AM
Anthony Hopkins, from
All Hallows' Eve
last year:
"For me time is God,
God is time. It's an equation,
like an Einstein equation."
James Joyce, from
June 26 (the day after
AntiChristmas) this year:
"… he glanced up at the clock
of the Ballast Office and smiled:
— It has not epiphanised yet,
he said."
Ezra Pound (from a page
linked to yesterday morning):
"It seems quite natural to me
that an artist should have
just as much pleasure in an
arrangement of planes
or in a pattern of figures,
as in painting portraits…."
From Epiphany 2008:
An arrangement of planes:

From May 10, 2008:
A pattern of figures:
See also Richard Wilhelm on
Hexagram 32 of the I Ching:
"Duration is a state whose movement is not worn down by hindrances. It is not a state of rest, for mere standstill is regression. Duration is rather the self-contained and therefore self-renewing movement of an organized, firmly integrated whole, taking place in accordance with immutable laws and beginning anew at every ending. The end is reached by an inward movement, by inhalation, systole, contraction, and this movement turns into a new beginning, in which the movement is directed outward, in exhalation, diastole, expansion."

— The Middle-English
Harrowing of Hell…
by Hulme, 1907, page 64
Comments Off on Wednesday July 9, 2008
Friday, July 4, 2008
Comments Off on Friday July 4, 2008
Friday, June 27, 2008
Comments Off on Friday June 27, 2008
Wednesday, June 25, 2008
"The Renaissance thinkers liked to
organize the four elements using
a chain of analogies running
from light to heavy:
fire : air :: air : water :: water : earth
They also organized them
in a diamond, like this:"

This figure of Baez
is related to a saying
attributed to Heraclitus:

For related thoughts by Jung,
see Aion, which contains the
following diagram:

"The formula reproduces exactly the essential features of the symbolic process of transformation. It shows the rotation of the mandala, the antithetical play of complementary (or compensatory) processes, then the apocatastasis, i.e., the restoration of an original state of wholeness, which the alchemists expressed through the symbol of the uroboros, and finally the formula repeats the ancient alchemical tetrameria, which is implicit in the fourfold structure of unity."
— Carl Gustav Jung
That the words Maximus of Tyre (second century A.D.) attributed to Heraclitus imply a cycle of the elements (analogous to the rotation in Jung's diagram) is not a new concept. For further details, see "The Rotation of the Elements," a 1995 webpage by one "John Opsopaus."
Related material:
Log24 entries of June 9, 2008, and
"Quintessence: A Glass Bead Game,"
by Charles Cameron.
Comments Off on Wednesday June 25, 2008
Tuesday, June 24, 2008
Plato’s Cave, continued:
… we know that we use
Only the eye as faculty, that the mind
Is the eye, and that this landscape of the mind
Is a landscape only of the eye; and that
We are ignorant men incapable
Of the least, minor, vital metaphor….
— Wallace Stevens, “Crude Foyer”
… So, so,
O son of man, the ignorant night, the travail
Of early morning, the mystery of the beginning
Again and again,
while history is unforgiven.
— Delmore Schwartz,
“In the Naked Bed, in Plato’s Cave“
Comments Off on Tuesday June 24, 2008
Saturday, June 21, 2008
The Kyoto Prize
for lifetime achievement
in arts and philosophy
this year goes to
Charles Taylor,

Montreal philosophy professor.
“The Kyoto Prize has been given in three domains since 1984:
advanced technology, basic sciences, and the arts and philosophy.
It is administered by the Inamori Foundation, whose president,
Kazuo Inamori, is founder and chairman emeritus of Kyocera and
KDDI Corporation, two Japanese telecommunications giants.”
|
|
|
“The Kyocera brand symbol is composed of a corporate mark
and our corporate logotype. The mark represents the initial
‘K’ (for Kyocera) encircling a ‘C’ (for ceramics). It was
introduced in October 1982 when the company name was
changed from ‘Kyoto Ceramic’ to ‘Kyocera.'”
— global.kyocera.com
|
Comments Off on Saturday June 21, 2008
Thursday, June 19, 2008
Soul Theorem
“The soul of the commonest object,
the structure of which is so adjusted,
seems to us radiant. The object
achieves its epiphany.”
— James Joyce, Stephen Hero

Above: Screenshot of today’s
New York Times obituary for
mathematician Detlef Gromoll,
known for the “soul theorem.”
Gromoll died on May 31
according to his son
Hans Christian.
From his obituary:
“Detlef Gromoll was born in Berlin
in 1938, and his childhood
was disrupted by the falling
bombs of World War II.”
Related material:
The discussion here
on June 1 of a lottery number
from the date of Gromoll’s death,
childhood, mathematics,
and prewar Berlin.
Comments Off on Thursday June 19, 2008
Wednesday, June 18, 2008
CHANGE
FEW CAN BELIEVE IN |
What I Loved, a novel by Siri Hustvedt (New York, Macmillan, 2003), contains a paragraph on the marriage of a fictional artist named Wechsler–
Page 67 —
“… Bill and Violet were married. The wedding was held in the Bowery loft on June 16th, the same day Joyce’s Jewish Ulysses had wandered around Dublin. A few minutes before the exchange of vows, I noted that Violet’s last name, Blom, was only an o away from Bloom, and that meaningless link led me to reflect on Bill’s name, Wechsler, which carries the German root for change, changing, and making change. Blooming and changing, I thought.”
For Hustvedt’s discussion of Wechsler’s art– sculptured cubes, which she calls “tightly orchestrated semantic bombs” (p. 169)– see Log24, May 25, 2008.
Related material:
Wechsler cubes
(after David Wechsler,
1896-1981, chief
psychologist at Bellevue)
These cubes are used to
make 3×3 patterns for
psychological testing.
Related 3×3 patterns appear
in “nine-patch” quilt blocks
and in the following–
Don Park at docuverse.com, Jan. 19, 2007:
“How to draw an Identicon

A 9-block is a small quilt using only 3 types of patches, out of 16 available, in 9 positions. Using the identicon code, 3 patches are selected: one for center position, one for 4 sides, and one for 4 corners.
Positions and Rotations
For center position, only a symmetric patch is selected (patch 1, 5, 9, and 16). For corner and side positions, patch is rotated by 90 degree moving clock-wise starting from top-left position and top position respectively.” |
| Jared Tarbell at levitated.net, May 15, 2002:
“The nine block is a common design pattern among quilters. Its construction methods and primitive building shapes are simple, yet produce millions of interesting variations.
Figure A. Four 9 block patterns, arbitrarily assembled, show the
grid composition of the block.
Each block is composed of 9 squares, arranged in a 3 x 3 grid. Each square is composed of one of 16 primitive shapes. Shapes are arranged such that the block is radially symmetric. Color is modified and assigned arbitrarily to each new block.
The basic building blocks of the nine block are limited to 16 unique geometric shapes. Each shape is allowed to rotate in 90 degree increments. Only 4 shapes are allowed in the center position to maintain radial symmetry.
Figure B. The 16 possible shapes allowed
for each grid space. The 4 shapes allowed
in the center have bold numbers.”
|
Such designs become of mathematical interest when their size is increased slightly, from square arrays of nine blocks to square arrays of sixteen. See Block Designs in Art and Mathematics.
(This entry was suggested by examples of 4×4 Identicons in use at Secret Blogging Seminar.)
Comments Off on Wednesday June 18, 2008
Monday, June 16, 2008
Bloomsday for Nash:
The Revelation Game
(American Mathematical Society Feb. 2008
review of Steven Brams’s Superior Beings:
If They Exist, How Would We Know?)
(pdf, 15 megabytes)
"Brams does not attempt to prove or disprove God. He uses elementary ideas from game theory to create situations between a Person (P) and God (Supreme Being, SB) and discusses how each reacts to the other in these model scenarios….
In the 'Revelation Game,' for example, the Person (P) has two options:
1) P can believe in SB's existence
2) P can not believe in SB's existence
The Supreme Being also has two options:
1) SB can reveal Himself
2) SB can not reveal Himself
Each player also has a primary and secondary goal. For the Person, the primary goal is to have his belief (or non-belief) confirmed by evidence (or lack thereof). The secondary goal is to 'prefer to believe in SB’s existence.' For the Supreme Being, the primary goal is to have P believe in His existence, while the secondary goal is to not reveal Himself. These goals allow us to rank all the outcomes for each player from best (4) to worst (1). We end up with a matrix as follows (the first number in the parentheses represents the SB's ranking for that box; the second number represents P's ranking):

The question we must answer is: what is the Nash equilibrium in this case?"
Analogously:
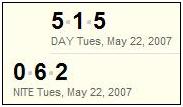
Lotteries on
Bloomsday,
June 16,
2008
|
Pennsylvania
(No revelation)
|
New York
(Revelation)
|
Mid-day
(No belief)
|
418

No belief,
no revelation
|
064

Revelation
without belief
|
Evening
(Belief)
|
709

Belief without
revelation
|
198
(A Cheap
Epiphany)

Belief and
revelation
|
The holy image

denoting belief and revelation
may be interpreted as
a black hole or as a
symbol by James Joyce:
|
When?
Going to dark bed there was a square round Sinbad the Sailor roc's auk's egg in the night of the bed of all the auks of the rocs of Darkinbad the Brightdayler.
Where?

— Ulysses, conclusion of Chapter 17
|
Comments Off on Monday June 16, 2008
Saturday, June 14, 2008
Cross and Wheel
An online tribute to Tim Russert
this morning had a song by a
Russert favorite, Bruce Springsteen:
"Wearin' the cross
of my calling,
on wheels of fire
I come rollin' down here."
— "The Rising"
Related material:
Hard Lessons

and the
five Log24 entries
ending on July 20, 2006,
which contain the following
example of what might be
caled "sacred order"
(see yesterday's entries)–

See also "Grave Matters" here
on November 8, 2006, and
the same date four years earlier,
as well as
"O Grave, Where Is Thy Victory?"
(pdf), a lecture by Jack Miles
at Clark Art Institute
(see Oct. 7-9, 2002)
on November 9, 2002.
The Miles lecture may be of
more comfort to Russert's
mourners than the
cross/wheel symbolism,
which has its dark side.
The cross, the wheel,
the Catholic faith, and
Russert's field of expertise,
politics, are of course
notably combined in the
crux gammata, discussed
here in a 2002 entry on
the Triumph of the Cross
and the Death of Grace
(Princess of Monaco).
Comments Off on Saturday June 14, 2008
Tuesday, June 10, 2008
Return to Paradise
Edward Rothstein's review in yesterday's New York Times–
Museum’s Vision:
West Coast Paradise —
seems to me more a description of Hell.
My own concept of paradise is closer to the Gary Cooper film "Return to Paradise," which impressed me greatly when I saw it on TV when I was in 10th grade.
A related vision: two frames from the Jodie Foster film "Contact"–
Comments Off on Tuesday June 10, 2008
Monday, June 9, 2008
Lying Rhymes
Readers of the previous entry
who wish to practice their pardes
may contemplate the following:

The evening 563 may, as in other recent entries, be interpreted as a page number in
Gravity’s Rainbow (Penguin Classics, 1995). From
that page:
“He brings out the mandala he found.
‘What’s it mean?’
[….]
Slothrop gives him the mandala. He hopes it will work like the mantra that Enzian told him once, mba-kayere (I am passed over), mba-kayere… a spell […]. A mezuzah. Safe passage through a bad night….”
Christ and the Four Elements
This 1495 image is found in
The Janus Faces of Genius:
The Role of Alchemy
in Newton’s Thought,
by B. J. T. Dobbs,
Cambridge University Press,
2002, p. 85
Related mandalas:
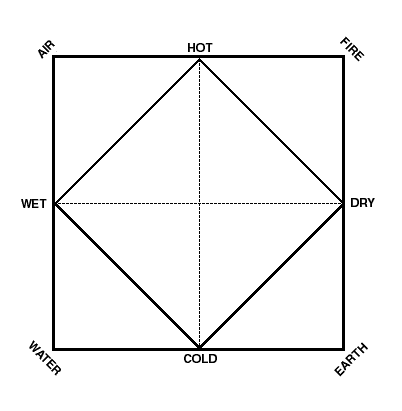
and

For further details,
click on any of the
three mandalas above.
“For every kind of vampire,
there is a kind of cross.”
— Thomas Pynchon, quoted
here on 9/13, 2007
(As for today’s New York Lottery midday number 007, see (for instance) Edward Rothstein in today’s New York Times on paradise, and also Tom Stoppard on heaven as “just a lying rhyme” for seven.)
Time of entry: 10:20:55 PM
Comments Off on Monday June 9, 2008
Interpret This
"With respect, you only interpret."
"Countries have gone to war
after misinterpreting one another."
— The Interpreter
"Once upon a time (
say, for Dante),
it must have been a revolutionary
and creative move to design works
of art so that they might be
experienced on several levels."
— Susan Sontag,
"Against Interpretation"
Edward Rothstein in today's
New York Times review of San Francisco's new Contemporary Jewish Museum:
"An introductory wall panel tells us that in the Jewish mystical tradition the four letters [in Hebrew] of pardes each stand for a level of biblical interpretation: very roughly, the literal, the allusive, the allegorical and the hidden. Pardes, we are told, became the museum’s symbol because it reflected the museum’s intention to cultivate different levels of interpretation: 'to create an environment for exploring multiple perspectives, encouraging open-mindedness' and 'acknowledging diverse backgrounds.' Pardes is treated as a form of mystical multiculturalism.
But even the most elaborate interpretations of a text or tradition require more rigor and must begin with the literal. What is being said? What does it mean? Where does it come from and where else is it used? Yet those are the types of questions– fundamental ones– that are not being asked or examined […].
How can multiple perspectives and open-mindedness and diverse backgrounds be celebrated without a grounding in knowledge, without history, detail, object and belief?"
"It's the system that matters.
How the data arrange
themselves inside it."
— Gravity's Rainbow
"Examples are the stained-
glass windows of knowledge."
— Vladimir Nabokov

Click on image to enlarge.
Comments Off on Monday June 9, 2008
Friday, June 6, 2008
The Dance of Chance
"Harvard seniors have
every right to demand a
Harvard-calibre speaker."
— Adam Goldenberg in
The Harvard Crimson
"Look down now, Cotton Mather"
— Wallace Stevens,
Harvard College
Class of 1901
For Thursday, June 5, 2008,
commencement day for Harvard's
Class of 2008, here are the
Pennsylvania Lottery numbers:
Mid-day 025
Evening 761
Thanks to the late
Harvard professor
Willard Van Orman Quine,
the mid-day number 025
suggests the name
"Isaac Newton."
(For the logic of this suggestion,
see On Linguistic Creation
and Raiders of the Lost Matrix.)
Thanks to Google search, the
name of Newton, combined with
Thursday's evening number 761,
suggests the following essay:
|
Daniel E. Koshland Jr.*
* D. E. Koshland Jr. passed away on 23 July 2007. He was a professor of biochemistry and molecular and cell biology at the University of California, Berkeley, since 1965. He served as Science's editor-in-chief from 1985 to 1995.
|
Comments Off on Friday June 6, 2008
Sunday, June 1, 2008
The conclusion of yesterday’s commentary on the May 30-31 Pennsylvania Lottery numbers:
Thomas Pynchon, Gravity’s Rainbow:
“The fear balloons again inside his brain. It will not be kept down with a simple Fuck You…. A smell, a forbidden room, at the bottom edge of his memory. He can’t see it, can’t make it out. Doesn’t want to. It is allied with the Worst Thing.
He knows what the smell has to be: though according to these papers it would have been too early for it, though he has never come across any of the stuff among the daytime coordinates of his life, still, down here, back here in the warm dark, among early shapes where the clocks and calendars don’t mean too much, he knows that’s what haunting him now will prove to be the smell of Imipolex G.
Then there’s this recent dream he is afraid of having again. He was in his old room, back home. A summer afternoon of lilacs and bees and
286”
What are we to make of this enigmatic 286? (No fair peeking at page 287.)
One possible meaning, given The Archivist‘s claim that “existence is infinitely cross-referenced”–
Page 286 of Ernest G. Schachtel, Metamorphosis: On the Conflict of Human Development and the Psychology of Creativity (first published in 1959), Hillsdale NJ and London, The Analytic Press, 2001 (chapter– “On Memory and Childhood Amnesia”):
“Both Freud and Proust speak of the autobiographical [my italics] memory, and it is only with regard to this memory that the striking phenomenon of childhood amnesia and the less obvious difficulty of recovering any past experience may be observed.”
The concluding “summer afternoon of lilacs and bees” suggests that 286 may also be a chance allusion to the golden afternoon of Disney’s Alice in Wonderland. (Cf. St. Sarah’s Day, 2008)
Some may find the Disney afternoon charming; others may see it as yet another of Paul Simon’s dreaded cartoon graveyards.
More tastefully, there is poem 286 in the 1919 Oxford Book of English Verse– “Love.”
For a midrash on this poem, see Simone Weil, who became acquainted with the poem by chance:
“I always prefer saying chance rather than Providence.”
— Simone Weil, letter of about May 15, 1942
Weil’s brother André might prefer Providence (source of the Bulletin of the American Mathematical Society.)
Related material:
Log24, December 20, 2003–
White, Geometric, and Eternal—
A description in
Gravity’s Rainbow of prewar Berlin as “white and geometric” suggested, in combination with a reference elsewhere to “the eternal,” a citation of the following
illustration of the concept “white, geometric, and eternal”–
For more on the mathematical significance of this figure, see (for instance) Happy Birthday, Hassler Whitney, and Combinatorics of Coxeter Groups, by Anders Björner and Francesco Brenti, Graduate Texts in Mathematics, vol. 231, Springer, New York, 2005.
This book is reviewed in the current issue (July 2008) of the above-mentioned Providence Bulletin.
The review in the Bulletin discusses reflection groups in continuous spaces.
Comments Off on Sunday June 1, 2008
Monday, May 26, 2008
Comments Off on Monday May 26, 2008
Sunday, May 25, 2008
Hall of Mirrors
Epigraph to
“Deploying the Glass Bead Game, Part II,”
by Robert de Marrais:
“For a complete logical argument,”
Arthur began
with admirable solemnity,
“we need two prim Misses –”
“Of course!” she interrupted.
“I remember that word now.
And they produce — ?”
“A Delusion,” said Arthur.
— Lewis Carroll,
Sylvie and Bruno
Prim Miss 1:
Erin O’Connor’s weblog “Critical Mass” on May 24:
Roger Rosenblatt’s Beet [Ecco hardcover, Jan. 29, 2008] is the latest addition to the noble sub-genre of campus fiction….
Curricular questions and the behavior of committees are at once dry as dust subjects and areas ripe for sarcastic send-up– not least because, as dull as they are, they are really both quite vital to the credibility and viability of higher education.
Here’s an excerpt from the first meeting, in which committee members propose their personal plans for a new, improved curriculum:
“… Once the students really got into playing with toy soldiers, they would understand history with hands-on excitement.”
To demonstrate his idea, he’d brought along a shoe box full of toy doughboys and grenadiers, and was about to reenact the Battle of Verdun on the committee table when Heilbrun stayed his hand. “We get it,” he said.
“That’s quite interesting, Molton,” said Booth [a chemist]. “But is it rigorous enough?”
At the mention of the word, everyone, save Peace, sat up straight.
“Rigor is so important,” said Kettlegorf.
“We must have rigor,” said Booth.
“You may be sure,” said the offended Kramer. “I never would propose anything lacking rigor.”
Smythe inhaled and looked at the ceiling. “I think I may have something of interest,” he said, as if he were at a poker game and was about to disclose a royal flush. “My proposal is called ‘Icons of Taste.’ It would consist of a galaxy of courses affixed to several departments consisting of lectures on examples of music, art, architecture, literature, and other cultural areas a student needed to indicate that he or she was sophisticated.”
“Why would a student want to do that?” asked Booth.
“Perhaps sophistication is not a problem for chemists,” said Smythe. Lipman tittered.
“What’s the subject matter?” asked Heilbrun. “Would it have rigor?”
“Of course it would have rigor. Yet it would also attract those additional students Bollovate is talking about.” Smythe inhaled again. “The material would be carefully selected,” he said. “One would need to pick out cultural icons the students were likely to bring up in conversation for the rest of their lives, so that when they spoke, others would recognize their taste as being exquisite yet eclectic and unpredictable.”
“You mean Rembrandt?” said Kramer.
Smythe smiled with weary contempt. “No, I do not mean Rembrandt. I don’t mean Beethoven or Shakespeare, either, unless something iconic has emerged about them to justify their more general appeal.”
“You mean, if they appeared on posters,” said Lipman.
“That’s it, precisely.”
Lipman blushed with pride.
“The subject matter would be fairly easy to amass,” Smythe said. “We could all make up a list off the top of our heads. Einstein–who does have a poster.” He nodded to the ecstatic Lipman. “Auden, for the same reason. Students would need to be able to quote ‘September 1939[ or at least the last lines. And it would be good to teach ‘Musee des Beaux Arts’ as well, which is off the beaten path, but not garishly. Mahler certainly. But Cole Porter too. And Sondheim, I think. Goya. Warhol, it goes without saying, Stephen Hawking, Kurosawa, Bergman, Bette Davis. They’d have to come up with some lines from Dark Victory, or better still, Jezebel. La Dolce Vita. Casablanca. King of Hearts. And Orson, naturally. Citizen Kane, I suppose, though personally I prefer F for Fake.”
“Judy!” cried Heilbrun.
“Yes, Judy too. But not ‘Over the Rainbow.’ It would be more impressive for them to do ‘The Trolley Song,’ don’t you think?” Kettlegorf hummed the intro.
“Guernica,” said Kramer. “Robert Capa.” 
“Edward R. Murrow,” said Lipman.
“No! Don’t be ridiculous!” said Smythe, ending Lipman’s brief foray into the world of respectable thought.
“Marilyn Monroe!” said Kettlegorf.
“Absolutely!” said Smythe, clapping to indicate his approval.
“And the Brooklyn Bridge,” said Booth, catching on. “And the Chrysler Building.”
“Maybe,” said Smythe. “But I wonder if the Chrysler Building isn’t becoming something of a cliche.”
Peace had had enough. “And you want students to nail this stuff so they’ll do well at cocktail parties?”
Smythe sniffed criticism, always a tetchy moment for him. “You make it sound so superficial,” he said.
Prim Miss 2:
Siri Hustvedt speaks at Adelaide Writers’ Week– a story dated March 24, 2008 †—
“I have come to think of my books as echo chambers or halls of mirrors in which themes, ideas, associations continually reflect and reverberate inside a text. There is always point and counterpoint, to use a musical illustration. There is always repetition with difference.”
A Delusion:
Exercise — Identify in the following article the sentence that one might (by unfairly taking it out of context) argue is a delusion.
(Hint: See Reflection Groups in Finite Geometry.)

Why Borovik’s Figure 4
is included above:
Comments Off on Sunday May 25, 2008
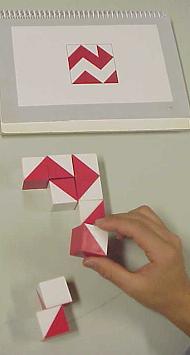
Wechsler Cubes
"Confusion is nothing new."
— Song lyric, Cyndi Lauper
Part I:
Magister Ludi
Hermann Hesse's 1943 The Glass Bead Game (Picador paperback, Dec. 6, 2002, pp. 139-140)–
"For the present, the Master showed him a bulky memorandum, a proposal he had received from an organist– one of the innumerable proposals which the directorate of the Game regularly had to examine. Usually these were suggestions for the admission of new material to the Archives. One man, for example, had made a meticulous study of the history of the madrigal and discovered in the development of the style a curved that he had expressed both musically and mathematically, so that it could be included in the vocabulary of the Game. Another had examined the rhythmic structure of Julius Caesar's Latin and discovered the most striking congruences with the results of well-known studies of the intervals in Byzantine hymns. Or again some fanatic had once more unearthed some new cabala hidden in the musical notation of the fifteenth century. Then there were the tempestuous letters from abstruse experimenters who could arrive at the most astounding conclusions from, say, a comparison of the horoscopes of Goethe and Spinoza; such letters often included pretty and seemingly enlightening geometric drawings in several colors."
Part II:
A Bulky Memorandum
From Siri Hustvedt, author of Mysteries of the Rectangle: Essays on Painting (Princeton Architectural Press, 2005)– What I Loved: A Novel (Picador paperback, March 1, 2004, page 168)–
A description of the work of Bill Wechsler, a fictional artist:
"Bill worked long hours on a series of autonomous pieces about numbers. Like O's Journey, the works took place inside glass cubes, but these were twice as large– about two feet square. He drew his inspiration from sources as varied as the Cabbala, physics, baseball box scores, and stock market reports. He painted, cut, sculpted, distorted, and broke the numerical signs in each work until they became unrecognizable. He included figures, objects, books, windows, and always the written word for the number. It was rambunctious art, thick with allusion– to voids, blanks, holes, to monotheism and the individual, the the dialectic and yin-yang, to the Trinity, the three fates, and three wishes, to the golden rectangle, to seven heavens, the seven lower orders of the sephiroth, the nine Muses, the nine circles of Hell, the nine worlds of Norse mythology, but also to popular references like A Better Marriage in Five Easy Lessons and Thinner Thighs in Seven Days. Twelve-step programs were referred to in both cube one and cube two. A miniature copy of a book called The Six Mistakes Parents Make Most Often lay at the bottom of cube six. Puns appeared, usually well disguised– one, won; two, too, and Tuesday; four, for, forth; ate, eight. Bill was partial to rhymes as well, both in images and words. In cube nine, the geometric figure for a line had been painted on one glass wall. In cube three, a tiny man wearing the black-and-white prison garb of cartoons and dragging a leg iron has
— End of page 168 —
opened the door to his cell. The hidden rhyme is "free." Looking closely through the walls of the cube, one can see the parallel rhyme in another language: the German word drei is scratched into one glass wall. Lying at the bottom of the same box is a tiny black-and-white photograph cut from a book that shows the entrance to Auschwitz: ARBEIT MACHT FREI. With every number, the arbitrary dance of associations worked togethere to create a tiny mental landscape that ranged in tone from wish-fulfillment dream to nightmare. Although dense, the effect of the cubes wasn't visually disorienting. Each object, painting, drawing, bit of text, or sculpted figure found its rightful place under the glass according to the necessary, if mad, logic of numerical, pictorial, and verbal connection– and the colors of each were startling. Every number had been given a thematic hue. Bill had been interested in Goethe's color wheel and in Alfred Jensen's use of it in his thick, hallucinatory paintings of numbers. He had assigned each number a color. Like Goethe, he included black and white, although he didn't bother with the poet's meanings. Zero and one were white. Two was blue. Three was red, four was yellow, and he mixed colors: pale blue for five, purples in six, oranges in seven, greens in eight, and blacks and grays in nine. Although other colors and omnipresent newsprint always intruded on the basic scheme, the myriad shades of a single color dominated each cube.
The number pieces were the work of a man at the top of his form. An organic extension of everything Bill had done before, these knots of symbols had an explosive effect. The longer I looked at them, the more the miniature constructions seemed on the brink of bursting from internal pressure. They were tightly orchestrated semantic bombs through which Bill laid bare the arbitrary roots of meaning itself– that peculiar social contract generated by little squiggles, dashes, lines, and loops on a page."
Part III:
Wechsler Cubes
(named not for
Bill Wechsler, the
fictional artist above,
but for the non-fictional
David Wechsler) –
Part IV:
A Magic Gallery
|
ZZ
WW
Figures from the
Kaleidoscope Puzzle
of Steven H. Cullinane:


Poem by Eugen Jost:
Zahlen und Zeichen,
Wörter und Worte
Mit Zeichen und Zahlen
vermessen wir Himmel und Erde
schwarz
auf weiss
schaffen wir neue Welten
oder gar Universen
Numbers and Names,
Wording and Words
With numbers and names
we measure heaven and earth
black
on white
we create new worlds
and universes
English translation
by Catherine Schelbert
A related poem:
Alphabets
by Hermann Hesse
From time to time
we take our pen in hand
and scribble symbols
on a blank white sheet
Their meaning is
at everyone's command;
it is a game whose rules
are nice and neat.
But if a savage
or a moon-man came
and found a page,
a furrowed runic field,
and curiously studied
lines and frame:
How strange would be
the world that they revealed.
a magic gallery of oddities.
He would see A and B
as man and beast,
as moving tongues or
arms or legs or eyes,
now slow, now rushing,
all constraint released,
like prints of ravens'
feet upon the snow.
He'd hop about with them,
fly to and fro,
and see a thousand worlds
of might-have-been
hidden within the black
and frozen symbols,
beneath the ornate strokes,
the thick and thin.
He'd see the way love burns
and anguish trembles,
He'd wonder, laugh,
shake with fear and weep
because beyond this cipher's
cross-barred keep
he'd see the world
in all its aimless passion,
diminished, dwarfed, and
spellbound in the symbols,
and rigorously marching
prisoner-fashion.
He'd think: each sign
all others so resembles
that love of life and death,
or lust and anguish,
are simply twins whom
no one can distinguish…
until at last the savage
with a sound
of mortal terror
lights and stirs a fire,
chants and beats his brow
against the ground
and consecrates the writing
to his pyre.
Perhaps before his
consciousness is drowned
in slumber there will come
to him some sense
of how this world
of magic fraudulence,
this horror utterly
behind endurance,
has vanished as if
it had never been.
He'll sigh, and smile,
and feel all right again.
— Hermann Hesse (1943),
"Buchstaben," from
Das Glasperlenspiel,
translated by
Richard and Clara Winston
|
Comments Off on Sunday May 25, 2008
Thursday, May 22, 2008
The Undertaking:
An Exercise in
Conceptual Art

Hexagram 54:
THE JUDGMENT
Undertakings bring misfortune.
Nothing that would further.

“Brian O’Doherty, an Irish-born artist,
before the [Tuesday, May 20] wake
of his alter ego* ‘Patrick Ireland’
on the grounds of the
Irish Museum of Modern Art.”
— New York Times, May 22, 2008
THE IMAGE
Thus the superior man
understands the transitory
in the light of
the eternity of the end.
Another version of
the image:
See 2/22/08
and 4/19/08.
Related material:
Michael Kimmelman in today’s New York Times—
“An essay from the ’70s by Mr. O’Doherty, ‘Inside the White Cube,’ became famous in art circles for describing how modern art interacted with the gallery spaces in which it was shown.”
Brian O’Doherty, “Inside the White Cube,” 1976 Artforum essays on the gallery space and 20th-century art:
“The history of modernism is intimately framed by that space. Or rather the history of modern art can be correlated with changes in that space and in the way we see it. We have now reached a point where we see not the art but the space first…. An image comes to mind of a white, ideal space that, more than any single picture, may be the archetypal image of 20th-century art.”
An archetypal image
THE SPACE:

A non-archetypal image
THE ART:

Natasha Wescoat, 2004
See also
Epiphany 2008:

“Nothing that would further.”
— Hexagram 54
|
Lear’s fool:
…. Now thou art an 0
without a figure. I am better
than thou art, now. I am a fool;
thou art nothing….
|
“…. in the last mystery of all the single figure of what is called the World goes joyously dancing in a state beyond moon and sun, and the number of the Trumps is done. Save only for that which has no number and is called the Fool, because mankind finds it folly till it is known. It is sovereign or it is nothing, and if it is nothing then man was born dead.”
— The Greater Trumps,
by Charles Williams, Ch. 14
Comments Off on Thursday May 22, 2008
Sunday, May 18, 2008
From the Grave
DENNIS OVERBYE
in yesterday's New York Times:
"From the grave, Albert Einstein
poured gasoline on the culture wars
between science and religion this week…."
An announcement of a
colloquium at Princeton:

Above: a cartoon,
"Coxeter exhuming Geometry,"
with the latter's tombstone inscribed
"GEOMETRY
600 B.C. —
1900 A.D.
R.I.P."

The above is from
The Paradise of Childhood,
a work first published in 1869.
"I need a photo-opportunity,
I want a shot at redemption.
Don't want to end up a cartoon
In a cartoon graveyard."
— Paul Simon

Albert Einstein,
1879-1955:
"It is quite clear to me that the religious paradise of youth, which was thus lost, was a first attempt to free myself from the chains of the 'merely-personal,' from an existence which is dominated by wishes, hopes and primitive feelings. Out yonder there was this huge world, which exists independently of us human beings and which stands before us like a great, eternal riddle, at least partially accessible to our inspection and thinking. The contemplation of this world beckoned like a liberation…."
— Autobiographical Notes, 1949
Related material:
A commentary on Tom Wolfe's
"Sorry, but Your Soul Just Died"–
"The Neural Buddhists," by David Brooks,
in the May 13 New York Times:
"The mind seems to have
the ability to transcend itself
and merge with a larger
presence that feels more real."
A New Yorker commentary on
a new translation of the Psalms:
"Suddenly, in a world without
Heaven, Hell, the soul, and
eternal salvation or redemption,
the theological stakes seem
more local and temporal:
'So teach us to number our days.'"
and a May 13 Log24 commentary
on Thomas Wolfe's
"Only the Dead Know Brooklyn"–
"… all good things — trout as well as
eternal salvation — come by grace
and grace comes by art
and art does not come easy."
— A River Runs Through It
"Art isn't easy."
— Stephen Sondheim,
quoted in
Solomon's Cube.
For further religious remarks,
consult Indiana Jones and the
Kingdom of the Crystal Skull
and The Librarian:
Return to King Solomon's Mines.
Comments Off on Sunday May 18, 2008
Friday, May 16, 2008
“From the grave, Albert Einstein poured gasoline on the culture wars between science and religion this week.
A letter the physicist wrote in 1954 to the philosopher Eric Gutkind, in which he described the Bible as ‘pretty childish’ and scoffed at the notion that the Jews could be a ‘chosen people,’ sold for $404,000 at an auction in London. That was 25 times the presale estimate.”
A less controversial Einstein-related remark:
“The relativity problem is one of central significance throughout geometry and algebra and has been recognized as such by the mathematicians at an early time.”
— Hermann Weyl, “Relativity Theory as a Stimulus in Mathematical Research,” Proceedings of the American Philosophical Society, Vol. 93, No. 7, Theory of Relativity in Contemporary Science: Papers Read at the Celebration of the Seventieth Birthday of Professor Albert Einstein in Princeton, March 19, 1949 (Dec. 30, 1949), pp. 535-541
Comments Off on Friday May 16, 2008
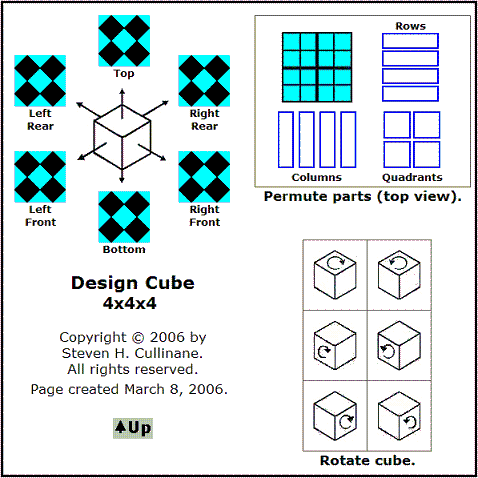
Saturday, May 10, 2008
MoMA Goes to
Kindergarten
"… the startling thesis of Mr. Brosterman's new book, 'Inventing Kindergarten' (Harry N. Abrams, $39.95): that everything the giants of modern art and architecture knew about abstraction they learned in kindergarten, thanks to building blocks and other educational toys designed by Friedrich Froebel, a German educator, who coined the term 'kindergarten' in the 1830's."
— "Was Modernism Born
in Toddler Toolboxes?"
by Trip Gabriel, New York Times,
April 10, 1997
RELATED MATERIAL
Figure 1 —
Concept from 1819:

(Footnotes 1 and 2)
Figure 2 —
The Third Gift, 1837:

Froebel's Third Gift
Froebel, the inventor of
kindergarten, worked as
an assistant to the
crystallographer Weiss
mentioned in Fig. 1.
(Footnote 3)
Figure 3 —
The Third Gift, 1906:

Figure 4 —
Solomon's Cube,
1981 and 1983:

Figure 5 —
Design Cube, 2006:
For some mathematical background, see
Footnotes:
Comments Off on Saturday May 10, 2008
Friday, May 9, 2008
Comments Off on Friday May 9, 2008
Wednesday, May 7, 2008
Forms of the Rock
“point A / In a perspective
that begins again / At B”
— Wallace Stevens,
The Rock
See also
August 2, 2002
January 20, 2003
April 8, 2003
December 5, 2004
December 10, 2004
January 11, 2006
April 30, 2006
August 25, 2006
August 26, 2006
February 6, 2007
July 23, 2007
July 24, 2007
September 30, 2007
April 14, 2008
Christmas Eve, 1981
Comments Off on Wednesday May 7, 2008
Friday, May 2, 2008
A Balliol Star
In memory of
mathematician
Graham Higman of
Balliol College and
Magdalen College,
Oxford,
Jan. 19, 1917 –
April 8, 2008
From a biography of an earlier Balliol student,
Gerard Manley Hopkins (1844-1889):
"In 1867 he won First-Class degrees in Classics
and 'Greats' (a rare 'double-first') and was
considered by Jowett to be the star of Balliol."
Hopkins, a poet who coined the term "inscape," was a member of the Society of Jesus.
According to a biography, Higman was the founder of Oxford's Invariant Society.
From a publication of that society, The Invariant, Issue 15– undated but (according to Issue 16, of 2005) from 1996 (pdf):
Taking the square root
of a function
by Ian Collier
"David Singmaster once gave a talk at the Invariants and afterwards asked this question:
What is the square root of the exponential function? In other words, can you define a function f such that for all x, f 2(x) — that is, f (f (x)) — is equal to e x ? He did not give the answer straight away so I attempted it…." |
Another approach to the expression f(f(x)), by myself in 1982:

For further details,
see Inscapes.
For more about Higman, see an interview in the September 2001 newsletter of the European Mathematical Society (pdf).
"Philosophers ponder the idea
of identity: what it is to give
something a name
on Monday
and have it respond to
that name on Friday…."
— Bernard Holland
Comments Off on Friday May 2, 2008
Tuesday, April 29, 2008
Sacerdotal Jargon
at Harvard:

Thomas Wolfe
(Harvard M.A., 1922)
versus

Rosalind Krauss
(Harvard M.A., 1964,
Ph.D., 1969)
on
The Kernel of Eternity
"No culture has a pact with eternity."
— George Steiner, interview in
The Guardian of April 19
"At that instant he saw,
in one blaze of light, an image
of unutterable conviction….
the core of life, the essential
pattern whence all other things
proceed, the kernel of eternity."
— Thomas Wolfe, Of Time
and the River, quoted in
Log24 on June 9, 2005
From today's online Harvard Crimson:
"… under the leadership of Faust,
Harvard students should look forward
to an ever-growing opportunity for
international experience
and artistic endeavor."

Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen
From a recent book
on Wolfgang Pauli,
The Innermost Kernel:

A belated happy birthday
to the late
Felix Christian Klein
(born on April 25) —

Another Harvard figure quoted here on Dec. 5, 2002:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951)
From a review of Rosalind Krauss's The Optical Unconscious (MIT Press hardcover, 1993):
Krauss is concerned to present Modernism less in terms of its history than its structure, which she seeks to represent by means of a kind of diagram: "It is more interesting to think of modernism as a graph or table than a history." The "table" is a square with diagonally connected corners, of the kind most likely to be familiar to readers as the Square of Opposition, found in elementary logic texts since the mid-19th century. The square, as Krauss sees it, defines a kind of idealized space "within which to work out unbearable contradictions produced within the real field of history." This she calls, using the inevitable gallicism, "the site of Jameson's Political Unconscious" and then, in art, the optical unconscious, which consists of what Utopian Modernism had to kick downstairs, to repress, to "evacuate… from its field."
— Arthur C. Danto in ArtForum, Summer 1993
Rosalind Krauss in The Optical Unconscious (MIT Press paperback, 1994):
For a presentation of the Klein Group, see Marc Barbut, "On the Meaning of the Word 'Structure' in Mathematics," in Introduction to Structuralism, ed. Michael Lane (New York: Basic Books, 1970). Claude Lévi-Strauss uses the Klein group in his analysis of the relation between Kwakiutl and Salish masks in The Way of the Masks, trans. Sylvia Modelski (Seattle: University of Washington Press, 1982), p. 125; and in relation to the Oedipus myth in "The Structural Analysis of Myth," Structural Anthropology, trans. Claire Jackobson [sic] and Brooke Grundfest Schoepf (New York: Basic Books, 1963). In a transformation of the Klein Group, A. J. Greimas has developed the semiotic square, which he describes as giving "a slightly different formulation to the same structure," in "The Interaction of Semiotic Constraints," On Meaning (Minneapolis: University of Minnesota Press, 1987), p. 50. Jameson uses the semiotic square in The Political Unconscious (see pp. 167, 254, 256, 277) [Fredric Jameson, The Political Unconscious: Narrative as a Socially Symbolic Act (Ithaca: Cornell University Press, 1981)], as does Louis Marin in "Disneyland: A Degenerate Utopia," Glyph, no. 1 (1977), p. 64.
For related non-sacerdotal jargon, see…
Wikipedia on the Klein group (denoted V, for Vierergruppe):
In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
Comments Off on Tuesday April 29, 2008
Monday, April 28, 2008
Religious Art
The black monolith of
Kubrick's 2001 is, in
its way, an example
of religious art.

One artistic shortcoming
(or strength– it is, after
all, monolithic) of
that artifact is its
resistance to being
analyzed as a whole
consisting of parts, as
in a Joycean epiphany.
The following
figure does
allow such
an epiphany.

One approach to
the epiphany:
"Transformations play
a major role in
modern mathematics."
– A biography of
Felix Christian Klein
The above 2×4 array
(2 columns, 4 rows)
furnishes an example of
a transformation acting
on the parts of
an organized whole:

For other transformations
acting on the eight parts,
hence on the 35 partitions, see
"Geometry of the 4×4 Square,"
as well as Peter J. Cameron's
"The Klein Quadric
and Triality" (pdf),
and (for added context)
"The Klein Correspondence,
Penrose Space-Time, and
a Finite Model."
For a related structure–
not rectangle but cube–
see Epiphany 2008.
Comments Off on Monday April 28, 2008
Sunday, April 27, 2008
Happy Birthday
to the late
Gian-Carlo Rota,
mathematician and
scholar of philosophy
Rota* on his favorite philosopher:
“I believe Husserl to be the greatest philosopher of all times….
Intellectual honesty is the striking quality of Husserl’s writings. He wrote what he honestly believed to be true, neither more nor less. However, honesty is not clarity; as a matter of fact, honesty and clarity are at opposite ends. Husserl proudly refused to stoop to the demands of showmanship that are indispensable in effective communication.”

Related material:
The Diamond Theorem
* Gian-Carlo Rota, “Ten Remarks on Husserl and Phenomenology,” in O.K. Wiegand et al. (eds.), Phenomenology on Kant, German Idealism, Hermeneutics and Logic, pp. 89-97, Kluwer Academic Publishers, 2000
Comments Off on Sunday April 27, 2008
Saturday, April 26, 2008
Mere Philosophy
In Memory of
Edmund Husserl
“Mereology (from the Greek μερος, ‘part’)
is the theory of parthood relations:
of the relations of part to whole and the
relations of part to part within a whole.
Its roots can be traced back to
the early days of philosophy….”
— Stanford Encyclopedia of Philosophy
“Beauty is the proper conformity
of the parts to one another
and to the whole.”
— Classic definition quoted
by Werner Heisenberg
(Log24, May 18-20, 2005)
“It seems, as one becomes older,
That the past has another pattern,
and ceases to be a mere sequence….”
— T. S. Eliot, Four Quartets

See also Time Fold
and Theme and Variations.
Comments Off on Saturday April 26, 2008
Saturday, April 19, 2008
A Midrash for Benedict
On April 16, the Pope’s birthday, the evening lottery number in Pennsylvania was 441. The Log24 entries of April 17 and April 18 supplied commentaries based on 441’s incarnation as a page number in an edition of Heidegger’s writings. Here is a related commentary on a different incarnation of 441. (For a context that includes both today’s commentary and those of April 17 and 18, see Gian-Carlo Rota– a Heidegger scholar as well as a mathematician– on mathematical Lichtung.)
From R. D. Carmichael, Introduction to the Theory of Groups of Finite Order (Boston, Ginn and Co., 1937)– an exercise from the final page, 441, of the final chapter, “Tactical Configurations”–
“23. Let G be a multiply transitive group of degree n whose degree of transitivity is k; and let G have the property that a set S of m elements exists in G such that when k of the elements S are changed by a permutation of G into k of these elements, then all these m elements are permuted among themselves; moreover, let G have the property P, namely, that the identity is the only element in G which leaves fixed the n – m elements not in S. Then show that G permutes the m elements S into
n(
n -1) … (
n –
k + 1)
____________________
m(m – 1) … (m – k + 1)
sets of
m elements each, these sets forming a configuration having the property that any (whatever) set of
k elements appears in one and just one of these sets of
m elements each. Discuss necessary conditions on
m,
n,
k in order that the foregoing conditions may be realized. Exhibit groups illustrating the theorem.”
This exercise concerns an important mathematical structure said to have been discovered independently by the American Carmichael and by the German Ernst Witt.
For some perhaps more comprehensible material from the preceding page in Carmichael– 440– see Diamond Theory in 1937.
Comments Off on Saturday April 19, 2008
Monday, April 14, 2008
Classical Quantum
From this morning's
New York Times:

"John A. Wheeler, a visionary physicist… died Sunday morning [April 13, 2008]….
… Dr. Wheeler set the agenda for generations of theoretical physicists, using metaphor as effectively as calculus to capture the imaginations of his students and colleagues and to pose questions that would send them, minds blazing, to the barricades to confront nature….
'He rejuvenated general relativity; he made it an experimental subject and took it away from the mathematicians,' said Freeman Dyson, a theorist at the Institute for Advanced Study….
… he [Wheeler] sailed to Copenhagen to work with Bohr, the godfather of the quantum revolution, which had shaken modern science with paradoxical statements about the nature of reality.
'You can talk about people like Buddha, Jesus, Moses, Confucius, but the thing that convinced me that such people existed were the conversations with Bohr,' Dr. Wheeler said….
… Dr. Wheeler was swept up in the Manhattan Project to build an atomic bomb. To his lasting regret, the bomb was not ready in time to change the course of the war in Europe….
Dr. Wheeler continued to do government work after the war, interrupting his research to help develop the hydrogen bomb, promote the building of fallout shelters and support the Vietnam War….
… Dr. Wheeler wondered if this quantum uncertainty somehow applied to the universe and its whole history, whether it was the key to understanding why anything exists at all.
'We are no longer satisfied with insights only into particles, or fields of force, or geometry, or even space and time,' Dr. Wheeler wrote in 1981. 'Today we demand of physics some understanding of existence itself.'
At a 90th birthday celebration in 2003, Dr. Dyson said that Dr. Wheeler was part prosaic calculator, a 'master craftsman,' who decoded nuclear fission, and part poet. 'The poetic Wheeler is a prophet,' he said, 'standing like Moses on the top of Mount Pisgah, looking out over the promised land that his people will one day inherit.'"
— Dennis Overbye, The New York Times,
Monday, April 14, 2008
As prophets go, I prefer
the poet Wallace Stevens:
"point A / In a perspective
that begins again / At B"
— Wallace Stevens,
"The Rock"
Comments Off on Monday April 14, 2008
Sunday, April 13, 2008
The Echo
in Plato’s Cave
“It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy.”
— Simon Blackburn, Think (Oxford, 1999)
Michael Harris, mathematician at the University of Paris:
“… three ‘parts’ of tragedy identified by Aristotle that transpose to fiction of all types– plot (mythos), character (ethos), and ‘thought’ (dianoia)….”
— paper (pdf) to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.
Mythos —
A visitor from France this morning viewed the entry of Jan. 23, 2006: “In Defense of Hilbert (On His Birthday).” That entry concerns a remark of Michael Harris.
A check of Harris’s website reveals a new article:
“Do Androids Prove Theorems in Their Sleep?” (slighly longer version of article to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.) (pdf).
From that article:
“The word ‘key’ functions here to structure the reading of the article, to draw the reader’s attention initially to the element of the proof the author considers most important. Compare E.M. Forster in Aspects of the Novel:
[plot is] something which is measured not be minutes or hours, but by intensity, so that when we look at our past it does not stretch back evenly but piles up into a few notable pinnacles.”
Ethos —
“Forster took pains to widen and deepen the enigmatic character of his novel, to make it a puzzle insoluble within its own terms, or without. Early drafts of A Passage to India reveal a number of false starts. Forster repeatedly revised drafts of chapters thirteen through sixteen, which comprise the crux of the novel, the visit to the Marabar Caves. When he began writing the novel, his intention was to make the cave scene central and significant, but he did not yet know how:
When I began a A Passage to India, I knew something important happened in the Malabar (sic) Caves, and that it would have a central place in the novel– but I didn’t know what it would be… The Malabar Caves represented an area in which concentration can take place. They were to engender an event like an egg.”
— E. M. Forster: A Passage to India, by Betty Jay
Dianoia —
Flagrant Triviality
or Resplendent Trinity?
“Despite the flagrant triviality of the proof… this result is the key point in the paper.”
— Michael Harris, op. cit., quoting a mathematical paper
Online Etymology Dictionary:
flagrant c.1500, “resplendent,” from L. flagrantem (nom. flagrans) “burning,” prp. of flagrare “to burn,” from L. root *flag-, corresponding to PIE *bhleg– (cf. Gk. phlegein “to burn, scorch,” O.E. blæc “black”). Sense of “glaringly offensive” first recorded 1706, probably from common legalese phrase in flagrante delicto “red-handed,” lit. “with the crime still blazing.”
A related use of “resplendent”– applied to a Trinity, not a triviality– appears in the Liturgy of Malabar:
— The Liturgies of SS. Mark, James, Clement, Chrysostom, and Basil, and the Church of Malabar, by the Rev. J.M. Neale and the Rev. R.F. Littledale, reprinted by Gorgias Press, 2002
On Universals and
A Passage to India:
“”The universe, then, is less intimation than cipher: a mask rather than a revelation in the romantic sense. Does love meet with love? Do we receive but what we give? The answer is surely a paradox, the paradox that there are Platonic universals beyond, but that the glass is too dark to see them. Is there a light beyond the glass, or is it a mirror only to the self? The Platonic cave is even darker than Plato made it, for it introduces the echo, and so leaves us back in the world of men, which does not carry total meaning, is just a story of events.”
Comments Off on Sunday April 13, 2008
Tuesday, April 8, 2008
Comments Off on Tuesday April 8, 2008
Monday, April 7, 2008
A year ago…
(Holy Saturday, 2007) —
From Friedrich Froebel,
who invented kindergarten:

For further details, see
Gift of the Third Kind
and
Kindergarten Relativity.
Related material:
“… There was a problem laid out on the board, a six-mover. I couldn’t solve it, like a lot of my problems. I reached down and moved a knight…. I looked down at the chessboard. The move with the knight was wrong. I put it back where I had moved it from. Knights had no meaning in this game. It wasn’t a game for knights.”
— Raymond Chandler, The Big Sleep
Comments Off on Monday April 7, 2008
“Lord Arglay had a suspicion that the Stone would be purely logical. Yes, he thought, but what, in that sense, were the rules of its pure logic?”
— Charles Williams, Many Dimensions
Comments Off on Monday April 7, 2008
Saturday, April 5, 2008
Comments Off on Saturday April 5, 2008
Monday, March 31, 2008
Comments Off on Monday March 31, 2008
Thursday, March 27, 2008
Back to the Garden
Film star Richard Widmark
died on Monday, March 24.
From Log24 on that date:
"Hanging from the highest limb
of the apple tree are
the three God's Eyes…"
— Ken Kesey
Related material:
The Beauty Test, 5/23/07–
H.S.M. Coxeter's classic
Introduction to Geometry (2nd ed.):

Note the resemblance of
the central part to
a magical counterpart–
the Ojo de Dios
of Mexico's Sierra Madre.
From a Richard Widmark film festival:
GARDEN OF EVIL
Henry Hathaway, 1954
"A severely underrated Scope western, shot in breathtaking mountain locations near Cuernavaca. Widmark, Gary Cooper and Cameron Mitchell are a trio of fortune hunters stranded in Mexico, when they are approached by Susan Hayward to rescue her husband (Hugh Marlowe) from a caved-in gold mine in Indian country. When they arrive at the 'Garden of Evil,' they must first battle with one another before they have to stave off their bloodthirsty Indian attackers. Widmark gives a tough, moving performance as Fiske, the one who sacrifices himself to save his friends. 'Every day it goes, and somebody goes with it,' he says as he watches the setting sun. 'Today it's me.' This was one of the best of Hollywood veteran Henry Hathaway's later films. With a brilliant score by Bernard Herrmann."
See also
the apple-tree
entries from Monday
(the date of Widmark's death)
and Tuesday, as well as
today's previous entry and
previous Log24
entries on Cuernavaca.
Comments Off on Thursday March 27, 2008
Saturday, March 8, 2008
of literary “signature passages” —

An answer:
“The whirligig of time”
— Shakespeare, Twelfth Night
and
Log24, Twelfth Night, 2006:
Hamilton’s Whirligigs

Click image to enlarge.
Related material:
Rotation in the complex plane.
The plane was discovered
in the late 1700’s by Wessel:
|
“Wessel’s paper [in Danish] was not noticed by the mathematical community until 1895… A French translation… was published in 1897 but an English translation of this most remarkable work was not published until 1999 (exactly 200 years after it was first published)….
We have called Wessel’s work remarkable, and indeed although the credit has gone to Argand, many historians of mathematics feel that Wessel’s contribution was [1]:-
… superior to and more modern in spirit to Argand’s.
In the [1] article the approaches by Argand and Wessel are compared and contrasted. Of course Wessel was a surveyor and his paper was motivated by his surveying and cartography work:-
Wessel’s development proceeded rather directly from geometric problems, through geometric-intuitive reasoning, to an algebraic formula. Argand began with algebraic quantities and sought a geometric representation for them. … Wessel’s initial formulation was remarkably clear, direct, concise and modern. It is regrettable that it was not appreciated for nearly a century and hence did not have the influence it merited.
However more is claimed for Wessel’s single mathematical paper than the first geometric interpretation of complex numbers. In [3] Crowe credits Wessel with being the first person to add vectors. Again this shows the depth of Wessel’s thinking but again, as the paper was unnoticed it had no influence on mathematical development despite appearing in the Memoirs of the Royal Danish Academy which by any standard was a major source of publications….
1. … Biography in Dictionary of Scientific Biography (New York 1970-1990).
3. M.J. Crowe, A History of Vector Analysis (Notre Dame, 1967).” |
Comments Off on Saturday March 8, 2008
Thursday, March 6, 2008
This note is prompted by the March 4 death of Richard D. Anderson, writer on geometry, President (1981-82) of the Mathematical Association of America (MAA), and member of the MAA's Icosahedron Society.
"The historical road
from the Platonic solids
to the finite simple groups
is well known."
— Steven H. Cullinane,
November 2000,
Symmetry from Plato to
the Four-Color Conjecture
Euclid is said to have remarked that "there is no royal road to geometry." The road to the end of the four-color conjecture may, however, be viewed as a royal road
from geometry to the wasteland of mathematical recreations.* (See, for instance, Ch. VIII, "Map-Colouring Problems," in
Mathematical Recreations and Essays, by
W. W. Rouse Ball and
H. S. M. Coxeter.) That road
ended in 1976 at the AMS-MAA summer meeting in Toronto– home of
H. S. M. Coxeter, a.k.a. "the king of geometry."
See also Log24, May 21, 2007.
A different road– from Plato to the finite simple groups– is, as I noted in November 2000, well known. But new roadside attractions continue to appear. One such attraction is the role played by a Platonic solid– the icosahedron– in design theory, coding theory, and the construction of the sporadic simple group M
24.
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," by Andries E. Brouwer (Ch. 14 (pp. 693-746) of
Handbook of Combinatorics, Vol. I, MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel, and László Lovász, Section 16 (p. 716))
This Steiner system is closely connected to M24 and to the extended binary Golay code. Brouwer gives an elegant construction of that code (and therefore of M24):
"Let N be the adjacency matrix of the icosahedron (points: 12 vertices, adjacent: joined by an edge). Then the rows of the 12×24 matrix (I J-N) generate the extended binary Golay code." [Here I is the identity matrix and J is the matrix of all 1's.]
— Op. cit., p. 719
Related material:
Finite Geometry of
the Square and Cube
and
Jewel in the Crown
"There is a pleasantly discursive
treatment of Pontius Pilate's
unanswered question
'What is truth?'"
— H. S. M. Coxeter, 1987,
introduction to Trudeau's
"story theory" of truth
Those who prefer stories to truth
may consult the Log24 entries
of March 1, 2, 3, 4, and 5.
They may also consult
the poet Rubén Darío:
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
Comments Off on Thursday March 6, 2008
Wednesday, March 5, 2008
For CENTRAL
Central Intelligence:
"God does not play dice."
— Paraphrase of a remark
by Albert Einstein
Another Nobel Prize winner,
Isaac Bashevis Singer—
"a God who speaks in deeds,
not in words, and whose
vocabulary is the Cosmos"
From "The Escapist:
The Reality of Fantasy Games"–
 Dungeons & Dragons Dice
Dungeons & Dragons Dice
From today's New York Times:

A Kaddish for Gygax:
|
"I was reading Durant's section on Plato, struggling to understand his theory of the ideal Forms that lay in inviolable perfection out beyond the phantasmagoria. (That was the first, and I think the last, time that I encountered that word.)"
|
Related material:
For more on the word
"phantasmagoria," see
Log24 on Dec. 12, 2004
and on Sept. 23, 2006.
For phantasmagoria in action,
see Dungeons & Dragons
and Singer's (and others')
Jewish fiction.
For non-phantasmagoria,
see (for instance) the Elements
of Euclid, which culminates
in the construction of the
Platonic solids illustrated above.
See also Geometry for Jews.
Comments Off on Wednesday March 5, 2008
Tuesday, March 4, 2008
… And for a
Swiftly Tilting
Shadowed Planet …

John Trever, Albuquerque Journal, 2/29/08
The pen's point:
Log24, Dec. 11, 2006
SINGER, ISAAC:
"Are Children the
Ultimate Literary Critics?"
— Top of the News 29
(Nov. 1972): 32-36.
"Sets forth his own aims in writing for children and laments 'slice of life' and chaos in children's literature. Maintains that children like good plots, logic, and clarity, and that they have a concern for 'so-called eternal questions.'"
— An Annotated Listing
of Criticism
by Linnea Hendrickson
"She returned the smile, then looked across the room to her youngest brother, Charles Wallace, and to their father, who were deep in concentration, bent over the model they were building of a tesseract: the square squared, and squared again: a construction of the dimension of time."
— A Swiftly Tilting Planet,
by Madeleine L'Engle

A Swiftly Tilting Planet is a fantasy for children set partly in Vespugia, a fictional country bordered by Chile and Argentina.
|
Comments Off on Tuesday March 4, 2008
Thursday, February 28, 2008
Popularity of MUB’s
From an entry today at the weblog of Lieven Le Bruyn (U. of Antwerp):
“MUBs (for Mutually Unbiased Bases) are quite popular at the moment. Kea is running a mini-series Mutual Unbias….”
The link to Kea (Marni Dee Sheppeard (pdf) of New Zealand) and a link in her Mutual Unbias III (Feb. 13) lead to the following illustration, from a talk, “Discrete phase space based on finite fields,” by William Wootters at the Perimeter Institute in 2005:

This illustration makes clear the
close relationship of MUB’s to the
finite geometry of the 4×4 square.
The Wootters talk was on
July 20, 2005. For related material from that July which some will find more entertaining, see “Steven Cullinane is a Crank,” conveniently reproduced as
a five-page thread in the Mathematics Forum at groupsrv.com.
Comments Off on Thursday February 28, 2008
Tuesday, February 26, 2008
Eight is a Gate (continued)
|
"To begin at the beginning: Is God?…" [very long pause]
|

|
From "Space," by Salomon Bochner
Makom. Our term “space” derives from the Latin, and is thus relatively late. The nearest to it among earlier terms in the West are the Hebrew makom and the Greek topos (τόπος). The literal meaning of these two terms is the same, namely “place,” and even the scope of connotations is virtually the same (Theol. Wörterbuch…, 1966). Either term denotes: area, region, province; the room occupied by a person or an object, or by a community of persons or arrangements of objects. But by first occurrences in extant sources, makom seems to be the earlier term and concept. Apparently, topos is attested for the first time in the early fifth century B.C., in plays of Aeschylus and fragments of Parmenides, and its meaning there is a rather literal one, even in Parmenides. Now, the Hebrew book Job is more or less contemporary with these Greek sources, but in chapter 16:18 occurs in a rather figurative sense:
O earth, cover not thou my blood, and let my cry have no place (makom).
Late antiquity was already debating whether this makom is meant to be a “hiding place” or a “resting place” (Dhorme, p. 217), and there have even been suggestions that it might have the logical meaning of “occasion,” “opportunity.” Long before it appears in Job, makom occurs in the very first chapter of Genesis, in:
And God said, Let the waters under the heaven be gathered together unto one place (makom) and the dry land appear, and it was so (Genesis 1:9).
This biblical account is more or less contemporary with Hesiod's Theogony, but the makom of the biblical account has a cosmological nuance as no corresponding term in Hesiod. Elsewhere in Genesis (for instance, 22:3; 28:11; 28:19), makom usually refers to a place of cultic significance, where God might be worshipped, eventually if not immediately. Similarly, in the Arabic language, which however has been a written one only since the seventh century A.D., the term makām designates the place of a saint or of a holy tomb (Jammer, p. 27). In post-biblical Hebrew and Aramaic, in the first centuries A.D., makom became a theological synonym for God, as expressed in the Talmudic sayings: “He is the place of His world,” and “His world is His place” (Jammer, p. 26). Pagan Hellenism of the same era did not identify God with place, not noticeably so; except that the One (τὸ ἕν) of Plotinus (third century A.D.) was conceived as something very comprehensive (see for instance J. M. Rist, pp. 21-27) and thus may have been intended to subsume God and place, among other concepts. In the much older One of Parmenides (early fifth century B.C.), from which the Plotinian One ultimately descended, the theological aspect was only faintly discernible. But the spatial aspect was clearly visible, even emphasized (Diels, frag. 8, lines 42-49).
BIBLIOGRAPHY
Paul Dhorme, Le livre de Job (Paris, 1926).
H. Diels and W. Kranz, Die Fragmente der Vorsokratiker, 6th ed. (Berlin, 1938).
Max Jammer, Concepts of Space… (Cambridge, Mass., 1954).
J. M. Rist, Plotinus: The Road to Reality (Cambridge, 1967).
Theologisches Wörterbuch zum Neuen Testament (1966), 8, 187-208, esp. 199ff.
— SALOMON BOCHNER
|
Related material: In the previous entry — "Father Clark seizes at one place (page eight)
upon the fact that…."
Father Clark's reviewer (previous entry) called a remark by Father Clark "far fetched."
This use of "place" by the reviewer is, one might say, "near fetched."
Comments Off on Tuesday February 26, 2008
The Just Word
The title of the previous entry, "Where Entertainment is God," comes (via Log24, Nov. 26, 2004) from Frank Rich.
The previous entry dealt, in part, with a dead Jesuit whose obituary appears in today's Los Angeles Times. The online obituaries page places the Jesuit, without a photo, beneath a picture of a dead sitcom writer and to the left of a picture of a dead guru.
"Walter John Burghardt was born July 10, 1914, in New York, the son of immigrants from what is now Poland. He entered a Jesuit seminary in Poughkeepsie, N.Y., at 16, and in 1937 received a master's degree from Woodstock College in Maryland. He was ordained in 1941." He died, by the way, on Saturday, Feb. 16, 2008.
The reference to Woodstock College brings to mind a fellow Jesuit, Joseph T. Clark, who wrote a book on logic published by that college.
From a review of the book:
"In order to show that Aristotelian logicians were at least vaguely aware of a kind of analogy or possible isomorphism between logical relations and mathematical relations, Father Clark seizes at one place (p. 8) upon the fact that Aristotle uses the word, 'figure' (schema), in describing the syllogism and concludes from this that 'it is obvious that the schema of the syllogism is to serve the logician precisely as the figure serves the geometer.' On the face of it, this strikes one as a bit far fetched…."
— Henry Veatch in Speculum, Vol. 29, No. 2, Part 1 (Apr., 1954), pp. 266-268 (review of Conventional Logic and Modern Logic: A Prelude to Transition (1952), by Joseph T. Clark, Society of Jesus)
Comments Off on Tuesday February 26, 2008
Monday, February 25, 2008
Comments Off on Monday February 25, 2008
Saturday, February 23, 2008
Thanks to a Virginia reader for a reminder:
An Exercise
of Power
Johnny Cash:
"And behold,
a white horse."

Chess Knight
(in German, Springer)
"
Liebe Frau vBayern,
mich würde interessieren wie man
mit diesem Hintergrund
(vonbayern.de/german/anna.html)
zu Springer kommt?"
Background of "Frau vBayern" from thePeerage.com:
Anna-Natascha Prinzessin zu Sayn-Wittgenstein-Berleburg
F, #64640, b. 15 March 1978Last Edited=20 Oct 2005
The date of the above "Liebe Frau vBayern" inquiry, Feb. 1, 2007, suggests the following:
From Log24 on
St. Bridget's Day, 2007:
The quotation
"Science is a Faustian bargain"
and the following figure–
Change

From a short story by
the above Princess:
"'I don't even think she would have wanted to change you. But she for sure did not want to change herself. And her values were simply a part of her.' It was true, too. I would even go so far as to say that they were her basis, if you think about her as a geometrical body. That's what they couldn't understand, because in this age of the full understanding for stretches of values in favor of self-realization of any kind, it was a completely foreign concept."
To make this excellent metaphor mathematically correct,
change "geometrical body" to "space"… as in
"For Princeton's Class of 2007"—
Review of a 2004 production of a 1972 Tom Stoppard play, "Jumpers"–

Related material:
Knight Moves (Log24, Jan. 16),
Kindergarten Theology (St. Bridget's Day, 2008),
and

(Click on image for details.)
Comments Off on Saturday February 23, 2008
Saturday, February 16, 2008
Bridges
Between Two Worlds
From the world of mathematics…
“… my advisor once told me, ‘If you ever find yourself drawing one of those meaningless diagrams with arrows connecting different areas of mathematics, it’s a good sign that you’re going senile.'”
— Scott Carnahan at Secret Blogging Seminar, December 14, 2007
Carnahan’s remark in context:
“About five years ago, Cheewhye Chin gave a great year-long seminar on Langlands correspondence for GLr over function fields…. In the beginning, he drew a diagram….
If we remove all of the explanatory text, the diagram looks like this:
I was a bit hesitant to draw this, because my advisor once told me, ‘If you ever find yourself drawing one of those meaningless diagrams with arrows connecting different areas of mathematics, it’s a good sign that you’re going senile.’ Anyway, I’ll explain roughly how it works.
Langlands correspondence is a ‘bridge between two worlds,’ or more specifically, an assertion of a bijection….”
Compare and contrast the above…
… to the world of Rudolf Kaehr:

The above reference to “diamond theory” is from Rudolf Kaehr‘s paper titled Double Cross Playing Diamonds.
Another bridge…
Carnahan’s advisor, referring to “meaningless diagrams with arrows connecting different areas of mathematics,” probably did not have in mind diagrams like the two above, but rather diagrams like the two below–
From the world of mathematics…

“A rough sketch of
how diamond theory is
related to some other
fields of mathematics”
— Steven H. Cullinane
Related material:
For further details on
the “diamond theory” of
Cullinane, see
Finite Geometry of the
Square and Cube.
For further details on
the “diamond theory” of
Kaehr, see
Rudy’s Diamond Strategies.
Those who prefer entertainment
may enjoy an excerpt
from Log24, October 2007:
Comments Off on Saturday February 16, 2008
Friday, February 1, 2008
Kindergarten Theology
On the late
James Edwin Loder,
a Presbyterian minister and
a professor of Christian education
at Princeton Theological Seminary,
co-author of
The Knight’s Move (1992):
“At his memorial service his daughter Tami told the story of ‘little Jimmy,’ whose kindergarten teacher recognized a special quality of mind that set him apart. ‘Every day we read a story, and after the story is over, Jimmy gets up and wants to tell us what the story means.'” —
Dana R. WrightFor a related story about
knight moves and kindergarten,
see Knight Moves: The Relativity
Theory of Kindergarten Blocks,
and Log24, Jan. 16, 17, and 18.
See also Loder’s book
(poorly written, but of some
interest in light of the above):

Opening of
The Knight’s Move —
“In a game of chess, the knight’s move is unique because it alone goes around corners. In this way, it combines the continuity of a set sequence with the discontinuity of an unpredictable turn in the middle. This meaningful combination of continuity and discontinuity in an otherwise linear set of possibilities has led some to refer to the creative act of discovery in any field of research as a ‘knight’s move’ in intelligence.
The significance of the title of this volume might stop there but for Kierkegaard’s use of the ‘knight’ image. The force of Kierkegaards’s usage might be described in relation to the chess metaphor by saying that not merely does Kierkegaard’s ‘knight of faith’ undertake a unique move within the rules of the human game, but faith transposes the whole idea of a ‘knight’s move’ into the mind of the Chess Master Himself. That is to say, chess is a game of multiple possibilities and interlocking strategies, so a chess master must combine the continuity represented by the whole complex of the game with the unpredictable decision he must make every time it is his turn. A master chess player, then, does not merely follow the rules; in him the game becomes a construct of consciousness. The better the player the more fully the game comes into its own as a creation of human intelligence. Similarly, for Kierkegaard, the knight of faith is a unique figure in human experience. The knight shows how, by existing in faith as a creative act of Christ’s Spirit, human existence comes into its own as an expression of the mind of Christ. Thus, the ultimate form of a ‘knight’s move’ is a creative act raised to the nth power by
Spiritus Creator, but it still partakes fully in the concrete pieces and patterns that comprise the nature of the human game and the game of nature.”
— James E. Loder and W. Jim Neidhardt (Helmers & Howard Publishing, 1992)
For a discussion, see Triplett’s
“Thinking Critically as a Christian.”
Many would deny that such
a thing is possible; let them
read the works of T. S. Eliot.
Related material:
The Knight’s Move
discusses (badly) Hofstadter’s
“strange loop” concept; see
Not Mathematics but Theology
(Log24, July 12, 2007).
Comments Off on Friday February 1, 2008
Wednesday, January 16, 2008
Knight Moves:
Geometry of the
Eightfold Cube

Click on the image for a larger version
and an expansion of some remarks
quoted here on Christmas 2005.
Comments Off on Wednesday January 16, 2008
Sunday, January 6, 2008
Comments Off on Sunday January 6, 2008
Friday, December 7, 2007
Comments Off on Friday December 7, 2007
Comments Off on Friday December 7, 2007
Sunday, November 4, 2007
Talking of Michelangelo:
High Concept
On this date in 1948, T. S. Eliot
won the Nobel Prize in Literature.

Non ha l’ottimo artista in se alcun concetto,
Ch’un marmo solo in se non circoscriva
Col suo soverchio; e solo a quello arriva
La man che ubbidisce all’intelletto.
(The best artist has in himself no concept
in a single block of marble not contained;
only the hand obeying mind will find it.)
— Michelangelo, as quoted
Idea: A Concept in Art Theory
Comments Off on Sunday November 4, 2007
Thursday, October 25, 2007
Something Anonymous

Yesterday:
Nineteenth-century quilt design:

Related material:
Battlefield Geometry
Comments Off on Thursday October 25, 2007
Wednesday, October 24, 2007
Descartes’s Twelfth Step
An earlier entry today (“Hollywood Midrash continued“) on a father and son suggests we might look for an appropriate holy ghost. In that context…
Descartes
A search for further background on Emmanuel Levinas, a favorite philosopher of the late R. B. Kitaj (previous two entries), led (somewhat indirectly) to the following figures of Descartes:
This trinity of figures is taken from Descartes’
Rule Twelve in
Rules for the Direction of the Mind. It seems to be meant to suggest an analogy between superposition of colors and superposition of shapes.Note that the first figure is made up of vertical lines, the second of vertical and horizontal lines, and the third of vertical, horizontal, and diagonal lines.
Leon R. Kass recently suggested that the Descartes figures might be replaced by a more modern concept– colors as wavelengths. (
Commentary, April 2007). This in turn suggests an analogy to Fourier series decomposition of a waveform in harmonic analysis. See the Kass essay for
a discussion of the Descartes figures in the context of (pdf)
Science, Religion, and the Human Future (not to be confused with
Life, the Universe, and Everything).
Compare and contrast:
The harmonic-analysis analogy suggests a review of an earlier entry’s
link today to 4/30– Structure and Logic— as well as
re-examination of Symmetry and a Trinity

(Dec. 4, 2002).
See also —
A Four-Color Theorem,
The Diamond Theorem, and
The Most Violent Poem,

from Mike Nichols’s birthday, 2003.
Comments Off on Wednesday October 24, 2007
Wednesday, October 3, 2007
Janitor Monitor

Will Hunting may be
interested in the following
vacant editorships at
The Open Directory:
Graph Theory
and
Combinatorics.
Related material:
The Long Hello and
On the Holy Trinity —
"Hey, Carrie-Anne, what's
your game now….?"

Picture sources:
azstarnet.com,
vibrationdata.com.
Personally, I prefer
Carol Ann:
From Criticism, Fall, 2001,
by Carol Ann Johnston—
"Drawing upon Platonic thought, Augustine argues that ideas are actually God's objective pattern and as such exist in God's mind. These ideas appear in the mirror of the soul. (35)."
(35.) In Augustine, De Trinitate, trans., Stephen McKenna (Washington, D.C.: Catholic University Press, 1970). See A. B. Acton, "Idealism," in The Encyclopedia of Philosophy, ed., Paul Edwards. Vol. 4 (New York: Macmillan, 1967): 110-118; Robert McRae, "`Idea' as a Philosophical Term in the Seventeenth Century," JHI 26 (1965): 175-190, and Erwin Panofsky, Idea: A Concept in Art History, trans., Joseph J. S. Peake (Columbia, S.C.: University of South Carolina Press, 1968) for explications of this term.
|
Comments Off on Wednesday October 3, 2007
Monday, October 1, 2007
Bright as Magnesium
"Definitive"
— The New York Times,
Sept. 30, 2007, on
Blade Runner:
The Final Cut
Institute for Advanced Study, Princeton, N.J.—
"The art historian Kirk Varnedoe died on August 14, 2003, after a long and valiant battle with cancer. He was 57. He was a faculty member in the Institute for Advanced Study’s School of Historical Studies, where he was the fourth art historian to hold this prestigious position, first held by the German Renaissance scholar Erwin Panofsky in the 1930s."
Hal Crowther—
"His final lecture was an eloquent, prophetic flight of free association….
Varnedoe chose to introduce his final lecture with the less-quoted last words of the android Roy Batty (Rutger Hauer) in Ridley Scott's film Blade Runner: 'I've seen things you people wouldn't believe– attack ships on fire off the shoulder of Orion, bright as magnesium; I rode on the back decks of a blinker and watched C-beams glitter in the dark near the Tannhauser Gate. All those moments will be lost in time, like tears in the rain. Time to die.'"
Related material:
tears in the rain–
Game Over
(Nov. 5, 2003):
Comments Off on Monday October 1, 2007
Friday, September 28, 2007
Comments Off on Friday September 28, 2007
Thursday, September 27, 2007
This afternoon I added a new page to finitegeometry.org and updated the Geometry of Logic page. These changes are due to my coming across the Usenet postings of Carol von der Lin.
Comments Off on Thursday September 27, 2007
Thursday, September 13, 2007
Scorsese Is
Kennedy Center
Honoree
“Scorsese, 64, a native New Yorker, thought of being a priest and went to the seminary after high school. But he changed his mind and built a catalogue of great films, many of which are considered the best of their time.” —
Washington Post, Sept. 12, 2007
His Life.

My Card.

Columbus Day, 2005
Click on image to enlarge.
Comments Off on Thursday September 13, 2007
Wednesday, September 12, 2007
Vector Logic

I learned yesterday from
Jonathan Westphal, a professor of philosophy at Idaho State University, that he and a colleague, Jim Hardy, have devised another geometric approach to logic: a system of arrow diagrams that illustrate classical propositional logic. The diagrams resemble those used to illustrate Euclidean vector spaces, and Westphal and Hardy call their approach “a vector system,” although it does not involve what a mathematician would regard as a vector space.
Journal of Logic and Computation
15(5) (October, 2005), pp. 751-765.
Related material:
(2) the quilt pattern
below (click for
the source) —
and
(3) yesterday’s entry
“Christ! What are
patterns for?”
Comments Off on Wednesday September 12, 2007
Tuesday, September 11, 2007
Battlefield Geometry
"The general, who wrote the Army's book on counterinsurgency, said he and his staff were 'trying to do the battlefield geometry right now' as he prepared his troop-level recommendations."
— Steven R. Hurst, The Associated Press, Wednesday, Aug. 15, 2007
"'… we are in the process of doing the battlefield geometry to determine the way ahead.'"
— Charles M. Sennott, Boston Globe, Friday, Sept. 7, 2007
"Based on these considerations, and having worked the battlefield geometry … I have recommended a drawdown of the surge forces from Iraq."
— United States Army, Monday, Sept. 10, 2007
Related material:
Log24 entries of
June 11 and 12, 2005:

"In the desert you can
remember your name
'Cause there ain't no one
for to give you no pain."
Monday, September 10, 2007
The Story Theory
of Truth —
“I’m a gun for hire,
I’m a saint, I’m a liar,
because there are no facts,
there is no truth,
just data to be manipulated.”
— The Garden of Allah
Data

The data in more poetic form:
To 23,
For 16.
Commentary:
23: See
The Prime Cut Gospel.
16: See
Happy Birthday, Benedict XVI.
Related material:
The remarks yesterday
of Harvard president
Drew G. Faust
to incoming freshmen.
Faust “encouraged
the incoming class
to explore Harvard’s
many opportunities.
‘Think of it as
a treasure room
of hidden objects
Harry discovers
at Hogwarts,’
Faust said.”
— Today’s Crimson
For a less Faustian approach,
see the Harvard-educated
philosopher Charles Hartshorne
at The Harvard Square Library
and the words of another
Harvard-educated Hartshorne:
“Whenever one
approaches a subject from
two different directions,
there is bound to be
an interesting theorem
expressing their relation.”
— Robin Hartshorne
Sunday, September 2, 2007
Re: This Week’s Finds in Mathematical Physics (Week 251)
On Spekkens’ toy system and finite geometry
Background–
- In “Week 251” (May 5, 2007), John wrote:
“Since Spekkens’ toy system resembles a qubit, he calls it a “toy bit”. He goes on to study systems of several toy bits – and the charming combinatorial geometry I just described gets even more interesting. Alas, I don’t really understand it well: I feel there must be some mathematically elegant way to describe it all, but I don’t know what it is…. All this is fascinating. It would be nice to find the mathematical structure that underlies this toy theory, much as the category of Hilbert spaces underlies honest quantum mechanics.”
- In the n-Category Cafe ( May 12, 2007, 12:26 AM, ) Matt Leifer wrote:
“It’s crucial to Spekkens’ constructions, and particularly to the analog of superposition, that the state-space is discrete. Finding a good mathematical formalism for his theory (I suspect finite fields may be the way to go) and placing it within a comprehensive framework for generalized theories would be very interesting.”
- In the n-category Cafe ( May 12, 2007, 6:25 AM) John Baez wrote:
“Spekkens and I spent an afternoon trying to think about his theory as quantum mechanics over some finite field, but failed — we almost came close to proving it couldnt’ work.”
On finite geometry:
The actions of permutations on a 4 × 4 square in Spekkens’ paper (quant-ph/0401052), and Leifer’s suggestion of the need for a “generalized framework,” suggest that finite geometry might supply such a framework. The geometry in the webpage John cited is that of the affine 4-space over the two-element field.
Related material:
Update of
Sept. 5, 2007
See also arXiv:0707.0074v1 [quant-ph], June 30, 2007:
A fully epistemic model for a local hidden variable emulation of quantum dynamics,
by Michael Skotiniotis, Aidan Roy, and Barry C. Sanders, Institute for Quantum Information Science, University of Calgary. Abstract: "In this article we consider an augmentation of Spekkens’ toy model for the epistemic view of quantum states [1]…."
Hypercube from the Skotiniotis paper:

Reference:
Comments Off on Sunday September 2, 2007
Tuesday, August 21, 2007
Comments Off on Tuesday August 21, 2007
Monday, August 20, 2007
Comments Off on Monday August 20, 2007
Monday, August 13, 2007
Adam Gopnik in
The New Yorker of
August 20, 2007–
On Philip K. Dick:
"… the kind of guy who can't drink one cup of coffee without drinking six, and then stays up all night to tell you what Schopenhauer really said and how it affects your understanding of Hitchcock and what that had to do with Christopher Marlowe."
Modernity: A Film by
Alfred Hitchcock:
"… the most thoroughgoing modernist design element in Hitchcock's films arises out of geometry, as Francois Regnault has argued, identifying 'a global movement for each one, or a "principal geometric or dynamic form," which can appear in the pure state in the credits….'" –Peter J. Hutchings (my italics)
More >>
Epilogue:
Adam Gopnik is also the author
of The King in the Window, a tale
of the Christian feast of Epiphany
and a sinister quantum computer.
For more on Epiphany, see
the Log24 entries of August 1.
For more on quantum computing,
see What is Quantum Computation?.
See also
the previous entry.
Comments Off on Monday August 13, 2007
Sunday, August 12, 2007
In the context of quantum information theory, the following structure seems to be of interest–
"… the full two-by-two matrix ring with entries in GF(2), M2(GF(2))– the unique simple non-commutative ring of order 16 featuring six units (invertible elements) and ten zero-divisors."
— "Geometry of Two-Qubits," by Metod Saniga (pdf, 17 pp.), Jan. 25, 2007
This ring is another way of looking at the 16 elements of the affine space A4(GF(2)) over the 2-element field. (Arrange the four coordinates of each element– 1's and 0's– into a square instead of a straight line, and regard the resulting squares as matrices.) (For more on A4(GF(2)), see Finite Relativity and related notes at Finite Geometry of the Square and Cube.) Using the above ring, Saniga constructs a system of 35 objects (not unlike the 35 lines of the finite geometry PG(3,2)) that he calls a "projective line" over the ring. This system of 35 objects has a subconfiguration isomorphic to the (2,2) generalized quadrangle W2 (which occurs naturally as a subconfiguration of PG(3,2)– see Inscapes.)
Saniga concludes:
"We have demonstrated that the basic properties of a system of two interacting spin-1/2 particles are uniquely embodied in the (sub)geometry of a particular projective line, found to be equivalent to the generalized quadrangle of order two. As such systems are the simplest ones exhibiting phenomena like quantum entanglement and quantum non-locality and play, therefore, a crucial role in numerous applications like quantum cryptography, quantum coding, quantum cloning/teleportation and/or quantum computing to mention the most salient ones, our discovery thus
-
not only offers a principally new geometrically-underlined insight into their intrinsic nature,
-
but also gives their applications a wholly new perspective
-
and opens up rather unexpected vistas for an algebraic geometrical modelling of their higher-dimensional counterparts."
is not without relevance to
the physics of quantum theory.
Comments Off on Sunday August 12, 2007
Friday, August 10, 2007
The Ring of Gyges
10:31:32 AM ET
Commentary by Richard Wilhelm
on I Ching Hexagram 32:
“Duration is… not a state of rest, for mere standstill is regression.
Duration is rather the self-contained and therefore self-renewing
movement of an organized, firmly integrated whole, taking place in
accordance with immutable laws and beginning anew at every ending.”
Related material

Jung and the Imago Dei
“Not Being There,”
by Christopher Caldwell,
from next Sunday’s
New York Times Magazine:
| “The chance to try on fresh identities was the great boon that life online was supposed to afford us. Multiuser role-playing games and discussion groups would be venues for living out fantasies. Shielded by anonymity, everyone could now pass a ‘second life’ online as Thor the Motorcycle Sex God or the Sage of Wherever. Some warned, though, that there were other possibilities. The Stanford Internet expert Lawrence Lessig likened online anonymity to the ring of invisibility that surrounds the shepherd Gyges in one of Plato’s dialogues. Under such circumstances, Plato feared, no one is ‘of such an iron nature that he would stand fast in justice.’Time, along with a string of sock-puppet scandals, has proved Lessig and Plato right.” |
“The Boy Who Lived,”
by Christopher Hitchens,
from next Sunday’s
New York Times Book Review:
| On the conclusion of the Harry Potter series:”The toys have been put firmly back in the box, the wand has been folded up, and the conjuror is discreetly accepting payment while the children clamor for fresh entertainments. (I recommend that they graduate to Philip Pullman, whose daemon scheme is finer than any patronus.)” |
I, on the other hand,
recommend Tolkien…
or, for those who are
already familiar with
Tolkien, Plato– to whom
“The Ring of Gyges” may
serve as an introduction.
“It’s all in Plato, all in Plato:
bless me, what do they
teach them at these schools!”
— C. S. Lewis
Comments Off on Friday August 10, 2007
Wednesday, August 1, 2007
Comments Off on Wednesday August 1, 2007
August First,
8:00:14 AM:
Cheap Epiphany
|
SPORTS OF THE TIMES
Restoring the Faith
After Hitting the Bottom
By SELENA ROBERTS
The New York Times
Published: August 1, 2007
What good is a nadir if it's denied or ignored? What's the value of reaching the lowest of the low if it can't buy a cheap epiphany?
|
Pennsylvania Lottery
on the Feast of
St. Ignatius Loyola:
|

|

|
Restoring the Booze:
A Look at the 50's-

Another Epiphany:

Box-style I Ching, January 6, 1989
(Click on image for background.)
Detail:

Related material:
Logos and Logic
and Diagon Alley.
"What a swell
party this is."
— adapted from
Cole Porter
Comments Off on Wednesday August 1, 2007
Monday, July 30, 2007
The Deathly Hallows Symbol

Some fear that the Harry Potter books introduce children to the occult; they are not entirely mistaken.
According to Wikipedia, the “Deathly Hallows” of the final Harry Potter novel are “three fictional magical objects that appear in the book.”
The vertical line, circle, and triangle in the symbol pictured above are said to refer to these three magical objects.
One fan relates the “Deathly Hallows” symbol above, taken from the spine of a British children’s edition of the book, to a symbol for “the divine (or sacred, or secret) fire” of alchemy. She relates this fire in turn to “serpent power” and the number seven:
Kristin Devoe at a Potter fan site:
“We know that seven is a powerful number in the novels. Tom Riddle calls it ‘the most powerfully magic number.‘ The ability to balance the seven chakras within oneself allows the person to harness the secret fire. This secret fire in alchemy is the same as the kundalini or coiled snake in yogic philosophy. It is also known as ‘serpent power’ or the ‘dragon’ depending on the tradition. The kundalini is polar in nature and this energy, this internal fire, is very powerful for those who are able to harness it and it purifies the aspirant allowing them the knowledge of the universe. This secret fire is the Serpent Power which transmutes the base metals into the Perfect Gold of the Sun.
It is interesting that the symbol of the caduceus in alchemy is thought to have been taken from the symbol of the kundalini. Perched on the top of the caduceus, or the staff of Hermes, the messenger of the gods and revealer of alchemy, is the golden snitch itself! Many fans have compared this to the scene in The Order of the Phoenix where Harry tells Dumbledore about the attack on Mr. Weasley and says, ‘I was the snake, I saw it from the snake’s point of view.‘
The chapter continues with Dumbledore consulting ‘one of the fragile silver instruments whose function Harry had never known,’ tapping it with his wand:
The instrument tinkled into life at once with rhythmic clinking noises. Tiny puffs of pale green smoke issued from the minuscule silver tube at the top. Dumbledore watched the smoke closely, his brow furrowed, and after a few seconds, the tiny puffs became a steady stream of smoke that thickened and coiled into he air… A serpent’s head grew out of the end of it, opening its mouth wide. Harry wondered whether the instrument was confirming his story; He looked eagerly at Dumbledore for a sign that he was right, but Dumbledore did not look up.
“Naturally, Naturally,” muttered Dumbledore apparently to himself, still observing the stream of smoke without the slightest sign of surprise. “But in essence divided?”
Harry could make neither head not tail of this question. The smoke serpent, however split instantly into two snakes, both coiling and undulating in the dark air. With a look of grim satisfaction Dumbledore gave the instrument another gentle tap with his wand; The clinking noise slowed and died, and the smoke serpents grew faint, became a formless haze, and vanished.
Could these coiling serpents of smoke be foreshadowing events to come in Deathly Hallows where Harry learns to ‘awaken the serpent’ within himself? Could the snake’s splitting in two symbolize the dual nature of the kundalini?”
Related material
The previous entry—
and the following
famous illustration of
the double-helix
structure of DNA:

This is taken from
a figure accompanying
an obituary, in today’s
New York Times, of the
artist who drew the figure.
The double helix
is not a structure
from magic; it may,
however, as the Rowling
quote above shows, have
certain occult uses,
better suited to
Don Henley’s
Garden of Allah
than to the
Garden of Apollo.

Similarly, the three objects
above (Log24 on April 9)
are from pure mathematics–
the realm of Apollo, not
of those in Henley’s song.
The similarity of the
top object of the three —
the “Fano plane” — to
the “Deathly Hallows”
symbol is probably
entirely coincidental.
Comments Off on Monday July 30, 2007
Sunday, July 29, 2007
Comments Off on Sunday July 29, 2007
Saturday, July 28, 2007
The Third Cross
“… he triumphed again with The Goldberg Variations. Mr Jay, assisted by Goldberg, a concentration camp survivor, is rehearsing a montage of biblical scenes in Jerusalem. It is inspired satire, laced with Jewish and Christian polemics, sparkling wit and dazzlingly simple effects. For Golgotha a stagehand brings on three crosses. ‘Just two,’ says Jay. ‘The boy is bringing his own.’ Tabori often claimed that the joke was the most perfect literary form.”
Comments Off on Saturday July 28, 2007
Wednesday, July 25, 2007
The Comedy of
George Tabori

From AP “Obituaries in the News”–
Filed with The New York Times
at 11:16 p.m. ET July 24, 2007–
George Tabori
“BERLIN (AP) — Hungarian-born playwright and director George Tabori, a legend in Germany’s postwar theater world whose avant-garde works confronted anti-Semitism, died Monday [July 23, 2007]. He was 93.
Tabori, who as recently as three years ago dreamed of returning to stage to play the title role in Shakespeare’s ‘King Lear,’ died in his apartment near the theater, the Berliner Ensemble said Tuesday, noting that friends and family had accompanied him through his final days. No cause of death was given.
Born into a Jewish family in Budapest on May 24, 1914, Tabori fled in 1936 to London, where he started working for the British Broadcasting Corp., and became a British citizen. His father, and other members of his family, were killed at Auschwitz.
Tabori moved to Hollywood in the 1950s, where he worked as a scriptwriter, most notably co-writing the script for Alfred Hitchcock’s 1953 film, ‘I Confess.’
He moved to Germany in the 1970s and launched a theater career that spanned from acting to directing to writing. He used sharp wit and humor in his plays to examine the relationship between Germany and the Jews, as well as attack anti-Semitism.
Among his best-known works are ‘Mein Kampf,’ set in the Viennese hostel where Adolf Hitler lived from 1910-1913, and the ‘Goldberg Variations,’ both dark farces that poke fun at the Nazis.”
From Year of Jewish Culture:
“The year 2006 marks the 100th anniversary of the establishment of the Jewish Museum in Prague.”
From the related page Programme (October-December):
“Divadlo v Dlouhé
George Tabori: GOLDBERGOVSKÉ VARIACE / THE GOLDBERG VARIATIONS, 19 October, 7 p.m. A comedy on creation and martyrdom.”
Variations on
Birth and Death
|
From Log24 on the date of
the Prague production of the
Tabori “Goldberg Variations,”
an illustration in honor of
Sir Thomas Browne, who
was born, and died,
on that date:
|
|
Theme
(Plato, Meno)

and Variations:
Click on “variations” above
for some material on
the “Goldberg Variations”
of Johann Sebastian Bach.
|
Comments Off on Wednesday July 25, 2007
Monday, July 23, 2007
Daniel Radcliffe
is 18 today.
Greetings.
“The greatest sorcerer (writes Novalis memorably)
would be the one who bewitched himself to the point of
taking his own phantasmagorias for autonomous apparitions.
Would not this be true of us?”
–Jorge Luis Borges, “Avatars of the Tortoise”
“El mayor hechicero (escribe memorablemente Novalis)
sería el que se hechizara hasta el punto de
tomar sus propias fantasmagorías por apariciones autónomas.
¿No sería este nuestro caso?”
–Jorge Luis Borges, “Los Avatares de la Tortuga“
Autonomous Apparition
At Midsummer Noon:
“In Many Dimensions (1931)
Williams sets before his reader the
mysterious Stone of King Solomon,
an image he probably drew from
a brief description in Waite’s
The Holy Kabbalah (1929) of
a supernatural cubic stone
on which was inscribed
‘the Divine Name.’”
Related material:
|
It is not enough to cover the rock with leaves.
We must be cured of it by a cure of the ground
Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And yet the leaves, if they broke into bud,
If they broke into bloom, if they bore fruit,
And if we ate the incipient colorings
Of their fresh culls might be a cure of the ground.
– Wallace Stevens, “The Rock”
|
See also
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions by
some central solid essence.
I tried to reconstruct
the central object, and
came up with this book.”
 Hofstadter’s cover.
Hofstadter’s cover.
Here are three patterns,
“shadows” of a sort,
derived from a different
“central object”:
Click on image for details.
Comments Off on Monday July 23, 2007
Today’s Birthday:
Daniel Radcliffe
(“Harry Potter”)
Theme
(Plato, Meno)

and Variations:

Click on picture for details.
“A diamond jubilance
beyond the fire,
That gives its power
to the wild-ringed eye”
— Wallace Stevens,
“The Owl in the Sarcophagus”
Comments Off on Monday July 23, 2007
Friday, July 13, 2007
Today’s birthday:
Harrison Ford is 65.

“Three times the concentred
self takes hold, three times
The thrice concentred self,
having possessed
The object, grips it
in savage scrutiny,
Once to make captive,
once to subjugate
Or yield to subjugation,
once to proclaim
The meaning of the capture,
this hard prize,
Fully made, fully apparent,
fully found.”
— “Credences of Summer,” VII,
by Wallace Stevens, from
Transport to Summer (1947) |
“It was Plato who best expressed– who veritably embodied– the tension between the narrative arts and mathematics….
Plato clearly loved them both, both mathematics and poetry. But he approved of mathematics, and heartily, if conflictedly, disapproved of poetry. Engraved above the entrance to his Academy, the first European university, was the admonition: Oudeis ageometretos eiseto. Let none ignorant of geometry enter. This is an expression of high approval indeed, and the symbolism could not have been more perfect, since mathematics was, for Plato, the very gateway for all future knowledge. Mathematics ushers one into the realm of abstraction and universality, grasped only through pure reason. Mathematics is the threshold we cross to pass into the ideal, the truly real.”
Comments Off on Friday July 13, 2007
Monday, July 9, 2007
Harry Potter and
the Xbox 360
Harry Potter and the Order of The Phoenix for Xbox 360 “is based on the fifth book and is timed to coincide with the release of the movie of the same name…. The game consists of Harry walking around and talking to characters and performing spells and tasks in order to advance the plot. I jokingly considered calling this review ‘Harry Potter and the Order of the Random Tasks Needed to Advance the Plot.'” —July 9 review at Digital Joystick
Today’s lottery numbers
in the Keystone State:
Mid-day 220
Evening 034
Related material:
2/20 and
Hexagram 34 in the
box-style I Ching:

The Power
of the Great
Let us hope that Harry fans remember the meaning of Hexagram 34 (
according to Richard Wilhelm)– “Perseverance furthers” and “That is truly great power which does not degenerate into mere force but remains inwardly united with the fundamental principles of right and of justice. When we understand this point– namely, that greatness and justice must be indissolubly united– we understand the true meaning of all that happens in heaven and on earth.”
Comments Off on Monday July 9, 2007
Friday, July 6, 2007
Comments Off on Friday July 6, 2007
Midnight in the Garden
of Good and Evil
continued from
Midsummer Night…
“The voodoo priestess looked across the table at her wealthy client, a man on trial for murder: ‘Now, you know how dead time works. Dead time lasts for one hour– from half an hour before midnight to half an hour after midnight. The half-hour before midnight is for doin’ good. The half-hour after midnight is for doin’ evil….'”
— Glenna Whitley, “Voodoo Justice,”
The New York Times, March 20, 1994

In Other Game News:
“In June, bloggers speculated that
the Xbox 360 return problem was getting so severe that the company was running out of ‘coffins,’ or special return-shipping boxes Microsoft provides to gamers with dead consoles. ‘We’ll make sure we have plenty of boxes to go back and forth,’ Bach said in an interview.”
Comments Off on Friday July 6, 2007
Thursday, July 5, 2007
In Defense of
Plato’s Realism
(vs. sophists’ nominalism–
see recent entries.)
Plato cited geometry,
notably in the Meno,
in defense of his realism.
Consideration of the
Meno’s diamond figure
leads to the following:

Click on image for details.
As noted in an entry,
Plato, Pegasus, and
the Evening Star,
linked to
at the end of today’s
previous entry,
the “universals”
of Platonic realism
are exemplified by
the hexagrams of
the I Ching,
which in turn are
based on the seven
trigrams above and
on the eighth trigram,
of all yin lines,
not shown above:

K’un
The Receptive
_____________________________________________
Update of Nov. 30, 2013:
From a little-known website in Kuala Lumpur:
(Click to enlarge.)

The remarks on Platonic realism are from Wikipedia.
The eightfold cube is apparently from this post.
Comments Off on Thursday July 5, 2007
Monday, July 2, 2007
A figure like Ecclesiast/
Rugged and luminous,
chants in the dark/
A text that is an answer,
although obscure.
— Wallace Stevens,
"An Ordinary Evening
in New Haven"
From 8/02
in 2005:
Result:
| The Man with the Blue Guitar |
| line 150 (xiii.6): |
The heraldic center of the world |
|
| Human Arrangement |
| line 13: |
The center of transformations that |
|
| This Solitude of Cataracts |
| line 18: |
Breathing his bronzen breath at the azury center of time. |
|
| A Primitive Like an Orb |
| line 1 (i.1): |
The essential poem at the center of things, |
| line 87 (xi.7): |
At the center on the horizon, concentrum, grave |
|
| Reply to Papini |
| line 33 (ii.15): |
And final. This is the center. The poet is |
|
| Study of Images II |
| line 7: |
As if the center of images had its |
|
| An Ordinary Evening in New Haven |
| line 291 (xvii.3): |
It fails. The strength at the center is serious. |
| line 371 (xxi.11): |
At the center, the object of the will, this place, |
|
| Things of August |
| line 154 (ix.18): |
At the center of the unintelligible, |
|
| The Hermitage at the Center |
| Title: |
The Hermitage at the Center |
|
| Owl's Clover, The Old Woman and the Statue (OP) |
| line 13 (ii.9): |
At the center of the mass, the haunches low, |
|
| The Sail of Ulysses (OP) |
| line 50 (iv.6): |
The center of the self, the self |
|
| Someone Puts a Pineapple Together (NA) |
| line 6 (i.6): |
The angel at the center of this rind, |
|
| Of Ideal Time and Choice (NA) |
| line 29: |
At last, the center of resemblance, found |
| line 32: |
Stand at the center of ideal time, |
For a text on today's
mid-day number, see
Theme and Variations.
Comments Off on Monday July 2, 2007
Sunday, July 1, 2007
Mozart
by the Numbers

2/21
A Superficial Beauty:

Structural Certainty:
| |
murphy plant, murphy grow, a maryamyria- |
|
10 |
| |
meliamurphies, in the lazily eye of his lapis, |
|
11 |
| |
|
|
12 |
| |
 |
|
13 |
| |
|
|
14 |
| Uteralterance or |
Vieus Von DVbLIn, ’twas one of dozedeams |
|
15 |
| the Interplay of |
a darkies ding in dewood) the Turnpike under |
|
16 |
| Bones in the |
the Great Ulm (with Mearingstone in Fore |
|
17 |
| Womb. |
ground). 1 Given now ann linch you take enn |
|
18 |
| |
all. Allow me! And, heaving alljawbreakical |
|
19 |
| |
expressions out of old Sare Isaac’s 2 universal |
|
20 |
| The Vortex. |
of specious aristmystic unsaid, A is for Anna |
|
21 |
| Spring of Sprung |
like L is for liv. Aha hahah, Ante Ann you’re |
|
22 |
| Verse. The Ver- |
apt to ape aunty annalive! Dawn gives rise. |
|
23 |
| tex. |
Lo, lo, lives love! Eve takes fall. La, la, laugh |
|
24 |
| |
leaves alass! Aiaiaiai, Antiann, we’re last to |
|
25 |
| |
the lost, Loulou! Tis perfect. Now (lens |
|
26 |
— Finnegans Wake, Book II,
Episode 2, page 293
1/27
“Mozart is a menace to musical progress, a relic of rituals that were losing relevance in his own time and are meaningless to ours. Beyond a superficial beauty and structural certainty, Mozart has nothing to give to mind or spirit in the 21st century. Let him rest.” —Norman Lebrecht
Comments Off on Sunday July 1, 2007
Saturday, June 30, 2007
November 2004–

Controversial
"Desperate Housewives"
ad on "Monday
Night Football"
"Desperate Housewives"… ranks No. 5 among all prime-time shows for ages 12-17. ("Monday Night Football" is No. 18.) This may explain in part why its current advertisers include products like Fisher-Price toys, the DVD of "Elf" and the forthcoming Tim Allen holiday vehicle, "Christmas With the Kranks."
Those who cherish the First Amendment can only hope that the Traditional Values Coalition, OneMillionMoms.com, OneMillionDads.com and all the rest send every e-mail they can to the F.C.C. demanding punitive action against the stations that broadcast "Desperate Housewives." A "moral values" crusade that stands between a TV show this popular and its audience will quickly learn the limits of its power in a country where entertainment is god.
— "The Great Indecency Hoax," a New York Times column by Frank Rich quoted in Log24 on Nov. 26, 2004
|
The entertainment continues. A rabbi's obituary in today's New York Times (see previous entry) served as ad-bait for "Joshua," a Fox Searchlight film opening July 6.
A search for a less sacrilegious memorial to the rabbi yields the following:

The "Project MUSE" link above
works only at
subscribing libraries.
It seems that here, too,
the rabbi is being
used as bait.
For a perhaps preferable
reference to bait, in the
context of St. Peter as
a "fisher of men," see
the Christian "mandorla"
or "vesica piscis,"
a figure hidden within
the geometry of Rome's
St. Peter's Square–
which, despite its name,
is an oval:

For the geometric
construction of the
Roman oval, see
"ovato tondo" in
Rudolf Arnheim's
The Power of the Center.
For a less theoretical account
of the religious significance
of the mandorla, see
the 2001 film
The Center of the World.
Comments Off on Saturday June 30, 2007
Monday, June 25, 2007
Object Lesson
"… the best definition
I have for Satan
is that it is a real
spirit of unreality."
M. Scott Peck,
People of the Lie
"Far in the woods they sang
their unreal songs,
Secure. It was difficult
to sing in face
Of the object. The singers
had to avert themselves
Or else avert the object."
— Wallace Stevens,
"Credences of Summer"
|
Today is June 25,
anniversary of the
birth in 1908 of
Willard Van Orman Quine.
Quine died on
Christmas Day, 2000.
Today, Quine's birthday, is,
as has been noted by
Quine's son, the point of the
calendar opposite Christmas–
i.e., "AntiChristmas."
If the Anti-Christ is,
as M. Scott Peck claims,
a spirit of unreality, it seems
fitting today to invoke
Quine, a student of reality,
and to borrow the title of
Quine's Word and Object…
Word:
An excerpt from
"Credences of Summer"
by Wallace Stevens:
"Three times the concentred
self takes hold, three times
The thrice concentred self,
having possessed
The object, grips it
in savage scrutiny,
Once to make captive,
once to subjugate
Or yield to subjugation,
once to proclaim
The meaning of the capture,
this hard prize,
Fully made, fully apparent,
fully found."
— "Credences of Summer," VII,
by Wallace Stevens, from
Transport to Summer (1947)
|
Object:
From Friedrich Froebel,
who invented kindergarten:

From Christmas 2005:

Click on the images
for further details.
For a larger and
more sophisticaled
relative of this object,
see yesterday's entry
At Midsummer Noon.
The object is real,
not as a particular
physical object, but
in the way that a
mathematical object
is real — as a
pure Platonic form.
"It's all in Plato…."
— C. S. Lewis
Comments Off on Monday June 25, 2007
Sunday, June 24, 2007
Raiders of
the Lost Stone
(Continued from June 23)
Charles Williams:
"In Many Dimensions (1931)
Williams sets before his reader the
mysterious Stone of King Solomon,
an image he probably drew
from a brief description in Waite's
The Holy Kabbalah (1929)
of a supernatural cubic stone
on which was inscribed
Comments Off on Sunday June 24, 2007
Thursday, June 21, 2007

"Ich aber, hier auf dem objektiven Wege, bin jetzt bemüht, das Positive der Sache nachzuweisen, daß nämlich das Ding an sich von der Zeit und Dem, was nur durch sie möglich ist, dem Entstehen und Vergehen, unberührt bleibt, und daß die Erscheinungen in der Zeit sogar jenes rastlos flüchtige, dem Nichts zunächst stehende Dasein nicht haben könnten, wenn nicht in ihnen ein Kern aus der Ewigkeit* wäre. Die Ewigkeit ist freilich ein Begriff, dem keine Anschauung zum Grunde liegt: er ist auch deshalb bloß negativen Inhalts, besagt nämlich ein zeitloses Dasein. Die Zeit ist demnach ein bloßes Bild der Ewigkeit, ho chronos eikôn tou aiônos,** wie es Plotinus*** hat: und ebenso ist unser zeitliches Dasein das bloße Bild unsers Wesens an sich. Dieses muß in der Ewigkeit liegen, eben weil die Zeit nur die Form unsers Erkennens ist: vermöge dieser allein aber erkennen wir unser und aller Dinge Wesen als vergänglich, endlich und der Vernichtung anheimgefallen."
* "a kernel of eternity"
** "Time is the image of eternity."
*** "wie es Plotinus hat"–
Actually, not Plotinus, but Plato,
according to Diogenes Laertius.
Related material:
Time Fold,
J. N. Darby,
"On the Greek Words for
Eternity and Eternal
(aion and aionios),"
Carl Gustav Jung, Aion,
which contains the following
four-diamond figure,

and Jung and the Imago Dei.
Comments Off on Thursday June 21, 2007
"His graceful accounts of the Bach Suites for Unaccompanied Cello illuminated the works’ structural logic as well as their inner spirituality."
—Allan Kozinn on Mstislav Rostropovich in The New York Times, quoted in Log24 on April 29, 2007
"At that instant he saw, in one blaze of light, an image of unutterable conviction…. the core of life, the essential pattern whence all other things proceed, the kernel of eternity."
— Thomas Wolfe, Of Time and the River, quoted in Log24 on June 9, 2005
"… the stabiliser of an octad preserves the affine space structure on its complement, and (from the construction) induces AGL(4,2) on it. (It induces A8 on the octad, the kernel of this action being the translation group of the affine space.)"
— Peter J. Cameron, "The Geometry of the Mathieu Groups" (pdf)
"… donc Dieu existe, réponse!"
"Only gradually did I discover
what the mandala really is:
'Formation, Transformation,
Eternal Mind's eternal recreation'"
(Faust, Part Two, as
quoted by Jung in
Memories, Dreams, Reflections)

"Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen.
The drawing is one of
many by George Gamow
illustrating the script."
— Physics Today
"Borja dropped the mutilated book on the floor with the others. He was looking at the nine engravings and at the circle, checking strange correspondences between them.
'To meet someone' was his enigmatic answer. 'To search for the stone that the Great Architect rejected, the philosopher's stone, the basis of the philosophical work. The stone of power. The devil likes metamorphoses, Corso.'"
— The Club Dumas, basis for the Roman Polanski film "The Ninth Gate" (See 12/24/05.)
"Pauli linked this symbolism
with the concept of automorphism."
— The Innermost Kernel
(previous entry)
And from
"Symmetry in Mathematics
and Mathematics of Symmetry"
(pdf), by Peter J. Cameron,
a paper presented at the
International Symmetry Conference,
Edinburgh, Jan. 14-17, 2007,
we have
The Epigraph–

(Here "whatever" should
of course be "whenever.")
Also from the
Cameron paper:
|
Local or global?
Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts;
• remaining unchanged by transformation.
Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous if every isomorphism between finite substructures of M can be extended to an automorphism of M; in other words, "any local symmetry is global."
|
Some Log24 entries
related to the above politically
(women in mathematics)–
Global and Local:
One Small Step
and mathematically–
Structural Logic continued:
Structure and Logic (4/30/07):
This entry cites
Alice Devillers of Brussels–

"The aim of this thesis
is to classify certain structures
which are, from a certain
point of view, as homogeneous
as possible, that is which have
as many symmetries as possible."
"There is such a thing
as a tesseract."
— Madeleine L'Engle
Comments Off on Thursday June 21, 2007
Wednesday, June 20, 2007
Kernel
Mathematical Reviews citation:
MR2163497 (2006g:81002) 81-03 (81P05)
Gieser, Suzanne The innermost kernel. Depth psychology and quantum physics. Wolfgang Pauli's dialogue with C. G. Jung. Springer-Verlag, Berlin, 2005. xiv+378 pp. ISBN: 3-540-20856-9
A quote from MR at Amazon.com:
"This revised translation of a Swedish Ph. D. thesis in philosophy offers far more than a discussion of Wolfgang Pauli's encounters with the psychoanalyst Carl Gustav Jung…. Here the book explains very well how Pauli attempted to extend his understanding beyond superficial esotericism and spiritism…. To understand Pauli one needs books like this one, which… seems to open a path to a fuller understanding of Pauli, who was seeking to solve a quest even deeper than quantum physics." (Arne Schirrmacher, Mathematical Reviews, Issue 2006g)
An excerpt:
I do not yet know what Gieser means by "the innermost kernel." The following is my version of a "kernel" of sorts– a diagram well-known to students of anthropologist
Claude Levi-Strauss and art theorist
Rosalind Krauss:
The four-group is also known as the Vierergruppe or Klein group. It appears, notably, as the translation subgroup of A, the group of 24 automorphisms of the affine plane over the 2-element field, and therefore as the kernel of the homomorphism taking A to the group of 6 automorphisms of the projective line over the 2-element field. (See Finite Geometry of the Square and Cube.)
Comments Off on Wednesday June 20, 2007
Friday, June 15, 2007
Geometry and Death
(continued from Dec. 11, 2006):
J. G. Ballard on "the architecture of death":
"… a huge system of German fortifications that included the Siegfried line, submarine pens and huge flak towers that threatened the surrounding land like lines of Teutonic knights. Almost all had survived the war and seemed to be waiting for the next one, left behind by a race of warrior scientists obsessed with geometry and death."
— The Guardian, March 20, 2006
From the previous entry, which provided a lesson in geometry related, if only by synchronicity, to the death of Jewish art theorist Rudolf Arnheim:
"We are going to keep doing this until we get it right."
Here is a lesson related, again by synchronicity, to the death of a Christian art scholar of "uncommon erudition, wit, and grace"– Robert R. Wark of the Huntington Library. Wark died on June 8, a date I think of as the feast day of St. Gerard Manley Hopkins, a Jesuit priest-poet of the nineteenth century.
From a Log24 entry on the date of Wark's death–
Samuel Pepys on a musical performance (Diary, Feb. 27, 1668):
"When the Angel comes down"
"When the Angel Comes Down, and the Soul Departs," a webpage on dance in Bali:
"Dance is also a devotion to the Supreme Being."
Julie Taymor, interview:
"I went to Bali to a remote village by a volcanic mountain…."
The above three quotations were intended to supply some background for a link to an entry on Taymor, on what Taymor has called "skewed mirrors," and on a related mathematical concept named, using a term Hopkins coined, "inscapes."
They might form part of an introductory class in mathematics and art given, like the class of the previous entry, in Purgatory.
Wark, who is now, one imagines, in Paradise, needs no such class. He nevertheless might enjoy listening in.
A guest teacher in
the purgatorial class
on mathematics
and art:


"Is it safe?"
Comments Off on Friday June 15, 2007
A Study in
Art Education
Rudolf Arnheim, a student of Gestalt psychology (which, an obituary notes, emphasizes "the perception of forms as organized wholes") was the first Professor of the Psychology of Art at Harvard. He died at 102 on Saturday, June 9, 2007.
The conclusion of yesterday's New York Times obituary of Arnheim:
"… in The New York Times Book Review in 1986, Celia McGee called Professor Arnheim 'the best kind of romantic,' adding, 'His wisdom, his patient explanations and lyrical enthusiasm are those of a teacher.'"
A related quotation:
"And you are teaching them a thing or two about yourself. They are learning that you are the living embodiment of two timeless characterizations of a teacher: 'I say what I mean, and I mean what I say' and 'We are going to keep doing this until we get it right.'"
— Tools for Teaching
Here, yet again, is an illustration that has often appeared in Log24– notably, on the date of Arnheim's death:

Related quotations:
"We have had a gutful of fast art and fast food. What we need more of is slow art: art that holds time as a vase holds water: art that grows out of modes of perception and whose skill and doggedness make you think and feel; art that isn't merely sensational, that doesn't get its message across in 10 seconds, that isn't falsely iconic, that hooks onto something deep-running in our natures. In a word, art that is the very opposite of mass media. For no spiritually authentic art can beat mass media at their own game."
— Robert Hughes, speech of June 2, 2004
"Whether the 3×3 square grid is fast art or slow art, truly or falsely iconic, perhaps depends upon the eye of the beholder."
— Log24, June 5, 2004
If the beholder is Rudolf Arnheim, whom we may now suppose to be viewing the above figure in the afterlife, the 3×3 square is apparently slow art. Consider the following review of his 1982 book The Power of the Center:
"Arnheim deals with the significance of two kinds of visual organization, the concentric arrangement (as exemplified in a bull's-eye target) and the grid (as exemplified in a Cartesian coordinate system)….
It is proposed that the two structures of grid and target are the symbolic vehicles par excellence for two metaphysical/psychological stances. The concentric configuration is the visual/structural equivalent of an egocentric view of the world. The self is the center, and all distances exist in relation to the focal spectator. The concentric arrangement is a hermetic, impregnable pattern suited to conveying the idea of unity and other-worldly completeness. By contrast, the grid structure has no clear center, and suggests an infinite, featureless extension…. Taking these two ideal types of structural scaffold and their symbolic potential (cosmic, egocentric vs. terrestrial, uncentered) as given, Arnheim reveals how their underlying presence organizes works of art."
— Review of Rudolf Arnheim's The Power of the Center: A Study of Composition in the Visual Arts (Univ. of Calif. Press, 1982). Review by David A. Pariser, Studies in Art Education, Vol. 24, No. 3 (1983), pp. 210-213
Arnheim himself says in this book (pp. viii-ix) that "With all its virtues, the framework of verticals and horizontals has one grave defect. It has no center, and therefore it has no way of defining any particular location. Taken by itself, it is an endless expanse in which no one place can be distinguished from the next. This renders it incomplete for any mathematical, scientific, and artistic purpose. For his geometrical analysis, Descartes had to impose a center, the point where a pair of coordinates [sic] crossed. In doing so he borrowed from the other spatial system, the centric and cosmic one."
Students of art theory should, having read the above passages, discuss in what way the 3×3 square embodies both "ideal types of structural scaffold and their symbolic potential."
We may imagine such a discussion in an afterlife art class– in, perhaps, Purgatory rather than Heaven– that now includes Arnheim as well as Ernst Gombrich and Kirk Varnedoe.
Such a class would be one prerequisite for a more advanced course– Finite geometry of the square and cube.
Comments Off on Friday June 15, 2007
Thursday, June 14, 2007
"Kurt Waldheim, the former United Nations Secretary General and President of Austria whose hidden ties to Nazi organizations and war crimes was [sic] exposed late in his career, died today at his home in Vienna. He was 88." —The New York Times this afternoon
Related material:
From a story by
Leonard Michaels
linked to on
Aaron Sorkin's
birthday, June 9:
"Induction and analogy, in which he was highly gifted, were critical to mathematical intelligence.
It has been said that the unexamined life isn't worth living. Nachman wasn't against examining his life, but then what was a life? ….
… As for 'a life,' it was what you read about in newspaper obituaries. He didn't need one. He would return to California and think only about mathematics."

"One two three four,
who are we for?"
Comments Off on Thursday June 14, 2007
Scholarly Notes
In memory of
Rudolf Arnheim,
who died on
Saturday, June 9
“Originally trained in Gestalt psychology, with its emphasis on the perception of forms as organized wholes, he was one of the first investigators to apply its principles to the study of art of all kinds.” —
Today’s New York Times
From the Wikipedia article on Gestalt psychology prior to its modification on May 31, 2007:
“Emergence, reification, multistability, and invariance are not separable modules to be modeled individually, but they are different aspects of a single unified dynamic mechanism.
For a mathematical example of such a mechanism using the cubes of psychologists’ block design tests, see Block Designs in Art and Mathematics and The Kaleidoscope Puzzle.”
The second paragraph of the above passage refers to my own work.
Some Gestalt-related work of Arnheim:

Comments Off on Thursday June 14, 2007
Wednesday, June 6, 2007
If Cullinane College were Hogwarts–
Last-minute exam info:
The Lapis Philosophorum
"The lapis was thought of as a unity and therefore often stands for the prima materia in general."
— Aion, by C. G. Jung
"Its discoverer was of the opinion that he had produced the equivalent of the primordial protomatter which exploded into the Universe."
— The Stars My Destination, by Alfred Bester
And from Bester's The Deceivers:
Meta Physics
"'… Think of a match. You've got a chemical head of potash, antimony, and stuff, full of energy waiting to be released. Friction does it. But when Meta excites and releases energy, it's like a stick of dynamite compared to a match. It's the chess legend for real.'
'I don't know it.'
'Oh, the story goes that a philosopher invented chess for the amusement of an Indian rajah. The king was so delighted that he told the inventor to name his reward and he'd get it, no matter what. The philosopher asked that one grain of rice be placed on the first square of the chessboard, two on the second, four on the third, and so on to the sixty-fourth.'
'That doesn't sound like much.'
'So the rajah said. …'"
Related material:
Geometry of the I Ching
Comments Off on Wednesday June 6, 2007
Sunday, June 3, 2007
Haunting Time
"Macquarrie remains one of the most
important commentators [on] …
Heidegger's work. His co-translation
of Being and Time into English is
considered the canonical version."
— Wikipedia

The Rev. Macquarrie died on
May 28. The Log24 entry
for that date contains the
following illustration:

The part of the illustration
relevant to the death of
Macquarrie is the color.
From my reply to
a comment on the
May 28 entry:
"I checked out [Terence] McKenna and found this site on the aging druggie. I didn't like the hippie scene in the sixties and I don't like it now. Booze was always my drug of choice. Still, checking further, I found that McKenna's afterword to Dick's In Pursuit of Valis was well written."
From McKenna's afterword:
"Schizophrenia is not a psychological disorder peculiar to human beings. Schizophrenia is not a disease at all but rather a localized traveling discontinuity of the space time matrix itself. It is like a travelling whirl-wind of radical understanding that haunts time. It haunts time in the same way that Alfred North Whitehead said that the color dove grey 'haunts time like a ghost.'"
I can find no source for
any remarks of Whitehead
on the color "dove grey"
(or "gray") but Whitehead
did say that
"A colour is eternal. It haunts time like a spirit. It comes and it goes. But where it comes it is the same colour. It neither survives nor does it live. It appears when it is wanted." —Science and the Modern World, 1925
The poetic remark of
McKenna on the color
"dove grey" may be
taken, in a schizophrenic
(or, similarly, a Christian) way,
as a reference to the Holy Spirit.
My own remarks on the hippie
scene seem appropriate as a
response to media celebration
of today's 40th anniversary of
the beginning of the 1967
"summer of love."
Comments Off on Sunday June 3, 2007
Saturday, June 2, 2007
“I don’t think the ‘diamond theorem’ is anything serious, so I started with blitzing that.”
— Charles Matthews at Wikipedia, Oct. 2, 2006
“The ‘seriousness’ of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is ‘significant’ if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas.”
— G. H. Hardy, A Mathematician’s Apology
Comments Off on Saturday June 2, 2007
Thursday, May 31, 2007
Blitz by anonymous
New Delhi user
From Wikipedia on 31 May, 2007:
Shown below is a list of 25 alterations to Wikipedia math articles made today by user 122.163.102.246.
All of the alterations involve removal of links placed by user Cullinane (myself).
The 122.163… IP address is from an internet service provider in New Delhi, India.
The New Delhi anonymous user was apparently inspired by an earlier blitz by Wikipedia administrator Charles Matthews. (See User talk: Cullinane.)
Related material:
Ashay Dharwadker and Usenet Postings
and Talk: Four color theorem/Archive 2.
See also some recent comments from 122.163…
at Talk: Four color theorem.
May 31, 2007, alterations by
user 122.163.102.246:
- 17:17 Orthogonality (rm spam)
- 17:16 Symmetry group (rm spam)
- 17:14 Boolean algebra (rm spam)
- 17:12 Permutation (rm spam)
- 17:10 Boolean logic (rm spam)
- 17:08 Gestalt psychology (rm spam)
- 17:05 Tesseract (rm spam)
- 17:02 Square (geometry) (rm spam)
- 17:00 Fano plane (rm spam)
- 16:55 Binary Golay code (rm spam)
- 16:53 Finite group (rm spam)
- 16:52 Quaternion group (rm spam)
- 16:50 Logical connective (rm spam)
- 16:48 Mathieu group (rm spam)
- 16:45 Tutte–Coxeter graph (rm spam)
- 16:42 Steiner system (rm spam)
- 16:40 Kaleidoscope (rm spam)
- 16:38 Efforts to Create A Glass Bead Game (rm spam)
- 16:36 Block design (rm spam)
- 16:35 Walsh function (rm spam)
- 16:24 Latin square (rm spam)
- 16:21 Finite geometry (rm spam)
- 16:17 PSL(2,7) (rm spam)
- 16:14 Translation plane (rm spam)
- 16:13 Block design test (rm spam)
The deletions should please Charles Matthews and fans of Ashay Dharwadker’s work as a four-color theorem enthusiast and as editor of the Open Directory sections on combinatorics and on graph theory.
There seems little point in protesting the deletions while Wikipedia still allows any anonymous user to change their articles.
— Cullinane 23:28, 31 May 2007 (UTC)
Comments Off on Thursday May 31, 2007
Monday, May 28, 2007
and a Finite Model
Notes by Steven H. Cullinane
May 28, 2007
Part I: A Model of Space-Time
The following paper includes a figure illustrating
Penrose’s model of “complexified, compactified Minkowski space-time as the Klein quadric in complex projective 5-space.”
Click on picture to enlarge.
For some background on the Klein quadric and space-time, see Roger Penrose, “
On the Origins of Twistor Theory,” from
Gravitation and Geometry:
A Volume in Honor of Ivor Robinson, Bibliopolis, 1987.
Part II: A Corresponding Finite Model
The Klein quadric also occurs in a finite model of projective 5-space. See a 1910 paper:
G. M. Conwell, The 3-space PG(3,2) and its group, Ann. of Math. 11, 60-76.
Conwell discusses the quadric, and the related Klein correspondence, in detail. This is noted in a more recent paper by Philippe Cara:
As Cara goes on to explain, the Klein correspondence underlies Conwell’s discussion of eight
heptads. These play an important role in another correspondence, illustrated in the
Miracle Octad Generator of R. T. Curtis, that may be used to picture actions of the large Mathieu group M
24.
Related material:
The projective space PG(5,2), home of the Klein quadric in the finite model, may be viewed as the set of 64 points of the affine space AG(6,2), minus the origin.
The 64 points of this affine space may in turn be viewed as the 64 hexagrams of the Classic of Transformation, China’s I Ching.
There is a natural correspondence between the 64 hexagrams and the 64 subcubes of a 4x4x4 cube. This correspondence leads to a natural way to generate the affine group AGL(6,2). This may in turn be viewed as a group of over a trillion natural transformations of the 64 hexagrams.
“Once Knecht confessed to his teacher that he wished to learn enough to be able to incorporate the system of the I Ching into the Glass Bead Game. Elder Brother laughed. ‘Go ahead and try,’ he exclaimed. ‘You’ll see how it turns out. Anyone can create a pretty little bamboo garden in the world. But I doubt that the gardener would succeed in incorporating the world in his bamboo grove.'”
translated by Richard and Clara Winston
Friday, May 25, 2007


Square Dance:

This "telling of what
I know" will of course
mean little to those
who, like Emerson,
have refused to learn
through quotations.
For those less obdurate
than Emerson —Harold Bloom
on Wallace Stevens
and Paul Valery's
"Dance and the Soul"–
"Stevens may be playful, yet seriously so, in describing desire, at winter's end, observing not only the emergence of the blue woman of early spring, but seeing also the myosotis, whose other name is 'forget-me-not.' Desire, hearing the calendar hymn, repudiates the negativity of the mind of winter, unable to bear what Valery's Eryximachus had called 'this cold, exact, reasonable, and moderate consideration of human life as it is.' The final form of this realization in Stevens comes in 1950, in The Course of a Particular, in the great monosyllabic line 'One feels the life of that which gives life as it is.' But even Stevens cannot bear that feeling for long. As Eryximachus goes on to say in Dance and the Soul:
A cold and perfect clarity is a poison impossible to combat. The real, in its pure state, stops the heart instantaneously….[…] To a handful of ashes is the past reduced, and the future to a tiny icicle. The soul appears to itself as an empty and measurable form. –Here, then, things as they are come together, limit one another, and are thus chained together in the most rigorous and mortal* fashion…. O Socrates, the universe cannot for one instant endure to be only what it is.
Valery's formula for reimagining the First Idea is, 'The idea introduces into what is, the leaven of what is not.' This 'murderous lucidity' can be cured only by what Valery's Socrates calls 'the intoxication due to act,' particularly Nietzschean or Dionysiac dance, for this will rescue us from the state of the Snow Man, 'the motionless and lucid observer.'" —Wallace Stevens: The Poems of Our Climate
* "la sorte… la plus mortelle":
mortal in the sense
"deadly, lethal"
Other quotations
(from March 28,
the birthday of
Reba McEntire):
Logical Songs

Logical Song I
(Supertramp)
"When I was young, it seemed that
Life was so wonderful, a miracle,
Oh it was beautiful, magical
And all the birds in the trees,
Well they'd be singing so happily,
Joyfully, playfully watching me"
Logical Song II
(Sinatra)
"You make me feel so young,
You make me feel like
Spring has sprung
And every time I see you grin
I'm such a happy in-
dividual….
You and I are
Just like a couple of tots
Running across the meadow
Picking up lots
Of forget-me-nots"
Comments Off on Friday May 25, 2007
Thursday, May 24, 2007
Comments Off on Thursday May 24, 2007
Wednesday, May 23, 2007
Strong Emergence Illustrated:
The Beauty Test
"There is no royal road
to geometry"
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):

The illustration (and definition) is
of
regular tessellations of the plane.
This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:

One might argue that such beauty is
strongly emergent because of the "harmonious way" the parts fit together: the regularity (or fitting together) of the whole is not reducible to the regularity of the parts. (Regular triangles, squares, and hexagons fit together, but regular pentagons do not.)
The symmetries of these regular tessellations of the plane are less well suited as illustrations of emergence, since they are tied rather closely to symmetries of the component parts.
But the symmetries of regular tessellations of the
sphere— i.e., of the five Platonic solids–
do emerge strongly, being apparently independent of symmetries of the component parts.
Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
Comments Off on Wednesday May 23, 2007
Tuesday, May 22, 2007
Jewel in the Crown

The Crown of Geometry
(according to Logothetti
in a 1980 article)
The crown jewels are the
Platonic solids, with the
icosahedron at the top.
Related material:
illustrates ways of partitioning the 12 vertices of an icosahedron into 3 sets of 4, so that each set forms the corners of a rectangle in the Golden Ratio. Each such rectangle is known as a duad. The short sides of a duad are opposite edges of the icosahedron, and there are 30 edges, so there are 15 duads.
Each partition of the vertices into duads is known as a syntheme. There are 15 synthemes; 5 consist of duads that are mutually perpendicular, while the other 10 consist of duads that share a common line of intersection."
— Greg Egan, Syntheme
Comments Off on Tuesday May 22, 2007
Monday, May 21, 2007
No Royal Roads
A more recent royal reference:
"'Yau wants to be the king of geometry,' Michael Anderson, a geometer at Stony Brook, said. 'He believes that everything should issue from him, that he should have oversight. He doesn't like people encroaching on his territory.'" –Sylvia Nasar and David Gruber in The New Yorker, issue dated Aug. 28, 2006
Wikipedia, Cultural references to the Royal Road:
"Euclid is said to have replied to King Ptolemy's request for an easier way of learning mathematics that 'there is no royal road to geometry.' Charles S. Peirce, in his 'How to Make Our Ideas Clear' (1878), says 'There is no royal road to logic, and really valuable ideas can only be had at the price of close attention.'"
Comments Off on Monday May 21, 2007
Sunday, May 20, 2007
Plato and Shakespeare:
Solid and Central
"I have another far more solid and central ground for submitting to it as a faith, instead of merely picking up hints from it as a scheme. And that is this: that the Christian Church in its practical relation to my soul is a living teacher, not a dead one. It not only certainly taught me yesterday, but will almost certainly teach me to-morrow. Once I saw suddenly the meaning of the shape of the cross; some day I may see suddenly the meaning of the shape of the mitre. One free morning I saw why windows were pointed; some fine morning I may see why priests were shaven. Plato has told you a truth; but Plato is dead. Shakespeare has startled you with an image; but Shakespeare will not startle you with any more. But imagine what it would be to live with such men still living, to know that Plato might break out with an original lecture to-morrow, or that at any moment Shakespeare might shatter everything with a single song. The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast. He is always expecting to see some truth that he has never seen before."
— G. K. Chesterton, Orthodoxy, Ch. IX
From Plato, Pegasus, and the Evening Star (11/11/99):
|
"Nonbeing must in some sense be, otherwise what is it that there is not? This tangled doctrine might be nicknamed Plato's beard; historically it has proved tough, frequently dulling the edge of Occam's razor…. I have dwelt at length on the inconvenience of putting up with it. It is time to think about taking steps."
— Willard Van Orman Quine, 1948, "On What There Is," reprinted in From a Logical Point of View, Harvard University Press, 1980
"The Consul could feel his glance at Hugh becoming a cold look of hatred. Keeping his eyes fixed gimlet-like upon him he saw him as he had appeared that morning, smiling, the razor edge keen in sunlight. But now he was advancing as if to decapitate him."
— Malcolm Lowry, Under the Volcano, 1947, Ch. 10
|
"O God, I could be
bounded in a nutshell
and count myself
a king of infinite space,
were it not that
I have bad dreams."
— Hamlet


From today's newspaper:
Comments Off on Sunday May 20, 2007
Thursday, May 3, 2007
A Web
of Links
"Some postmodern theorists like to talk about the relationship between 'intertextuality' and 'hypertextuality'; intertextuality makes each text a 'mosaic of quotations' [Kristeva, Desire in Language, Columbia U. Pr., 1980, 66] and part of a larger mosaic of texts, just as each hypertext can be a web of links and part of the whole World-Wide Web." —Wikipedia
Comments Off on Thursday May 3, 2007
Monday, April 30, 2007
Structure and Logic
The phrase “structural logic” in yesterday’s entry was applied to Bach’s cello suites. It may equally well be applied to geometry. In particular:
“The aim of this thesis is to classify certain structures which are, from a certain point of view, as homogeneous as possible, that is which have as many symmetries as possible.”
— Alice Devillers, “Classification of Some Homogeneous and Ultrahomogeneous Structures,” Ph.D. thesis, Université Libre de Bruxelles, academic year 2001-2002
Related material:

The above models for the corresponding projective spaces may be regarded as illustrating the phrase “structural logic.”
For a possible application of the 16-point space’s “many symmetries” to logic proper, see The Geometry of Logic.
Comments Off on Monday April 30, 2007
Saturday, April 28, 2007
Cubism

See last year’s
entries for 5/10 —
My Space

and for 2/23 —
Cubist Epiphany

“This is a crazy world and
the only way to enjoy it
is to treat it as a joke.”
— Robert A. Heinlein,
The Number of the Beast
Comments Off on Saturday April 28, 2007
Sunday, April 22, 2007
Built
continued from
March 25, 2006
In honor of Scarlett Johansson's recent London films "Match Point" and "Scoop," here is a link to an entry of Women's History Month, 2006, with a discussion of an exhibition of the works of artist Liza Lou at London's White Cube Gallery. That entry includes the following illustrations:
Comments Off on Sunday April 22, 2007
Saturday, April 7, 2007
Comments Off on Saturday April 7, 2007
Tuesday, April 3, 2007
Our Judeo-Christian
Heritage –
Lottery
Hermeneutics
Part II: Christian
Part III:
Imago Dei
Click on picture
for details.
Related material:
It is perhaps relevant to
this Holy Week that the
date 6/04 (2006) above
refers to both the Christian
holy day of Pentecost and
to the day of the
facetious baccalaureate
of the Class of 2006 in
the University Chapel
at Princeton.
For further context for the
Log24 remarks of that same
date, see June 1-15, 2006.
Comments Off on Tuesday April 3, 2007
Saturday, March 24, 2007
Savage Scrutiny
“They sang desiring an object that was near,
In face of which desire no longer moved,
Nor made of itself that which it could not find…
Three times the concentred self takes hold, three times
The thrice concentred self, having possessed
The object, grips it in savage scrutiny,
Once to make captive, once to subjugate
Or yield to subjugation, once to proclaim
The meaning of the capture, this hard prize,
Fully made, fully apparent, fully found.”
— “Credences of Summer,” VII,
by Wallace Stevens, from
Transport to Summer (1947)
Clifford Geertz on Levi-Strauss, from The Cerebral Savage:
“Savage logic works like a kaleidoscope whose chips can fall into a variety of patterns…. “
Related material:
The kaleidoscope puzzle and “Claude Levi-Strauss and the Aesthetic Object,” a videotaped interview with Dr. Boris Wiseman.
Comments Off on Saturday March 24, 2007
Wednesday, March 21, 2007
Art Appreciation
A rectangle in memory of
Harvard mathematician
George Mackey:
The five Log24 entries ending at
7:00 PM on March 14, 2006,
the last day of Mackey's life:
A rectangle in memory of
artist Mark Rothko:

Sotheby's
Rothko Painting
Is Up for Auction
By CAROL VOGEL of
THE NEW YORK TIMES,
March 21, 5:35 PM ET
"David Rockefeller plans to sell
a seminal painting by Mark Rothko
for what Sotheby's hopes will be
more than $40 million. Above,
a detail from the painting."
From the story:
"Mr. Rockefeller has owned the
painting since 1960, when he
bought it for less than $10,000….
He said that in November, during a
periodic appraisal of his art collection,
he noticed to his surprise that of all
his paintings, the Rothko had
appreciated in value the most.
'That got me thinking,' he said."
Art appreciation:
When Crayolas worked, I dreamed an angel,
a bar of light, your messenger,
beckoning from a wallpaper corner,
blushing in the porcelain gas glow.
When Crayolas worked and chariots swung low,
and America was beautiful and time was slow.
Then all that died in life's longer year.
Autumn came, colors turned sere.
Brittle Crayolas crumbled when touched.
The friends of life were cold and hushed.
Still you were there, shining and warm
behind snow clouds, safe from our harm.
The seed I am again burst out,
drank your heat, suckled your light
in another fair spring to live again
on billowing oceans of bottomless green.
— Excerpt from C. K. Latham's
When Crayolas Worked,
from Shiva Dancing:
The Rothko Chapel Songs,
1972-1997
|
Finite Relativity
continued
This afternoon I added a paragraph to
The Geometry of Logic that makes it, in a way, a sequel to the webpage
Finite Relativity:
"As noted previously, in Figure 2 viewed as a lattice the 16 digital labels 0000, 0001, etc., may be interpreted as naming the 16 subsets of a 4-set; in this case the partial ordering in the lattice is the structure preserved by the lattice's group of 24 automorphisms– the same automorphism group as that of the 16 Boolean connectives. If, however, these 16 digital labels are interpreted as naming the 16 functions from a 4-set to a 2-set (of two truth values, of two colors, of two finite-field elements, and so forth), it is not obvious that the notion of partial order is relevant. For such a set of 16 functions, the relevant group of automorphisms may be the affine group of A mentioned above. One might argue that each Venn diagram in Fig. 3 constitutes such a function– specifically, a mapping of four nonoverlapping regions within a rectangle to a set of two colors– and that the diagrams, considered simply as a set of two-color mappings, have an automorphism group of order larger than 24… in fact, of order 322,560. Whether such a group can be regarded as forming part of a 'geometry of logic' is open to debate."
The epigraph to "Finite Relativity" is:
"This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them."
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
The added paragraph seems to fit this description.
Comments Off on Wednesday March 21, 2007
Sunday, March 18, 2007
Update to
The Geometry of Logic:
A detailed description of a group of 16 “logical automorphisms” of the 16 binary connectives has been given in the paper “Simetria y Logica: La notacion de Peirce para los 16 conectivos binarios,” by Mireya Garcia, Jhon Fredy Gomez, and Arnold Oostra. (Published in the Memorias del XII Encuentro de Geometria y sus Aplicaciones, Universidad Pedagogica Nacional, Bogota, June 2001; on the Web at http://www.unav.es/gep/Articulos/SimetriaYLogica.pdf.) The authors do not identify this group as a subgroup of the affine group of A (the finite affine geometry of four dimensions over the two-element field); this can serve as an exercise. Another exercise: determining whether the authors’ order-16 group includes all transformations that might reasonably be called “logical automorphisms” of the 16 binary connectives.
Comments Off on Sunday March 18, 2007
« Newer Posts —
Older Posts »




































 (Photo from
(Photo from