Wednesday, July 22, 2009
Alphabet vs. Goddess
Continued…
… from June 11, 2008.
"Just as both tragedy and comedy can be written by using the same letters of the alphabet, the vast variety of events in this world can be realized by the same atoms through their different arrangements and movements. Geometry and kinematics, which were made possible by the void, proved to be still more important in some way than pure being."
Werner, Kimberly;
Kimberly, Werner.
Happy Feast of
St. Mary Magdalene.
Comments Off on Wednesday July 22, 2009
Tuesday, July 14, 2009
For Galois on Bastille Day Elements
of Finite Geometry
Some fans of the alchemy in
Katherine Neville’s novel
The Eight and in Dan Brown’s
novel Angels & Demons may
enjoy the following analogy–
Note that the alchemical structure
at left, suited more to narrative
than to mathematics, nevertheless
is mirrored within the pure
mathematics at right.
Related material
on Galois and geometry:
Geometries of the group PSL(2, 11)
by Francis Buekenhout, Philippe Cara, and Koen Vanmeerbeek. Geom. Dedicata, 83 (1-3): 169–206, 2000–
|
Comments Off on Tuesday July 14, 2009
Monday, July 13, 2009
Comments Off on Monday July 13, 2009
Saturday, July 11, 2009
Comments Off on Saturday July 11, 2009
Wednesday, July 8, 2009
Comments Off on Wednesday July 8, 2009
Monday, June 29, 2009
Calvinist Epiphany
for St. Peter's Day
"Have your people
call my people."
— George Carlin
Diamond life, lover boy;
we move in space
with minimum waste
and maximum joy.
— Sade, quoted here on
Lincoln's Birthday, 2003
This is perhaps suitable
for the soundtrack of
the film "Blockheads"
(currently in development)–
Diamond Life —
Related material from Wikipedia:
"Uta Frith, in her book Autism: Explaining the Enigma,[5] addresses the superior performance of autistic individuals on the block design [link not in Wikipedia] test. This was also addressed in [an] earlier paper.[6] A particularly interesting article demonstrates the differences in construction time in the performance of the block design task by Asperger syndrome individuals and non-Asperger's individuals. An essential point here is that in an unsegmented version of the task, Asperger's individuals performed dramatically faster than non-Asperger's individuals: [7]."
5. Frith, Uta (2003). Autism: explaining the enigma (2nd ed. ). Cambridge, MA: Blackwell Pub. ISBN 0-631-22901-9.
6. Shah A, Frith U (Nov 1993). "Why do autistic individuals show superior performance on the block design task?". J Child Psychol Psychiatry 34 (8): 1351–64. PMID 8294523.
7. Caron MJ, Mottron L, Berthiaume C, Dawson M (Jul 2006). "Cognitive mechanisms, specificity and neural underpinnings of visuospatial peaks in autism". Brain 129 (Pt 7): 1789–802. doi:10.1093/brain/awl072. PMID 16597652. "Fig 3".
Comments Off on Monday June 29, 2009
Thursday, June 25, 2009
“… T. S. Eliot tried to recompose,
in
Four Quartets, the fragments
he had grieved over
in
The Waste Land.”
— “Beauty and Desecration,”
Roger Scruton
(link at aldaily.com today)
| “The formula reproduces exactly the essential features of the symbolic process of transformation. It shows the rotation of the mandala, the antithetical play of complementary (or compensatory) processes, then the apocatastasis, i.e., the restoration of an original state of wholeness….”
— Carl G. Jung in Aion
|
Related material:
Comments Off on Thursday June 25, 2009
Monday, June 22, 2009
Text
Today’s birthday:
Kris Kristofferson
Online Etymology Dictionary
-
-
1369, “wording of anything written,” from O.Fr. texte, O.N.Fr. tixte (12c.), from M.L. textus “the Scriptures, text, treatise,” in L.L. “written account, content, characters used in a document,” from L. textus “style or texture of a work,” lit. “thing woven,” from pp. stem of texere “to weave,” from PIE base *tek- “make” (see texture).
“An ancient metaphor: thought is a thread, and the raconteur is a spinner of yarns– but the true storyteller, the poet, is a weaver. The scribes made this old and audible abstraction into a new and visible fact. After long practice, their work took on such an even, flexible texture that they called the written page a textus, which means cloth.” [Robert Bringhurst, “The Elements of Typographic Style”]
Text-book is from 1779.
|
“Discuss the geometry
underlying the above picture.”
— Log24, June 11, 2009
Comments Off on Monday June 22, 2009
Thursday, June 11, 2009
Geometry for Jews
(continued from Michelangelo’s birthday, 2003)
“Discuss the geometry underlying the above picture.”
— Log24, March 6, 2003
Abstraction and the Holocaust (Mark Godfrey, Yale University Press, 2007) describes one approach to such a discussion: Bochner “took a photograph of a new arrangement of blocks, cut it up, reprinted it as a negative, and arranged the four corners in every possible configuration using the serial principles of rotation and reversal to make Sixteen Isomorphs (Negative) of 1967, which he later illustrated alongside works by Donald Judd, Sol LeWitt and Eva Hesse in his Artforum article ‘The Serial Attitude.’ [December 1967, pp. 28-33]” Bochner’s picture of “every possible configuration”–
Compare with the 24 figures in Frame Tales
(Log24, Nov. 10, 2008) and in Theme and Variations.
Comments Off on Thursday June 11, 2009
Thursday, June 4, 2009
New collection release:
Pattern in Islamic Art
from David Wade
David Wade has partnered with ARTstor to distribute approximately 1,500 images of Islamic art, now available in the Digital Library. These images illustrate patterns and designs found throughout the Islamic world, from the Middle East and Europe to Central and South Asia. They depict works Wade photographed during his travels, as well as drawings and diagrams produced for publication. Reflective of Wade's particular interest in symmetry and geometry, these images analyze and break down common patterns into their basic elements, thereby revealing the underlying principles of order and balance in Islamic art. Islamic artists and craftsmen employed these intricate patterns to adorn all types of surfaces, such as stone, brick, plaster, ceramic, glass, metal, wood, and textiles. The collection contains examples of ornamentation from monumental architecture to the decorative arts.
To view the David Wade: Pattern in Islamic Art collection: go to the ARTstor Digital Library, browse by collection, and click "David Wade: Pattern in Islamic Art;" or enter the Keyword Search: patterninislamicart.
For more detailed information about this collection, visit the David Wade: Pattern in Islamic Art collection page.
|
The above prose illustrates
the institutional mind at work.
Those who actually try to view
the Wade collection will
encounter the following warning:
| To access the images in the ARTstor Digital Library you need to be affiliated with a participating institution (university, college, museum, public library or K-12 school). |
Comments Off on Thursday June 4, 2009
Wednesday, June 3, 2009
Epigraphs
to Four Quartets:
The Dissertations of Maximus Tyrius, translated from the Greek by Thomas Taylor, printed by C. Whittingham, London, for the translator, 1804, Vol. II, p. 55:
"You see the mutation of bodies, and the transition of generation, a path upwards and downwards according to Heraclitus; and again, as he says, one thing living the death, but dying the life of another. Thus fire lives the death of earth, and air lives the death of fire; water lives the death of air, and earth lives the death of water. You see a succession of life, and a mutation of bodies, both of which are the renovation of the whole."
For an interpretation
of the above figure
in terms of the classical
four elements discussed
in Four Quartets,
in Dissertations, and
in Angels & Demons,
see
Notes on Mathematics
and Narrative.
For a more entertaining
interpretation, see Fritz Leiber's
classic story "Damnation Morning."
Comments Off on Wednesday June 3, 2009
Thursday, May 28, 2009
Spelling
At right below, an image from the opening of Fox Studios Australia in Sydney on November 7, 1999. The Fox ceremonies included, notably, Kylie Minogue singing “Diamonds are a Girl’s Best Friend.”
|
Red Windmill
|
Kylie Minogue
|
For the mathematical properties of the red windmill (moulin rouge) figure at left, see Diamond Theory.
|
“There comes a time when you have
learned enough to decide whether
the way of the Craft is for you….
First you will need to
prepare your sacred space….
Calling the Corners (or Quarters)
is something you will always do.”
Happy birthday, Kylie.
Comments Off on Thursday May 28, 2009
Friday, May 22, 2009
Steiner System
New York Times
banner this morning:
Click to enlarge.
Related material from
July 11, 2008:
|
The HSBC Logo Designer —
Henry Steiner
 He is an internationally recognized corporate identity consultant. Based in Hong Kong, his work for clients such as HongkongBank, IBM and Unilever is a major influence in Pacific Rim design.
Born in Austria and raised in New York, Steiner was educated at Yale under Paul Rand and attended the Sorbonne as a Fulbright Fellow. He is a past President of Alliance Graphique Internationale. Other professional affiliations include the American Institute of Graphic Arts, Chartered Society of Designers, Design Austria, and the New York Art Directors' Club.
His Cross-Cultural Design: Communicating in the Global Marketplace was published by Thames and Hudson (1995).
— Yaneff.com
|
Charles Taylor,
"Epiphanies of Modernism,"
Chapter 24 of Sources of the Self
(Cambridge U. Press, 1989, p. 477):
"… the object sets up
a kind of frame or space or field
within which there can be epiphany."
|
Related material suggested by
an ad last night on
ABC's Ugly Betty season finale:
Diamond from last night's
Log24 entry, with
four colored pencils from
Diane Robertson Design:
See also
A Four-Color Theorem.
Comments Off on Friday May 22, 2009
Wednesday, May 20, 2009
From Quilt Blocks to the
Mathieu Group M24
Click on illustrations for details.
The connection:
The relationship of S(5,8,24) to the finite geometry PG(3,2) has also been discussed in–
- "A Geometric Construction of the Steiner System S(4,7,23)," by Alphonse Baartmans, Walter Wallis, and Joseph Yucas, Discrete Mathematics 102 (1992) 177-186.
Abstract: "The Steiner system S(4,7,23) is constructed from the geometry of PG(3,2)."
- "A Geometric Construction of the Steiner System S(5,8,24)," by R. Mandrell and J. Yucas, Journal of Statistical Planning and Inference 56 (1996), 223-228.
Abstract: "The Steiner system S(5,8,24) is constructed from the geometry of PG(3,2)."
Comments Off on Wednesday May 20, 2009
Tuesday, May 19, 2009
Exquisite Geometries
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," 1995, by Andries E. Brouwer
"The Steiner system S(5, 8, 24) is a set S of 759 eight-element subsets ('octads') of a twenty-four-element set T such that any five-element subset of T is contained in exactly one of the 759 octads. Its automorphism group is the large Mathieu group M24."
— The Miracle Octad Generator (MOG) of R.T. Curtis (webpage)
"… in 1861 Mathieu… discovered five multiply transitive permutation groups…. In a little-known 1931 paper of Carmichael… they were first observed to be automorphism groups of exquisite finite geometries."
— William M. Kantor, 1981
The 1931 paper of Carmichael is now available online from the publisher for $10.
Comments Off on Tuesday May 19, 2009
Sunday, May 17, 2009
Comments Off on Sunday May 17, 2009
Design Theory
"My work is motivated by a hope that there may be a way to recapture the ancient and medieval vision of both Beauty and purpose in a way which is relevant to our own century. I even dare to hope that the two ideas may be related, that Beauty is actually part of the meaning and purpose of life."
Hans Ludwig de Vries, "
On Orthogonal Resolutions of the Classical Steiner Quadruple System SQS(16),"
Designs, Codes and Cryptography Vol. 48, No. 3 (Sept. 2008) 287-292 (DOI 10.1007/s10623-008-9207-5)–
"The Reverend T. P. Kirkman knew in 1862 that there exists a group of degree 16 and order 322560 with a normal, elementary abelian, subgroup of order 16 [1, p. 108]. Frobenius identified this group in 1904 as a subgroup of the Mathieu group M24 [4, p. 570]…."
1. Biggs N.L., "T. P. Kirkman, Mathematician," Bulletin of the London Mathematical Society 13, 97–120 (1981).
4. Frobenius G., "Über die Charaktere der mehrfach transitiven Gruppen," Sitzungsber. Königl. Preuss. Akad. Wiss. zu Berlin, 558–571 (1904). Reprinted in Frobenius, Gesammelte Abhandlungen III (J.-P. Serre, editor), pp. 335–348. Springer, Berlin (1968).
Olli Pottonen, "Classification of Steiner Quadruple Systems" (Master's thesis, Helsinki, 2005)–
"The concept of group actions is very useful in the study of isomorphisms of combinatorial structures."
"Simplify, simplify."
— Thoreau
"Beauty is bound up
with symmetry."
— Weyl
Pottonen's thesis is
dated Nov. 16, 2005.
For some remarks on
images and theology,
see Log24 on that date.
Click on the above image
for some further details.
Comments Off on Sunday May 17, 2009
Sunday, May 10, 2009
Mother’s Day
at MAA
Rick’s Tricky Six Puzzle:
S5 Sits Specially in S6
by Alex Fink and Richard Guy
Abstract. Rick Wilson identified a sliding block puzzle, the Tricky Six puzzle, in which a uniquely small fraction of the possible scrambled arrangements of the six moving pieces can be restored to the solved state. The permutations one can perform form the abstract group S5, the symmetric group on five letters, but surprisingly they aren’t any of the “obvious” copies of S5 in S6 that fix a single point and allow the other five to be permuted arbitrarily. This special S5 comes from the outer automorphism of S6, a remarkable group-theoretic map whose presence is felt in several combinatorial objects. We track down this outer automorphism in the Tricky Six puzzle as well as the projective plane of order 4, the Hoffman-Singleton graph, the Steiner system S(5,6,12), and a couple of error-correcting codes.
Meanwhile:
Click to enlarge.
Background:
A pair of matronly women
gave readings of
bad mathematical poetry
on April 28 at
the MAA’s Carriage House
Conference Center in
Washington, D.C.
Wednesday, May 6, 2009
Joke
“My pursuits are a joke
in that the universe is a joke.
One has to reflect
the universe faithfully.”
— John Frederick Michell
Feb. 9, 1933 –
April 24, 2009
“I laugh because I dare not cry.
This is a crazy world and
the only way to enjoy it
is to treat it as a joke.”
— Robert A. Heinlein,
The Number of the Beast
For Marisa Tomei
(born Dec. 4, 1964) —
on the day that
Bob Seger turns 64 —
A Joke:
Points All Her Own
Points All Her Own,
Part I:
(For the backstory, see
the Log24 entries and links
on Marisa Tomei’s birthday
last year.)
Points All Her Own,
Part II:
(For the backstory, see
Galois Geometry:
The Simplest Examples.)
Points All Her Own,
Part III:
(For the backstory, see
Geometry of the I Ching
and the history of
Chinese philosophy.)
In simpler terms:
Smackdown!
Comments Off on Wednesday May 6, 2009
Sunday, May 3, 2009
Michell, who wrote on Glastonbury
(a site associated with King Arthur)
and on sacred geometry, seems to
have had a better education than
most sacred-geometry enthusiasts.
He is said to have studied at
Eton and at Trinity College,
Cambridge.
He is not to be
confused with an earlier
Trinity figure, mathematician
John Henry Michell,
who died at 76 on the third
day of February in 1940.
Related material:
See the Log24 entry
from the date of death
of the later Michell —
April 24 —
and, in light of the later
Michell’s interest in
geometry and King Arthur,
the Log24 remarks for
Easter Sunday this year
(April 12).
These remarks include the
following figure by
Sebastian Egner related,
if only through myth,
to Arthur’s round table —
— and the classic Delmore Schwartz
poem “Starlight Like Intuition
Pierced the Twelve.”
Which of the two John Michells
(each a Merlin figure of sorts)
would be more welcome in
Camelot is open to debate.
Comments Off on Sunday May 3, 2009
Saturday, April 25, 2009
State of Play
Footprints from California today
(all by a person or persons using Firefox browsers):
7:10 AM
http://m759.xanga.com/679142359/concepts-of-space/?
Concepts of Space: Euclid vs. Galois
8:51 AM
http://m759.xanga.com/689601851/art-wars-continued/?
Art Wars continued: Behind the Picture
1:33 PM
http://m759.xanga.com/678995132/a-riff-for-dave/?
A Riff for Dave: Me and My Shadow
2:11 PM
http://m759.xanga.com/638308002/a-death-of-kings/?
A Death of Kings: In Memory of Bobby Fischer
2:48 PM
http://m759.xanga.com/691644175/art-wars-in-review–/?
Art Wars in review– Through the Looking Glass: A Sort of Eternity
3:28 PM and
http://m759.xanga.com/684680406/annals-of-philosophy/?
Annals of Philosophy: The Dormouse of Perception
4:28 PM
http://m759.xanga.com/641536988/epiphany-for-roy-part-i/?
Epiphany for Roy, Part I
6:03 PM
http://m759.xanga.com/641949564/art-wars-continued/?
At the Still Point: All That Jazz
6:22 PM
http://m759.xanga.com/644330798/where-entertainment-is-not-god/?
Where Entertainment is Not God: The Just Word
7:14 PM
http://m759.xanga.com/643490468/happy-new-yorker-day/?
Happy New Yorker Day– Class Galore
7:16 PM
http://m759.xanga.com/643812753/the-politics-of-change/?
The Politics of Change: Jumpers
"Relax," said the night man.
"We are programmed to receive."
— Hotel California
Comments Off on Saturday April 25, 2009
Thursday, April 23, 2009
Comments Off on Thursday April 23, 2009
Friday, April 17, 2009
Begettings of
the Broken Bold
Thanks for the following
quotation (“Non deve…
nella testa“) go to the
weblog writer who signs
himself “Conrad H. Roth.”
|
… Yesterday I took leave of my Captain, with a promise of visiting him at Bologna on my return. He is a true
A PAPAL SOLDIER’S IDEAS OF PROTESTANTS 339
representative of the majority of his countrymen. Here, however, I would record a peculiarity which personally distinguished him. As I often sat quiet and lost in thought he once exclaimed “Che pensa? non deve mai pensar l’uomo, pensando s’invecchia;” which being interpreted is as much as to say, “What are you thinking about: a man ought never to think; thinking makes one old.” And now for another apophthegm of his; “Non deve fermarsi l’uomo in una sola cosa, perche allora divien matto; bisogna aver mille cose, una confusione nella testa;” in plain English, “A man ought not to rivet his thoughts exclusively on any one thing, otherwise he is sure to go mad; he ought to have in his head a thousand things, a regular medley.”
Certainly the good man could not know that the very thing that made me so thoughtful was my having my head mazed by a regular confusion of things, old and new. The following anecdote will serve to elucidate still more clearly the mental character of an Italian of this class. Having soon discovered that I was a Protestant, he observed after some circumlocution, that he hoped I would allow him to ask me a few questions, for he had heard such strange things about us Protestants that he wished to know for a certainty what to think of us.
|
Notes for Roth:
The title of this entry,
“Begettings of the Broken Bold,”
is from Wallace Stevens’s
“The Owl in the Sarcophagus”–
This was peace after death, the brother of sleep,
The inhuman brother so much like, so near,
Yet vested in a foreign absolute,
Adorned with cryptic stones and sliding shines,
An immaculate personage in nothingness,
With the whole spirit sparkling in its cloth,
Generations of the imagination piled
In the manner of its stitchings, of its thread,
In the weaving round the wonder of its need,
And the first flowers upon it, an alphabet
By which to spell out holy doom and end,
A bee for the remembering of happiness.
Peace stood with our last blood adorned, last mind,
Damasked in the originals of green,
A thousand begettings of the broken bold.
This is that figure stationed at our end,
Always, in brilliance, fatal, final, formed
Out of our lives to keep us in our death....
|
Related material:
Some further context:
Roth’s entry of Nov. 3, 2006–
“Why blog, sinners?“–
and Log24 on that date:
“First to Illuminate.”
Comments Off on Friday April 17, 2009
Thursday, April 16, 2009
Comments Off on Thursday April 16, 2009
Friday, April 10, 2009
Pilate Goes
to Kindergarten
“There is a pleasantly discursive
treatment of Pontius Pilate’s
unanswered question
‘What is truth?’.”
— H. S. M. Coxeter, 1987,
introduction to Trudeau’s
remarks on the “Story Theory“
of truth as opposed to the
“Diamond Theory” of truth in
The Non-Euclidean Revolution
Consider the following question in a paper cited by V. S. Varadarajan:
E. G. Beltrametti, “Can a finite geometry describe physical space-time?” Universita degli studi di Perugia, Atti del convegno di geometria combinatoria e sue applicazioni, Perugia 1971, 57–62.
Simplifying:
“Can a finite geometry describe physical space?”
Simplifying further:
“Yes. Vide ‘The Eightfold Cube.'”
Comments Off on Friday April 10, 2009
Wednesday, April 8, 2009
“Since 1963, when Pynchon’s first novel, V., came out, the writer– widely considered America’s most important novelist since World War II– has become an almost mythical figure, a kind of cross between the Nutty Professor (Jerry Lewis’s) and Caine in Kung Fu.”
— Nancy Jo Sales in the November 11, 1996, issue of New York Magazine
A Cross Between
(Click on images for their
source in past entries.)
Comments Off on Wednesday April 8, 2009
Saturday, April 4, 2009
Steiner Systems
"Music, mathematics, and chess are in vital respects dynamic acts of location. Symbolic counters are arranged in significant rows. Solutions, be they of a discord, of an algebraic equation, or of a positional impasse, are achieved by a regrouping, by a sequential reordering of individual units and unit-clusters (notes, integers, rooks or pawns). The child-master, like his adult counterpart, is able to visualize in an instantaneous yet preternaturally confident way how the thing should look several moves hence. He sees the logical, the necessary harmonic and melodic argument as it arises out of an initial key relation or the preliminary fragments of a theme. He knows the order, the appropriate dimension, of the sum or geometric figure before he has performed the intervening steps. He announces mate in six because the victorious end position, the maximally efficient configuration of his pieces on the board, lies somehow 'out there' in graphic, inexplicably clear sight of his mind…."
"… in some autistic enchantment,
pure as one of Bach's inverted canons or Euler's formula for polyhedra."
— George Steiner, "A Death of Kings," in The New Yorker, issue dated Sept. 7, 1968
Comments Off on Saturday April 4, 2009
Annual Tribute to
The Eight
Other knight figures:
Click on the SpringerLink
knight for a free copy
(pdf, 1.2 mb) of
the following paper
dealing with the geometry
underlying the R.T. Curtis
knight figures above:
Context:
Literature and Chess and
Sporadic Group References
Details:
| Adapted (for HTML) from the opening paragraphs of the above paper, W. Jonsson's 1970 "On the Mathieu Groups M22, M23, M24…"–
"[A]… uniqueness proof is offered here based upon a detailed knowledge of the geometric aspects of the elementary abelian group of order 16 together with a knowledge of the geometries associated with certain subgroups of its automorphism group. This construction was motivated by a question posed by D.R. Hughes and by the discussion Edge [5] (see also Conwell [4]) gives of certain isomorphisms between classical groups, namely
PGL(4,2)~PSL(4,2)~SL(4,2)~A8,
PSp(4,2)~Sp(4,2)~S6,
where A8 is the alternating group on eight symbols, S6 the symmetric group on six symbols, Sp(4,2) and PSp(4,2) the symplectic and projective symplectic groups in four variables over the field GF(2) of two elements, [and] PGL, PSL and SL are the projective linear, projective special linear and special linear groups (see for example [7], Kapitel II).
The symplectic group PSp(4,2) is the group of collineations of the three dimensional projective space PG(3,2) over GF(2) which commute with a fixed null polarity tau…."
References
4. Conwell, George M.: The three space PG(3,2) and its group. Ann. of Math. (2) 11, 60-76 (1910).
5. Edge, W.L.: The geometry of the linear fractional group LF(4,2). Proc. London Math. Soc. (3) 4, 317-342 (1954).
7. Huppert, B.: Endliche Gruppen I. Berlin-Heidelberg-New York: Springer 1967. |
Comments Off on Saturday April 4, 2009
Friday, April 3, 2009
Notes on Finite Geometry
The web pages at finitegeometry.org are currently down, but most of them are still available at the Internet Archive.
Comments Off on Friday April 3, 2009
“Philosophers ponder the idea of identity: what it is to give something a name on Monday and have it respond to that name on Friday….”
— Bernard Holland in The New York Times of Monday, May 20, 1996
Yesterday’s afternoon entry cited philosopher John Holbo on chess. This, together with Holland’s remark above and Monday’s entries on Zizek, suggests…
Holbo on Zizek(
pdf, 11 pages)
In this excellent analysis,
Holbo quotes Kierkegaard:
“… the knight of faith
‘has the pain of being unable to
make himself intelligible to others'”
(Kierkegaard, Fear and Trembling)
For some material that may serve to illustrate Kierkegaard’s remark, see Log24 on
Twelfth Night and
Epiphany this year.
“… There was a problem laid out on the board, a six-mover. I couldn’t solve it, like a lot of my problems. I reached down and moved a knight…. I looked down at the chessboard. The move with the knight was wrong. I put it back where I had moved it from. Knights had no meaning in this game. It wasn’t a game for knights.”
— Raymond Chandler, The Big Sleep
Context: the five entries
ending at 9:26 AM
on March 10, 2009…
and, for Kierkegaard,
Diamonds Are Forever.
Comments Off on Friday April 3, 2009
Thursday, April 2, 2009
Transformative
Hermeneutics
In memory of
physics historian
Martin J. Klein,
(June 25, 1924-
March 28, 2009)
"… in physics itself, there was what appeared, briefly, to be an ending, which then very quickly gave way to a new beginning: The quest for the ultimate building-blocks of the universe had been taken down to the molecular level in nineteenth-century kinetic theory… and finally to the nuclear level in the second and third decades of the twentieth century. For a moment in the 1920s the quest appeared to have ended…. However… this paradise turned out to be, if not exactly a fool's paradise, then perhaps an Eden lost."
— No Truth Except in the Details: Essays in Honor of Martin J. Klein, introduction by A.J. Kox and Daniel Siegel, June 25, 1994
New York Times obituary dated April 1, 2009:
"Martin J. Klein, a historian of modern physics…. died Saturday, [March 28, 2009] in Chapel Hill, N.C. He was 84 and lived in Chapel Hill."
Klein edited, among other things, Paul Ehrenfest: Collected Scientific Papers (publ. by North-Holland, Amsterdam, 1959).
Related material:
"Almost every famous chess game
is a well-wrought urn
in Cleanth Brooks’ sense."
— John Holbo,
Now We See
Wherein Lies the Pleasure
"The entire sequence of moves in these… chapters reminds one– or should remind one– of a certain type of chess problem where the point is not merely the finding of a mate in so many moves, but what is termed 'retrograde analysis'…."
— Vladimir Nabokov, foreword to The Defense
Comments Off on Thursday April 2, 2009
Sunday, March 29, 2009
Getting All
the Meaning In
Webpage heading for the
2009 meeting of the
American Comparative
Literature Association:
The mysterious symbols on
the above map suggest the
following reflections:
From A Cure of the Mind: The Poetics of Wallace Stevens, by Theodore Sampson, published by Black Rose Books Ltd., 2000–
Page x:
"… if what he calls 'the spirit's alchemicana' (CP [Collected Poems] 471) addresses itself to the irrational element in poetry, to what extent is such an element dominant in his theory and practice of poetry, and therefore in what way is Stevens' intricate verbal music dependent on his irrational use of language– a 'pure rhetoric of a language without words?' (CP 374)?"
Related material:
| From "'When Novelists Become Cubists:' The Prose Ideograms of Guy Davenport," by Andre Furlani:
Laurence Zachar argues that Davenport's writing is situated "aux frontieres intergeneriques" where manifold modes are brought into concord: "L'etonnant chez Davenport est la facon don't ce materiau qui parait l'incarnation meme du chaos– hermetique, enigmatique, obscur, avec son tropplein de references– se revele en fait etre construit, ordonne, structure. Plus l'on s'y plonge, et plus l'on distingue de cohesion dans le texte." 'What astonishes in Davenport is the way in which material that seems the very incarnation of chaos– hermetic, enigmatic, obscure, with its proliferation of allusions– in fact reveals itself to be constructed, organized, structured. The more one immerses oneself in them the more one discerns the texts' cohesion.' (62).
Davenport also works along the intergeneric border between text and graphic, for he illustrates many of his texts. (1) "The prime use of words is for imagery: my writing is drawing," he states in an interview (Hoeppfner 123). Visual imagery is not subordinated to writing in Davenport, who draws on the assemblage practice of superimposing image and writing. "I trust the image; my business is to get it onto the page," he writes in the essay "Ernst Machs Max Ernst." "A page, which I think of as a picture, is essentially a texture of images. […] The text of a story is therefore a continuous graph, kin to the imagist poem, to a collage (Ernst, Willi Baumeister, El Lissitzky), a page of Pound, a Brakhage film" (Geography 374-75).
Note:
(1.) Davenport is an illustrator of books (such as Hugh Kenner's The Stoic Comedians and The Counterfeiters) and journals (such as The Kenyon Review, Parnassus, and Paideuma). His art is the subject of Erik Anderson Reece's monograph, A Balance of Quinces, which reveals the inseparable relationship between Davenport's literary and pictorial work.
References:
Davenport, Guy. The Geography of the Imagination. San Francisco: North Point Press, 1981. Rpt. New York: Pantheon, 1992.
Hoepffner, Bernard. "Pleasant Hill: An Interview with Guy Davenport." Conjunctions 24 (1995): 118-24.
Reece, Erik Anderson. A Balance of Quinces: The Paintings and Drawings of Guy Davenport. New York: New Directions, 1996.
Zachar, Laurence. "Guy Davenport: Une Mosaique du genres." Recherches Anglaises et Nord-Americaines 21 (1994): 51-63.
|
"… when novelists become Cubists; that is, when they see the possibilities of making a hieroglyph, a coherent symbol, an ideogram of the total work. A symbol comes into being when an artist sees that it is the only way to get all the meaning in."
— Guy Davenport, The Geography of the Imagination
See also last night's
commentary on the
following symbols:
Comments Off on Sunday March 29, 2009
Saturday, March 28, 2009
The Rest
of the Story
Today's previous entry discussed the hermeneutics of the midday NY and PA lottery numbers.
The rest of the story:
Lotteries
on Reba's
birthday,
2009 |
Pennsylvania
(No revelation) |
New York
(Revelation) |
Mid-day
(No belief) |
No belief,
no revelation
726 |
Revelation
without belief
378 |
Evening
(Belief) |
Belief without
revelation
006 |
Belief and
revelation
091 |
Interpretations of the evening numbers–
The PA evening number, 006, may be viewed as a followup to the PA midday 726 (or 7/26, the birthday of Kate Beckinsale and Carl Jung). Here 006 is the prestigious "00" number assigned to Beckinsale.
Will: Do you like apples?
Clark: Yeah.
Will: Well, I got her number.
How do you like them apples?
— "Good Will Hunting"
The NY evening number, 091, may be viewed as a followup to the NY midday 378 (the number of pages in The Innermost Kernel by Suzanne Gieser, published by Springer, 2005)–
Page 91: The entire page is devoted to the title of the book's Part 3– "The Copenhagen School and Psychology"–
The next page begins: "With the crisis of physics, interest in epistemological and psychological questions grew among many theoretical physicists. This interest was particularly marked in the circle around Niels Bohr."
A particularly
marked circle
from March 15:
The circle above is
marked with a version of
the classic Chinese symbol
adopted as a personal emblem
by Danish physicist Niels Bohr,
leader of the Copenhagen School.
"Two things of opposite natures seem to depend
On one another, as a man depends
On a woman, day on night, the imagined
On the real. This is the origin of change.
Winter and spring, cold copulars, embrace
And forth the particulars of rapture come."
-- Wallace Stevens,
"Notes Toward a Supreme Fiction,"
Canto IV of "It Must Change"
The square above is marked
with a graphic design
related to the four-diamond
figure of Jung's Aion.
Comments Off on Saturday March 28, 2009
Saturday, March 21, 2009
Counters in Rows
"Music, mathematics, and chess are in vital respects dynamic acts of location. Symbolic counters are arranged in significant rows. Solutions, be they of a discord, of an algebraic equation, or of a positional impasse, are achieved by a regrouping, by a sequential reordering of individual units and unit-clusters (notes, integers, rooks or pawns)."
— George Steiner
(See March 10, "Language Game.")
Comments Off on Saturday March 21, 2009
Thursday, March 19, 2009
Two-Face
[Note: Janus is Roman, not Greek, and
the photo is from one “Fubar Obfusco”] 
Click on image for details.
From January 8:
Religion and Narrative, continued:
| A Public Square
In memory of
Richard John Neuhaus,
who died today at 72:
“It seems, as one becomes older,
That the past has another pattern,
and ceases to be a mere sequence….”
— T. S. Eliot, Four Quartets
Click on image for details.
See also The Folding.
Posted 1/8/2009 7:00 PM |
|
Context:
Notes on Mathematics and Narrative
(entries in chronological order,
March 13 through 19)
Comments Off on Thursday March 19, 2009
Tuesday, March 17, 2009
"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."
— Edward Buckner at The Logic Museum
From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:
The Semiotic Square
"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."
* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter
Another version of the semiotic square:
Krauss says that her figure "is, of course, a Klein Group."
Here is a more explicit figure representing the Klein group:
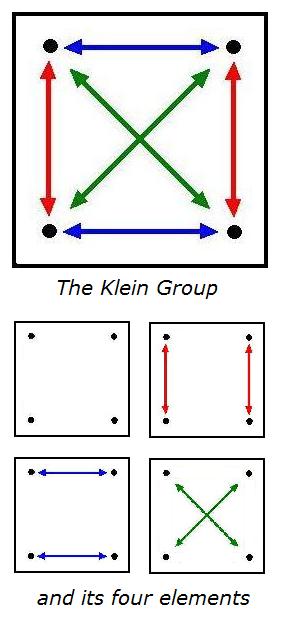
There is also the logical
diamond of opposition —
A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
a Marxist literary theorist:
|
"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–
The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.)
Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1):
As Jameson explains in the Foreword to Greimas' On Meaning, "-s 1 and -s 2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.
* Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.
|
"The Game in the Ship cannot be approached as a job, a vocation, a career, or a recreation. To the contrary, it is Life and Death itself at work there. In the Inner Game, we call the Game Dhum Welur, the Mind of God."
— The Gameplayers of Zan, by M.A. Foster
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
Gravity's Rainbow
Crosses used by semioticians
to baffle their opponents
are illustrated above.
Some other kinds of crosses,
and another kind of opponent:
Monday, July 11, 2005
Logos
for St. Benedict's Day
Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org.
 
Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth. Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion. I personally prefer the "diamond theory" of truth, represented by the logo below.
See also the previous entry
(below) and the entries
of 7/11, 2003.
Sunday, July 10, 2005
|
From Artemiadis's website:
|
|
1986:
|
Elected Regular Member
of the Academy of Athens
|
|
1999:
|
Vice President
of the Academy of Athens
|
|
2000:
|
President
of the Academy of Athens
|
"First of all, I'd like to
thank the Academy…"
— Remark attributed to Plato
|
Comments Off on Tuesday March 17, 2009
Monday, March 16, 2009
(Cf. Sinatra’s birthday, 2004)
This is, in turn, related to
Harvard’s
Barry Mazur‘s recent
essay on time in mathematics
and literature
(pdf).
L’Chaim.
Comments Off on Monday March 16, 2009
Sunday, March 15, 2009
The Origin of Change
A note on the figure
from this morning's sermon:
"Two things of opposite natures seem to depend
On one another, as a man depends
On a woman, day on night, the imagined
On the real. This is the origin of change.
Winter and spring, cold copulars, embrace
And forth the particulars of rapture come."
-- Wallace Stevens,
"Notes Toward a Supreme Fiction,"
Canto IV of "It Must Change"
Comments Off on Sunday March 15, 2009
Angels, Demons,
"Symbology"
"On Monday morning, 9 March, after visiting the Mayor of Rome and the Municipal Council on the Capitoline Hill, the Holy Father spoke to the Romans who gathered in the square outside the Senatorial Palace…
'… a verse by Ovid, the great Latin poet, springs to mind. In one of his elegies he encouraged the Romans of his time with these words:
"Perfer et obdura: multo graviora tulisti."
"Hold out and persist:
you have got through
far more difficult situations."
(Tristia, Liber V, Elegia XI, verse 7).'"
Note the color-interchange
symmetry of each symbol
under 180-degree rotation.
Related material:
The Illuminati Diamond:
Dan Brown's novel Angels & Demons introduced in the year 2000 the fictional academic discipline of "symbology" and a fictional Harvard professor of that discipline, Robert Langdon (named after ambigram* artist John Langdon).
Tom Hanks as Robert Langdon
A possible source for Brown's term "symbology" is a 1995 web page, "The Rotation of the Elements," by one "John Opsopaus." (Cf. Art History Club.)
"The four qualities are the key to understanding the rotation of the elements and many other applications of the symbology of the four elements." –John Opsopaus
* "…ambigrams were common in symbology…." —Angels & Demons
Comments Off on Sunday March 15, 2009
Saturday, March 14, 2009
Flowers for Barry
|
On Time
(in Mathematics and Literature)
“… I want to spend these twenty minutes savoring, and working up, the real complexity of the metaphorical relationship of time and distance– to defamiliarize it for us. And then I will give a few examples of how imaginative literature makes use of the inherent strangeness in this relationship:
Time ↔ Distance.
And finally I will offer my opinion (which I think must be everyone’s opinion) about why we derive significant– but not total– comfort from this equation.”
— Barry Mazur, March 8, 2009, draft (pdf) of talk for conference on comparative literature* |
Another version of
Mazur’s metaphor
Time ↔ Distance:
— Steven H. Cullinane,
October 8, 2003
For some context in
comparative literature,
see Time Fold
(Oct. 10, 2003)
and A Hanukkah Tale
(Dec. 22, 2008).
Related material:
Rat Psychology
yesterday.
Comments Off on Saturday March 14, 2009
Wednesday, March 11, 2009
The Times describes one of the empty rooms on exhibit as…
"… Yves Klein’s 'La spécialisation de la sensibilité à l’état matière première en sensibilité picturale stabilisée, Le Vide' ('The Specialization of Sensibility in the Raw Material State Into Stabilized Pictorial Sensibility, the Void')"
This is a mistranslation. See "An Aesthetics of Matter" (pdf), by Kiyohiko Kitamura and Tomoyuki Kitamura, pp. 85-101 in International Yearbook of Aesthetics, Volume 6, 2002—
"The exhibition «La spécialisation de la sensibilité à l’état matière-première en sensibilité picturale stabilisée», better known as «Le Vide» (The Void) was held at the Gallery Iris Clert in Paris from April 28th till May 5th, 1955." –p. 94
"… «Sensibility in the state of prime matter»… filled the emptiness." –p. 95
Kitamura and Kitamura translate matière première correctly as "prime matter" (the prima materia of the scholastic philosophers) rather than "raw material." (The phrase in French can mean either.)
Comments Off on Wednesday March 11, 2009
Tuesday, March 10, 2009
Language Game
“Music, mathematics, and chess are in vital respects dynamic acts of location. Symbolic counters are arranged in significant rows. Solutions, be they of a discord, of an algebraic equation, or of a positional impasse, are achieved by a regrouping, by a sequential reordering of individual units and unit-clusters (notes, integers, rooks or pawns). The child-master, like his adult counterpart, is able to visualize in an instantaneous yet preternaturally confident way how the thing should look several moves hence. He sees the logical, the necessary harmonic and melodic argument as it arises out of an initial key relation or the preliminary fragments of a theme. He knows the order, the appropriate dimension, of the sum or geometric figure before he has performed the intervening steps. He announces mate in six because the victorious end position, the maximally efficient configuration of his pieces on the board, lies somehow ‘out there’ in graphic, inexplicably clear sight of his mind….”
“… in some autistic enchantment, pure as one of Bach’s inverted canons or Euler’s formula for polyhedra.”
— George Steiner, “A Death of Kings,” in The New Yorker, issue dated Sept. 7, 1968
Related material:
“Classrooms are filled with discussions not of the Bible and Jesus but of 10 ‘core values’– perseverance and curiosity, for instance– that are woven into the curriculum.”
— “Secular Education, Catholic Values,” by Javier C. Hernandez, The New York Times, Sunday, March 8, 2009
“… There was a problem laid out on the board, a six-mover. I couldn’t solve it, like a lot of my problems. I reached down and moved a knight…. I looked down at the chessboard. The move with the knight was wrong. I put it back where I had moved it from. Knights had no meaning in this game. It wasn’t a game for knights.”
— Raymond Chandler, The Big Sleep
The Chandler quotation appears in “Language Game,” an entry in this journal on April 7, 2008.
Some say the “Language Game” date, April 7, is the true date (fixed, permanent) of the Crucifixion– by analogy, Eliot’s “still point” and Jung’s “centre.” (See yesterday, noon.)
Comments Off on Tuesday March 10, 2009
Monday, March 9, 2009
Humorism
"Always with a
little humor."
— Dr. Yen Lo
From Temperament: A Brief Survey
For other interpretations
of the above shape, see
The Illuminati Diamond.
from Jung's Aion:
"From the circle and quaternity motif is derived the symbol of the geometrically formed crystal and the wonder-working stone. From here analogy formation leads on to the city, castle, church, house, room, and vessel. Another variant is the wheel. The former motif emphasizes the ego’s containment in the greater dimension of the self; the latter emphasizes the rotation which also appears as a ritual circumambulation. Psychologically, it denotes concentration on and preoccupation with a centre…." –Jung,
Collected Works, Vol. 9, Part II, paragraph 352
As for rotation, see the ambigrams in Dan Brown's Angels & Demons (to appear as a film May 15) and the following figures:
Comments Off on Monday March 9, 2009
Saturday, March 7, 2009
One or Two Ideas
|
From James Joyce's A Portrait of the Artist as a Young Man:
he hearth and began to stroke his chin.
–When may we expect to have something from you on the esthetic question? he asked.
–From me! said Stephen in astonishment. I stumble on an idea once a fortnight if I am lucky.
–These questions are very profound, Mr Dedalus, said the dean. It is like looking down from the cliffs of Moher into the depths. Many go down into the depths and never come up. Only the trained diver can go down into those depths and explore them and come to the surface again.
–If you mean speculation, sir, said Stephen, I also am sure that there is no such thing as free thinking inasmuch as all thinking must be bound by its own laws.
–Ha!
–For my purpose I can work on at present by the light of one or two ideas of Aristotle and Aquinas.
–I see. I quite see your point.
|
Besides being Mondrian's birthday, today is also the dies natalis (in the birth-into-heaven sense) of St. Thomas Aquinas and, for those who believe worthy pre-Christians also enter heaven, possibly of Aristotle.
Pope Benedict XVI explained the dies natalis concept on Dec. 26, 2006:
"For believers the day of death, and even more the day of martyrdom, is not the end of all; rather, it is the 'transit' towards immortal life. It is the day of definitive birth, in Latin, dies natalis."
The Pope's remarks on that date
were in St. Peter's Square.
Pictorial version
of Hexagram 20,
Contemplation (View)
Comments Off on Saturday March 7, 2009
Friday, March 6, 2009
The Illuminati Stone
TV listing for this evening —
Family Channel, 7:30 PM:
"Harry Potter and
the Sorcerer's Stone"
In other entertainment news —
Scheduled to open May 15:
"Only gradually did I discover
what the mandala really is:
'Formation, Transformation,
Eternal Mind's eternal recreation'"
(Faust, Part Two)
— Carl Gustav Jung
Related material:
|
"For just about half a century, E.J. Holmyard's concisely-titled Alchemy has served as a literate, well-informed, and charming introduction to the history and literature of Western alchemy." —Ian Myles Slater
|
For more about this
"prime matter" (prima materia)
see The Diamond Archetype
and Holy the Firm.
Background:
Holmyard —
— and Aristotle's
On Generation and Corruption.
Comments Off on Friday March 6, 2009
Monday, March 2, 2009
Today in History – March 2
By The Associated Press
Today is Monday, March 2, the 61st day of 2009. There are 304 days left in the year.
Today's Highlight in History:
On March 2, 1939, Roman Catholic Cardinal Eugenio Pacelli was elected Pope on his 63rd birthday; he took the name Pius XII.
|
Log24 on June 9, 2008—
From Gravity's Rainbow (Penguin Classics, 1995), page 563:
"He brings out the mandala he found.
'What's it mean?'
[….]
Slothrop gives him the mandala. He hopes it will work like the mantra that Enzian told him once, mba-kayere (I am passed over), mba-kayere… a spell […]. A mezuzah. Safe passage through a bad night…."
In lieu of Slothrop's mandala, here is another…
Related mandalas:
and
For further details,
click on any of the
three mandalas above.
|
Comments Off on Monday March 2, 2009
Sunday, March 1, 2009
Solomon's Cube
continued
"There is a book… called A Fellow of Trinity, one of series dealing with what is supposed to be Cambridge college life…. There are two heroes, a primary hero called Flowers, who is almost wholly good, and a secondary hero, a much weaker vessel, called Brown. Flowers and Brown find many dangers in university life, but the worst is a gambling saloon in Chesterton run by the Misses Bellenden, two fascinating but extremely wicked young ladies. Flowers survives all these troubles, is Second Wrangler and Senior Classic, and succeeds automatically to a Fellowship (as I suppose he would have done then). Brown succumbs, ruins his parents, takes to drink, is saved from delirium tremens during a thunderstorm only by the prayers of the Junior Dean, has much difficulty in obtaining even an Ordinary Degree, and ultimately becomes a missionary. The friendship is not shattered by these unhappy events, and Flowers's thoughts stray to Brown, with affectionate pity, as he drinks port and eats walnuts for the first time in Senior Combination Room."
— G. H. Hardy, A Mathematician's Apology
"The Solomon Key is the working title of an unreleased novel in progress by American author Dan Brown. The Solomon Key will be the third book involving the character of the Harvard professor Robert Langdon, of which the first two were Angels & Demons (2000) and The Da Vinci Code (2003)." — Wikipedia
"One has O+(6) ≅ S8, the symmetric group of order 8! …."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint.
"The complete projective group of collineations and dualities of the [projective] 3-space is shown to be of order [in modern notation] 8! …. To every transformation of the 3-space there corresponds a transformation of the [projective] 5-space. In the 5-space, there are determined 8 sets of 7 points each, 'heptads' …."
— George M. Conwell, "The 3-space PG(3, 2) and Its Group," The Annals of Mathematics, Second Series, Vol. 11, No. 2 (Jan., 1910), pp. 60-76
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference (July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Comments Off on Sunday March 1, 2009
Saturday, February 28, 2009
Mathematics
and Narrative
continued
Narrative:
xxx
Mathematics:
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference, (July 2000), Springer, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Mathematics:
"Regular graphs are considered, whose automorphism groups are permutation representations P of the orthogonal groups in various dimensions over GF(2). Vertices and adjacencies are defined by quadratic forms, and after graphical displays of the trivial isomorphisms between the symmetric groups S2, S3, S5, S6 and corresponding orthogonal groups, a 28-vertex graph is constructed that displays the isomorphism between S8 and O6 + (2)."
— J. Sutherland Frame in "Orthogonal Groups over GF(2) and Related Graphs," Springer Lecture Notes in Mathematics vol. 642, Theory and Applications of Graphs (Proceedings, Michigan, May 11–15, 1976), edited by Y. Alavi and D. R. Lick, pp. 174-185
"One has O+(6) ≅ S8, the symmetric group of order 8!…."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint. This paper gives some context in superstring theory for the following work of Frame:
[F1] J.S. Frame, The classes and representations of the group of 27 lines and 28 bitangents, Annali
di Mathematica Pura ed Applicata, 32 (1951) 83–119.
[F2] J.S. Frame, Some characters of orthogonal groups over the field of two elements, In: Proc. of the
Second Inter. Conf. on the Theory of Groups, Lecture Notes in Math., Vol. 372, pp. 298–314,
Springer, 1974.
[F3] J. S. Frame, Degree polynomials for the orthogonal groups over GF(2), C. R. Math. Rep. Acad.
Sci. Canada 2 (1980) 253–258.
Comments Off on Saturday February 28, 2009
Friday, February 27, 2009
Time and Chance
continued
Today's Pennsylvania lottery numbers suggest the following meditations…
Midday: Lot 497, Bloomsbury Auctions May 15, 2008– Raum und Zeit (Space and Time), by Minkowski, 1909. Background: Minkowski Space and "100 Years of Space-Time."*
Evening: 5/07, 2008, in this journal– "Forms of the Rock."
Related material:
A current competition at Harvard Graduate School of Design, "The Space of Representation," has a deadline of 8 PM tonight, February 27, 2009.
The announcement of the competition quotes the Marxist Henri Lefebvre on "the social production of space."
A related quotation by Lefebvre (cf. 2/22 2009):
"… an epoch-making event so generally ignored that we have to be reminded of it at every moment. The fact is that around 1910 a certain space was shattered… the space… of classical perspective and geometry…."
— Page 25 of The Production of Space (Blackwell Publishing, 1991)
This suggests, for those who prefer Harvard's past glories to its current state, a different Raum from the Zeit 1910.
In January 1910 Annals of Mathematics, then edited at Harvard, published George M. Conwell's "The 3-space PG(3, 2) and Its Group." This paper, while perhaps neither epoch-making nor shattering, has a certain beauty. For some background, see this journal on February 24, 2009.†
* Ending on Stephen King's birthday, 2008
† Mardi Gras
Comments Off on Friday February 27, 2009
Tuesday, February 24, 2009
Hollywood Nihilism
Meets
Pantheistic Solipsism
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."
|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer…
A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination.
|
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
Heinlein:
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
Stevens:
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in
The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
This interpretation might appeal to Joan Didion, who, as author of the classic novel
Play It As It Lays, is perhaps the world's leading expert on
Hollywood nihilism.
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)
over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Heinlein should perhaps have had in mind
the Klein correspondence when he discussed "some way to project six dimensions into three." While such a projection is of course trivial for anyone who has taken an undergraduate course in linear algebra, the following remarks by Philippe Cara present a much more meaningful mapping, using the Klein correspondence, of structures in six (affine) dimensions to structures in three.
Cara:
Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Comments Off on Tuesday February 24, 2009
Sunday, February 22, 2009
Themes and
Variations
“Designed objects, Brock writes, can be broken down into ‘themes’ and ‘transformations.’ A theme is a motif, such as an S-curve; a transformation might see that curve appear elsewhere in the design, but stretched, rotated 90 degrees, mirrored, or otherwise reworked.
Aesthetic satisfaction comes from an apprehension of how those themes and transformations relate to each other, or of what Brock calls their ‘relative complexity.’ Basically– and this is the nub of it– ‘if the theme is simple, then we are most satisfied when its echoes are complex… and vice versa.'”
Related material:
Theme
and Variations
See also earlier tributes to
Hollywood Game Theory
and Hollywood Religion:
For some variations on the
above checkerboard theme, see
Finite Relativity and
A Wealth of Algebraic Structure.
Comments Off on Sunday February 22, 2009
Friday, February 20, 2009
The Cross
of Constantine
mentioned in
this afternoon's entry
"Emblematizing the Modern"
was the object of a recent
cinematic chase sequence
(successful and inspiring)
starring Mira Sorvino
at the Metropolitan
Museum of Art.
In memory of
Dr. Hunter S. Thompson,
dead by his own hand
on this date
four years ago —
Click for details.
There is
another sort of object
we may associate with a
different museum and with
a modern Constantine …
See "Art Wars for MoMA"
(Dec. 14, 2008).
This object, modern
rather than medieval,
is the ninefold square:
It may suit those who,
like Rosalind Krauss
(see "Emblematizing"),
admire the grids of modern art
but view any sort of Christian
cross with fear and loathing.
For some background that
Dr. Thompson might appreciate,
see notes on Geometry and Death
in this journal, June 1-15, 2007,
and the five Log24 entries
ending at 9 AM Dec. 10. 2006,
which include this astute
observation by J. G. Ballard:
"Modernism's attempt to build a better world with the aid of science and technology now seems almost heroic. Bertolt Brecht, no fan of modernism, remarked that the mud, blood and carnage of the first world war trenches left its survivors longing for a future that resembled a white-tiled bathroom."
Selah.
Comments Off on Friday February 20, 2009
Note that in applications, the vertical axis
of the Cross of Descartes often symbolizes
the timeless (money, temperature, etc.)
while the horizontal axis often symbolizes time.
T.S. Eliot:
"Men’s curiosity searches past and future
And clings to that dimension. But to apprehend
The point of intersection of the timeless
With time, is an occupation for the saint…."
|
There is a reason, apart from her
ethnic origins, that Rosalind Krauss (cf.
9/13/06) rejects, with a shudder, the cross as a key to "the Pandora's box of spiritual reference that is opened once one uses it." The rejection occurs in the context of her attempt to establish not the cross, but the
grid, as a religious symbol:
|
"In suggesting that the success [1] of the grid
is somehow connected to its structure as myth,
I may of course be accused of stretching a point
beyond the limits of common sense, since myths
are stories, and like all narratives they unravel
through time, whereas grids are not only spatial
to start with, they are visual structures
that explicitly reject a narrative
or sequential reading of any kind.
[1] Success here refers to
three things at once:
a sheerly quantitative success,
involving the number of artists
in this century who have used grids;
a qualitative success through which
the grid has become the medium
for some of the greatest works
of modernism; and an ideological
success, in that the grid is able–
in a work of whatever quality–
to emblematize the Modern."
— Rosalind Krauss, "Grids" (1979)
|
Related material:
Time Fold and Weyl on
objectivity and frames of reference.
See also Stambaugh on
The Formless Self
as well as
A Study in Art Education
and
Jung and the Imago Dei.
Comments Off on Friday February 20, 2009
Tuesday, February 17, 2009
Diamond-Faceted:
Transformations
of the Rock
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
A mathematical version of
this poetic concept appears
in a rather cryptic note
from 1981 written with
Stevens's poem in mind:
For some explanation of the
groups of 8 and 24
motions referred to in the note,
see an earlier note from 1981.
For the Perlis "diamond facets,"
see the Diamond 16 Puzzle.
For a much larger group
of motions, see
Solomon's Cube.
As for "the mind itself"
and "possibilities for
human thought," see
Geometry of the I Ching.
Comments Off on Tuesday February 17, 2009
Sunday, February 15, 2009
| From April 28, 2008:
Religious Art
The black monolith of
Kubrick's 2001 is, in
its way, an example
of religious art.
One artistic shortcoming
(or strength– it is, after
all, monolithic) of
that artifact is its
resistance to being
analyzed as a whole
consisting of parts, as
in a Joycean epiphany.
The following
figure does
allow such
an epiphany.
One approach to
the epiphany:
"Transformations play
a major role in
modern mathematics."
– A biography of
Felix Christian Klein
See 4/28/08 for examples
of such transformations.
|
Related material:
| From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, pp. 117-118:
"… his point of origin is external nature, the fount to which we come seeking inspiration for our fictions. We come, many of Stevens's poems suggest, as initiates, ritualistically celebrating the place through which we will travel to achieve fictive shape. Stevens's 'real' is a bountiful place, continually giving forth life, continually changing. It is fertile enough to meet any imagination, as florid and as multifaceted as the tropical flora about which the poet often writes. It therefore naturally lends itself to rituals of spring rebirth, summer fruition, and fall harvest. But in Stevens's fictive world, these rituals are symbols: they acknowledge the real and thereby enable the initiate to pass beyond it into the realms of his fictions.
Two counter rituals help to explain the function of celebration as Stevens envisions it. The first occurs in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer. A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. For in 'Notes Toward a Supreme Fiction' he tells us that
... the first idea was not to shape the clouds
In imitation. The clouds preceded us.
There was a muddy centre before we breathed.
There was a myth before the myth began,
Venerable and articulate and complete.
From this the poem springs: that we live in a place
That is not our own and, much more, not ourselves
And hard it is in spite of blazoned days.
We are the mimics.
(Collected Poems, 383-84)
Believing that they are the life and not the mimics thereof, the world and not its fiction-forming imitators, these young men cannot find the savage transparence for which they are looking. In its place they find the pediment, a scowling rock that, far from being life's source, is symbol of the human delusion that there exists a 'form alone,' apart from 'chains of circumstance.'
A far more productive ritual occurs in 'Sunday Morning.'…." |
For transformations of a more
specifically religious nature,
see the remarks on
Richard Strauss,
"Death and Transfiguration,"
(Tod und Verklärung, Opus 24)
in Mathematics and Metaphor
on July 31, 2008, and the entries
of August 3, 2008, related to the
death of Alexander Solzhenitsyn.
Comments Off on Sunday February 15, 2009
Monday, February 9, 2009
The Vision Thing
The British Academy Awards last night showed two Paul Newman clips:
"Sometimes nothin' can be a real cool hand."
"Boy, I got vision and the rest of the world wears bifocals."
Related material: This journal, September 2008.
As for bifocals…
Pennsylvania Lottery
|
|
|
|
Comments Off on Monday February 9, 2009
Sunday, February 8, 2009
Comments Off on Sunday February 8, 2009
Saturday, February 7, 2009
DENNIS OVERBYE
"From the grave, Albert Einstein poured gasoline on the culture wars between science and religion this week.
A letter the physicist wrote in 1954 to the philosopher Eric Gutkind, in which he described the Bible as 'pretty childish' and scoffed at the notion that the Jews could be a 'chosen people,' sold for $404,000 at an auction in London. That was 25 times the presale estimate."
Einstein did not, at least in the place alleged, call the Bible "childish." Proof:
The image of the letter is
from the Sept./Oct. 2008
Search Magazine.
By the way, today is
the birthday of G. H. Hardy.
Here is an excerpt from his
thoughts on childish things:
"What 'purely aesthetic' qualities can we distinguish in such theorems as Euclid's or Pythagoras's?…. In both theorems (and in the theorems, of course, I include the proofs) there is a very high degree of unexpectedness, combined with inevitability and economy. The arguments take so odd and surprising a form; the weapons used seem so childishly simple when compared with the far-reaching results; but there is no escape from the conclusions."
"Space: what you
damn well have to see."
— James Joyce, Ulysses
Comments Off on Saturday February 7, 2009
Thursday, February 5, 2009
Through the
Looking Glass:
A Sort of Eternity
From the new president’s inaugural address:
“… in the words of Scripture, the time has come to set aside childish things.”
The words of Scripture:
| 9 |
For we know in part, and we prophesy in part. |
| 10 |
But when that which is perfect is come, then that which is in part shall be done away. |
| 11 |
When I was a child, I spake as a child, I understood as a child, I thought as a child: but when I became a man, I put away childish things. |
| 12 |
For now we see through a glass, darkly, but then face to face: now I know in part; but then shall I know even as also I am known.
— First Corinthians 13 |
“through a glass”—
[di’ esoptrou].
By means of
a mirror [esoptron].
Childish things:
© 2005 The Institute for Figuring
Photo by Norman Brosterman
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
Not-so-childish:
Three planes through
the center of a cube
that split it into
eight subcubes:
Through a glass, darkly:
A group of 8 transformations is
generated by affine reflections
in the above three planes.
Shown below is a pattern on
the faces of the 2x2x2 cube
that is symmetric under one of
these 8 transformations–
a 180-degree rotation:
(Click on image
for further details.)
But then face to face:
A larger group of 1344,
rather than 8, transformations
of the 2x2x2 cube
is generated by a different
sort of affine reflections– not
in the infinite Euclidean 3-space
over the field of real numbers,
but rather in the finite Galois
3-space over the 2-element field.
Galois age fifteen,
drawn by a classmate.
These transformations
in the Galois space with
finitely many points
produce a set of 168 patterns
like the one above.
For each such pattern,
at least one nontrivial
transformation in the group of 8
described above is a symmetry
in the Euclidean space with
infinitely many points.
For some generalizations,
see Galois Geometry.
Related material:
The central aim of Western religion–
"Each of us has something to offer the Creator...
the bridging of
masculine and feminine,
life and death.
It's redemption.... nothing else matters."
-- Martha Cooley in The Archivist (1998)
The central aim of Western philosophy–
Dualities of Pythagoras
as reconstructed by Aristotle:
Limited Unlimited
Odd Even
Male Female
Light Dark
Straight Curved
... and so on ....
“Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy.”
— Jamie James in The Music of the Spheres (1993)
“In the garden of Adding
live Even and Odd…
And the song of love’s recision
is the music of the spheres.”
— The Midrash Jazz Quartet in City of God, by E. L. Doctorow (2000)
A quotation today at art critic Carol Kino’s website, slightly expanded:
“Art inherited from the old religion
the power of consecrating things
and endowing them with
a sort of eternity;
museums are our temples,
and the objects displayed in them
are beyond history.”
— Octavio Paz,”Seeing and Using: Art and Craftsmanship,” in Convergences: Essays on Art and Literature (New York: Harcourt Brace Jovanovich 1987), 52
From Brian O’Doherty’s 1976 Artforum essays– not on museums, but rather on gallery space:
“Inside the White Cube“
“We have now reached
a point where we see
not the art but the space first….
An image comes to mind
of a white, ideal space
that, more than any single picture,
may be the archetypal image
of 20th-century art.”
“Space: what you
damn well have to see.”
— James Joyce, Ulysses
|
Comments Off on Thursday February 5, 2009
Monday, February 2, 2009
Against the Day
The Candlebrow Conference
in Pynchon's Against the Day:
The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries.
"Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–"
Quaternion by
S. H. Cullinane
"Um, Professor–"….
… Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"….
… In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions….
"Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions."
"Build a time machine."
"Not the way I would have put it, but if you like, fine."
Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up….
"We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly."
"Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened.
Above, the devastation had begun.
|
Comments Off on Monday February 2, 2009
Sunday, February 1, 2009
Comments Off on Sunday February 1, 2009
Friday, January 30, 2009
Two-Part Invention
This journal on
October 8, 2008,
at noon:
“There is a pleasantly discursive treatment of Pontius Pilate’s unanswered question ‘What is truth?'”
— H. S. M. Coxeter, introduction to Richard J. Trudeau’s remarks on the “story theory” of truth as opposed to the “diamond theory” of truth in The Non-Euclidean Revolution
Trudeau’s 1987 book uses the phrase “diamond theory” to denote the philosophical theory, common since Plato and Euclid, that there exist truths (which Trudeau calls “diamonds”) that are certain and eternal– for instance, the truth in Euclidean geometry that the sum of a triangle’s angles is 180 degrees.
Insidehighered.com on
the same day, October 8, 2008,
at 12:45 PM EDT
“Future readers may consider Updike our era’s Mozart; Mozart was once written off as a too-prolific composer of ‘charming nothings,’ and some speak of Updike that way.”
— Comment by BPJ |
“Birthday, death-day–
what day is not both?”
—
John Updike
Updike died on January 27.
On the same date,
Mozart was born.
Requiem
Mr. Best entered,
tall, young, mild, light.
He bore in his hand
with grace a notebook,
new, large, clean, bright.
— James Joyce, Ulysses,
Shakespeare and Company,
Paris, 1922, page 178 |
Related material:
Comments Off on Friday January 30, 2009
Thursday, January 15, 2009
Comments Off on Thursday January 15, 2009
Wednesday, January 14, 2009
Eight is a Gate
From the most highly
rated negative review:
“I never did figure out
what ‘The Eight’ was.”
Various approaches
to this concept
(click images for details):
Comments Off on Wednesday January 14, 2009
Thursday, January 8, 2009
Report of Arrival
A PBS broadcast of Cyrano de Bergerac was shown yesterday nationally and this evening, a day late, by WNED TV, Buffalo.
From the translation by Anthony Burgess:
Cyrano speaks of falling leaves–
They fall well. With a sort of panache.
They plume down in their last
Loveliness, disguising their fear
Of being dried and pounded to ash
To mix with the common dust.
They go in grace, making their fall appear
Like flying.
ROXANE You’re melancholy today.
CYRANO Never. I’m not the melancholy sort.
ROXANE Very well, then. We’ll let
The leaves of the fall fall while you
Turn the leaves of my gazette.
What’s new at court?
CYRANO … There have been some scandals
To do with witches. A bishop went to heaven,
Or so it’s believed: there’s been as yet no report
Of his arrival….”
Later….
CYRANO … See it there, a white plume
Over the battle– A diamond in the ash
Of the ultimate combustion–
My panache.”
Comments Off on Thursday January 8, 2009
A Public Square
In memory of
Richard John Neuhaus,
who died today at 72:
“It seems, as one becomes older,
That the past has another pattern,
and ceases to be a mere sequence….”
— T. S. Eliot, Four Quartets
See also The Folding.
Comments Off on Thursday January 8, 2009
Tuesday, January 6, 2009
Archetypes, Synchronicity,
and Dyson on Jung
The current (Feb. 2009) Notices of the American Mathematical Society has a written version of Freeman Dyson's 2008 Einstein Lecture, which was to have been given in October but had to be canceled. Dyson paraphrases a mathematician on Carl Jung's theory of archetypes:
"… we do not need to accept Jung’s theory as true in order to find it illuminating."
The same is true of Jung's remarks on synchronicity.
For example —
Yesterday's entry, "A Wealth of Algebraic Structure," lists two articles– each, as it happens, related to Jung's four-diamond figure from Aion as well as to my own Notes on Finite Geometry. The articles were placed online recently by Cambridge University Press on the following dates:
R. T. Curtis's 1974 article defining his Miracle Octad Generator (MOG) was published online on Oct. 24, 2008.
Curtis's 1987 article on geometry and algebraic structure in the MOG was published online on Dec. 19, 2008.
On these dates, the entries in this journal discussed…
Oct. 24:
Cube Space, 1984-2003
Material related to that entry:
Dec. 19:
Art and Religion: Inside the White Cube
That entry discusses a book by Mark C. Taylor:
The Picture in Question: Mark Tansey and the Ends of Representation (U. of Chicago Press, 1999).
In Chapter 3, "Sutures of Structures," Taylor asks —
"What, then, is a frame, and what is frame work?"
One possible answer —
Hermann Weyl on the relativity problem in the context of the 4×4 "frame of reference" found in the above Cambridge University Press articles.
"Examples are the stained-glass
windows of knowledge."
— Vladimir Nabokov
Comments Off on Tuesday January 6, 2009
Monday, January 5, 2009
A 1987 article by R. T. Curtis on the geometry of his Miracle Octad Generator (MOG) as it relates to the geometry of the 4×4 square is now available online ($20):
Further elementary techniques using the miracle octad generator, by R. T. Curtis. Abstract:
“In this paper we describe various techniques, some of which are already used by devotees of the art, which relate certain maximal subgroups of the Mathieu group M24, as seen in the MOG, to matrix groups over finite fields. We hope to bring out the wealth of algebraic structure* underlying the device and to enable the reader to move freely between these matrices and permutations. Perhaps the MOG was mis-named as simply an ‘octad generator’; in this paper we intend to show that it is in reality a natural diagram of the binary Golay code.”
(Received July 20 1987)
— Proceedings of the Edinburgh Mathematical Society (Series 2) (1989), 32: 345-353, doi:10.1017/S0013091500004600.
(Published online by Cambridge University Press 19 Dec 2008.)
In the above article, Curtis explains how two-thirds of his 4×6 MOG array may be viewed as the 4×4 model of the four-dimensional affine space over GF(2). (His earlier 1974 paper (below) defining the MOG discussed the 4×4 structure in a purely combinatorial, not geometric, way.)
For further details, see The Miracle Octad Generator as well as Geometry of the 4×4 Square and Curtis’s original 1974 article, which is now also available online ($20):
A new combinatorial approach to M24, by R. T. Curtis. Abstract:
“In this paper, we define M24 from scratch as the subgroup of S24 preserving a Steiner system S(5, 8, 24). The Steiner system is produced and proved to be unique and the group emerges naturally with many of its properties apparent.”
(Received June 15 1974)
— Mathematical Proceedings of the Cambridge Philosophical Society (1976), 79: 25-42, doi:10.1017/S0305004100052075.
(Published online by Cambridge University Press 24 Oct 2008.)
* For instance:
Click for details.
Comments Off on Monday January 5, 2009
Monday, December 22, 2008
The Folding
Hamlet, Act 1, Scene 5 —
Ghost:
"I could a tale unfold whose lightest word
Would harrow up thy soul, freeze thy young blood,
Make thy two eyes, like stars, start from their spheres,
Thy knotted and combined locks to part
And each particular hair to stand on end,
Like quills upon the fretful porpentine:
But this eternal blazon must not be
To ears of flesh and blood. List, list, O, list!"
This recalls the title of a piece in this week's New Yorker:"The Book of Lists:
Susan Sontag’s early journals." (See Log24 on Thursday, Dec. 18.)
In the rather grim holiday spirit of that piece, here are some journal notes for Sontag, whom we may imagine as the ghost of Hanukkah past.
There are at least two ways of folding a list (or tale) to fit a rectangular frame.The normal way, used in typesetting English prose and poetry, starts at the top, runs from left to right, jumps down a line, then again runs left to right, and so on until the passage is done or the bottom right corner of the frame is reached.
The boustrophedonic way again goes from top to bottom, with the first line running from left to right, the next from right to left, the next from left to right, and so on, with the lines' directions alternating.
The word "boustrophedon" is from the Greek words describing the turning, at the end of each row, of an ox plowing (or "harrowing") a field.
The Tale of
the Eternal Blazon
by Washington Irving
"Blazon meant originally a shield, and then the heraldic bearings on a shield.
Later it was applied to the art of describing or depicting heraldic bearings
in the proper manner; and finally the term came to signify ostentatious display
and also description or record by words or other means. In Hamlet, Act I. Sc. 5,
the Ghost, while talking with Prince Hamlet, says:
'But this eternal blazon
must not be
To ears of flesh and blood.'
Eternal blazon signifies revelation or description of things pertaining to eternity."
— Irving's Sketch Book, p. 461
By Washington Irving and Mary Elizabeth Litchfield, Ginn & Company, 1901
Related material:
Folding (and harrowing up)
some eternal blazons —
These are the foldings
described above.
They are two of the 322,560
natural ways to fit
the list (or tale)
"1, 2, 3, … 15, 16"
into a 4×4 frame.
For further details, see
The Diamond 16 Puzzle.
Moral of the tale:
Cynthia Zarin in The New Yorker, issue dated April 12, 2004–
"Time, for L'Engle, is accordion-pleated. She elaborated, 'When you bring a sheet off the line, you can't handle it until it's folded, and in a sense, I think, the universe can't exist until it's folded– or it's a story without a book.'"
Comments Off on Monday December 22, 2008
Fides et Ratio
Part I:
Ratio
Continued from…
|
Makin' the Changes
(From "Flag Matroids," by
Borovik, Gelfand, and White)
Edward Rothstein,
White and Geometric,
but not Eternal.
|
Part II:
Fides
For more information,
click on the cocktail.
Comments Off on Monday December 22, 2008
Sunday, December 21, 2008
Interpretive Grids
The 15 grids in the picture at right above may be regarded as
interpreting the structure of the space at left above.
This pair of pictures was suggested by yesterday’s entry at Ars Mathematica containing the phrase “a dramatic extension of the notion of points.”
For other uses of the phrase “interpretive grid,” see today’s previous entry.
Comments Off on Sunday December 21, 2008
Friday, December 19, 2008
Inside the
White Cube
Part I: The White Cube
Part II: Inside
Part III: Outside
Click to enlarge.
Mark Tansey, The Key (1984)
For remarks on religion
related to the above, see
Log24 on the Garden of Eden
and also Mark C. Taylor,
"What Derrida Really Meant"
(New York Times, Oct. 14, 2004).
For some background on Taylor,
see Wikipedia. Taylor, Chairman
of the Department of Religion at
Columbia University, has a
1973 doctorate in religion from
Harvard University. His opinion
of Derrida indicates that his
sympathies lie more with
the serpent than with the angel
in the Tansey picture above.
For some remarks by Taylor on
the art of Tansey relevant to the
structure of the white cube
(Part I above), see Taylor's
The Picture in Question:
Mark Tansey and the
Ends of Representation
(U. of Chicago Press, 1999):
From Chapter 3,
"Sutures* of Structures," p. 58:
"What, then, is a frame, and what is frame work?
This question is deceptive in its simplicity. A frame is, of course, 'a basic skeletal structure designed to give shape or support' (American Heritage Dictionary)…. when the frame is in question, it is difficult to determine what is inside and what is outside. Rather than being on one side or the other, the frame is neither inside nor outside. Where, then, Derrida queries, 'does the frame take place….'"
* P. 61:
"… the frame forms the suture of structure. A suture is 'a seamless [sic**] joint or line of articulation,' which, while joining two surfaces, leaves the trace of their separation."
** A dictionary says "a seamlike joint or line of articulation," with no mention of "trace," a term from Derrida's jargon.
|
Comments Off on Friday December 19, 2008
Tuesday, December 16, 2008
The Square Wheel
(continued)
From The n-Category Cafe today:
David Corfield at 2:33 PM UTC quoting a chapter from a projected second volume of a biography:
"Grothendieck’s spontaneous reaction to whatever appeared to be causing a difficulty… was to adopt and embrace the very phenomenon that was problematic, weaving it in as an integral feature of the structure he was studying, and thus transforming it from a difficulty into a clarifying feature of the situation."
John Baez at 7:14 PM UTC on research:
"I just don’t want to reinvent a wheel, or waste my time inventing a square one."
For the adoption and embracing of such a problematic phenomenon, see The Square Wheel (this journal, Sept. 14, 2004).
For a connection of the square wheel with yesterday's entry for Julie Taymor's birthday, see a note from 2002:
Comments Off on Tuesday December 16, 2008
Monday, December 15, 2008
Happy Birthday,
Julie Taymor
"Julie Taymor… will be directing Helen Mirren in a big-screen adaptation of
The Tempest. Dame Helen, in a gender-switch from the original, will be playing Prospera, the usurped Duchess possessed of a vast library and magical powers."
— John Murphy at Bardolatry.com on November 21, 2008
A vast library…
On searching for Garden of Eden patterns (GEP's):
"The grid is a staircase to the Universal…."
— Rosalind Krauss, quoted here on Weyl's birthday, 2004
"I find the whole topic of GEPs a deeply interesting one, from many viewpoints: mathematical, philosophical, physical….
… the obvious problem is, that the required computational time is growing rapidly with the size of the grid, and even for a small grid, like 4×4 (=16 cells) there are 216=65536 possible patterns…."
— cateye at RichardDawkins.net
… and magical powers
The date of cateye's post was Sunday, October 21, 2007.
For related material see Log24 on Sunday, October 21, 2007.
Comments Off on Monday December 15, 2008
Sunday, December 14, 2008
Epigraphs
The New York Times of Sunday, May 6, 2007, on a writer of pulp fiction:
His early novels, written in two weeks or less, were published in double-decker Ace paperbacks that included two books in one, with a lurid cover for each. “If the Holy Bible was printed as an Ace Double,” an editor once remarked, “it would be cut down to two 20,000-word halves with the Old Testament retitled as ‘Master of Chaos’ and the New Testament as ‘The Thing With Three Souls.'”
Epigraph for Part One:
“Ours is a very gutsy religion, Cullinane.“
— James A. Michener
Lurid cover:
The Pussycat
Epigraph for Part Two:
“Beware lest you believe that you can comprehend the Incomprehensible….“
— Saint Bonaventure
Lurid cover:
The Owl
Click on the image for a
relevant Wallace Stevens poem.
Comments Off on Sunday December 14, 2008
Friday, December 12, 2008
On the Symmetric Group S8
Wikipedia on Rubik's 2×2×2 "Pocket Cube"–
"Any permutation of the 8 corner cubies is possible (8! positions)."
Some pages related to this claim–
Simple Groups at Play
Analyzing Rubik's Cube with GAP
Online JavaScript Pocket Cube.
The claim is of course trivially true for the unconnected subcubes of Froebel's Third Gift:
© 2005 The Institute for Figuring
Comments Off on Friday December 12, 2008
Wednesday, December 10, 2008
Comments Off on Wednesday December 10, 2008
Sunday, December 7, 2008
Space and
the Soul
On a book by Margaret Wertheim:
“She traces the history of space beginning with the cosmology of Dante. Her journey continues through the historical foundations of celestial space, relativistic space, hyperspace, and, finally, cyberspace.” –Joe J. Accardi, Northeastern Illinois Univ. Lib., Chicago, in Library Journal, 1999 (quoted at Amazon.com)
There are also other sorts of space.
© 2005 The Institute for Figuring
Comments Off on Sunday December 7, 2008
Saturday, December 6, 2008
Another Opening,
Another Show
"While feasts of Saint Nicholas are not observed nationally, cities with strong German influences like Milwaukee, Cincinnati, and St. Louis celebrate St. Nick's Day on a scale similar to the German custom." —
Wikipedia
A footprint from Germany:
The link in the above footprint leads
to an entry of July 5, 2006.
The access method:
|
"The Python urllib module implements a fairly high-level abstraction for making any web object with a URL act like a Python file: i.e., you open it, and get back an object…."
|
For more pictures and discussion
of the object fetched by Python,
see
AntiChristmas 2007.
For a larger and more sophisticated
relative of that object,
see Solomon's Cube and
the related three presents
from the German link's target:
Comments Off on Saturday December 6, 2008
Surprise Package
Comments Off on Saturday December 6, 2008
Friday, December 5, 2008
Comments Off on Friday December 5, 2008
Thursday, December 4, 2008
Comments Off on Thursday December 4, 2008
Sunday, November 30, 2008
Abstraction and Faith
|
From Sol LeWitt: A Retrospective, edited by Gary Garrels, Yale University Press, 2000, p. 376:
THE SQUARE AND THE CUBE
by Sol LeWitt
The best that can be said for either the square or the cube is that they are relatively uninteresting in themselves. Being basic representations of two- and three-dimensional form, they lack the expressive force of other more interesting forms and shapes. They are standard and universally recognized, no initiation being required of the viewer; it is immediately evident that a square is a square and a cube a cube. Released from the necessity of being significant in themselves, they can be better used as grammatical devices from which the work may proceed.
Reprinted from Lucy R. Lippard et al., "Homage to the Square," Art in America 55, No. 4 (July-August 1967): 54. (LeWitt's contribution was originally untitled.)
|
A vulgarized version
of LeWitt's remarks
appears on a webpage of
the National Gallery of Art.
|
Today's Sermon
"Closing the Circle on Abstract Art"
On Kirk Varnedoe's National Gallery lectures in 2003 (Philip Kennicott, Washington Post, Sunday, May 18, 2003):
"Varnedoe's lectures were ultimately about faith, about his faith in the power of abstraction, and abstraction as a kind of anti-religious faith in itself."
|
Comments Off on Sunday November 30, 2008
Wednesday, November 26, 2008
Meanwhile…
Recent abstracts of interest:
Kuwait Foundation Lectures —
Jan. 29, 2008: J. P. Wintenberger, “On the Proof of Serre’s Conjecture“
Oct. 28, 2008: Chandrashekhar Khare, “Modular Forms and Galois Representations“
Background:
The Last Theorem, a novel by Arthur C. Clarke and Frederik Pohl published Aug. 5, 2008
“Going Beyond Fermat’s Last Theorem,” a news article in The Hindu published April 25, 2005
Wikipedia: Serre Conjecture (Number Theory)
Henri Darmon, “Serre’s Conjectures“
Comments Off on Wednesday November 26, 2008
Monday, November 24, 2008
Frame Tale
Click on image for details.
Comments Off on Monday November 24, 2008
Sunday, November 23, 2008
The Idea of Identity
“The first credential
we should demand of a critic
is his ideograph of the good.”
— Ezra Pound,
How to Read
Music critic Bernard Holland in The New York Times on Monday, May 20, 1996:
The Juilliard’s
Half-Century Ripening
Philosophers ponder the idea of identity: what it is to give something a name on Monday and have it respond to that name on Friday regardless of what might have changed in the interim. Medical science tells us that the body’s cells replace themselves wholesale within every seven years, yet we tell ourselves that we are what we were….
Schubert at the end of his life had already passed on to another level of spirit. Beethoven went back and forth between the temporal world and the world beyond right up to his dying day.
Exercise
Part I:
Apply Holland’s Monday-to-Friday “idea of identity” to the lives and deaths during the week of Monday, Nov. 10 (“Frame Tales“), through Friday, Nov. 14, of a musician and a maker of music documentaries– Mitch Mitchell (d. Nov. 12) and Baird Bryant (d. Nov. 13).
Part II:
Apply Holland’s “idea of identity” to last week (Monday, Nov. 17, through Friday, Nov. 21), combining it with Wigner’s remarks on invariance (discussed here on Monday) and with the “red dragon” (Log24, Nov. 15) concept of flight over “the Hump”– the Himalayas– and the 1991 documentary filmed by Bryant, “Heart of Tibet.”
Part III:
Discuss Parts I and II in the context of Eliot’s Four Quartets. (See Time Fold, The Field of Reason, and Balance.)
Comments Off on Sunday November 23, 2008
Wednesday, November 19, 2008
"Through the unknown,
remembered gate…."
— Four Quartets
(Epigraph to the introduction,
Parallelisms of Complete Designs
by Peter J. Cameron,
Merton College, Oxford)
"It's still the same old story…."
— Song lyric
The Great Gatsby, Chapter 6:
"An instinct toward his future glory had led him, some months before, to the small Lutheran college of St. Olaf in southern Minnesota. He stayed there two weeks, dismayed at its ferocious indifference to the drums of his destiny, to destiny itself, and despising the janitor’s work with which he was to pay his way through."
There is a link to an article on St. Olaf College in Arts & Letters Daily today:
"John Milton, boring? Paradise Lost has a little bit of something for everybody. Hot sex! Hellfire! Some damned good poetry, too…" more»
The "more" link is to The Chronicle of Higher Education.
For related material on Paradise Lost and higher education, see Mathematics and Narrative.
Comments Off on Wednesday November 19, 2008
Monday, November 17, 2008
Limits
From the previous entry:
“If it’s a seamless whole you want,
pray to Apollo, who sets the limits
within which such a work can exist.”
— Margaret Atwood,
author of Cat’s Eye
Happy birthday
to the late
Eugene Wigner
… and a belated
Merry Christmas
to Paul Newman:
“The laws of nature permit us to foresee events on the basis of the knowledge of other events; the principles of
invariance should permit us to establish new correlations between events, on the basis of the knowledge of established correlations between events. This is exactly what they do.”
— Eugene Wigner, Nobel Prize Lecture, December 12, 1963
Comments Off on Monday November 17, 2008
Sunday, November 16, 2008
Art and Lies
Observations suggested by an article on author Lewis Hyde– "What is Art For?"– in today's New York Times Magazine:
Margaret Atwood (pdf) on Lewis Hyde's
Trickster Makes This World: Mischief, Myth, and Art —
"Trickster," says Hyde, "feels no anxiety when he deceives…. He… can tell his lies with creative abandon, charm, playfulness, and by that affirm the pleasures of fabulation." (71) As Hyde says, "… almost everything that can be said about psychopaths can also be said about tricksters," (158), although the reverse is not the case. "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159)
What is "the next world"? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning to join, to fit, and to make. (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
|
"What happened to that… cube?"
Apollinax laughed until his eyes teared. "I'll give you a hint, my dear. Perhaps it slid off into a higher dimension."
"Are you pulling my leg?"
"I wish I were," he sighed. "The fourth dimension, as you know, is an extension along a fourth coordinate perpendicular to the three coordinates of three-dimensional space. Now consider a cube. It has four main diagonals, each running from one corner through the cube's center to the opposite corner. Because of the cube's symmetry, each diagonal is clearly at right angles to the other three. So why shouldn't a cube, if it feels like it, slide along a fourth coordinate?"
— "Mr. Apollinax Visits New York," by Martin Gardner, Scientific American, May 1961, reprinted in The Night is Large
|
Comments Off on Sunday November 16, 2008
From Koestler’s Darkness at Noon, a fictional Communist on propaganda:
“It is necessary to hammer every sentence into the masses by repetition and simplification. What is presented as right must shine like gold; what is presented as wrong must be black as pitch.”
Thanks for this quotation to Kati Marton, author of The Great Escape: Nine Jews Who Fled Hitler and Changed the World (Simon & Schuster, paperback edition Nov. 6, 2007). One of Marton’s nine was Koestler.
From another book related to this exodus:
“Riesz was one of the most elegant mathematical writers in the world, known for his precise, concise, and clear expositions. He was one of the originators of the theory of function spaces– an analysis which is geometrical in nature.”
— Stanislaw Ulam, Adventures of a Mathematician
And from Gian-Carlo Rota, a friend of Ulam:
“Riesz’s example is well worth following today.”
Related material: Misunderstanding in the Theory of Design and Geometry for Jews.
For a different approach to ethnicity and the number nine that is also “geometrical in nature,” see The Pope in Plato’s Cave and the four entries preceding it, as well as A Study in Art Education.
Comments Off on Sunday November 16, 2008
Monday, November 10, 2008
Frame Tales
From June 30 —
("Will this be on the test?")
Frame Tale One:
Frame Tale Two:
Barry Sharples
on his version of the
Kaleidoscope Puzzle —
Background:
"A possible origin of this puzzle is found in a dialogue
between Socrates and Meno written by the Greek philosopher,
Plato, where a square is drawn inside a square such that
the blue square is twice the area of the yellow square.
Colouring the triangles produces a starting pattern
which is a one-diamond figure made up of four tiles
and there are 24 different possible arrangements."
The King and the Corpse —
"The king asked, in compensation for his toils during this strangest
of all the nights he had ever known, that the twenty-four riddle tales
told him by the specter, together with the story of the night itself,
should be made known over the whole earth
and remain eternally famous among men."
Frame Tale Three:
Finnegans Wake —
"The quad gospellers may own the targum
but any of the Zingari shoolerim may pick a peck
of kindlings yet from the sack of auld hensyne."
Comments Off on Monday November 10, 2008
Saturday, November 8, 2008
From a
Cartoon Graveyard
“That corpse you planted
last year in your garden,
Has it begun to sprout?
Will it bloom this year?
Or has the sudden frost
disturbed its bed?”
— T. S. Eliot, “The Waste Land“
Wikipedia:
“In the Roman Catholic tradition, the term ‘Body of Christ’ refers not only to the body of Christ in the spiritual realm, but also to two distinct though related things: the Church and the reality of the transubstantiated bread of the Eucharist….
According to the Catechism of the Catholic Church, ‘the comparison of the Church with the body casts light on the intimate bond between Christ and his Church. Not only is she gathered around him; she is united in him, in his body….’
….To distinguish the Body of Christ in this sense from his physical body, the term ‘Mystical Body of Christ’ is often used. This term was used as the first words, and so as the title, of the encyclical Mystici Corporis Christi of Pope Pius XII.”
Pope Pius XII:
“83. The Sacrament of the Eucharist is itself a striking and wonderful figure of the unity of the Church, if we consider how in the bread to be consecrated many grains go to form one whole, and that in it the very Author of supernatural grace is given to us, so that through Him we may receive the spirit of charity in which we are bidden to live now no longer our own life but the life of Christ, and to love the Redeemer Himself in all the members of His social Body.”
Comments Off on Saturday November 8, 2008
Friday, November 7, 2008
The Sincerest Form
of Flattery
At a British puzzle website today I found this, titled “Tiles Puzzle by Steven H. Cullinane”–
The version there states that
“there are 322,560 patterns made by swapping rows, swapping columns and swapping the four 2×2 quadrants!”
Comments Off on Friday November 7, 2008
Thursday, November 6, 2008
Death of a Classmate
Michael Crichton,
Harvard College, 1964
Authors Michael Crichton and
David Foster Wallace in today’s
New York Times obituaries
The Times’s remarks above
on the prose styles of
Crichton and Wallace–
“compelling formula” vs.
“intricate complexity”–
suggest the following works
of visual art in memory
of Crichton.
“Crystal”—
“Dragon”
(from Crichton’s
Jurassic Park)–
For the mathematics
(dyadic harmonic analysis)
relating these two figures,
see
Crystal and Dragon.
Some philosophical
remarks related to
the Harvard background
that Crichton and I share–
Hitler’s Still Point
and
The Crimson Passion.
Comments Off on Thursday November 6, 2008
Thursday, October 30, 2008
Readings for
Devil’s Night
Comments Off on Thursday October 30, 2008
Friday, October 24, 2008
“The Cube Space” is a name given to the eightfold cube in a vulgarized mathematics text, Discrete Mathematics: Elementary and Beyond, by Laszlo Lovasz et al., published by Springer in 2003. The identification in a natural way of the eight points of the linear 3-space over the 2-element field GF(2) with the eight vertices of a cube is an elementary and rather obvious construction, doubtless found in a number of discussions of discrete mathematics. But the less-obvious generation of the affine group AGL(3,2) of order 1344 by permutations of parallel edges in such a cube may (or may not) have originated with me. For descriptions of this process I wrote in 1984, see Diamonds and Whirls and Binary Coordinate Systems. For a vulgarized description of this process by Lovasz, without any acknowledgement of his sources, see an excerpt from his book.
Comments Off on Friday October 24, 2008
Thursday, October 23, 2008
Comments Off on Thursday October 23, 2008
Wednesday, October 22, 2008
Euclid vs. Galois
On May 4, 2005, I wrote a note about how to visualize the 7-point Fano plane within a cube.
Last month, John Baez showed slides that touched on the same topic. This note is to clear up possible confusion between our two approaches.
From Baez’s Rankin Lectures at the University of Glasgow:
Note that Baez’s statement (
pdf) “Lines in the Fano plane correspond to planes through the origin [the vertex labeled ‘1’] in this cube” is, if taken (wrongly) as a statement about a cube in Euclidean 3-space, false.
The statement is, however, true of the eightfold cube, whose eight subcubes correspond to points of the linear 3-space over the two-element field, if “planes through the origin” is interpreted as planes within that linear 3-space, as in Galois geometry, rather than within the Euclidean cube that Baez’s slides seem to picture.
This Galois-geometry interpretation is, as an article of his from 2001 shows, actually what Baez was driving at. His remarks, however, both in 2001 and 2008, on the plane-cube relationship are both somewhat trivial– since “planes through the origin” is a standard definition of lines in projective geometry– and also unrelated– apart from the possibility of confusion– to my own efforts in this area. For further details, see The Eightfold Cube.
Comments Off on Wednesday October 22, 2008
Wednesday, October 15, 2008
Links for the birthday of the late mathematician Bernhard H. Neumann:
MacTutor biography of Neumann
Variety (Universal Algebra) at Wikipedia
Preface to Varieties of Groups (1967), by Hanna Neumann
Biography (1974 obituary) of Hanna Neumann
Peter M. Neumann home page
Some related notes on algebra suggested by finite geometry:
Dynamic and algebraic compatibility of groups (1985 Dec. 11)
Groups related by a nontrivial identity (1985 Nov. 17)
Transformations over a bridge (1983 Aug. 16)
Group identity algebras (1983 Aug. 4)
I have no idea if any work has been done in this area since my own efforts in 1983-1985.
Comments Off on Wednesday October 15, 2008
Sunday, October 12, 2008
Comments Off on Sunday October 12, 2008
“Elegant”
— Today’s New York Times
review of the Very Rev.
Francis Bowes Sayre Jr.
Related material:
Log24 entries from
the anniversary this
year of Sayre’s birth
and from the date
of his death:
A link from the former
suggests the following
graphic meditation–
(Click on figure for details.)
A link from the latter
suggests another
graphic meditation–
(Click on figure for details.)
Although less specifically
American than the late
Reverend, who was
born in the White House,
hence perhaps irrelevant
to his political views,
these figures are not
without relevance to
his religion, which is
more about metanoia
than about paranoia.
Comments Off on Sunday October 12, 2008
Wednesday, October 8, 2008
Serious Numbers
A Yom Kippur
Meditation
"When times are mysterious
Serious numbers
Will always be heard."
— Paul Simon,
"When Numbers Get Serious"
"There is a pleasantly discursive treatment of Pontius Pilate's unanswered question 'What is truth?'"
— H. S. M. Coxeter, introduction to Richard J. Trudeau's remarks on the "story theory" of truth as opposed to the "diamond theory" of truth in The Non-Euclidean Revolution
Trudeau's 1987 book uses the phrase "diamond theory" to denote the philosophical theory, common since Plato and Euclid, that there exist truths (which Trudeau calls "diamonds") that are certain and eternal– for instance, the truth in Euclidean geometry that the sum of a triangle's angles is 180 degrees. As the excerpt below shows, Trudeau prefers what he calls the "story theory" of truth–
"There are no diamonds. People make up stories about what they experience. Stories that catch on are called 'true.'"
(By the way, the phrase "diamond theory" was used earlier, in 1976, as the title of a monograph on geometry of which Coxeter was aware.)
Excerpt from
The Non-Euclidean RevolutionWhat does this have to do with numbers?
Pilate's skeptical tone suggests he may have shared a certain confusion about geometric truth with thinkers like Trudeau and the slave boy in Plato's Meno. Truth in a different part of mathematics– elementary arithmetic– is perhaps more easily understood, although even there, the existence of what might be called "non-Euclidean number theory"– i.e., arithmetic over finite fields, in which 1+1 can equal zero– might prove baffling to thinkers like Trudeau.
Trudeau's book exhibits, though it does not discuss, a less confusing use of numbers– to mark the location of pages. For some philosophical background on this version of numerical truth that may be of interest to devotees of the Semitic religions on this evening's High Holiday, see Zen and Language Games.
For uses of numbers that are more confusing, see– for instance– the new website The Daily Beast and the old website Story Theory and the Number of the Beast.
Comments Off on Wednesday October 8, 2008
Monday, October 6, 2008
Leap Day of Faith
Yesterday's entry contained the following unattributed quotation:
"One must join forces with friends of like mind."
As the link to Leap Day indicated, the source of the quotation is the I Ching.
Yesterday's entry also quoted the late Terence McKenna, a confused writer on psychosis and the I Ching. Lest the reader conclude that I consider McKenna or similar authors (for instance, Timothy Leary in Cuernavaca) as "friends of like mind," I would point rather to more sober students of the I Ching (cf. my June 2002 notes on philosophy, religion, and science) and to the late Scottish theologian John Macquarrie:
Macquarrie's connection in this journal to the I Ching is, like that book itself, purely coincidental. For details, click on the figure below.
The persistent reader will
find a further link that
leads to an entry titled
"Notes on the I Ching."
McKenna's writing was of value to me for its (garbled) reference to a thought of Alfred North Whitehead:
"A colour is eternal. It haunts time like a spirit. It comes and it goes. But where it comes it is the same colour. It neither survives nor does it live. It appears when it is wanted."
— Science and the Modern World, 1925
Comments Off on Monday October 6, 2008
Friday, September 26, 2008
Christmas Knotfor T.S. Eliot’s birthday
(Continued from Sept. 22–
“A Rose for Ecclesiastes.”)
From Kibler’s
“Variations on a Theme of
Heisenberg, Pauli, and Weyl,”
July 17, 2008:
“It is to be emphasized
that the 15 operators…
are underlaid by the geometry
of the generalized quadrangle
of order 2…. In this geometry,
the five sets… correspond to
a spread of this quadrangle,
i.e., to a set of 5 pairwise
skew lines….”
— Maurice R. Kibler,
July 17, 2008
For ways to visualize
this quadrangle,
see Inscapes.
|
Related material
A remark of Heisenberg
quoted here on Christmas 2005:
“… die Schönheit… [ist] die
richtige Übereinstimmung
der Teile miteinander
und mit dem Ganzen.”
“Beauty is the proper conformity
of the parts to one another
and to the whole.”
|
Comments Off on Friday September 26, 2008
Thursday, August 28, 2008
Associations
for the writer
known as UD
"Have liberty not as
the air within a grave
Or down a well. Breathe freedom,
oh, my native,
In the space of horizons
that neither love nor hate."
— Wallace Stevens,
"Things of August"
|
A related visual
association of ideas —
("The association is the idea"
— Ian Lee, The Third Word War)
From UD Jewelry:
|
by John Braheny
"Hook" is the term you'll hear most often in the business and craft of commercial songwriting. (Well, maybe not as much as "Sorry, we can't use your song," but it's possible that the more you hear about hooks now, the less you'll hear "we can't use it" later.)
The hook has been described as "the part(s) you remember after the song is over," "the part that reaches out and grabs you," "the part you can't stop singing (even when you hate it)" and "the catchy repeated chorus…."
|
See also UD's recent
A Must-Read and In My Day*
as well as the five
Log24 entries ending
Sept. 20, 2002.
More seriously:
Stevens's "space of horizons" may, if one likes, be interpreted as a reference to
projective geometry. Despite the bleak physicist's view of mathematics quoted above, this discipline is– thanks to
Blaise Pascal— not totally lacking in literary and spiritual
associations.
* Hey Hey
Comments Off on Thursday August 28, 2008
Friday, August 22, 2008
Tentative movie title:
Blockheads
The Kohs Block Design
Intelligence Test
Samuel Calmin Kohs, the designer (but not the originator) of the above intelligence test, would likely disapprove of the "Aryan Youth types" mentioned in passing by a film reviewer in today's New York Times. (See below.) The Aryan Youth would also likely disapprove of Dr. Kohs.
Other related material:
1. Wechsler Cubes (intelligence testing cubes derived from the Kohs cubes shown above). See…
Harvard psychiatry and…
The Montessori Method;
The Crimson Passion;
The Lottery Covenant.
2. Wechsler Cubes of a different sort (Log24, May 25, 2008)
3. Manohla Dargis in today's New York Times:
"… 'Momma’s Man' is a touchingly true film, part weepie, part comedy, about the agonies of navigating that slippery slope called adulthood. It was written and directed by Azazel Jacobs, a native New Yorker who has set his modestly scaled movie with a heart the size of the Ritz in the same downtown warren where he was raised. Being a child of the avant-garde as well as an A student, he cast his parents, the filmmaker Ken Jacobs and the artist Flo Jacobs, as the puzzled progenitors of his centerpiece, a wayward son of bohemia….
In American movies, growing up tends to be a job for either Aryan Youth types or the oddballs and outsiders…."
4. The bohemian who named his son
Azazel:
"… I think that the deeper opportunity, the greater opportunity film can offer us is as an exercise of the mind. But an exercise, I hate to use the word, I won't say 'soul,' I won't say 'soul' and I won't say 'spirit,' but that it can really put our deepest psychological existence through stuff. It can be a powerful exercise. It can make us think, but I don't mean think about this and think about that. The very, very process of powerful thinking, in a way that it can afford, is I think very, very valuable. I basically think that the mind is not complete yet, that we are working on creating the mind. Okay. And that the higher function of art for me is its contribution to the making of mind."
— Interview with Ken Jacobs, UC Berkeley, October 1999
5. For Dargis's "Aryan Youth types"–
Comments Off on Friday August 22, 2008
Tuesday, August 19, 2008
Three Times
"Credences of Summer," VII,
by Wallace Stevens, from
Transport to Summer (1947)
"Three times the concentred
self takes hold, three times
The thrice concentred self,
having possessed
The object, grips it
in savage scrutiny,
Once to make captive,
once to subjugate
Or yield to subjugation,
once to proclaim
The meaning of the capture,
this hard prize,
Fully made, fully apparent,
fully found." |
Stevens does not say what object he is discussing.
One possibility —
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in a recent New Yorker:
"A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Another possibility —
A more modest object —
the 4×4 square.
Update of Aug. 20-21 —
Symmetries and Facets
Kostant's poetic comparison might be applied also to this object.
The natural rearrangements (symmetries) of the 4×4 array might also be described poetically as "thousands of facets, each facet offering a different view of… internal structure."
More precisely, there are 322,560 natural rearrangements– which a poet might call facets*— of the array, each offering a different view of the array's internal structure– encoded as a unique ordered pair of symmetric graphic designs. The symmetry of the array's internal structure is reflected in the symmetry of the graphic designs. For examples, see the Diamond 16 Puzzle.
For an instance of Stevens's "three times" process, see the three parts of the 2004 web page Ideas and Art.
* For the metaphor of rearrangements as facets, note that each symmetry (rearrangement) of a Platonic solid corresponds to a rotated facet: the number of symmetries equals the number of facets times the number of rotations (edges) of each facet–
The metaphor of rearrangements as facets breaks down, however, when we try to use it to compute, as above with the Platonic solids, the
number of natural rearrangements, or symmetries, of the 4×4 array. Actually, the true analogy is between the 16 unit squares of the 4×4 array, regarded as the 16 points of a finite 4-space (which has finitely many symmetries), and the infinitely many points of Euclidean 4-space (which has infinitely many symmetries).
If Greek geometers had started with a finite space (as in The Eightfold Cube), the history of mathematics might have dramatically illustrated Halmos's saying (Aug. 16) that
"The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question."
The Greeks, of course, answered the infinite questions first– at least for Euclidean space. Halmos was concerned with more general modern infinite spaces (such as Hilbert space) where the intuition to be gained from finite questions is still of value.
Comments Off on Tuesday August 19, 2008
Monday, August 18, 2008
Lotteries on
August 17,
2008 |
Pennsylvania
(No revelation) |
New York
(Revelation) |
Mid-day
(No belief) |
No belief,
no revelation
492
Chinese
Magic
Square:
4 9 2
3 5 7
8 1 6
(See below.)
|
Revelation
without belief
423
4/23:
Upscale
Realism:
Triangles
in Toronto
|
Evening
(Belief) |
Belief without
revelation
272
Rahner
on Grace
(See below.) |
Belief and
revelation
406
4/06:
Ideas
and Art
|
No belief, no revelation:
An encounter with “492”–
“What is combinatorial mathematics? Combinatorial mathematics, also referred to as combinatorial analysis or combinatorics, is a mathematical discipline that began in ancient times. According to legend the Chinese Emperor Yu (c. 2200 B.C.) observed the magic square
4 9 2
3 5 7
8 1 6
on the shell of a divine turtle….”
— H.J. Ryser, Combinatorial Mathematics, Mathematical Association of America, Carus Mathematical Monographs 14 (1963) |
Belief without revelation:
Theology and human experience,
and the experience of “272”–
From Christian Tradition Today,
by Jeffrey C. K. Goh
(Peeters Publishers, 2004), p. 438:
“Insisting that theological statements are not simply deduced from human experience, Rahner nevertheless stresses the experience of grace as the ‘real, fundamental reality of Christianity itself.’ 272
272 ‘Grace’ is a key category in Rahner’s theology. He has expended a great deal of energy on this topic, earning himself the title, amongst others, of a ‘theologian of the graced search for meaning.’ See G. B. Kelly (ed.), Karl Rahner, in The Making of Modern Theology series (Edinburgh: T&T Clark, 1992).” |
Comments Off on Monday August 18, 2008
Saturday, August 16, 2008
Seeing the Finite Structure
The following supplies some context for remarks of Halmos on combinatorics.
From Paul Halmos: Celebrating 50 years of Mathematics, by John H. Ewing, Paul Richard Halmos, Frederick W. Gehring, published by Springer, 1991–
Interviews with Halmos, “Paul Halmos by Parts,” by Donald J. Albers–
“Part II: In Touch with God*“– on pp. 27-28:
The Root of All Deep Mathematics
“Albers. In the conclusion of ‘Fifty Years of Linear Algebra,’ you wrote: ‘I am inclined to believe that at the root of all deep mathematics there is a combinatorial insight… I think that in this subject (in every subject?) the really original, really deep insights are always combinatorial, and I think for the new discoveries that we need– the pendulum needs– to swing back, and will swing back in the combinatorial direction.’ I always thought of you as an analyst.
Halmos: People call me an analyst, but I think I’m a born algebraist, and I mean the same thing, analytic versus combinatorial-algebraic. I think the finite case illustrates and guides and simplifies the infinite.
Some people called me full of baloney when I asserted that the deep problems of operator theory could all be solved if we knew the answer to every finite dimensional matrix question. I still have this religion that if you knew the answer to every matrix question, somehow you could answer every operator question. But the ‘somehow’ would require genius. The problem is not, given an operator question, to ask the same question in finite dimensions– that’s silly. The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question.
Combinatorics, the finite case, is where the genuine, deep insight is. Generalizing, making it infinite, is sometimes intricate and sometimes difficult, and I might even be willing to say that it’s sometimes deep, but it is nowhere near as fundamental as seeing the finite structure.”
Finite Structure
on a Book Cover:
Walsh Series: An Introduction
to Dyadic Harmonic Analysis,
by F. Schipp
et al.,
Taylor & Francis, 1990
Halmos’s above remarks on combinatorics as a source of “deep mathematics” were in the context of operator theory. For connections between operator theory and harmonic analysis, see (for instance) H.S. Shapiro, “Operator Theory and Harmonic Analysis,” pp. 31-56 in
Twentieth Century Harmonic Analysis– A Celebration, ed. by J.S. Byrnes, published by Springer, 2001.
Walsh Series states that Walsh functions provide “the simplest non-trivial model for harmonic analysis.”
The patterns on the faces of the cube on the cover of
Walsh Series above illustrate both the
Walsh functions of order 3 and the same structure in a different guise,
subspaces of the affine 3-space over the binary field. For a note on the relationship of Walsh functions to finite geometry, see
Symmetry of Walsh Functions.
Whether the above sketch of the passage from operator theory to harmonic analysis to Walsh functions to finite geometry can ever help find “the right finite question to ask,” I do not know. It at least suggests that finite geometry (and my own work on models in finite geometry) may not be completely irrelevant to mathematics generally regarded as more deep.
* See the Log24 entries following Halmos’s death.
Comments Off on Saturday August 16, 2008
Thursday, August 14, 2008
Magister Ludi(
The Glass Bead Game)
is now available for
download in pdf or
text format at
Scribd.
“How far back the historian wishes to place the origins and antecedents of the Glass Bead Game is, ultimately, a matter of his personal choice. For like every great idea it has no real beginning; rather, it has always been, at least the idea of it. We find it foreshadowed, as a dim anticipation and hope, in a good many earlier ages. There are hints of it in Pythagoras, for example, and then among Hellenistic Gnostic circles in the late period of classical civilization. We find it equally among the ancient Chinese, then again at the several pinnacles of Arabic-Moorish culture; and the path of its prehistory leads on through Scholasticism and Humanism to the academies of mathematicians of the seventeenth and eighteenth centuries and on to the Romantic philosophies and the runes of Novalis’s hallucinatory visions. This same eternal idea, which for us has been embodied in the Glass Bead Game, has underlain every movement of Mind toward the ideal goal of a
universitas litterarum, every Platonic academy, every league of an intellectual elite, every
rapprochement between the exact and the more liberal disciplines, every effort toward reconciliation between science and art or science and religion. Men like Abelard, Leibniz, and Hegel unquestionably were familiar with the dream of capturing the universe of the intellect in concentric systems, and pairing the living beauty of thought and art with the magical expressiveness of the exact sciences. In that age in which music and mathematics almost simultaneously attained classical heights, approaches and cross-fertilizations between the two disciplines occurred frequently.”
— Hermann Hesse
Author’s dedication:
to the Journeyers
to the East
Related material:
Ring Theory
Comments Off on Thursday August 14, 2008
Monday, August 11, 2008
Comments Off on Monday August 11, 2008
Sunday, August 10, 2008
“Duration is… not a state of rest, for mere standstill is regression. Duration is rather the self-contained and therefore self-renewing movement of an organized, firmly integrated whole [click on link for an example], taking place in accordance with immutable laws and beginning anew at every ending.”
Globe at the
Beijing 2008 Olympics
Opening Ceremony
The eight trigrams
were perhaps implied in
the opening’s date,
8/8/8.
Comments Off on Sunday August 10, 2008
Friday, August 8, 2008
Click on image for details.
Comments Off on Friday August 8, 2008
Sunday, August 3, 2008
Kindergarten
Geometry
Preview of a Tom Stoppard play presented at Town Hall in Manhattan on March 14, 2008 (Pi Day and Einstein's birthday):
The play's title, "Every Good Boy Deserves Favour," is a mnemonic for the notes of the treble clef EGBDF.
The place, Town Hall, West 43rd Street. The time, 8 p.m., Friday, March 14. One single performance only, to the tinkle– or the clang?– of a triangle. Echoing perhaps the clang-clack of Warsaw Pact tanks muscling into Prague in August 1968.
The “u” in favour is the British way, the Stoppard way, "EGBDF" being "a Play for Actors and Orchestra" by Tom Stoppard (words) and André Previn (music).
And what a play!– as luminescent as always where Stoppard is concerned. The music component of the one-nighter at Town Hall– a showcase for the Boston University College of Fine Arts– is by a 47-piece live orchestra, the significant instrument being, well, a triangle.
When, in 1974, André Previn, then principal conductor of the London Symphony, invited Stoppard "to write something which had the need of a live full-time orchestra onstage," the 36-year-old playwright jumped at the chance.
One hitch: Stoppard at the time knew "very little about 'serious' music… My qualifications for writing about an orchestra," he says in his introduction to the 1978 Grove Press edition of "EGBDF," "amounted to a spell as a triangle player in a kindergarten percussion band."
— Jerry Tallmer in The Villager, March 12-18, 2008
Review of the same play as presented at Chautauqua Institution on July 24, 2008:
"Stoppard's modus operandi– to teasingly introduce numerous clever tidbits designed to challenge the audience."
— Jane Vranish, Pittsburgh Post-Gazette, Saturday, August 2, 2008
"The leader of the band is tired
And his eyes are growing old
But his blood runs through
My instrument
And his song is in my soul."
— Dan Fogelberg
"He's watching us all the time."
— Lucia Joyce
Finnegans Wake,
Book II, Episode 2, pp. 296-297:
I'll make you to see figuratleavely the whome of your eternal geomater. And if you flung her headdress on her from under her highlows you'd wheeze whyse Salmonson set his seel on a hexengown.1 Hissss!, Arrah, go on! Fin for fun!
1 The chape of Doña Speranza of the Nacion.
|
Reciprocity
From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity….
… E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies."
— William Boyd, review of Himmelfarb, a novel by Michael Kruger, in The New York Times Book Review, October 30, 1994
Last year's entry on this date:
The picture above is of the complete graph K6 … Six points with an edge connecting every pair of points… Fifteen edges in all.
Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24.
If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See
Reciprocity and Reversal in Lao Tzu.
For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in
Shu: Reciprocity.
Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the K6 graph above, expresses the reciprocity of male and female, as my May 2003 archives illustrate. The star of David also appears as part of a graphic design for cubes that illustrate the concepts of diamond theory:
Click on the design for details.
Those who prefer a Jewish approach to physics can find the star of David, in the form of K6, applied to the sixteen 4×4 Dirac matrices, in
A Graphical Representation
of the Dirac Algebra.
The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See
The Jewel of Arithmetic and
|
FinnegansWiki:
Salmonson set his seel:
"Finn MacCool ate the Salmon of Knowledge."
Wikipedia:
"George Salmon spent his boyhood in Cork City, Ireland. His father was a linen merchant. He graduated from Trinity College Dublin at the age of 19 with exceptionally high honours in mathematics. In 1841 at age 21 he was appointed to a position in the mathematics department at Trinity College Dublin. In 1845 he was appointed concurrently to a position in the theology department at Trinity College Dublin, having been confirmed in that year as an Anglican priest."
Comments Off on Sunday August 3, 2008
Saturday, August 2, 2008
Comments Off on Saturday August 2, 2008
Prattle
“Simon studies one of the most complicated groups of all: the Monster. He is, still, the world expert on it ….
Simon tells me he has a quasi-religious faith in the Monster. One day, he says, … the Monster will expose the structure of the universe.
… although Simon says he is keen for me to write a book about him and his work on the Monster and his obsession with buses, he doesn’t like talking, has no sense of anecdotes or extended conversation, and can’t remember (or never paid any attention to) 90 per cent of the things I want him to tell me about in his past. It is not modesty. Simon is not modest or immodest: he just has no self-curiosity. To Simon, Simon is a collection of disparate facts and no interpretative glue. He is a man without adjectives. His speech is made up almost entirely of short bursts of grunts and nouns.
This is the main reason why we spent three weeks together …. I needed to find a way to make him prattle.”
Those in search of prattle and interpretive glue should consult Anthony Judge’s essay ““Potential Psychosocial Significance of Monstrous Moonshine: An Exceptional Form of Symmetry as a Rosetta Stone for Cognitive Frameworks.” This was cited here in Thursday’s entry “Symmetry in Review.” (That entry is just a list of items related in part by synchronicity, in part by mathematical content. The list, while meaningful to me and perhaps a few others, is also lacking in prattle and interpretive glue.)
Those in search of knowledge, rather than glue and prattle, should consult Symmetry and the Monster, by Mark Ronan. If they have a good undergraduate education in mathematics, Terry Gannon‘s survey paper “Monstrous Moonshine: The First Twenty-Five Years” (pdf) and book– Moonshine Beyond the Monster— may also be of interest.
Comments Off on Saturday August 2, 2008
Thursday, July 31, 2008
Symmetry in Review
“Put bluntly, who is kidding whom?”
— Anthony Judge, draft of
“Potential Psychosocial Significance
of Monstrous Moonshine:
An Exceptional Form of Symmetry
as a Rosetta Stone for
Cognitive Frameworks,”
dated September 6, 2007.
Good question.
Also from
September 6, 2007 —
the date of
Madeleine L’Engle‘s death —
Related material:
1. The performance of a work by
Richard Strauss,
“Death and Transfiguration,”
(Tod und Verklärung, Opus 24)
by the Chautauqua Symphony
at Chautauqua Institution on
July 24, 2008
2. Headline of a music review
in today’s New York Times:
Welcoming a Fresh Season of
Transformation and Death
3. The picture of the R. T. Curtis
Miracle Octad Generator
on the cover of the book
Twelve Sporadic Groups:
4. Freeman Dyson’s hope, quoted by
Gorenstein in 1986, Ronan in 2006,
and Judge in 2007, that the Monster
group is “built in some way into
the structure of the universe.”
5. Symmetry from Plato to
the Four-Color Conjecture
6. Geometry of the 4×4 Square
7. Yesterday’s entry,
“Theories of Everything“
Coda:
“There is such a thing
as a tesseract.“
— Madeleine L’Engle
For a profile of
L’Engle, click on
the Easter eggs.
Comments Off on Thursday July 31, 2008
Wednesday, July 30, 2008
Like
Garrett Lisi’s, it is based on an unusual and highly symmetric mathematical structure. Lisi’s approach is related to the exceptional simple Lie group E
8.* Dharwadker uses
a structure long associated with the sporadic simple Mathieu group M
24.
| GRAND UNIFICATION
OF THE STANDARD MODEL WITH QUANTUM GRAVITY
by Ashay Dharwadker
Abstract
“We show that the mathematical proof of the four colour theorem [1] directly implies the existence of the standard model, together with quantum gravity, in its physical interpretation. Conversely, the experimentally observable standard model and quantum gravity show that nature applies the mathematical proof of the four colour theorem, at the most fundamental level. We preserve all the established working theories of physics: Quantum Mechanics, Special and General Relativity, Quantum Electrodynamics (QED), the Electroweak model and Quantum Chromodynamics (QCD). We build upon these theories, unifying all of them with Einstein’s law of gravity. Quantum gravity is a direct and unavoidable consequence of the theory. The main construction of the Steiner system in the proof of the four colour theorem already defines the gravitational fields of all the particles of the standard model. Our first goal is to construct all the particles constituting the classic standard model, in exact agreement with t’Hooft’s table [8]. We are able to predict the exact mass of the Higgs particle and the CP violation and mixing angle of weak interactions. Our second goal is to construct the gauge groups and explicitly calculate the gauge coupling constants of the force fields. We show how the gauge groups are embedded in a sequence along the cosmological timeline in the grand unification. Finally, we calculate the mass ratios of the particles of the standard model. Thus, the mathematical proof of the four colour theorem shows that the grand unification of the standard model with quantum gravity is complete, and rules out the possibility of finding any other kinds of particles.” |
* See, for instance, “The Scientific Promise of Perfect Symmetry” in The New York Times of March 20, 2007.
Comments Off on Wednesday July 30, 2008
Friday, July 25, 2008
56 Triangles
"This wonderful picture was drawn by Greg Egan with the help of ideas from Mike Stay and Gerard Westendorp. It's probably the best way for a nonmathematician to appreciate the symmetry of Klein's quartic. It's a 3-holed torus, but drawn in a way that emphasizes the tetrahedral symmetry lurking in this surface! You can see there are 56 triangles: 2 for each of the tetrahedron's 4 corners, and 8 for each of its 6 edges."
Exercise:
Click on image for further details.
Note that if eight points are arranged
in a cube (like the centers of the
eight subcubes in the figure above),
there are 56 triangles formed by
the 8 points taken 3 at a time.
Baez's discussion says that the Klein quartic's 56 triangles can be partitioned into 7 eight-triangle Egan "cubes" that correspond to the 7 points of the Fano plane in such a way that automorphisms of the Klein quartic correspond to automorphisms of the Fano plane. Show that the 56 triangles within the eightfold cube can also be partitioned into 7 eight-triangle sets that correspond to the 7 points of the Fano plane in such a way that (affine) transformations of the eightfold cube induce (projective) automorphisms of the Fano plane.
Comments Off on Friday July 25, 2008
Thursday, July 24, 2008
Tried out the new knol.google.com site
with a copy of The Diamond Theorem.
Comments Off on Thursday July 24, 2008
« Newer Posts —
Older Posts »
