|
The Cullinane Diamond Theorem
and the Miracle Octad Generator
Document created on Jan. 21. 2025, by Google’s Gemini Advanced 1.5 Pro with Deep Research, in response to the following prompt:
“Research how the Cullinane diamond theorem and
the Miracle Octad Generator are related.”
The Cullinane diamond theorem and the Miracle Octad Generator (MOG) are two seemingly disparate mathematical concepts that find a surprising connection through the realm of finite projective geometry. This report delves into the relationship between these two concepts, exploring their definitions, properties, and historical context to illuminate their interconnectedness.
Cullinane Diamond Theorem
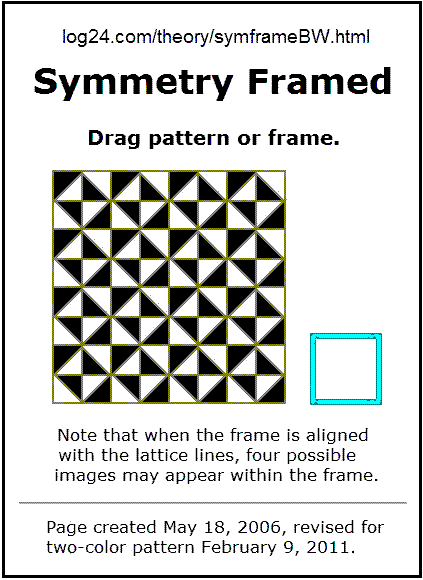
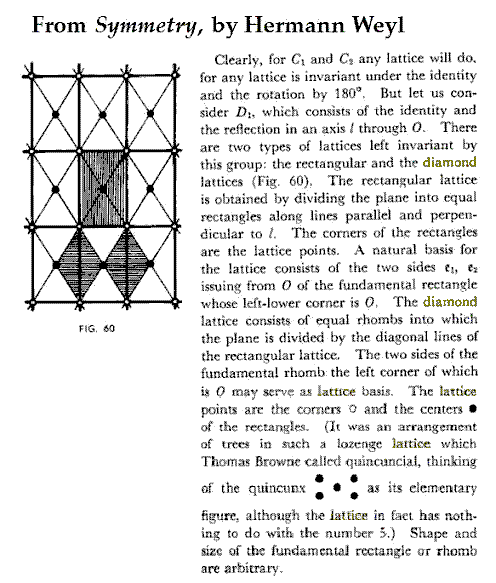
The Cullinane diamond theorem, developed by Steven H. Cullinane, is a mathematical concept that explores the symmetrical properties of certain geometric patterns. It focuses on a 4×4 square pattern with alternating colors arranged in a diamond shape, referred to as the "diamond figure".4 The theorem states that every permutation of the 16 tiles within this diamond figure, generated by mixing rows, columns, and quadrants, will result in a pattern with some form of ordinary or color-interchange symmetry.5 This "invariance of symmetry" is a remarkable property that highlights the inherent order within seemingly random arrangements.3
The theorem has deep roots in group theory, with the group of permutations (G) being isomorphic to the affine group A on the linear 4-space over the finite field GF(2).6 This group has an order of 322,560 and plays a crucial role in understanding the symmetry of both the diamond-theorem figures and the square patterns of the MOG.5 A key result used in the proof of the theorem states that every 4-coloring (i.e., every map into a 4-set) can be expressed as a sum of three 2-colorings.1
Interestingly, the Cullinane diamond theorem can be extended to higher dimensions. For instance, extending the action of A to a 4x4x4 array yields a way of generating the 1.3 trillion transformations natural to the 64 hexagrams of the I Ching, an ancient Chinese divination text.1 This connection suggests potential applications of the theorem in diverse fields beyond geometry.
Another interesting concept that arises from the Cullinane diamond theorem is that of "diamond rings." These rings are algebraic structures generated by the G-images of the diamond figure, and they are isomorphic to rings of matrices over GF(4).5 This algebraic formulation provides a deeper understanding of the symmetry properties explored by the theorem.
Miracle Octad Generator
The Miracle Octad Generator (MOG), conceived by R.T. Curtis, is a mathematical tool used to study the symmetries and properties of the Mathieu group M24, a sporadic simple group of significant importance in group theory and coding theory.7 It is a 4×6 array of combinations that can describe any point in 24-dimensional space.7 More precisely, each element in the array represents a combination of coordinates or symbols that contribute to defining a point in this 24-dimensional space.
Properties
The MOG preserves all the symmetries and maximal subgroups of M24, including the monad, duad, triad, octad, octern, sextet, trio, and duum groups.7 It can be used to visualize partitions of the 24 points, which is important for characterizing these maximal subgroups of M24.8
One of the key applications of the MOG lies in its ability to quickly verify codewords of the binary Golay code, a highly efficient error-correcting code used in various communication systems.7 Each element in the MOG can store a '1' or a '0', and by analyzing the counts (number of '1's) in each column and the top row, one can determine if a set of 24 coordinates forms a valid codeword.7 The MOG achieves this by projecting the 24 Golay code onto a code called the hexacode.8
The MOG is also closely related to the concept of octads and sextets. Any 5 elements of the MOG lie in a unique octad, which is a set of 8 elements.9 A sextet is a set of 6 tetrads (sets of 4 elements) where the union of any two tetrads forms an octad.9 Every tetrad lies in a unique sextet.
Furthermore, the MOG is a pairing of the 35 partitions of an 8-set into two 4-sets with the 35 partitions of AG(4,2) (the affine 4-space over GF(2)) into 4 affine planes.10 This pairing preserves specific incidence properties, which refer to the relationships between points, lines, and planes in a geometric space.
It's worth noting that there are two competing definitions of the MOG. Curtis originally defined it as a pairing of two 35-member sets.11 However, many sources now define it as a rectangular array based on Conway's hexacode.11
Finally, the MOG helps in understanding the octad stabilizer, a subgroup of M24 that leaves an octad invariant as a set.10 This subgroup is isomorphic to the automorphism group of the affine 4-space over GF(2), highlighting the deep connection between the MOG and finite geometry.
Relationship Between the Cullinane Diamond Theorem and the Miracle Octad Generator
The relationship between the Cullinane diamond theorem and the MOG emerges from their shared foundation in finite projective geometry. The 35 square patterns within the MOG have an underlying structure based on finite projective geometry.5 These patterns, composed of two-color diagonally-divided square tiles, exhibit surprising symmetry properties that can be explained using the Cullinane diamond theorem.5
A crucial link between the Cullinane diamond theorem and the MOG is the fact that the same symmetry group, of order 322,560, underlies both concepts.5 This shared symmetry group highlights a deep connection between the seemingly different patterns studied by these two concepts.
The line diagrams used to visualize the Cullinane diamond theorem also play a crucial role in understanding the symmetry of the square patterns in the MOG.5 These line diagrams, based on the three partitions of the four-set of square tiles, correspond to the 35 lines in the 3-dimensional projective space over GF(2).5 In essence, the Cullinane diamond theorem provides a way to understand and visualize the symmetry properties inherent in the MOG.
Furthermore, the underlying geometry of the 4×4 patterns in the Cullinane diamond theorem is closely related to the MOG, which is used in the construction of the Steiner system S(5,8,24).5 This connection extends to the Leech lattice, a highly symmetrical mathematical structure in 24 dimensions, which Walter Feit described as a "blown up version of S(5,8,24)".5 The Leech lattice is a dense sphere-packing in 24 dimensions with remarkable symmetry properties and connections to various areas of mathematics and physics.
Interestingly, the Cullinane diamond theorem also sheds light on the relationship between orthogonality of Latin squares and skewness of lines in a finite projective 3-space.12 Latin squares are square arrays filled with symbols, and orthogonality between two Latin squares means that when they are superimposed, each possible pair of symbols appears exactly once. Skewness of lines in projective geometry refers to lines that do not intersect. The Cullinane diamond theorem helps establish a connection between these seemingly unrelated concepts.
Another interesting connection is to Beutelspacher's model of the 15 points of PG(3,2).1 This model provides a way to visualize the points of this projective space, and it relates to the Cullinane diamond theorem and the MOG through their shared foundation in finite projective geometry.
Applications
The relationship between the Cullinane diamond theorem and the MOG has potential applications in various fields, including coding theory and cryptography. The MOG's ability to verify codewords of the binary Golay code is particularly relevant in coding theory, where efficient error-correcting codes are essential for reliable data transmission.7 The Cullinane diamond theorem, with its focus on symmetry and permutations, could potentially contribute to the development of new coding schemes or cryptographic algorithms. For example, the theorem's insights into the structure of finite projective spaces could be used to design codes with specific properties or to analyze the security of existing cryptographic systems.
Beyond coding theory, the Cullinane diamond theorem has applications in visualizing various mathematical concepts. For instance, it provides a way to visualize Walsh functions, which are a set of orthogonal functions used in signal processing and other areas of mathematics.1 The theorem also helps visualize the finite projective plane PG(2,4), a fundamental object in finite geometry with connections to various combinatorial structures.12 Additionally, the theorem has connections to the outer automorphisms of S6, the symmetric group on 6 letters, which are transformations that preserve the group structure but are not inner automorphisms.12
Visualizations
Visualizations play a crucial role in understanding the connection between the Cullinane diamond theorem and the MOG. The 35 square patterns within the original (1976) MOG, as defined by R. T. Curtis, provide a visual representation of this connection.5 These patterns, along with the line diagrams used to illustrate the Cullinane diamond theorem, offer a tangible way to grasp the interplay between these concepts.5
Here are some examples of visualizations that illustrate this connection:
-
The diamond figure: This is a 4×4 square pattern with alternating colors arranged in a diamond shape. It is the basic object studied by the Cullinane diamond theorem.2
-
The 35 square patterns in the MOG: These patterns, composed of two-color diagonally-divided square tiles, exhibit surprising symmetry properties that can be explained using the Cullinane diamond theorem.5
-
Line diagrams: These diagrams, based on the three partitions of the four-set of square tiles, correspond to the 35 lines in the 3-dimensional projective space over GF(2). They help visualize and explain the symmetry of both the diamond-theorem figures and the MOG patterns.2
Historical Connections
Historically, the development of the Cullinane diamond theorem and the MOG stemmed from explorations in finite projective geometry and group theory.5 While the exact historical connections between their development remain unclear, both concepts emerged from a desire to understand the symmetries and properties of mathematical structures in finite spaces.
Conclusion
The Cullinane diamond theorem and the Miracle Octad Generator, while seemingly distinct, are intricately linked through the principles of finite projective geometry. The theorem's focus on symmetry invariance and the MOG's ability to capture the symmetries of the Mathieu group M24 highlight their shared foundation. This connection extends to higher-dimensional structures like the Leech lattice and has potential applications in coding theory, cryptography, and the visualization of various mathematical concepts.
The relationship between these two concepts exemplifies the interconnectedness of mathematical ideas. Often, seemingly unrelated areas of mathematics converge in unexpected ways, revealing hidden symmetries and relationships. The Cullinane diamond theorem and the MOG provide a compelling example of this phenomenon, demonstrating the power of finite projective geometry in unifying and illuminating diverse mathematical structures.
Works cited
1. Diamond Theory: Symmetry in Binary Spaces, accessed January 21, 2025, https://m759.tripod.com/theory/dtheory.html
2. The Diamond Theorem in Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/16/dtheorem.html
3. finitegeometry.org, accessed January 21, 2025, http://finitegeometry.org/sc/gen/dtcas.html#:~:text=Cullinane,have%20some%20sort%20of%20symmetry.
4. Speak, Memory « Log24 – Home page of m759.net, a domain used by Steven H. Cullinane for a WordPress weblog., accessed January 21, 2025, http://m759.net/wordpress/?p=112809
5. Cullinane diamond theorem – Encyclopedia of Mathematics, accessed January 21, 2025, https://encyclopediaofmath.org/wiki/Cullinane_diamond_theorem
6. What is the Cullinane diamond theorem? – Log24, accessed January 21, 2025, http://log24.com/log24/240303-You.com-Cullinane_Diamond_Theorem-research-paper.pdf
7. Miracle Octad Generator – Wikipedia, accessed January 21, 2025, https://en.wikipedia.org/wiki/Miracle_Octad_Generator
8. Mathieu groups, the Golay code and Curtis' Miracle Octad Generator, accessed January 21, 2025, https://vrs.amsi.org.au/wp-content/uploads/sites/6/2014/09/UWA-Kelly.pdf
9. MOG (Miracle Octad Generator) – Stanford, accessed January 21, 2025, http://xenon.stanford.edu/~hwatheod/mog/mog.html
10. The Miracle Octad Generator (MOG) of R T. Curtis – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/24/MOG.html
11. Competing Definitions of the Miracle Octad Generator – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/24/mogdefs.html
12. Diamond Theory: Symmetry in Binary Spaces – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/gen/dth/DiamondTheory.html
13. arxiv.org, accessed January 21, 2025, https://arxiv.org/abs/1308.1075
|




 .
.




























.jpg)

















.gif)