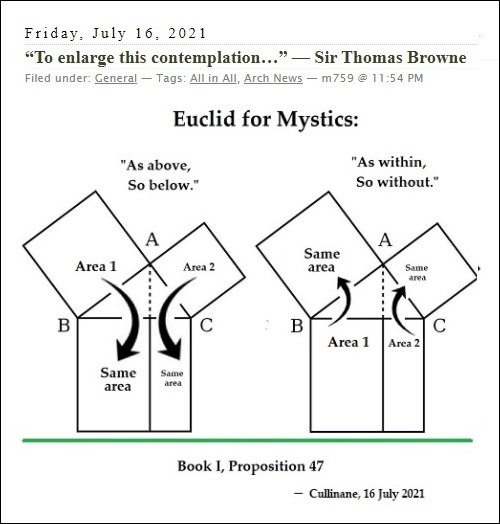
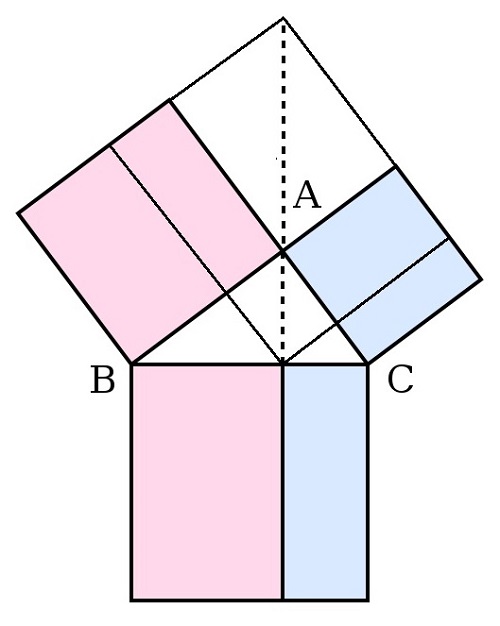
(A sequel to this morning's post A Subtle Knife for Sean.)
Exhibit A —
Einstein in The Saturday Review, 1949 —
"In any case it was quite sufficient for me
if I could peg proofs upon propositions
the validity of which did not seem to me to be dubious.
For example, I remember that an uncle told me
the Pythagorean theorem before the holy geometry booklet
had come into my hands. After much effort I succeeded
in 'proving' this theorem on the basis of the similarity
of triangles; in doing so it seemed to me 'evident' that
the relations of the sides of the right-angled triangles
would have to be completely determined by one of the
acute angles. Only something which did not in similar fashion
seem to be 'evident' appeared to me to be in need of any proof
at all. Also, the objects with which geometry deals seemed to
be of no different type than the objects of sensory perception,
'which can be seen and touched.' This primitive idea, which
probably also lies at the bottom of the well-known Kantian
problematic concerning the possibility of 'synthetic judgments
a priori' rests obviously upon the fact that the relation of
geometrical concepts to objects of direct experience
(rigid rod, finite interval, etc.) was unconsciously present."
Exhibit B —
Strogatz in The New Yorker, 2015 —
"Einstein, unfortunately, left no … record of his childhood proof.
In his Saturday Review essay, he described it in general terms,
mentioning only that it relied on 'the similarity of triangles.'
The consensus among Einstein’s biographers is that he probably
discovered, on his own, a standard textbook proof in which similar
triangles (meaning triangles that are like photographic reductions
or enlargements of one another) do indeed play a starring role.
Walter Isaacson, Jeremy Bernstein, and Banesh Hoffman all come
to this deflating conclusion, and each of them describes the steps
that Einstein would have followed as he unwittingly reinvented
a well-known proof."
Exhibit C —
Schroeder in a book, 1991 —

Schroeder presents an elegant and memorable proof. He attributes
the proof to Einstein, citing purely hearsay evidence in a footnote.
The only other evidence for Einstein's connection with the proof
is his 1949 Saturday Review remarks. If Einstein did come up with
the proof at age 11 and discuss it with others later, as Schroeder
claims, it seems he might have felt a certain pride and been more
specific in 1949, instead of merely mentioning the theorem in passing
before he discussed Kantian philosophy relating concepts to objects.
Strogatz says that . . .
"What we’re seeing here is a quintessential use of
a symmetry argument… scaling….
Throughout his career, Einstein would continue to
deploy symmetry arguments like a scalpel, getting to
the hidden heart of things."
Connoisseurs of bullshit may prefer a faux-Chinese approach to
"the hidden heart of things." See Log24 on August 16, 2021 —
http://m759.net/wordpress/?p=96023 —
In a Nutshell: The Core of Everything .