"Before time began, there was the Cube."
— Optimus Prime
"Spiel ist nicht Spielerei."
— Friedrich Froebel
Friday, February 2, 2024
Friday, March 23, 2018
From the Personal to the Platonic
On the Oslo artist Josefine Lyche —
"Josefine has taken me through beautiful stories,
ranging from the personal to the platonic
explaining the extensive use of geometry in her art.
I now know that she bursts into laughter when reading
Dostoyevsky, and that she has a weird connection
with a retired mathematician."
— Ann Cathrin Andersen,
http://bryggmagasin.no/2017/behind-the-glitter/
Personal —
The Rushkoff Logo
— From a 2016 graphic novel by Douglas Rushkoff.
See also Rushkoff and Talisman in this journal.
Platonic —
Compare and contrast the shifting hexagon logo in the Rushkoff novel above
with the hexagon-inside-a-cube in my "Diamonds and Whirls" note (1984).
Comments Off on From the Personal to the Platonic
Thursday, December 18, 2014
Platonic Analogy
(Five by Five continued)
As the 3×3 grid underlies the order-3 finite projective plane,
whose 13 points may be modeled by
the 13 symmetry axes of the cube,
so the 5×5 grid underlies the order-5 finite projective plane,
whose 31 points may be modeled by
the 31 symmetry axes of the dodecahedron.
See posts tagged Galois-Plane Models.
Comments Off on Platonic Analogy
Sunday, August 3, 2014
Unplatonic Dialogue
Dialogue from “The Osterman Weekend”—
01:57:22 “Why did he make us try to believe Omega existed?”
01:57:25 ….
01:57:26 “The existence of Omega has not been disproved.
01:57:28 Don’t you understand that?
01:57:31 Omega is as real as we need it to be.”
See also Omega elsewhere in this journal.
Update of 9:15 PM ET —
Comments Off on Unplatonic Dialogue
Friday, September 22, 2023
Figurate Space
For the purpose of defining figurate geometry , a figurate space might be
loosely described as any space consisting of finitely many congruent figures —
subsets of Euclidean space such as points, line segments, squares,
triangles, hexagons, cubes, etc., — that are permuted by some finite group
acting upon them.
Thus each of the five Platonic solids constructed at the end of Euclid's Elements
is itself a figurate space, considered as a collection of figures — vertices, edges,
faces — seen in the nineteenth century as acted upon by a group of symmetries .
More recently, the 4×6 array of points (or, equivalently, square cells) in the Miracle
Octad Generator of R. T. Curtis is also a figurate space . The relevant group of
symmetries is the large Mathieu group M24 . That group may be viewed as acting
on various subsets of a 24-set… for instance, the 759 octads that are analogous
to the faces of a Platonic solid. The geometry of the 4×6 array was shown by
Curtis to be very helpful in describing these 759 octads.
Comments Off on Figurate Space
Thursday, July 13, 2023
Generative Preformed* Transformers
"Before time began . . ." — Optimus Prime
Structures from pure mathematics, by Plato and R. T. Curtis —
* See other "Preform" posts in this journal.
Comments Off on Generative Preformed* Transformers
Sunday, June 25, 2023
High Concept: The Dreaming Gemstones
Comments Off on High Concept: The Dreaming Gemstones
Sunday, January 22, 2023
Preform
Sometimes the word "preform" is not a misspelling.
"… there are present in every psyche forms which are unconscious
but nonetheless active — living dispositions, ideas in the Platonic sense,
that preform and continually influence our thoughts and feelings and actions."
The Source: Jung on a facultas praeformandi . . .
Illustration —
"A primordial image . . . .
the axial system of a crystal"
For those who prefer a Jewish approach to these matters —
(Post last updated at about 2:10 PM ET on Jan. 23, 2023.)
Comments Off on Preform
Tuesday, January 17, 2023
Annals of Scientific Theology
"Think of it as a cybernetic version of prayer…."
— Dennis Overbye in today's online New York Times ,
https://www.nytimes.com/2023/01/17/science/
cosmology-universe-programming.html .
Related remarks: The Log24 tag Geheimnis der Einheit, and . . .
Related art — "The Difference," a Log24 post of Epiphany 2010.
Comments Off on Annals of Scientific Theology
Monday, October 3, 2022
The Abstract and the Concrete
The above art by Steven H. Cullinane is not unrelated to
art by Josefine Lyche. Her work includes sculpted replicas
of the above abstract Platonic solids, as well as replicas of
my own work related to properties of the 4×6 rectangle above.
Symmetries of both the solids and the rectangle may be
viewed as permutations of parts — In the Platonic solids,
the parts are permuted by continuous rotations of space itself.
In the rectangle, the parts are permuted by non-continuous
transformations, as in the I Ching . . . i.e., by concrete illustrations
of change.
Comments Off on The Abstract and the Concrete
Wednesday, September 14, 2022
Thursday, December 16, 2021
Plato’s Retreat
"I've heard of affairs that are strictly Platonic…" — Song lyric
From a photo story by Marcela Nowak, Dec. 15, 2021 —
Asteras eisathreis, Aster emos.
Eithe genoimen ouranos,
‘os pollois ommasin eis se blepo.
Comments Off on Plato’s Retreat
Friday, June 25, 2021
Critical Space Theory, Devil’s Night 2016: Around Zero
Comments Off on Critical Space Theory, Devil’s Night 2016: Around Zero
Tuesday, September 1, 2020
Story Space
“The universe, then, is less intimation
than cipher: a mask rather than a revelation
in the romantic sense. Does love meet with love?
Do we receive but what we give? The answer is
surely a paradox, the paradox that there are
Platonic universals beyond, but that the glass
is too dark to see them. Is there a light beyond
the glass, or is it a mirror only to the self?
The Platonic cave is even darker than Plato
made it, for it introduces the echo, and so
leaves us back in the world of men, which does
not carry total meaning, is just a story of events.”
than cipher: a mask rather than a revelation
in the romantic sense. Does love meet with love?
Do we receive but what we give? The answer is
surely a paradox, the paradox that there are
Platonic universals beyond, but that the glass
is too dark to see them. Is there a light beyond
the glass, or is it a mirror only to the self?
The Platonic cave is even darker than Plato
made it, for it introduces the echo, and so
leaves us back in the world of men, which does
not carry total meaning, is just a story of events.”
– Betty Jay, reader’s guide to A Passage to India
Comments Off on Story Space
Friday, July 17, 2020
Poetic as Well as Prosaic
Prosaic —
Poetic —
Prosaic —
“These devices may have some
theoretical as well as practical value.“
Poetic —
Comments Off on Poetic as Well as Prosaic
Thursday, April 23, 2020
Octads and Geometry
See the web pages octad.group and octad.us.
Related geometry (not the 759 octads, but closely related to them) —
#/media/File:150614-PG-3-2-schoolgirls-arrangement.png)
The 4×6 rectangle of R. T. Curtis
illustrates the geometry of octads —
Curtis splits the 4×6 rectangle into three 4×2 "bricks" —
.
"In fact the construction enables us to describe the octads
in a very revealing manner. It shows that each octad,
other than Λ1, Λ2, Λ3, intersects at least one of these ' bricks' —
the 'heavy brick' – in just four points." . . . .
— R. T. Curtis (1976). "A new combinatorial approach to M24,"
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42.
Comments Off on Octads and Geometry
Saturday, January 4, 2020
Xmas Colors: Green to Red
Comments Off on Xmas Colors: Green to Red
Monday, December 23, 2019
Orbit
"December 22, the birth anniversary of India’s famed mathematician
Srinivasa Ramanujan, is celebrated as National Mathematics Day."
— Indian Express yesterday
"Orbits and stabilizers are closely related." — Wikipedia
Symmetries by Plato and R. T. Curtis —
In the above, 322,560 is the order
of the octad stabilizer group .
Comments Off on Orbit
Saturday, May 4, 2019
The Chinese Jars of Shing-Tung Yau
The title refers to Calabi-Yau spaces.
Four Quartets
. . . Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness.
A less "cosmic" but still noteworthy code — The Golay code.
This resides in a 12-dimensional space over GF(2).
Related material from Plato and R. T. Curtis —
A related Calabi-Yau "Chinese jar" first described in detail in 1905 —
A figure that may or may not be related to the 4x4x4 cube that
holds the classical Chinese "cosmic code" — the I Ching —
ftp://ftp.cs.indiana.edu/pub/hanson/forSha/AK3/old/K3-pix.pdf
Comments Off on The Chinese Jars of Shing-Tung Yau
Sunday, March 24, 2019
Espacement: Geometry of the Interstice in Literary Theory
"You said something about the significance of spaces between
elements being repeated. Not only the element itself being repeated,
but the space between. I'm very interested in the space between.
That is where we come together." — Peter Eisenman, 1982
|
https://www.parrhesiajournal.org/ Parrhesia No. 3 • 2007 • 22–32
(Up) Against the (In) Between: Interstitial Spatiality by Clare Blackburne Blackburne — www.parrhesiajournal.org 24 — "The excessive notion of espacement as the resurgent spatiality of that which is supposedly ‘without space’ (most notably, writing), alerts us to the highly dynamic nature of the interstice – a movement whose discontinuous and ‘aberrant’ nature requires further analysis." Blackburne — www.parrhesiajournal.org 25 — "Espacement also evokes the ambiguous figure of the interstice, and is related to the equally complex derridean notions of chora , différance , the trace and the supplement. Derrida’s reading of the Platonic chora in Chora L Works (a series of discussions with the architect Peter Eisenman) as something which defies the logics of non-contradiction and binarity, implies the internal heterogeneity and instability of all structures, neither ‘sensible’ nor ‘intelligible’ but a third genus which escapes conceptual capture.25 Crucially, chora , spacing, dissemination and différance are highly dynamic concepts, involving hybridity, an ongoing ‘corruption’ of categories, and a ‘bastard reasoning.’26 Derrida identification of différance in Margins of Philosophy , as an ‘unappropriable excess’ that operates through spacing as ‘the becoming-space of time or the becoming-time of space,’27 chimes with his description of chora as an ‘unidentifiable excess’ that is ‘the spacing which is the condition for everything to take place,’ opening up the interval as the plurivocity of writing in defiance of ‘origin’ and ‘essence.’28 In this unfolding of différance , spacing ‘insinuates into presence an interval,’29 again alerting us to the crucial role of the interstice in deconstruction, and, as Derrida observes in Positions , its impact as ‘a movement, a displacement that indicates an irreducible alterity’: ‘Spacing is the impossibility for an identity to be closed on itself, on the inside of its proper interiority, or on its coincidence with itself. The irreducibility of spacing is the irreducibility of the other.’30"
25. Quoted in Jeffrey Kipnis and Thomas Leeser, eds., 26. Ibid, 25.
27. Derrida, Margins of Philosophy. 28. Derrida, Chora L Works , 19 and 10. 29. Ibid, 203. 30. Derrida, Positions , 94. |
Comments Off on Espacement: Geometry of the Interstice in Literary Theory
Thursday, March 7, 2019
In Reality
The previous post, quoting a characterization of the R. T. Curtis
Miracle Octad Generator , describes it as a "hand calculator ."
Other views
A "natural diagram " —
A geometric object —
Comments Off on In Reality
Sunday, December 2, 2018
Symmetry at Hiroshima
A search this morning for articles mentioning the Miracle Octad Generator
of R. T. Curtis within the last year yielded an abstract for two talks given
at Hiroshima on March 8 and 9, 2018 —
|
http://www.math.sci.hiroshima-u.ac.jp/ branched/files/2018/abstract/Aitchison.txt
Iain AITCHISON Title: Construction of highly symmetric Riemann surfaces, related manifolds, and some exceptional objects, I, II Abstract: Since antiquity, some mathematical objects have played a special role, underpinning new mathematics as understanding deepened. Perhaps archetypal are the Platonic polyhedra, subsequently related to Platonic idealism, and the contentious notion of existence of mathematical reality independent of human consciousness. Exceptional or unique objects are often associated with symmetry – manifest or hidden. In topology and geometry, we have natural base points for the moduli spaces of closed genus 2 and 3 surfaces (arising from the 2-fold branched cover of the sphere over the 6 vertices of the octahedron, and Klein's quartic curve, respectively), and Bring's genus 4 curve arises in Klein's description of the solution of polynomial equations of degree greater than 4, as well as in the construction of the Horrocks-Mumford bundle. Poincare's homology 3-sphere, and Kummer's surface in real dimension 4 also play special roles. In other areas: we have the exceptional Lie algebras such as E8; the sporadic finite simple groups; the division algebras: Golay's binary and ternary codes; the Steiner triple systems S(5,6,12) and S(5,8,24); the Leech lattice; the outer automorphisms of the symmetric group S6; the triality map in dimension 8; and so on. We also note such as: the 27 lines on a cubic, the 28 bitangents of a quartic curve, the 120 tritangents of a sextic curve, and so on, related to Galois' exceptional finite groups PSL2(p) (for p= 5,7,11), and various other so-called `Arnol'd Trinities'. Motivated originally by the `Eightfold Way' sculpture at MSRI in Berkeley, we discuss inter-relationships between a selection of these objects, illustrating connections arising via highly symmetric Riemann surface patterns. These are constructed starting with a labeled polygon and an involution on its label set. Necessarily, in two lectures, we will neither delve deeply into, nor describe in full, contexts within which exceptional objects arise. We will, however, give sufficient definition and detail to illustrate essential inter-connectedness of those exceptional objects considered. Our starting point will be simplistic, arising from ancient Greek ideas underlying atomism, and Plato's concepts of space. There will be some overlap with a previous talk on this material, but we will illustrate with some different examples, and from a different philosophical perspective. Some new results arising from this work will also be given, such as an alternative graphic-illustrated MOG (Miracle Octad Generator) for the Steiner system S(5,8,24), and an alternative to Singerman – Jones' genus 70 Riemann surface previously proposed as a completion of an Arnol'd Trinity. Our alternative candidate also completes a Trinity whose two other elements are Thurston's highly symmetric 6- and 8-component links, the latter related by Thurston to Klein's quartic curve. |
See also yesterday morning's post, "Character."
Update: For a followup, see the next Log24 post.
Comments Off on Symmetry at Hiroshima
Thursday, November 8, 2018
Reality vs. Axiomatic Thinking
From https://blogs.scientificamerican.com/…
|
A Few of My Favorite Spaces:
The intuition-challenging Fano plane may be By Evelyn Lamb on October 24, 2015
"…finite projective planes seem like |
For Fano's axiomatic approach, see the Nov. 3 Log24 post
"Foundations of Geometry."
For the Fano plane's basis in reality , see the eightfold cube
at finitegeometry.org/sc/ and in this journal.
See as well "Two Views of Finite Space" (in this journal on the date
of Lamb's remarks — Oct. 24, 2015).
Some context: Gödel's Platonic realism vs. Hilbert's axiomatics
in remarks by Manuel Alfonseca on June 7, 2018. (See too remarks
in this journal on that date, in posts tagged "Road to Hell.")
Comments Off on Reality vs. Axiomatic Thinking
Wednesday, September 5, 2018
Multifaceted Narrative
See also, in this journal, 23-cycle.
Update of Sept. 6, 2018, 9:05 AM ET: "The Cubist Method" —
Multifaceted narrative by James Joyce —
Multifaceted structures in pure mathematics, from Plato and R. T. Curtis —
Comments Off on Multifaceted Narrative
Saturday, August 4, 2018
Manifestations of Exquisite Geometry
An alleged manifestation in physics, from Scientific American —
Manifestations in pure mathematics, from Plato and R. T. Curtis —
For some entertaining literary manifestations, see Wrinkle.
Comments Off on Manifestations of Exquisite Geometry
Wednesday, July 18, 2018
Doodle
From "The Educated Imagination: A Website Dedicated
to Northrop Frye" —
"In one of the notebooks for his first Bible book Frye writes,
'For at least 25 years I’ve been preoccupied by
the notion of a key to all mythologies.' . . . .
Frye made a valiant effort to provide a key to all mythology,
trying to fit everything into what he called the Great Doodle. . . ."
From a different page at the same website —
Here Frye provides a diagram of four sextets.
I prefer the Miracle Octad Generator of R. T. Curtis —
.
Comments Off on Doodle
Thursday, June 28, 2018
All in Plato
Comments Off on All in Plato
Sunday, May 6, 2018
The Osterman Omega
From "The Osterman Weekend" (1983) —
Counting symmetries of the R. T. Curtis Omega:
An Illustration from Shakespeare's birthday —
Comments Off on The Osterman Omega
Monday, April 23, 2018
Facets
See also the Feb. 17, 2017, post on Bertram Kostant
as well as "Mathieu Moonshine and Symmetry Surfing."
Comments Off on Facets
Saturday, April 1, 2017
Art Space
Click image for some backstory.
“Whatever he drew was the platonic ideal
of what a cartoon should look like.”
— Bob Mankoff on Jack Ziegler, who reportedly
died on Wednesday, March 29, 2017.
See also "Hexagram 64 in Context," March 16, 2017.
Comments Off on Art Space
Thursday, March 16, 2017
Iacta Est
"Though realism is excellent rhetoric, maybe the best,
in a purely technical or instrumental sense,
that cannot be an adequate reason to accept it
as a serious intellectual position. In its tropes of
Death and Furniture we see a rhetoric that refuses
to acknowledge its own existence; a politics that
can claim a critical-radical credibility only by
the selective use of its opponents' analytic tools;
and a theology which is deeply conservative and
seeks nothing less than the death of disruptive,
disturbing inquiry. While tedium, good taste, political
and moral sensibility will properly determine what
sorts of given realities are thought worthy of inquiry,
those considerations are no grounds for promoting
a realist ontology for social science, nor any other
science, nor for rejecting relativism. On the contrary,
relativism is social science par excellence . . . ."
Loughborough University
— Edwards, D., Ashmore, M., and Potter, J. (1995),
"Death and furniture: The rhetoric, politics and theology
of bottom line arguments against relativism,"
History of the Human Sciences , 8, 25-49.
Related material:
Platonic realism in this journal, yesterday's post Ripples, and …
Gravity's Shadow , 2004 —
Gravity's Ghost , 2010 —
See also an "Inception"-related object —
Comments Off on Iacta Est
Friday, February 17, 2017
Kostant Is Dead
"Bertram Kostant, professor emeritus of mathematics at MIT,
died at the Hebrew Senior Rehabilitation Center in Roslindale,
Massachusetts, on Thursday, Feb. 2, at the age of 88."
— MIT News, story dated Feb. 16, 2017
See also a search for Kostant in this journal.
Regarding the discussions of symmetries and "facets" found in
that search —
Kostant:
“A word about E(8). In my opinion, and shared by others,
E(8) is the most magnificent ‘object’ in all of mathematics.
It is like a diamond with thousands of facets. Each facet
offering a different view of its unbelievable intricate internal
structure.”
Cullinane:
In the Steiner system S(5, 8, 24) each octad might be
regarded as a "facet," with the order of the system's
automorphism group, the Mathieu group M24 , obtained
by multiplying the number of such facets, 759, by the
order of the octad stabilizer group, 322,560.
Analogously …
Comments Off on Kostant Is Dead
Sunday, October 23, 2016
Quartet
“The man who lives in contact with what he believes to be a living Church
is a man always expecting to meet Plato and Shakespeare to-morrow
at breakfast.”
— G. K. Chesterton
Or Sunday dinner.
|
Platonic |
Shakespearean |
| Not to mention Euclid and Picasso. | |
|
|
|
|
In the above pictures, Euclid is represented by |
|
Comments Off on Quartet
Sunday, April 12, 2015
The Greek Fifth Element:
The Dodecahedron .
This Platonic solid appears, for instance, on the cover
of a colorful text titled The Heart of Mathematics
(Wiley, third edition, 2009) —
For serious students, here is a better book, more in
keeping with the above authors' later interpretation
of the fifth element as change :
Comments Off on The Greek Fifth Element:
Monday, December 29, 2014
Dodecahedron Model of PG(2,5)
Recent posts tagged Sagan Dodecahedron
mention an association between that Platonic
solid and the 5×5 grid. That grid, when extended
by the six points on a "line at infinity," yields
the 31 points of the finite projective plane of
order five.
For details of how the dodecahedron serves as
a model of this projective plane (PG(2,5)), see
Polster's A Geometrical Picture Book , p. 120:
For associations of the grid with magic rather than
with Plato, see a search for 5×5 in this journal.
Comments Off on Dodecahedron Model of PG(2,5)
Friday, December 19, 2014
Colorful Tale
Wikipedia on a tale about one Hippasus of Metapontum,
who supposedly was drowned by Pythagoreans for his
discovery of irrational numbers and/or of the dodecahedron —
"In the hands of modern writers this combination of vague
ancient reports and modern guesswork has sometimes
evolved into a much more emphatic and colourful tale."
See, for instance, a tale told by the late Carl Sagan,
who was bitterly anti-Pythagorean (and anti-Platonic):
For a related colorful tale, see "Patrick Blackburn" in this journal.
Comments Off on Colorful Tale
Wednesday, November 26, 2014
A Tetrahedral Fano-Plane Model
Update of Nov. 30, 2014 —
It turns out that the following construction appears on
pages 16-17 of A Geometrical Picture Book , by
Burkard Polster (Springer, 1998).
"Experienced mathematicians know that often the hardest
part of researching a problem is understanding precisely
what that problem says. They often follow Polya's wise
advice: 'If you can't solve a problem, then there is an
easier problem you can't solve: find it.'"
—John H. Conway, foreword to the 2004 Princeton
Science Library edition of How to Solve It , by G. Polya
For a similar but more difficult problem involving the
31-point projective plane, see yesterday's post
"Euclidean-Galois Interplay."
The above new [see update above] Fano-plane model was
suggested by some 1998 remarks of the late Stephen Eberhart.
See this morning's followup to "Euclidean-Galois Interplay"
quoting Eberhart on the topic of how some of the smallest finite
projective planes relate to the symmetries of the five Platonic solids.
Update of Nov. 27, 2014: The seventh "line" of the tetrahedral
Fano model was redefined for greater symmetry.
Comments Off on A Tetrahedral Fano-Plane Model
Class Act
Update of Nov. 30, 2014 —
For further information on the geometry in
the remarks by Eberhart below, see
pp. 16-17 of A Geometrical Picture Book ,
by Burkard Polster (Springer, 1998). Polster
cites a different article by Lemay.
A search for background to the exercise in the previous post
yields a passage from the late Stephen Eberhart:
|
The first three primes p = 2, 3, and 5 therefore yield finite projective planes with 7, 13, and 31 points and lines, respectively. But these are just the numbers of symmetry axes of the five regular solids, as described in Plato's Timaeus : The tetrahedron has 4 pairs of face planes and corner points + 3 pairs of opposite edges, totalling 7 axes; the cube has 3 pairs of faces + 6 pairs of edges + 4 pairs of corners, totalling 13 axes (the octahedron simply interchanges the roles of faces and corners); and the pentagon dodecahedron has 6 pairs of faces + 15 pairs of edges + 10 pairs of corners, totalling 31 axes (the icosahedron again interchanging roles of faces and corners). This is such a suggestive result, one would expect to find it dealt with in most texts on related subjects; instead, while "well known to those who well know such things" (as Richard Guy likes to quip), it is scarcely to be found in the formal literature [9]. The reason for the common numbers, it turns out, is that the groups of symmetry motions of the regular solids are subgroups of the groups of collineations of the respective finite planes, a face axis being different from an edge axis of a regular solid but all points of a projective plane being alike, so the latter has more symmetries than the former. [9] I am aware only of a series of in-house publications by Fernand Lemay of the Laboratoire de Didactique, Faculté des Sciences de I 'Éducation, Univ. Laval, Québec, in particular those collectively titled Genèse de la géométrie I-X.
— Stephen Eberhart, Dept. of Mathematics, |
Eberhart died of bone cancer in 2003. A memorial by his
high school class includes an Aug. 7, 2003, transcribed
letter from Eberhart to a classmate that ends…
|
… I earned MA’s in math (UW, Seattle) and history (UM, Missoula) where a math/history PhD program had been announced but canceled. So 1984 to 2002 I taught math (esp. non-Euclidean geometry) at C.S.U. Northridge. It’s been a rich life. I’m grateful. Steve |
See also another informative BRIDGES paper by Eberhart
on mathematics and the seven traditional liberal arts.
Comments Off on Class Act
Thursday, July 17, 2014
Paradigm Shift:
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Comments Off on Paradigm Shift:
Wednesday, July 16, 2014
Friday, April 25, 2014
Bingo
For John Milton at the Cervecería XX —
- Bingo in this journal, as well as
- Black Mass and
- “The Platonic Heritage in Under the Volcano“
(Studies in Canadian Literature, v. 3, no. 1, 1978)
Related material: Peter J. Cameron on Bertrand Russell
in A Midnight Exorcism.
Comments Off on Bingo
Tuesday, December 31, 2013
Christmas Ornaments
Continued from December 25—
A link from Sunday afternoon to Nov. 26, 2012,
suggests a review of one of the above structures.
The Dreaming Jewels cover at left is taken from a review
by Jo Walton at Tor.com—
"This is a book that it’s clearly been difficult
for publishers to market. The covers have been
generally pretty awful, and also very different.
I own a 1975 Corgi SF Collectors Library
paperback that I bought new for 40p in the later
seventies. It’s purple, and it has a slightly grainy
cover, and it matches my editions of The Menace
From Earth and A Canticle for Leibowitz .
(Dear old Corgi SF Collectors Editions with their
very seventies fonts! How I imprinted on them at
an early age!) I mention this, however, because
the (uncredited) illustration actually represents and
illustrates the book much better than any of the other
cover pictures I’ve seen. It shows a hexagon with an
attempt at facets, a man, a woman, hands, a snake,
and stars, all in shades of green. It isn’t attractive,
but it wouldn’t put off people who’d enjoy what’s inside
either."
The "hexagon with an attempt at facets" is actually
an icosahedron, as the above diagram shows.
(The geometric part of the diagram is from a Euclid webpage.)
For Plato's dream about these jewels, see his Timaeus.
Comments Off on Christmas Ornaments
Thursday, December 26, 2013
Dicey
For fans of Hunger Games and Elysium —
Roberta Smith in this evening's* online New York Times—
"Especially with the gap between the wealthiest
and everyone else so wide, it is dicey
for a major museum to celebrate the often frivolous
objects on which the rich spend their ever increasing
surplus income. Such a show must be beyond reproach
in every way: transparent in organization, impeccable
in exhibition design, illuminating in catalog and labeling
and, most of all, self-evidently excellent in the quality of
the objects on display."
Da capo: "I've heard of affairs that are strictly Platonic."
Comments Off on Dicey
Wednesday, December 25, 2013
Rotating the Facets
“… her mind rotated the facts….”
Related material— hypercube rotation,* in the context
of rotational symmetries of the Platonic solids:
“I’ve heard of affairs that are strictly Platonic”
* Footnote added on Dec. 26, 2013 —
See Arnold Emch, “Triple and Multiple Systems, Their Geometric
Configurations and Groups,” Trans. Amer. Math. Soc. 31 (1929),
No. 1, 25–42.
On page 42, Emch describes the above method of rotating a
hypercube’s 8 facets (i.e., three-dimensional cubes) to count
rotational symmetries —
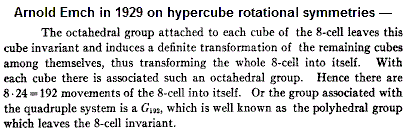
See also Diamond Theory in 1937.
Also on p. 42, Emch mentions work of Carmichael on a
Steiner system with the Mathieu group M11 as automorphism
group, and poses the problem of finding such systems and
groups that are larger. This may have inspired the 1931
discovery by Carmichael of the Steiner system S(5, 8, 24),
which has as automorphisms the Mathieu group M24 .
Comments Off on Rotating the Facets
Saturday, November 30, 2013
For Sean Connery
On St. Andrew's Day.
A Connery adventure in Kuala Lumpur—
For another Kuala Lumpur adventure, see today's update
to "In Defense of Plato's Realism"—
The July 5, 2007, post linked to
"Plato, Pegasus, and the Evening Star."
For related drama from Kuala Lumpur, see
"Occam's Razor, Plato's Beard."
Comments Off on For Sean Connery
Saturday, November 16, 2013
Mathematics and Rhetoric
Jim Holt in the current (Dec. 5) New York Review of Books—
| One form of Eros is the sexual desire aroused by the physical beauty of a particular beloved person. That, according to Diotima, is the lowest form. With philosophical refinement, however, Eros can be made to ascend toward loftier and loftier objects. The penultimate of these—just short of the Platonic idea of beauty itself—is the perfect and timeless beauty discovered by the mathematical sciences. Such beauty evokes in those able to grasp it a desire to reproduce—not biologically, but intellectually, by begetting additional “gloriously beautiful ideas and theories.” For Diotima, and presumably for Plato as well, the fitting response to mathematical beauty is the form of Eros we call love. |
Consider (for example) the beauty of the rolling donut—
(Animation source: MIQEL.com)
Comments Off on Mathematics and Rhetoric
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—
Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Comments Off on Vril Chick
Tuesday, July 31, 2012
Logo
"The universe, then, is less intimation
than cipher: a mask rather than a revelation
in the romantic sense. Does love meet with love?
Do we receive but what we give? The answer is
surely a paradox, the paradox that there are
Platonic universals beyond, but that the glass
is too dark to see them. Is there a light beyond
the glass, or is it a mirror only to the self?
The Platonic cave is even darker than Plato
made it, for it introduces the echo, and so
leaves us back in the world of men, which does
not carry total meaning, is just a story of events."
than cipher: a mask rather than a revelation
in the romantic sense. Does love meet with love?
Do we receive but what we give? The answer is
surely a paradox, the paradox that there are
Platonic universals beyond, but that the glass
is too dark to see them. Is there a light beyond
the glass, or is it a mirror only to the self?
The Platonic cave is even darker than Plato
made it, for it introduces the echo, and so
leaves us back in the world of men, which does
not carry total meaning, is just a story of events."
– Betty Jay, reader's guide to A Passage to India
Judy Davis in the Marabar Caves
The above image is from this journal on Sunday, April 13, 2008.
The preceding cover of a book by Northrop Frye was suggested
by material in this journal from February 2003.
See also Yankee Puzzle and Doodle Dandy.
Comments Off on Logo
Saturday, June 9, 2012
Misquoting Nietzsche
Jim Holt in tomorrow’s New York Times—
“Allow me to quote Nietzsche
(although I know that will be considered
by some to be in bad taste):
‘As the circle of science grows larger,
it touches paradox at more places.'”
A possible source for this misquotation—
Harvard University Press—
A more accurate quotation—
Anyone who has ever experienced the pleasure of Socratic insight and felt how, spreading in ever-widening circles, it seeks to embrace the whole world of appearances, will never again find any stimulus toward existence more violent than the craving to complete this conquest and to weave the net impenetrably tight. To one who feels that way, the Platonic Socrates will appear as the teacher of an altogether new form of “Greek cheerfulness” and blissful affirmation of existence that seeks to discharge itself in actions— most often in maieutic and educational influences on noble youths, with a view to eventually producing a genius.
But science, spurred by its powerful illusion, speeds irresistibly towards its limits where its optimism, concealed in the essence of logic, suffers shipwreck. For the periphery of the circle of science has an infinite number of points; and while there is no telling how this circle could ever be surveyed completely, noble and gifted men nevertheless reach, e’er half their time and inevitably, such boundary points on the periphery from which one gazes into what defies illumination. When they see to their horror how logic coils up at these boundaries and finally bites its own tail— suddenly the new form of insight breaks through, tragic insight which, merely to be endured, needs art as a protection and remedy.
— Friedrich Nietzsche, The Birth of Tragedy , translated by Walter Kaufmann (Modern Library)
Comments Off on Misquoting Nietzsche
Tuesday, March 13, 2012
Geometry and Death
Continued from other posts.
Related material from Washington Jewish Week—
"Abramson did not always get his way; he didn't have to win, but never took his eye off the ball— the Museum had to emerge the better. He did not take loses personally but pragmatically. A design for the Museum building done by an architect from his firm was charitably speaking 'mediocre.' It was replaced by a brilliant building designed by James Ingo Freed who rightfully regarded it as the master work of his distinguished career. Abramson became Freed's champion. He pushed the design team for a happy ending, saying that he knew the American people and they needed an uplifting ending since the subject of the Holocaust was so very depressing."
— and from the Holocaust Memorial Museum—
Update of 5:01 AM March 13—
See also yesterday's post The Line and
the section "The Pythagorean/ Platonic tradition"
at David Wade's website Pattern in Islamic Art.
Comments Off on Geometry and Death
Friday, November 18, 2011
Hypercube Rotations
The hypercube has 192 rotational symmetries.
Its full symmetry group, including reflections,
is of order 384.
See (for instance) Coxeter—
Related material—
The rotational symmetry groups of the Platonic solids
(from April 25, 2011)—
— and the figure in yesterday evening's post on the hypercube—
(Animation source: MIQEL.com)
Clearly hypercube rotations of this sort carry any
of the eight 3D subcubes to the central subcube
of a central projection of the hypercube—
The 24 rotational symmeties of that subcube induce
24 rigid rotations of the entire hypercube. Hence,
as in the logic of the Platonic symmetry groups
illustrated above, the hypercube has
rotational symmetries.
Comments Off on Hypercube Rotations
Monday, April 25, 2011
Poetry and Physics
One approach to the storied philosophers' stone, that of Jim Dodge in Stone Junction , was sketched in yesterday's Easter post. Dodge described a mystical "spherical diamond." The symmetries of the sphere form what is called in mathematics a Lie group . The "spherical" of Dodge therefore suggests a review of the Lie group E8 in Garrett Lisi's poetic theory of everything.
A check of the Wikipedia article on Lisi's theory yields…
Diamond and E8 at Wikipedia
Related material — E8 as "a diamond with thousands of facets"—
Also from the New Yorker article—
“There’s a dream that underlying the physical universe is some beautiful mathematical structure, and that the job of physics is to discover that,” Smolin told me later. “The dream is in bad shape,” he added. “And it’s a dream that most of us are like recovering alcoholics from.” Lisi’s talk, he said, “was like being offered a drink.”
A simpler theory of everything was offered by Plato. See, in the Timaeus , the Platonic solids—
Figure from this journal on August 19th, 2008.
See also July 19th, 2008.
“It’s all in Plato, all in Plato:
bless me, what do they
teach them at these schools!”
— C. S. Lewis
Comments Off on Poetry and Physics
Friday, December 17, 2010
Fare Thee Well
Excerpt from a post of 8 AM May 26, 2006 —
|
A Living Church "The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast." – G. K. Chesterton
|
A related scene from the opening of Blake Edwards's "S.O.B." —
Comments Off on Fare Thee Well
Sunday, August 8, 2010
Scowl
From the preface to the inaugural issue of Tympanum: A Journal of Comparative Literary Studies—
Regarding the choice of name for this journal, tympan or tympanum is a word that designates several objects at once. Tympan is perhaps first of all a typographical term: as a printer's term in early book production, a tympan designated "the iron frame covered with parchment on which the paper was placed." Taken as an anatomical term, the word tympanum is another term for the eardrum, the oblique stretching of tissue between the auditory canal and the middle ear that allows one to hear: to hear others, to hear music, or even to hear oneself speak. The tympanum is a partition of the ear that separates inside from outside, translating various tones and punctuations, a liminal membrane traversed by hearing others speak. In this instance, the tympanum is a tissue, a weave or web that mediates hearing. It is by extension the term for the diaphragm of a tele-phone, that technological figure of the spatialization of the voice. As an architectural term tympanum names the pediment that sits atop the cornice or frieze of a building. And to this heterogeneous list one might add that in ancient Greece a tympanum, like the stoa or colonnades, was a gathering space for the discussion of philosophy. All these meanings could be enlisted to indicate the interests of this new journal.
By its very nature, a world wide web site would be a site of a mediation of or meditation on the problematic of space and place (in short: of "site" itself), and of their dislocation. In this way the web opens the possibility for a journal concerned with the problem of a mediated or textualized hearing.
Several of the articles contained in this first issue of Tympanum share a thematic of location and of reading and hearing….
Deborah Levitt's essay on Heidegger and theatre, in its exploration of the problem of space and place, implicitly touches on the very medium of the web: the perpetual dislocation of place from space. Levitt couples several of Heidegger's writings together with Artaud's on her Freiburg-Paris Express. Levitt's meditation on theory and theatre is at once incisive and innovative, and locates its opening problematic in the substitution of a metaphysics of sight by site, a move which she says opens a spatiality. In a recent issue of Assemblage, Sam Weber makes some remarks on the metaphysics of site that could indeed be used as a succinct introduction to the problems that Levitt's essay, Heidegger and the Theatre of Truth, engages:
If what we call "space" is, like the Platonic chora, on the one hand always already caught up in the process of making room for that determinate other of space that can be called place or site, and if, on the other hand, this process of making room remains distinct from the particular places and sites it makes way for, then the emergence of the latter from the former will inevitably appear as a more or less violent event. Violent, because the staking out of territory and the assignment of positions and posts can never simply legitimate itself in terms of preexisting borders. It cannot do this, since there is no original order to which such a process of partition might appeal without equivocation. In place of such an origin, there is chora: the process of partition and repartition as such, except that "as such" here is impossible to distinguish from: "as other." Such partition and repartition constitute the law, the nomos, of chora…3
3 Samuel Weber, "The Parallax View: Place and Space in Plato and Benjamin," Assemblage 20, MIT Press: 1993: 88.
The Tympanum preface (1998) is by Peter Woodruff.
Wallace Stevens—
"The pediment
Lifts up its heavy scowl before them."
Scowl courtesy of Samuel Weber—
- Author of "The Parallax View"
- Assemblage, No. 20, Violence, Space
(Apr., 1993), pp. 88-89
(article consists of 2 pages) - Published by: The MIT Press
Comments Off on Scowl
Monday, July 26, 2010
Brightness at Noon continued
Riddle
"Midnight in the Garden continued," a post of 12:00 AM July 20, posed the riddle of what the previous day's NY Lottery midday "440" might mean.
A jocular answer was given. Some background for a more serious answer—
Paul Newall, “Kieślowski’s Three Colours Trilogy”—
“Julie recognises the music of the busker outside playing a recorder as that of her husband’s. When she asks him where he heard it, he replies that he makes up all sorts of things. This is an instance of a theory of Kieślowski’s that ‘different people, in different places, are thinking the same thing but for different reasons.’ With regard to music in particular, he held what might be characterised as a Platonic view according to which notes pre-exist and are picked out and assembled by people. That these can accord with one another is a sign of what connects people, or so he believed.”
In honor of Wye Jamison Allanbrook, author of Rhythmic Gesture in Mozart, we note that 440 is Concert A.
Allanbrook died on July 15. See this journal on that date—
Angels in the Architecture,
Happy Birthday, and
Brightness at Noon.
Comments Off on Brightness at Noon continued
Saturday, June 26, 2010
Bold and Brilliant Emergence
"Rosemary Desjardins argues boldly and brilliantly that the Theaetetus contains not only an answer to the question of the character of knowledge, but considerably more besides — an outline of a Platonic ontology. That ontology is neither materialist nor idealist (it is not a theory of forms), but like the twentieth century theory known as generative emergence holds that beings are particular interactive combinations of material elements. On this view, while wholes (for example, words, to use a Platonic model) may be analyzed into their elemental parts (letters), each whole has a property or quality separate from the aggregated properties of its parts."
— Stephen G. Salkever, 1991 review of The Rational Enterprise : Logos in Plato's Theaetetus (SUNY Press, 1990)
See also "strong emergence" in this journal.
Comments Off on Bold and Brilliant Emergence
Monday, June 7, 2010
Inspirational Combinatorics
According to the Mathematical Association of America this morning, one purpose of the upcoming June/July issue of the Notices of the American Mathematical Society is
"…to stress the inspirational role of combinatorics…."
Here is another contribution along those lines—
Eidetic Variation
from page 244 of
From Combinatorics to Philosophy: The Legacy of G.-C. Rota,
hardcover, published by Springer on August 4, 2009
(Edited by Ernesto Damiani, Ottavio D'Antona, Vincenzo Marra, and Fabrizio Palombi)
"Rota's Philosophical Insights," by Massimo Mugnai—
"… In other words, 'objectivism' is the attitude [that tries] to render a particular aspect absolute and dominant over the others; it is a kind of narrow-mindedness attempting to reduce to only one the multiple layers which constitute what we call 'reality.' According to Rota, this narrow-mindedness limits in an essential way even of [sic ] the most basic facts of our cognitive activity, as, for example, the understanding of a simple declarative sentence: 'So objectivism is the error we [make when we] persist in believing that we can understand what a declarative sentence means without a possible thematization of this declarative sentence in one of [an] endless variety of possible contexts' (Rota, 1991*, p. 155). Rota here implicitly refers to what, amongst phenomenologists is known as eidetic variation, i.e. the change of perspective, imposed by experience or performed voluntarily, from which to look at things, facts or sentences of the world. A typical example, proposed by Heidegger, in Sein und Zeit (1927) and repeated many times by Rota, is that of the hammer."
* Rota, G.-C. (1991), The End of Objectivity: The Legacy of Phenomenology. Lectures at MIT, Cambridge, MA, MIT Mathematics Department
The example of the hammer appears also on yesterday's online New York Times front page—
Related material:
From The Blackwell Dictionary of Western Philosophy—
Eidetic variation — an alternative expression for eidetic reduction
Husserl's term for an intuitive act toward an essence or universal, in contrast to an empirical intuition or perception. He also called this act an essential intuition, eidetic intuition, or eidetic variation. In Greek, eideo means “to see” and what is seen is an eidos (Platonic Form), that is, the common characteristic of a number of entities or regularities in experience. For Plato, eidos means what is seen by the eye of the soul and is identical with essence. Husserl also called this act “ideation,” for ideo is synonymous with eideo and also means “to see” in Greek. Correspondingly, idea is identical to eidos.
An example of eidos— Plato's diamond (from the Meno )—
For examples of variation of this eidos, see the diamond theorem.
See also Blockheads (8/22/08).
Related poetic remarks— The Trials of Device.
Comments Off on Inspirational Combinatorics
Wednesday, May 19, 2010
Preforming
Photo caption in NY Times today— a pianist "preforming" in 1967. (See today's previous post.)
The pianist's life story seems in part to echo that of Juliette Binoche in the film "Bleu." Binoche appeared in this journal yesterday, before I had seen the pianist in today's Times obituaries. The Binoche appearance was related to the blue diamond in the film "Duelle " (Tuesday morning's post) and the saying of Heraclitus "immortals mortal, mortals immortal" (Tuesday afternoon's post).
This somewhat uncanny echo brings to mind Nabokov—
Life Everlasting—based on a misprint!
I mused as I drove homeward: take the hint,
And stop investigating my abyss?
But all at once it dawned on me that this
Was the real point, the contrapuntal theme;
Just this: not text, but texture; not the dream
But topsy-turvical coincidence,
Not flimsy nonsense, but a web of sense.
Whether sense or nonsense, the following quotation seems relevant—
"Archetypes function as living dispositions, ideas in the Platonic sense, that preform and continually influence our thoughts and feelings and actions." –C.G. Jung in Four Archetypes: Mother, Rebirth, Spirit, Trickster, the section titled "On the Concept of the Archetype."
That section is notable for its likening of Jungian archetypes to Platonic ideas and to axial systems of crystals. See also "Cubist Tune," March 18 —
Comments Off on Preforming
Saturday, November 14, 2009
For St. Lawrence O’Toole’s Day
“We have a need to tell ourselves stories
that explain it all. We use these stories to
supply the metaphysics,* without which
life seems pointless and empty.”
— David Brooks, NY Times of Nov. 10
“The story-teller of hell”
— Publisher’s promotional quotation
for The Nick Tosches Reader
* “the metaphysics“– This link leads to a web page at the Archdiocese of Dublin whose relevance to metaphysics is not obvious. Of course, from the point of view popular with viXra authors (see Thursday), everything is related to metaphysics. The link is to a homily that mentions Sr. Joan Chittester, O.S.B. A search on her works at Amazon.com leads to Welcome to the Wisdom of the World And Its Meaning for You: Universal Spiritual Insights Distilled from Five Religious Traditions. The title indicates that despite Chittester’s personal virtues, her book is, unlike the Tosches book above, less than first-rate. Still, a “meaning for you” is, in my case, not lacking. Continuing the search for a Joycean epiphany related to metaphysics, I found that the Chittester book‘s date of publication (by Eerdmans, the Grand Rapids Calvinist publisher) was July 24, 2007. For a metaphysical phrase on that date– “the Platonic ‘source of all images,'” see The Church of St. Frank. For metaphysics and the Church of some other saints, see the essay on the “metaphysics of goodness” linked to on the publication date of Chittester’s book.
Comments Off on For St. Lawrence O’Toole’s Day
Saturday, October 24, 2009
Chinese Cubes Continued
A search for “Chinese Cube” (based on the the previous entry’s title) reveals the existence of a most interesting character, who…
“… has attempted in his books to produce a Science and Art of Reasoning using the simplest of the Platonic solids, the Cube. [His] model also parallels, in some ways, the Cube of Space constructed from the Sepher Yetzirah’s attributions for the Hebrew letters and their direction. [He] elucidated his theories at great length….”
— More…
For related remarks, see the link to Solomon’s Cube from the previous entry.
Then of course there is…
Click on figure for details.
Comments Off on Chinese Cubes Continued
Wednesday, August 5, 2009
Wednesday August 5, 2009
Word and Image
"Edward T. Hall, a cultural anthropologist
who pioneered the study of nonverbal
communication and interactions between
members of different ethnic groups,
died July 20 at his home in
Santa Fe, N.M. He was 95."
NY Times piece quoted here on
the date of Hall's death:
|
"July 20, 1969, was the moment NASA needed, more than anything else in this world, the Word. But that was something NASA's engineers had no specifications for. At this moment, that remains the only solution to recovering NASA's true destiny, which is, of course, to build that bridge to the stars." Commentary —
The Word according to St. John:
|
From Hall's obituary:
"Mr. Hall first became interested in
space and time as forms of cultural
expression while working on
Navajo and Hopi reservations
in the 1930s."
Log24, July 29:
|
"Kaleidoscope turning…
Shifting pattern within |
"We are the key."
— Eye of Cat
Update of about 4:45 PM 8/5:
Paul Newall, "Kieślowski's Three Colours Trilogy"—
"Julie recognises the music of the busker outside playing a recorder as that of her husband's. When she asks him where he heard it, he replies that he makes up all sorts of things. This is an instance of a theory of Kieślowski's that 'different people, in different places, are thinking the same thing but for different reasons.' With regard to music in particular, he held what might be characterised as a Platonic view according to which notes pre-exist and are picked out and assembled by people. That these can accord with one another is a sign of what connects people, or so he believed."
The above photo of Juliette Binoche in Blue accompanying the quotations from Zelazny illustrates Kieślowski's concept, with graphic designs instead of musical notes. Some of the same designs are discussed in Abstraction and the Holocaust (Mark Godfrey, Yale University Press, 2007). (See the Log24 entries of June 11, 2009.)
Related material:
"Jeffrey Overstreet, in his book Through a Screen Darkly, comments extensively on Blue. He says these stones 'are like strands of suspended crystalline tears, pieces of sharp-edged grief that Julie has not been able to express.'….
Throughout the film the color blue crops up, highlighting the mood of Julie's grief. A blue light occurs frequently, when Julie is caught by some fleeting memory. Accompanied by strains of an orchestral composition, possibly her husband's, these blue screen shots hold for several seconds while Julie is clearly processing something. The meaning of this blue light is unexplained. For Overstreet, it is the spirit of reunification of broken things."
— Martin Baggs at Mosaic Movie Connect Group on Sunday, March 15, 2009. (Cf. Log24 on that date.)
For such a spirit, compare Binoche's blue mobile in Blue with Binoche's gathered shards in Bee Season.
Comments Off on Wednesday August 5, 2009
Tuesday, August 19, 2008
Tuesday August 19, 2008
Three Times
|
"Credences of Summer," VII,
by Wallace Stevens, from
"Three times the concentred |
Stevens does not say what object he is discussing.
One possibility —
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in a recent New Yorker:
"A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Another possibility —
A more modest object —
the 4×4 square.
Update of Aug. 20-21 —
Symmetries and Facets
Kostant's poetic comparison might be applied also to this object.
The natural rearrangements (symmetries) of the 4×4 array might also be described poetically as "thousands of facets, each facet offering a different view of… internal structure."
More precisely, there are 322,560 natural rearrangements– which a poet might call facets*— of the array, each offering a different view of the array's internal structure– encoded as a unique ordered pair of symmetric graphic designs. The symmetry of the array's internal structure is reflected in the symmetry of the graphic designs. For examples, see the Diamond 16 Puzzle.
For an instance of Stevens's "three times" process, see the three parts of the 2004 web page Ideas and Art.
* For the metaphor of rearrangements as facets, note that each symmetry (rearrangement) of a Platonic solid corresponds to a rotated facet: the number of symmetries equals the number of facets times the number of rotations (edges) of each facet–
The metaphor of rearrangements as facets breaks down, however, when we try to use it to compute, as above with the Platonic solids, the number of natural rearrangements, or symmetries, of the 4×4 array. Actually, the true analogy is between the 16 unit squares of the 4×4 array, regarded as the 16 points of a finite 4-space (which has finitely many symmetries), and the infinitely many points of Euclidean 4-space (which has infinitely many symmetries).
If Greek geometers had started with a finite space (as in The Eightfold Cube), the history of mathematics might have dramatically illustrated Halmos's saying (Aug. 16) that
"The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question."
The Greeks, of course, answered the infinite questions first– at least for Euclidean space. Halmos was concerned with more general modern infinite spaces (such as Hilbert space) where the intuition to be gained from finite questions is still of value.
Comments Off on Tuesday August 19, 2008
Thursday, August 14, 2008
Thursday August 14, 2008
Magister Ludi
(The Glass Bead Game)
is now available for
download in pdf or
text format at Scribd.
(The Glass Bead Game)
is now available for
download in pdf or
text format at Scribd.
“How far back the historian wishes to place the origins and antecedents of the Glass Bead Game is, ultimately, a matter of his personal choice. For like every great idea it has no real beginning; rather, it has always been, at least the idea of it. We find it foreshadowed, as a dim anticipation and hope, in a good many earlier ages. There are hints of it in Pythagoras, for example, and then among Hellenistic Gnostic circles in the late period of classical civilization. We find it equally among the ancient Chinese, then again at the several pinnacles of Arabic-Moorish culture; and the path of its prehistory leads on through Scholasticism and Humanism to the academies of mathematicians of the seventeenth and eighteenth centuries and on to the Romantic philosophies and the runes of Novalis’s hallucinatory visions. This same eternal idea, which for us has been embodied in the Glass Bead Game, has underlain every movement of Mind toward the ideal goal of a universitas litterarum, every Platonic academy, every league of an intellectual elite, every rapprochement between the exact and the more liberal disciplines, every effort toward reconciliation between science and art or science and religion. Men like Abelard, Leibniz, and Hegel unquestionably were familiar with the dream of capturing the universe of the intellect in concentric systems, and pairing the living beauty of thought and art with the magical expressiveness of the exact sciences. In that age in which music and mathematics almost simultaneously attained classical heights, approaches and cross-fertilizations between the two disciplines occurred frequently.”
Comments Off on Thursday August 14, 2008
Sunday, April 13, 2008
Sunday April 13, 2008
The Echo
in Plato’s Cave
in Plato’s Cave
“It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy.”
— Simon Blackburn, Think (Oxford, 1999)
Michael Harris, mathematician at the University of Paris:
“… three ‘parts’ of tragedy identified by Aristotle that transpose to fiction of all types– plot (mythos), character (ethos), and ‘thought’ (dianoia)….”
— paper (pdf) to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.
Mythos —
A visitor from France this morning viewed the entry of Jan. 23, 2006: “In Defense of Hilbert (On His Birthday).” That entry concerns a remark of Michael Harris.
A check of Harris’s website reveals a new article:
“Do Androids Prove Theorems in Their Sleep?” (slighly longer version of article to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.) (pdf).
From that article:
“The word ‘key’ functions here to structure the reading of the article, to draw the reader’s attention initially to the element of the proof the author considers most important. Compare E.M. Forster in Aspects of the Novel:
[plot is] something which is measured not be minutes or hours, but by intensity, so that when we look at our past it does not stretch back evenly but piles up into a few notable pinnacles.”
Ethos —
“Forster took pains to widen and deepen the enigmatic character of his novel, to make it a puzzle insoluble within its own terms, or without. Early drafts of A Passage to India reveal a number of false starts. Forster repeatedly revised drafts of chapters thirteen through sixteen, which comprise the crux of the novel, the visit to the Marabar Caves. When he began writing the novel, his intention was to make the cave scene central and significant, but he did not yet know how:
When I began a A Passage to India, I knew something important happened in the Malabar (sic) Caves, and that it would have a central place in the novel– but I didn’t know what it would be… The Malabar Caves represented an area in which concentration can take place. They were to engender an event like an egg.”
— E. M. Forster: A Passage to India, by Betty Jay
Dianoia —
Flagrant Triviality
or Resplendent Trinity?
or Resplendent Trinity?
“Despite the flagrant triviality of the proof… this result is the key point in the paper.”
— Michael Harris, op. cit., quoting a mathematical paper
Online Etymology Dictionary:
flagrant c.1500, “resplendent,” from L. flagrantem (nom. flagrans) “burning,” prp. of flagrare “to burn,” from L. root *flag-, corresponding to PIE *bhleg– (cf. Gk. phlegein “to burn, scorch,” O.E. blæc “black”). Sense of “glaringly offensive” first recorded 1706, probably from common legalese phrase in flagrante delicto “red-handed,” lit. “with the crime still blazing.”
A related use of “resplendent”– applied to a Trinity, not a triviality– appears in the Liturgy of Malabar:
— The Liturgies of SS. Mark, James, Clement, Chrysostom, and Basil, and the Church of Malabar, by the Rev. J.M. Neale and the Rev. R.F. Littledale, reprinted by Gorgias Press, 2002
On Universals and
A Passage to India:
A Passage to India:
“”The universe, then, is less intimation than cipher: a mask rather than a revelation in the romantic sense. Does love meet with love? Do we receive but what we give? The answer is surely a paradox, the paradox that there are Platonic universals beyond, but that the glass is too dark to see them. Is there a light beyond the glass, or is it a mirror only to the self? The Platonic cave is even darker than Plato made it, for it introduces the echo, and so leaves us back in the world of men, which does not carry total meaning, is just a story of events.”
— Betty Jay, op. cit.
Judy Davis in the Marabar Caves
In mathematics
(as opposed to narrative),
somewhere between
a flagrant triviality and
a resplendent Trinity we
have what might be called
“a resplendent triviality.”
For further details, see
“A Four-Color Theorem.”
Comments Off on Sunday April 13, 2008
Thursday, March 6, 2008
Thursday March 6, 2008
This note is prompted by the March 4 death of Richard D. Anderson, writer on geometry, President (1981-82) of the Mathematical Association of America (MAA), and member of the MAA's Icosahedron Society.
Royal Road
"The historical road
from the Platonic solids
to the finite simple groups
is well known."
— Steven H. Cullinane,
November 2000,
Symmetry from Plato to
the Four-Color Conjecture
Euclid is said to have remarked that "there is no royal road to geometry." The road to the end of the four-color conjecture may, however, be viewed as a royal road from geometry to the wasteland of mathematical recreations.* (See, for instance, Ch. VIII, "Map-Colouring Problems," in Mathematical Recreations and Essays, by W. W. Rouse Ball and H. S. M. Coxeter.) That road ended in 1976 at the AMS-MAA summer meeting in Toronto– home of H. S. M. Coxeter, a.k.a. "the king of geometry."
A different road– from Plato to the finite simple groups– is, as I noted in November 2000, well known. But new roadside attractions continue to appear. One such attraction is the role played by a Platonic solid– the icosahedron– in design theory, coding theory, and the construction of the sporadic simple group M24.
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," by Andries E. Brouwer (Ch. 14 (pp. 693-746) of Handbook of Combinatorics, Vol. I, MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel, and László Lovász, Section 16 (p. 716))
This Steiner system is closely connected to M24 and to the extended binary Golay code. Brouwer gives an elegant construction of that code (and therefore of M24):
"Let N be the adjacency matrix of the icosahedron (points: 12 vertices, adjacent: joined by an edge). Then the rows of the 12×24 matrix
— Op. cit., p. 719
Related material:
* For a road out of this wasteland, back to geometry, see The Kaleidoscope Puzzle and Reflection Groups in Finite Geometry.
Finite Geometry of
the Square and Cube
and
Jewel in the Crown
"There is a pleasantly discursive
treatment of Pontius Pilate's
unanswered question
'What is truth?'"
— H. S. M. Coxeter, 1987,
introduction to Trudeau's
"story theory" of truth
Those who prefer stories to truth
may consult the Log24 entries
of March 1, 2, 3, 4, and 5.
They may also consult
the poet Rubén Darío:
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
* For a road out of this wasteland, back to geometry, see The Kaleidoscope Puzzle and Reflection Groups in Finite Geometry.
Comments Off on Thursday March 6, 2008
Wednesday, March 5, 2008
Wednesday March 5, 2008
(Context: March 2-4)
For CENTRAL
Central Intelligence:
"God does not play dice."
— Paraphrase of a remark
by Albert Einstein
Another Nobel Prize winner,
Isaac Bashevis Singer—
"a God who speaks in deeds,
not in words, and whose
vocabulary is the Cosmos"
From "The Escapist:
The Reality of Fantasy Games"–
Dungeons & Dragons Dice
From today's New York Times:
A Kaddish for Gygax:
| "I was reading Durant's section on Plato, struggling to understand his theory of the ideal Forms that lay in inviolable perfection out beyond the phantasmagoria. (That was the first, and I think the last, time that I encountered that word.)" |
Related material:
For more on the word
"phantasmagoria," see
Log24 on Dec. 12, 2004
and on Sept. 23, 2006.
For phantasmagoria in action,
see Dungeons & Dragons
and Singer's (and others')
Jewish fiction.
For non-phantasmagoria,
see (for instance) the Elements
of Euclid, which culminates
in the construction of the
Platonic solids illustrated above.
See also Geometry for Jews.
Comments Off on Wednesday March 5, 2008
Wednesday, October 3, 2007
Wednesday October 3, 2007
Janitor Monitor
Will Hunting may be
interested in the following
vacant editorships at
The Open Directory:
Graph Theory
and
Combinatorics.
Related material:
The Long Hello and
On the Holy Trinity —
"Hey, Carrie-Anne, what's
your game now….?"
Picture sources:
azstarnet.com,
vibrationdata.com.
Personally, I prefer
Carol Ann:
From Criticism, Fall, 2001,
by Carol Ann Johnston—
|
"Drawing upon Platonic thought, Augustine argues that ideas are actually God's objective pattern and as such exist in God's mind. These ideas appear in the mirror of the soul. (35)." (35.) In Augustine, De Trinitate, trans., Stephen McKenna (Washington, D.C.: Catholic University Press, 1970). See A. B. Acton, "Idealism," in The Encyclopedia of Philosophy, ed., Paul Edwards. Vol. 4 (New York: Macmillan, 1967): 110-118; Robert McRae, "`Idea' as a Philosophical Term in the Seventeenth Century," JHI 26 (1965): 175-190, and Erwin Panofsky, Idea: A Concept in Art History, trans., Joseph J. S. Peake (Columbia, S.C.: University of South Carolina Press, 1968) for explications of this term. |
See also
Art Wars: Geometry as Conceptual Art
and Ideas and Art: Notes on Iconology.
Art Wars: Geometry as Conceptual Art
and Ideas and Art: Notes on Iconology.
For more on Augustine and geometry,
see Today's Sinner (Aug. 28, 2006).
Comments Off on Wednesday October 3, 2007
Tuesday, July 24, 2007
Tuesday July 24, 2007
The Church of St. Frank
See yesterday’s entries for
some relevant quotations
from Wallace Stevens.
Further quotations for what
Marjorie Garber, replying to
a book review by
Frank Kermode, has called
“the Church of St. Frank“–
Frank Kermode on
Harold Bloom:
“He has… a great, almost
selfish passion for poetry,
and he interprets difficult
texts as if there were no
more important activity
in the world, which may
be right.”
Page 348 of Wallace Stevens:
The Poems of Our Climate,
by Harold Bloom
(1977, Cornell U. Press):
“The fiction of the leaves is now Stevens’ fiction…. Spring, summer, and autumn adorn the rock of reality even as a woman is adorned, the principle being the Platonic one of copying the sun as source of all images….
… They are more than leaves
that cover the barren rock….
They bear their fruit
so that the year is known….
If they are more than leaves, then they are no longer language, and the leaves have ceased to be tropes or poems and have become magic or mysticism, a Will-to-Power over nature rather than over the anteriority of poetic imagery.”
For more on magic, mysticism, and the Platonic “source of all images,” see Scott McLaren on “Hermeticism and the Metaphysics of Goodness in the Novels of Charles Williams.” McLaren quotes Evelyn Underhill on magic vs. mysticism:
The fundamental difference between the two is this: magic wants to get, mysticism wants to give […] In mysticism the will is united with the emotions in an impassioned desire to transcend the sense-world in order that the self may be joined by love to the one eternal and ultimate Object of love […] In magic, the will unites with the intellect in an impassioned desire for supersensible knowledge. This is the intellectual, aggressive, and scientific temperament trying to extend its field of consciousness […] (Underhill 84; see also 178ff.)
— Underhill, Evelyn. Mysticism: A Study in the Nature and Development of Man’s Spiritual Consciousness. New York: Dutton, 1911.
For more on what Bloom calls the “Will-to-Power over nature,” see Faust in Copenhagen and the recent (20th- and 21st-century) history of Harvard University. These matters are also discussed in “Log24 – Juneteenth through Midsummer Night.”
For more on what Underhill calls “the intellectual, aggressive, and scientific temperament trying to extend its field of consciousness,” see the review, in the August 2007 Notices of the American Mathematical Society, of a book by Douglas Hofstadter– a writer on the nature of consciousness— by magician Martin Gardner.
Comments Off on Tuesday July 24, 2007
Thursday, July 5, 2007
Thursday July 5, 2007
In Defense of
Plato’s Realism
(vs. sophists’ nominalism–
see recent entries.)
Plato cited geometry,
notably in the Meno,
in defense of his realism.
Consideration of the
Meno’s diamond figure
leads to the following:
Click on image for details.
As noted in an entry,
Plato, Pegasus, and
the Evening Star,
linked to
at the end of today’s
previous entry,
the “universals”
of Platonic realism
are exemplified by
the hexagrams of
the I Ching,
which in turn are
based on the seven
trigrams above and
on the eighth trigram,
of all yin lines,
not shown above:
K’un
The Receptive
_____________________________________________
Update of Nov. 30, 2013:
From a little-known website in Kuala Lumpur:
(Click to enlarge.)
The remarks on Platonic realism are from Wikipedia.
The eightfold cube is apparently from this post.
Comments Off on Thursday July 5, 2007
Monday, June 25, 2007
Monday June 25, 2007
Object Lesson
"… the best definition
I have for Satan
is that it is a real
spirit of unreality."
"… the best definition
I have for Satan
is that it is a real
spirit of unreality."
M. Scott Peck,
People of the Lie
|
"Far in the woods they sang their unreal songs, Secure. It was difficult to sing in face Of the object. The singers had to avert themselves Or else avert the object."
— Wallace Stevens, |
Today is June 25,
anniversary of the
birth in 1908 of
Willard Van Orman Quine.
Quine died on
Christmas Day, 2000.
Today, Quine's birthday, is,
as has been noted by
Quine's son, the point of the
calendar opposite Christmas–
i.e., "AntiChristmas."
If the Anti-Christ is,
as M. Scott Peck claims,
a spirit of unreality, it seems
fitting today to invoke
Quine, a student of reality,
and to borrow the title of
Quine's Word and Object…
Word:
An excerpt from
"Credences of Summer"
by Wallace Stevens:
|
"Three times the concentred self takes hold, three times The thrice concentred self, having possessed
The object, grips it
— "Credences of Summer," VII, |
Object:
From Friedrich Froebel,
who invented kindergarten:
From Christmas 2005:
Click on the images
for further details.
For a larger and
more sophisticaled
relative of this object,
see yesterday's entry
At Midsummer Noon.
The object is real,
not as a particular
physical object, but
in the way that a
mathematical object
is real — as a
pure Platonic form.
"It's all in Plato…."
— C. S. Lewis
Comments Off on Monday June 25, 2007
Wednesday, May 23, 2007
Wednesday May 23, 2007
Strong Emergence Illustrated:
The Beauty Test
"There is no royal road
to geometry"
to geometry"
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
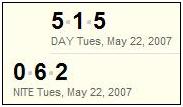
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):
The illustration (and definition) is
of regular tessellations of the plane.
of regular tessellations of the plane.
This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:
One might argue that such beauty is strongly emergent because of the "harmonious way" the parts fit together: the regularity (or fitting together) of the whole is not reducible to the regularity of the parts. (Regular triangles, squares, and hexagons fit together, but regular pentagons do not.)
The symmetries of these regular tessellations of the plane are less well suited as illustrations of emergence, since they are tied rather closely to symmetries of the component parts.
But the symmetries of regular tessellations of the sphere— i.e., of the five Platonic solids– do emerge strongly, being apparently independent of symmetries of the component parts.
Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
Comments Off on Wednesday May 23, 2007
Tuesday, May 22, 2007
Tuesday May 22, 2007
Jewel in the Crown
The Crown of Geometry
(according to Logothetti
in a 1980 article)
The crown jewels are the
Platonic solids, with the
icosahedron at the top.
Related material:
"[The applet] Syntheme illustrates ways of partitioning the 12 vertices of an icosahedron into 3 sets of 4, so that each set forms the corners of a rectangle in the Golden Ratio. Each such rectangle is known as a duad. The short sides of a duad are opposite edges of the icosahedron, and there are 30 edges, so there are 15 duads.
Each partition of the vertices into duads is known as a syntheme. There are 15 synthemes; 5 consist of duads that are mutually perpendicular, while the other 10 consist of duads that share a common line of intersection."
— Greg Egan, Syntheme
Duads and synthemes
(discovered by Sylvester)
also appear in this note
from May 26, 1986
(click to enlarge):
(discovered by Sylvester)
also appear in this note
from May 26, 1986
(click to enlarge):
The above note shows
duads and synthemes related
to the diamond theorem.
See also John Baez's essay
"Some Thoughts on the Number 6."
That essay was written 15 years
ago today– which happens
to be the birthday of
Sir Laurence Olivier, who,
were he alive today, would
be 100 years old.
"Is it safe?"
Comments Off on Tuesday May 22, 2007
Wednesday, December 13, 2006
Wednesday December 13, 2006
Best Wishes for a
C. S. Lewis
Christmas
Image of Lewis from |
“What on earth is a concrete universal?” — Robert M. Pirsig, author of Zen and the Art of Motorcyle Maintenance For one approach to an answer, click on the picture at left. |
Update of 4:23 PM:
The Lewis link above deals with the separation of Heaven from Hell. The emphasis is on Heaven. A mysterious visitor to this website, “United States,” seems to be seeking equal time for Hell. And so…
Storyboard
Based on Xanga footprints of Dec. 13, 2006
from m759’s site-visitor “United States”
(possibly a robot; if so, a robot with strange tastes).“How much story do you want?”
— George Balanchine
Based on Xanga footprints of Dec. 13, 2006
from m759’s site-visitor “United States”
(possibly a robot; if so, a robot with strange tastes).
TIME OF DATE OF PAGE VISITED
VISIT PAGE VISITED
1217 040520 Parable
1218 060606 The Omen
1220 051205 Don’t Know Much About History
1225 030822 Mr. Holland’s Week (And in Three Days…)
1233 030114 Remarks on Day 14 (What is Truth?)
1238 040818 Train of Thought (Oh, My Lolita)
1244 020929 Angel Night (Ellis Larkins)
1249 040715 Identity Crisis (Bourne and Treadstone)
1252 050322 Make a Differance (Lacan, Derrida, Reba)
1255 050221 Quarter to Three on Night of HST’s death
1256 040408 Triple Crown on Holy Thursday
1258 040714 Welcome to Mr. Motley’s Neighborhood
1258 030221 All About Lilith
0103 040808 Quartet (for Alexander Hammid)
0104 030106 Dead Poet in the City of Angels
0109 030914 Skewed Mirrors (Readings on Aesthetics)
0110 050126 A Theorem in Musical Form
0125 021007 Music for R. D. Laing
0138 020806 Butterflies & Popes (Transfiguration)
0140 060606 The Omen (again)
0156 030313 ART WARS: Perennial Tutti-Frutti
0202 030112 Ask Not (A Bee Gees Requiem)
0202 050527 Drama of the Diagonal, Part Deux
0202 060514 STAR WARS continued (Eclipse and Venus)
0207 030112 Ask Not (again… Victory of the Goddess)
0207 030221 All About Lilith (again… Roll credits.)
— George Balanchine
Sunday, October 8, 2006
Sunday October 8, 2006
Today’s Birthday:
Matt Damon
Matt Damon
“The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast.”
— G. K. Chesterton
 Natasha Wescoat, 2004 Shakespearean Fool |
|
| Not to mention Euclid and Picasso | |
 |
|
(Click on pictures for details. Euclid is represented by Alexander Bogomolny, Picasso by Robert Foote.)
|
|
See also works by the late Arthur Loeb of Harvard’s Department of Visual and Environmental Studies.
“I don’t want to be a product of my environment. I want my environment to be a product of me.” — Frank Costello in The Departed
For more on the Harvard environment,
see today’s online Crimson:
| The Harvard Crimson, Online Edition |
Sunday, Oct. 8, 2006 |
|
POMP AND
Friday, Oct. 6: The Ringling Bros. Barnum & Bailey Circus has come to town, and yesterday the animals were disembarked near MIT and paraded to their temporary home at the Banknorth Garden. |
OPINION At Last, a By THE CRIMSON STAFF The Trouble By SAHIL K. MAHTANI |
Comments Off on Sunday October 8, 2006
Monday, September 18, 2006
Monday September 18, 2006
Apology
| Excerpts from Log 24, January 18, 2004: A Living Church "Plato has told you a truth; but Plato is dead. Shakespeare has startled you with an image; but Shakespeare will not startle you with any more. But imagine what it would be to live with such men still living. To know that Plato might break out with an original lecture to-morrow, or that at any moment Shakespeare might shatter everything with a single song. The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast. He is always expecting to see some truth that he has never seen before." — G. K. Chesterton, Orthodoxy C. P. Snow on G. H. Hardy in the foreword to A Mathematician's Apology: "… he had another favourite entertainment…." … If, as Chesterton might surmise, he… met Plato and Shakespeare in Heaven, the former might discuss with him the eternal Platonic form of the number 17*, while the latter might offer…. * Footnote of 9/18/06: For the Platonic form of 17, see Feast of the Triumph of the Cross (9/14/06) and Medal (9/15/06). |
A Living Church,
continued…
Apology:
An Exercise in Rhetoric
An Exercise in Rhetoric
Related material:
|
ON 6-6-6 —
"Seamus Davey-Fitzpatrick stars in a scene from the R-rated movie 'The Omen.' An official of the Australian bishops conference took on the superstition surrounding the movie's release date of June 6, 2006, noting that 'I take evil far too seriously to think "The Omen" is telling me anything realistic or important.'" (CNS/20th Century Fox) |
and
 |
Comments Off on Monday September 18, 2006
Monday, August 28, 2006
Monday August 28, 2006
Today's Sinner:
Augustine of Hippo, who is said to
have died on this date in 430 A.D.
"He is, after all, not merely taking over a Neoplatonic ontology, but he is attempting to combine it with a scriptural tradition of a rather different sort, one wherein the divine attributes most prized in the Greek tradition (e.g. necessity, immutability, and atemporal eternity) must somehow be combined with the personal attributes (e.g. will, justice, and historical purpose) of the God of Abraham, Isaac, and Jacob."
— Stanford Encyclopedia of Philosophy on Augustine
Here is a rather different attempt
to combine the eternal with the temporal:
|
The Eternal
Symbol of necessity,
For details, see |
The Temporal
Symbol of the
For details, see |
|
The eternal
combined with the temporal:
|
|
Related material:
Comments Off on Monday August 28, 2006
Friday, May 26, 2006
Friday May 26, 2006
A Living Church
continued from March 27
"The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast."
— G. K. Chesterton
Shakespearean Fool |
Related material:
Yesterday's entries
and their link to
The Line
as well as
and the remarks
of Oxford professor
Marcus du Sautoy,
who claims that
"the right side of the brain
is responsible for mathematics."
Let us hope that Professor du Sautoy
is more reliable on zeta functions,
his real field of expertise,
than on neurology.
The picture below may help
to clear up his confusion
between left and right.
His confusion about
pseudoscience may not
be so easily remedied.
flickr.com/photos/jaycross/3975200/
(Any resemblance to the film
"Hannibal" is purely coincidental.)
Comments Off on Friday May 26, 2006
Wednesday, November 30, 2005
Wednesday November 30, 2005
Hobgoblin?
Brian Davies is a professor of mathematics at King’s College London. In the December Notices of the American Mathematical Society, he claims that arithmetic may, for all we know, be inconsistent:
“Gödel taught us that it is not possible to prove that Peano arithmetic is consistent, but everyone has taken it for granted that in fact it is indeed consistent.
Platonistically-inclined mathematicians would deny the possibility that Peano arithmetic could be flawed. From Kronecker onwards many consider that they have a direct insight into the natural numbers, which guarantees their existence. If the natural numbers exist and Peano’s axioms describe properties that they possess then, since the axioms can be instantiated, they must be consistent.”
Platonistically-inclined mathematicians would deny the possibility that Peano arithmetic could be flawed. From Kronecker onwards many consider that they have a direct insight into the natural numbers, which guarantees their existence. If the natural numbers exist and Peano’s axioms describe properties that they possess then, since the axioms can be instantiated, they must be consistent.”
“It is not possible to prove that Peano arithmetic is consistent”…?!
Where did Gödel say this? Gödel proved, in fact, according to a well-known mathematician at Princeton, that (letting PA stand for Peano Arithmetic),
“If PA is consistent, the formula expressing ‘PA is consistent’ is unprovable in PA.”
— Edward Nelson,
Mathematics and Faith (pdf)
Remarkably, even after he has stated correctly Gödel’s result, Nelson, like Davies, concludes that
“The consistency of PA cannot be concretely demonstrated.”
I prefer the argument that the existence of a model ensures the consistency of a theory.
For instance, the Toronto philosopher William Seager writes that
“Our judgement as to the consistency of some system is not dependent upon that system’s being able to prove its own consistency (i.e. generate a formula that states, e.g. ‘0=1’ is not provable). For if that was the sole basis, how could we trust it? If the system was inconsistent, it could generate this formula as well (see Smullyan, Gödel’s Incompleteness Theorems, (Oxford, 1992, p. 109)). Furthermore, [George] Boolos allows that we do know that certain systems, such as Peano Arithmetic, are consistent even though they cannot prove their own consistency. Presumably, we know this because we can see that a certain model satisfies the axioms of the system at issue, hence that they are true in that model and so must be consistent.”
The relationship between consistency and the existence of a model is brought home by the following weblog entry that neatly summarizes a fallacious argument offered in the AMS Notices by Davies:
The following is an interesting example that I came across in the article “Whither Mathematics?” by Brian Davies in the December issue of Notices of the American Mathematical Society.
Consider the following list A1 of axioms.
(1) There is a natural number 0.
(2) Every natural number a has a successor, denoted by S(a).
(3) There is no natural number whose successor is 0.
(4) Distinct natural numbers have distinct successors: a = b if and only if S(a) = S(b).
(5) If a property is possessed by 0 and also by the successor of every natural number which possesses it, then it is possessed by all the natural numbers.
Now consider the following list A2 of axioms.
(1) G is a set of elements and these elements obey the group axioms.
(2) G is finite but not isomorphic to any known list of finite simple groups.
(3) G is simple, in other words, if N is a subset of G satisfying certain properties then N=G.
We can roughly compare A2 with A1. The second axiom in A2 can be thought of as analogous to the third axiom of A1. Also the third axiom of A2 is analogous to the fifth axiom of A1, insofar as it refers to an unspecified set with cetain properties and concludes that it is equal to G.
Now, as is generally believed by most group theorists, the system A2 is internally inconsistent and the proof its inconsistency runs for more than 10000 pages.
So who is to deny that the system A1 is also probably internally inconsistent! Particularly since Godel proved that you can not prove it is consistent (staying inside the system). May be the shortest proof of its inconsistency is one hundred million pages long!
— Posted by Krishna,
11/29/2005 11:46:00 PM,
at his weblog,
“Quasi-Coherent Ruminations”
An important difference between A1 (the set of axioms of Peano arithmetic) and A2 (a set of axioms that describe a new, unknown, finite simple group) is that A1 is known to have a model (the nonnegative integers) and A2 is not known to have a model.
Therefore, according to Seager’s argument, A1 is consistent and A2 may or may not be consistent.
The degree to which Seager’s argument invokes Platonic realism is debatable. Less debatable is the quasireligious faith in nominalism proclaimed by Davies and Nelson. Nelson’s own account of a religious experience in 1976 at Toronto is instructive.
I must relate how I lost my faith in Pythagorean numbers. One morning at the 1976 Summer Meeting of the American Mathematical Society in Toronto, I woke early. As I lay meditating about numbers, I felt the momentary overwhelming presence of one who convicted me of arrogance for my belief in the real existence of an infinite world of numbers, leaving me like an infant in a crib reduced to counting on my fingers. Now I live in a world in which there are no numbers save those that human beings on occasion construct.
— Edward Nelson,
Mathematics and Faith (pdf)
Nelson’s “Mathematics and Faith” was written for the Jubilee for Men and Women from the World of Learning held at the Vatican, 23-24 May 2000. It concludes with an invocation of St. Paul:
During my first stay in Rome I used to play chess with Ernesto Buonaiuti. In his writings and in his life, Buonaiuti with passionate eloquence opposed the reification of human abstractions. I close by quoting one sentence from his Pellegrino di Roma. “For [St. Paul] abstract truth, absolute laws, do not exist, because all of our thinking is subordinated to the construction of this holy temple of the Spirit, whose manifestations are not abstract ideas, but fruits of goodness, of peace, of charity and forgiveness.”
— Edward Nelson,
Mathematics and Faith (pdf)
Belief in the consistency of arithmetic may or may not be foolish, and therefore an Emersonian hobgoblin of little minds, but bullshit is bullshit, whether in London, in Princeton, in Toronto, or in Rome.
Comments Off on Wednesday November 30, 2005
Thursday, November 3, 2005
Thursday November 3, 2005
Bond
USA Today on last night’s White House dinner:
“In his toast, Bush said the royal visit was ‘a reminder of the unique and enduring bond’ between the two countries.”
| From Log24, July 18, 2003:
The use of the word “idea” in my entries’ headlines yesterday was not accidental. It is related to an occurrence of the word in Understanding: On Death and Truth, a set of journal entries from May 9-12. The relevant passage on “ideas” is quoted there, within commentary by an Oberlin professor: “That the truth we understand must be a truth we stand under is brought out nicely in C. S. Lewis’ That Hideous Strength when Mark Studdock gradually learns what an ‘Idea’ is. While Frost attempts to give Mark a ‘training in objectivity’ that will destroy in him any natural moral sense, and while Mark tries desperately to find a way out of the moral void into which he is being drawn, he discovers what it means to under-stand.
This too, I fear, is seldom communicated in the classroom, where opinion reigns supreme. But it has important implications for the way we understand argument.” — “On Bringing One’s Life to a Point,” by Gilbert Meilaender, First Things, November 1994 The old philosophical conflict between realism and nominalism can, it seems, have life-and-death consequences. I prefer Plato’s realism, with its “ideas,” such as the idea of seven-ness. A reductio ad absurdum of nominalism may be found in the Stanford Encyclopedia of Philosophy under Realism: “A certain kind of nominalist rejects the existence claim which the platonic realist makes: there are no abstract objects, so sentences such as ‘7 is prime’ are false….” The claim that 7 is not prime is, regardless of its motives, dangerously stupid. |
The New York Lottery evening number
for All Souls’ Day, Nov. 2, 2005, was
007.
Related material:
Entries for Nov. 1, 2005 and
the song Planned Obsolescence
by the 10,000 Maniacs
Comments Off on Thursday November 3, 2005
Thursday, August 11, 2005
Thursday, January 27, 2005
Thursday January 27, 2005
Crystal Night
Mies van der Rohe:
Mies in Berlin
Winner of
The Society of Architectural Historians
2002 Philip Johnson Award
for Excellence
Exhibition Catalog
"Published to accompany
a groundbreaking 2001 exhibition at
The Museum of Modern Art, New York."
a groundbreaking 2001 exhibition at
The Museum of Modern Art, New York."
"It would have been wiser for the new MoMA catalog… to have addressed the issue of his politics…. By ignoring such a central subject… the show gives off a mild stench of cover-up…. Only the German-born Rosemarie Haag Bletter (full disclosure: she is my wife) alludes to the verboten topic in her [catalog] essay on Mies's flirtation with crystal imagery, drawing a sharp parallel between the architect's extensive use of Kristallglas (plate glass) and the ensuing devastation of Kristallnacht, which erupted just three months after he left for the States."
Also from Filler's essay:
"Mies's rigorously simplified structures, typified by grids of steel and glass and an absence of applied ornament, represented the Platonic ideal of modernism for many people."
For more on aesthetics, see the
Log24.net entry of Tuesday noon,
Log24.net entry of Tuesday noon,
For more on a Platonic ideal of sorts,
see the following figure in two versions:
see the following figure in two versions:
Version A, from Plato's Meno and
Diamond Theory,
Diamond Theory,
and Version B,
from the date of Johnson's death
at his "famous crystalline box."
Was less more?
Comments Off on Thursday January 27, 2005
Sunday, January 18, 2004
Sunday January 18, 2004
A Living Church
"Plato has told you a truth; but Plato is dead. Shakespeare has startled you with an image; but Shakespeare will not startle you with any more. But imagine what it would be to live with such men still living. To know that Plato might break out with an original lecture to-morrow, or that at any moment Shakespeare might shatter everything with a single song. The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast. He is always expecting to see some truth that he has never seen before."
— G. K. Chesterton, Orthodoxy
C. P. Snow on G. H. Hardy in the foreword to A Mathematician's Apology:
"… he had another favourite entertainment. 'Mark that man we met last night,' he said, and someone had to be marked out of 100 in each of the categories Hardy had long since invented and defined. STARK, BLEAK ('a stark man is not necessarily bleak: but all bleak men without exception want to be considered stark')…."
S. H. Cullinane on religion and Hollywood:
"If the incomparable Max Bialystock were to remake 'Up Close and Personal,' he might retitle it 'Distant and Impersonal.' A Google search on this phrase suggests
a plot outline for Mel Brooks & Co."
In memory of
producer Ray Stark,
an excerpt from that plot outline:
The Oxford University Press summary of
God:
Myths of the Male Divine,
by David Leeming and Jake Page
"They [Leeming and Page] describe the rise of a male sky God as 'the equal to, the true mate, of Goddess, who was still associated with Earth.' In the Iron Age, the sky God became more aggressive, separating from the Goddess and taking his place as the King God, as Zeus, Odin, and Horus. Ultimately he emerged as the creator, a more distant and impersonal force. Here Leeming and Page also illuminate an important trend–a sense that the divine is beyond gender, that it permeates all things (as seen in the Chinese Tao and En Sof of the Kabbalah). They see a movement in the biography of God toward a reunion with the Goddess."
As for the Goddess, see
(December 17, 2002).
Stark, a saint among Hollywood producers, died yesterday, January 17. If, as Chesterton might surmise, he then met Plato and Shakespeare in Heaven, the former might discuss with him the eternal Platonic form of the number 17, while the latter might offer the following links on Stark's new heavenly laptop:
This concludes the tribute to Stark. For a tribute to Bleak, click here.
Comments Off on Sunday January 18, 2004
Saturday, July 26, 2003
Saturday July 26, 2003
The Transcendent
Signified
“God is both the transcendent signifier
and transcendent signified.”
— Caryn Broitman,
Deconstruction and the Bible
“Central to deconstructive theory is the notion that there is no ‘transcendent signified,’ or ‘logos,’ that ultimately grounds ‘meaning’ in language….”
— Henry P. Mills,
The Significance of Language,
Footnote 2
“It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy. It structures Plato’s (realist) reaction to the sophists (nominalists). What is often called ‘postmodernism’ is really just nominalism, colourfully presented as the doctrine that there is nothing except texts. It is the variety of nominalism represented in many modern humanities, paralysing appeals to reason and truth.”
— Simon Blackburn, Think,
Oxford University Press, 1999, page 268
The question of universals is still being debated in Paris. See my July 25 entry,
That entry discusses an essay on
mathematics and postmodern thought
by Michael Harris,
professor of mathematics
at l’Université Paris 7 – Denis Diderot.
A different essay by Harris has a discussion that gets to the heart of this matter: whether pi exists as a platonic idea apart from any human definitions. Harris notes that “one might recall that the theorem that pi is transcendental can be stated as follows: the homomorphism
Harris illustrates this with
an X in a rectangle:
For the complete passage, click here.
If we rotate the Harris X by 90 degrees, we get a representation of the Christian Logos that seems closely related to the God-symbol of Arthur C. Clarke and Stanley Kubrick in 2001: A Space Odyssey. On the left below, we have a (1x)4×9 black monolith, representing God, and on the right below, we have the Harris slab, with X representing (as in “Xmas,” or the Chi-rho page of the Book of Kells) Christ… who is, in theological terms, also “the variable par excellence.”
|
Kubrick’s |
Harris’s |
For a more serious discussion of deconstruction and Christian theology, see
Comments Off on Saturday July 26, 2003
Friday, July 18, 2003
Friday July 18, 2003
Hideous Strength
On a Report from London:
Assuming rather prematurely that the body found in Oxfordshire today is that of David Kelly, Ministry of Defence germ-warfare expert and alleged leaker of information to the press, the Financial Times has the following:
“Mr Kelly’s death has stunned all the players involved in this drama, resembling as it does a fictitious political thriller.”
— Financial Times, July 18,
2003, 19:06 London time
I feel it resembles rather a fictitious religious thriller… Namely, That Hideous Strength, by C. S. Lewis. The use of the word “idea” in my entries’ headlines yesterday was not accidental. It is related to an occurrence of the word in Understanding: On Death and Truth, a set of journal entries from May 9-12. The relevant passage on “ideas” is quoted there, within commentary by an Oberlin professor:
“That the truth we understand must be a truth we stand under is brought out nicely in C. S. Lewis’ That Hideous Strength when Mark Studdock gradually learns what an ‘Idea’ is. While Frost attempts to give Mark a ‘training in objectivity’ that will destroy in him any natural moral sense, and while Mark tries desperately to find a way out of the moral void into which he is being drawn, he discovers what it means to under-stand.
‘He had never before known what an Idea meant: he had always thought till now that they were things inside one’s own head. But now, when his head was continually attacked and often completely filled with the clinging corruption of the training, this Idea towered up above him-something which obviously existed quite independently of himself and had hard rock surfaces which would not give, surfaces he could cling to.’
This too, I fear, is seldom communicated in the classroom, where opinion reigns supreme. But it has important implications for the way we understand argument.”
— “On Bringing One’s Life to a Point,” by Gilbert Meilaender, First Things, November 1994
The old philosophical conflict between realism and nominalism can, it seems, have life-and-death consequences. I prefer Plato’s realism, with its “ideas,” such as the idea of seven-ness. A reductio ad absurdum of nominalism may be found in the Stanford Encyclopedia of Philosophy under Realism:
“A certain kind of nominalist rejects the existence claim which the platonic realist makes: there are no abstract objects, so sentences such as ‘7 is prime’ are false….”
The claim that 7 is not prime is, regardless of its motives, dangerously stupid… A quality shared, it seems, by many in power these days.
Comments Off on Friday July 18, 2003
Tuesday, February 11, 2003
Tuesday February 11, 2003
St. John von Neumann's Song
The mathematician John von Neumann, a heavy drinker and party animal, advocated a nuclear first strike on Moscow.* Confined to a wheelchair before his death, he was, some say, the inspiration for Kubrick's Dr. Strangelove. He was a Jew converted to Catholicism. His saint's day was February 8. Here is an excerpt from a book titled Abstract Harmonic Analysis**, just one of the fields illuminated by von Neumann's brilliance:
"…von Neumann showed that an intrinsic definition can be given for the mean M(f) of an almost periodic function…. Von Neumann proved the existence and properties of M(f) by completely elementary methods…."
Should W. B. Yeats wander into the Catholic Anticommunists' section of Paradise, he might encounter, as in "Sailing to Byzantium," an unexpected set of "singing-masters" there: the Platonic archetypes of the Hollywood Argyles.
The Argyles' attire is in keeping with Yeats's desire for gold in his "artifice of eternity"… In this case, gold lamé, but hey, it's Hollywood. The Argyles' lyrics will no doubt be somewhat more explicit in heaven. For instance, in "Alley Oop," the line
"He's a mean motor scooter and a bad go-getter"
will in its purer heavenly version be rendered
"He's a mean M(f)er and…"
in keeping with von Neumann's artifice of eternity described above.
This theological meditation was suggested by previous entries on Yeats, music and Catholicism (see Feb. 8, von Neumann's saint's day) and by the following recent weblog entries of a Harvard senior majoring in mathematics:
"I changed my profile picture to Oedipus last night because I felt cursed by fate…."
"It's not rational for me to believe that I am cursed, that the gods are set against me. Because I don't even believe in any gods!"
The spiritual benefits of a Harvard education are summarized by this student's new profile picture:
M(f)
*Source: Von Neumann and the Development of Game Theory
**by Harvard professor Lynn H. Loomis, Van Nostrand, 1953, p. 169.
Monday, December 30, 2002
Monday December 30, 2002
Homer
“No matter how it’s done, you won’t like it.”
— Robert Redford to Robert M. Pirsig in Lila
“The evening before Harriet injures Roy,
she asks him, in a restaurant car,
whether he has read Homer.”
— Oxford website on the film of The Natural
“Brush Up Your Shakespeare”
— Cole Porter lyric for a show that opened
on December 30, 1948
Judy Davis as Harriet Bird
Thine eyes I love…
Shakespeare, Sonnet 132
“Roy’s Guenevere-like lover is named Memo Paris,
presumably the face that launched a thousand strikes.”
— Oxford website on the film of The Natural
Nicole Kidman
as Memo Paris
“Iris is someone to watch over Roy.”
— Oxford website on the film of The Natural
Kate Winslet as young Iris Murdoch
From the second-draft screenplay
for The Sting,
with Robert Redford as Hooker:
HOOKER
(shuffling a little)
I, ah…thought you might wanna come out for a while. Maybe have a drink or somethin’.
LORETTA
You move right along, don’t ya.
HOOKER
(with more innocence than confidence)
I don’t mean nothin’ by it. I just don’t know many regular girls, that’s all.
LORETTA
And you expect me to come over, just like that.
HOOKER
If I expected somethin’, I wouldn’t be still standin’ out here in the hall.
Loretta looks at him carefully. She knows it’s not a line.
LORETTA
(with less resistance now)
I don’t even know you.
HOOKER
(slowly)
You know me. I’m just like you…
It’s two in the morning and I don’t know nobody.
The two just stand there in silence a second. There’s nothing more to say. She stands back and lets him in.
Iris Murdoch on Plato’s Form of the Good,
by Joseph Malikail:
“For Murdoch as for Plato, the Good belongs to Plato’s Realm of Being not the Realm of Becoming…. However, Murdoch does not read Plato as declaring his faith in a divine being when he says that the Good is
the universal author of all things beautiful and right, parent of light and the lord of light in the visible world, and the immediate source of reason and truth in the intellectual; and that this is the power upon which [one who] would act rationally either in public or private life must have his eyes fixed (Republic…).
Though she acknowledges the influence of Simone Weil in her reading of Plato, her understanding of Plato on Good and God is not Weil’s (1952, ch.7)*. For Murdoch,
Plato never identified his Form of the Good with God (the use of theos in the Republic… is a façon de parler), and this separation is for him an essential one. Religion is above the level of the ‘gods.’ There are no gods and no God either. Neo-Platonic thinkers made the identification (of God with good) possible; and the Judaeo-Christian tradition has made it easy and natural for us to gather together the aesthetic and consoling impression of Good as a person (1992, 38)**.
As she understands Plato:
The Form of the Good as creative power is not a Book of Genesis creator ex nihilo … Plato does not set up the Form of the Good as God, this would be absolutely un-Platonic, nor does he anywhere give the sign of missing or needing a real God to assist his explanations. On the contrary, Good is above the level of the gods or God (ibid., 475)**.
Mary Warnock, her friend and fellow-philosopher, sums up Murdoch’s metaphysical view of the Vision of the Good:
She [Murdoch] holds that goodness has a real though abstract existence in the world. The actual existence of goodness is, in her view, the way it is now possible to understand the idea of God.
Or as Murdoch herself puts it, ‘Good represents the reality of which God is the dream.’ (1992, 496)**”
*Weil, Simone. 1952. Intimations of Christianity Among The Ancient Greeks. Ark Paperbacks, 1987/1952.
**Murdoch, Iris. 1992. Metaphysics As A Guide To Morals. London: Chatto and Windus.
From the conclusion of Lila,
by Robert M. Pirsig:
“Good is a noun. That was it. That was what Phaedrus had been looking for. That was the homer over the fence that ended the ballgame.”
Comments Off on Monday December 30, 2002
Monday, December 16, 2002
Monday December 16, 2002
Rebecca Goldstein
at Heaven’s Gate
This entry is in gratitude for Rebecca Goldstein’s
excellent essay
in The New York Times of December 16, 2002.
She talks about the perennial conflict between two theories of truth that Richard Trudeau called the “story theory” and the “diamond theory.” My entry of December 13, 2002, “Rhyme Scheme,” links the word “real” to an article in the Stanford Encyclopedia of Philosophy that contains the following:
“According to a platonist about arithmetic, the truth of the sentence ‘7 is prime’ entails the existence of an abstract object, the number 7. This object is abstract because it has no spatial or temporal location, and is causally inert. A platonic realist about arithmetic will say that the number 7 exists and instantiates the property of being prime independently of anyone’s beliefs, linguistic practices, conceptual schemes, and so on. A certain kind of nominalist rejects the existence claim which the platonic realist makes: there are no abstract objects, so sentences such as ‘7 is prime’ are false…”
This discussion of “sevenness,” along with the discussion of “eightness” in my December 14, 2002, note on Bach, suggest that I supply a transcription of a note in my paper journal from 2001 that deals with these matters.
From a paper journal note of October 5, 2001:
|
The 2001 Silver Cup Award Glynis Johns is 78 today. “Seven is heaven, “There is no highway in the sky.” “Don’t give up until you See also page |
Added 12/17/02: See also
the portrait of Rebecca Goldstein in
Hadassah Magazine
Volume
78
Number 10
(June/July 1997).
For more on the Jewish propensity to
assign mystical significance to numbers, see
Rabbi Zwerin’s Kol Nidre Sermon.
For the significance of “seven” in Judaism, see
Zayin: The Woman of Valor.
For the significance of “eight” in Judaism, see
Chet: The Life Dynamic.
For the cabalistic significance of
“Seven is heaven, Eight is a gate,”
note that Zayin, Seven, signifies
“seven chambers of Paradise”
and that Chet, Eight, signifies
the “gateway to infinity.”
For the significance of the date 12.17, see
Tet: The Concealed Good.
Comments Off on Monday December 16, 2002