Log24

From the current Wikipedia article "Symmetry (physics)"—

"In physics, symmetry includes all features of a physical system that exhibit the property of symmetry—that is, under certain transformations, aspects of these systems are 'unchanged', according to a particular observation. A symmetry of a physical system is a physical or mathematical feature of the system (observed or intrinsic) that is 'preserved' under some change.

A family of particular transformations may be continuous  (such as rotation of a circle) or discrete  (e.g., reflection of a bilaterally symmetric figure, or rotation of a regular polygon). Continuous and discrete transformations give rise to corresponding types of symmetries. Continuous symmetries can be described by Lie groups while discrete symmetries are described by finite groups (see Symmetry group)."….

"A discrete symmetry is a symmetry that describes non-continuous changes in a system. For example, a square possesses discrete rotational symmetry, as only rotations by multiples of right angles will preserve the square's original appearance."

Note the confusion here between continuous (or discontinuous) transformations  and "continuous" (or "discontinuous," i.e. "discrete") groups .

This confusion may impede efforts to think clearly about some pure mathematics related to current physics— in particular, about the geometry of spaces made up of individual units ("points") that are not joined together in a continuous manifold.

For an attempt to forestall such confusion, see Noncontinuous Groups.

For related material, see Erlanger and Galois as well as the opening paragraphs of Diamond Theory—

Symmetry is often described as invariance under a group of transformations. An unspoken assumption about symmetry in Euclidean 3-space is that the transformations involved are continuous.

Diamond theory rejects this assumption, and in so doing reveals that Euclidean symmetry may itself  be invariant under rather interesting groups of non-continuous (and a-symmetric) transformations. (These might be called noncontinuous  groups, as opposed to so-called discontinuous  (or discrete ) symmetry groups. See Weyl's Symmetry .)

For example, the affine group A on the 4-space over the 2-element field has a natural noncontinuous and asymmetric but symmetry-preserving action on the elements of a 4×4 array. (Details)

(Version first archived on March 27, 2002)

Update of Sunday, February 19, 2012—

The abuse of language by the anonymous authors
of the above Wikipedia article occurs also in more
reputable sources. For instance—

Some transformations referred to by Brading and Castellani
and their editees as "discrete symmetries" are, in fact, as
linear transformations of continuous spaces, themselves
continuous  transformations.

This unfortunate abuse of language is at least made explicit
in a 2003 text, Mathematical Perspectives on Theoretical
Physics  (Nirmala Prakash, Imperial College Press)—

"… associated[*] with any given symmetry there always exists
a continuous or a discrete group of transformations….
A symmetry whose associated group is continuous (discrete)
is called a continuous  (discrete ) symmetry ." — Pp. 235, 236

[* Associated how?]

From the conclusion of Weyl's Symmetry —

One example of Weyl's "structure-endowed entity" is a partition of a six-element set into three disjoint two-element sets– for instance, the partition of the six faces of a cube into three pairs of opposite faces.

The automorphism group of this faces-partition contains an order-8 subgroup that is isomorphic to the abstract group C₂×C₂×C₂ of order eight–

The action of Klein's simple group of order 168 on the Cayley diagram of C₂×C₂×C₂ in yesterday's post furnishes an example of Weyl's statement that

"… one may ask with respect to a given abstract group: What is the group of its automorphisms…?"

The webpage Galois.us, on Galois matrices , has been created as
a starting point for remarks on the algebra  (as opposed to the geometry)
underlying the rings of matrices mentioned in AMS abstract 79T-A37,
“Symmetry invariance in a diamond ring.”

See also related historical remarks by Weyl and by Atiyah.

"And in the Master's chambers…" — Eagles

Related fiction — Amy + Dinner and today's previous post.

Related non-fiction — a page of pure mathematics —

The title of a recent contribution to a London art-related "Piracy Project" begins with the phrase "The Search for Invariants."

A search for that phrase  elsewhere yields a notable 1944* paper by Ernst Cassirer, "The Concept of Group and the Theory of Perception."

Page 20: "It is a process of objectification, the characteristic nature
and tendency of which finds expression in the formation of invariants."

Cassirer's concepts seem related to Weyl's famous remark that

“Objectivity means invariance with respect to the group of automorphisms.”
—Symmetry  (Princeton University Press, 1952, page 132)

See also this journal on June 23, 2010— "Group Theory and Philosophy"— as well as some Math Forum remarks on Cassirer and Weyl.

Update of 6 to 7:50 PM June 20, 2011—

Weyl's 1952 remark seems to echo remarks in 1910 and 1921 by Cassirer.
See Cassirer in 1910 and 1921 on Objectivity.

Another source on Cassirer, invariance, and objectivity—

The conclusion of Maja Lovrenov's 
"The Role of Invariance in Cassirer’s Interpretation of the Theory of Relativity"—

"… physical theories prove to be theories of invariants
with regard to certain groups of transformations and
it is exactly the invariance that secures the objectivity
of a physical theory."

— SYNTHESIS PHILOSOPHICA 42 (2/2006), pp. 233–241

A search in Weyl's Symmetry  for any reference to Ernst Cassirer yields no results.

* Published in French in 1938.

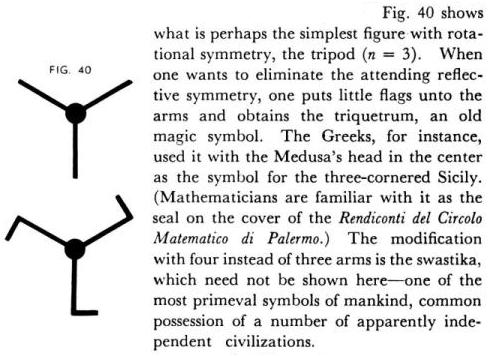
Théorie de l'Ambiguité

According to a 2008 paper by Yves André of the École Normale Supérieure  of Paris—

"Ambiguity theory was the name which Galois used
 when he referred to his own theory and its future developments."

The phrase "the theory of ambiguity" occurs in the testamentary letter Galois wrote to a friend, Auguste Chevalier, on the night before Galois was shot in a duel.

Hermann Weyl in Symmetry, Princeton University Press, 1952—

"This letter, if judged by the novelty and profundity of ideas it contains, is perhaps
  the most substantial piece of writing in the whole literature of mankind."

Conclusion of the Galois testamentary letter, according to
the 1897 Paris edition of Galois's collected works—

The original—

A transcription—

Évariste GALOIS, Lettre-testament, adressée à Auguste Chevalier—

Tu sais mon cher Auguste, que ces sujets ne sont pas les seuls que j'aie
explorés. Mes principales méditations, depuis quelques temps,
étaient dirigées sur l'application à l'analyse transcendante de la théorie de
l'ambiguité. Il s'agissait de voir a priori, dans une relation entre des quantités
ou fonctions transcendantes, quels échanges on pouvait faire, quelles
quantités on pouvait substituer aux quantités données, sans que la relation
put cesser d'avoir lieu. Cela fait reconnaitre de suite l'impossibilité de beaucoup
d'expressions que l'on pourrait chercher. Mais je n'ai pas le temps, et mes idées
ne sont pas encore bien développées sur ce terrain, qui est
immense.

Tu feras imprimer cette lettre dans la Revue encyclopédique.

Je me suis souvent hasardé dans ma vie à avancer des propositions dont je n'étais
pas sûr. Mais tout ce que j'ai écrit là est depuis bientôt un an dans ma
tête, et il est trop de mon intérêt de ne pas me tromper pour qu'on
me soupconne d'avoir énoncé des théorèmes dont je n'aurais pas la démonstration
complète.

Tu prieras publiquement Jacobi et Gauss de donner leur avis,
non sur la vérité, mais sur l'importance des théorèmes.

Après cela, il y aura, j'espère, des gens qui trouveront leur profit
à déchiffrer tout ce gachis.

Je t'embrasse avec effusion.

                                               E. Galois   Le 29 Mai 1832

A translation by Dr. Louis Weisner, Hunter College of the City of New York, from A Source Book in Mathematics, by David Eugene Smith, Dover Publications, 1959–

You know, my dear Auguste, that these subjects are not the only ones I have explored. My reflections, for some time, have been directed principally to the application of the theory of ambiguity to transcendental analysis. It is desired see a priori  in a relation among quantities or transcendental functions, what transformations one may make, what quantities one may substitute for the given quantities, without the relation ceasing to be valid. This enables us to recognize at once the impossibility of many expressions which we might seek. But I have no time, and my ideas are not developed in this field, which is immense.

Print this letter in the Revue Encyclopédique.

I have often in my life ventured to advance propositions of which I was uncertain; but all that I have written here has been in my head nearly a year, and it is too much to my interest not to deceive myself that I have been suspected of announcing theorems of which I had not the complete demonstration.

Ask Jacobi or Gauss publicly to give their opinion, not as to the truth, but as to the importance of the theorems.

Subsequently there will be, I hope, some people who will find it to their profit to decipher all this mess.

J t'embrasse avec effusion.
                        
                                                     E. Galois.   May 29, 1832.

Translation, in part, in The Unravelers: Mathematical Snapshots, by Jean Francois Dars, Annick Lesne, and Anne Papillaut (A.K. Peters, 2008)–

"You know, dear Auguste, that these subjects are not the only ones I have explored. For some time my main meditations have been directed on the application to transcendental analysis of the theory of ambiguity. The aim was to see in a relation between quantities or transcendental functions, what exchanges we could make, what quantities could be substituted to the given quantities without the relation ceasing to take place. In that way we see immediately that many expressions that we might look for are impossible. But I don't have the time and my ideas are not yet developed enough in this vast field."

Another translation, by James Dolan at the n-Category Café—

"My principal meditations for some time have been directed towards the application of the theory of ambiguity to transcendental analysis. It was a question of seeing a priori in a relation between quantities or transcendent functions, what exchanges one could make, which quantities one could substitute for the given quantities without the original relation ceasing to hold. That immediately made clear the impossibility of finding many expressions that one could look for. But I do not have time and my ideas are not yet well developed on this ground which is immense."

Related material—

"Renormalisation et Ambiguité Galoisienne," by Alain Connes, 2004

"La Théorie de l’Ambiguïté : De Galois aux Systèmes Dynamiques," by Jean-Pierre Ramis, 2006

"Ambiguity Theory, Old and New," preprint by Yves André, May 16, 2008,

"Ambiguity Theory," post by David Corfield at the n-Category Café, May 19, 2008

"Measuring Ambiguity," inaugural lecture at Utrecht University by Gunther Cornelissen, Jan. 16, 2009

Today is the 21st birthday of my note “The Relativity Problem in Finite Geometry.”

Some relevant quotations:

“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”

— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16

Describing the branch of mathematics known as Galois theory, Weyl says that it

“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”

— Weyl, Symmetry, Princeton University Press, 1952, p. 138

Weyl’s set Sigma is a finite set of complex numbers.   Some other sets with “discrete and finite character” are those of 4, 8, 16, or 64 points, arranged in squares and cubes.  For illustrations, see Finite Geometry of the Square and Cube.  What Weyl calls “the relativity problem” for these sets involves fixing “objectively” a class of equivalent coordinatizations.  For what Weyl’s “objectively” means, see the article “Symmetry and Symmetry  Breaking,” by Katherine Brading and Elena Castellani, in the Stanford Encyclopedia of Philosophy:

“The old and natural idea that what is objective should not depend upon the particular perspective under which it is taken into consideration is thus reformulated in the following group-theoretical terms: what is objective is what is invariant with respect to the transformation group of reference frames, or, quoting Hermann Weyl (1952, p. 132), ‘objectivity means invariance with respect to the group of automorphisms [of space-time].^‘[22]

22. The significance of the notion of invariance and its group-theoretic treatment for the issue of objectivity is explored in Born (1953), for example. For more recent discussions see Kosso (2003) and Earman (2002, Sections 6 and 7).

References:

Born, M., 1953, “Physical Reality,” Philosophical Quarterly, 3, 139-149. Reprinted in E. Castellani (ed.), Interpreting Bodies: Classical and Quantum Objects in Modern Physics, Princeton, NJ: Princeton University Press, 1998, pp. 155-167.

Earman, J., 2002, “Laws, Symmetry, and Symmetry Breaking; Invariance, Conservation Principles, and Objectivity,’ PSA 2002, Proceedings of the Biennial Meeting of the Philosophy of Science Association 2002, forthcoming [Abstract/Preprint available online]

Kosso, P., 2003, “Symmetry, objectivity, and design,” in K. Brading and E. Castellani (eds.), Symmetries in Physics: Philosophical Reflections, Cambridge: Cambridge University Press, pp. 410-421.

Weyl, H., 1952, Symmetry, Princeton, NJ: Princeton University Press.

See also

Archives Henri Poincaré (research unit UMR 7117, at Université Nancy 2, of the CNRS)–

“Minkowski, Mathematicians, and the Mathematical Theory of Relativity,” by Scott Walter, in The Expanding Worlds of General Relativity (Einstein Studies, volume 7), H. Goenner, J. Renn, J. Ritter and T. Sauer, editors, Boston/Basel: Birkhäuser, 1999, pp. 45-86–

“Developing his ideas before Göttingen mathematicians in April 1909, Klein pointed out that the new theory based on the Lorentz group (which he preferred to call ‘Invariantentheorie’) could have come from pure mathematics (1910: 19). He felt that the new theory was anticipated by the ideas on geometry and groups that he had introduced in 1872, otherwise known as the Erlangen program (see Gray 1989: 229).”

References:

Gray, Jeremy J. (1989). Ideas of Space. 2d ed. Oxford: Oxford University Press.

Klein, Felix. (1910). “Über die geometrischen Grundlagen der Lorentzgruppe.” Jahresbericht der deutschen Mathematiker-Vereinigung 19: 281-300. [Reprinted: Physikalische Zeitschrift 12 (1911): 17-27].

Related material: A pathetically garbled version of the above concepts was published in 2001 by Harvard University Press.  See Invariances: The Structure of the Objective World, by Robert Nozick.

Mackey was born, according to Wikipedia, on Feb. 1, 1916.  He died, according to Harvard University, on the night of March 14-15, 2006.  He was the author of, notably, “Harmonic Analysis as the Exploitation of Symmetry — A Historical Survey,” pp. 543-698 in Bulletin of the American Mathematical Society (New Series), Vol. 3, No. 1, July 1980.  This is available in a hardcover book published in 1992 by the A.M.S., The Scope and History of Commutative and Noncommutative Harmonic Analysis. (370 pages, ISBN 0-8218-9903-1).  A paperback edition of this book will apparently be published this month by Oxford University Press (ISBN 978-0-8218-3790-7). 

From Oxford U.P.–

Contents

• Introduction
• Harmonic analysis as the exploitation of symmetry: A historical survey
• Herman Weyl and the application of group theory to quantum mechanics
• The significance of invariant measures for harmonic analysis
• Weyl’s program and modern physics
• Induced representations and the applications of harmonic analysis
• Von Neumann and the early days of ergodic theory
• Final remarks

Related material:
Log24, Oct. 22, 2002.
Women’s history month continues.

Symmetry and a Trinity

From a web page titled Spectra:

"What we learn from our whole discussion and what has indeed become a guiding principle in modern mathematics is this lesson:

Whenever you have to do with a structure-endowed entity  S try to determine its group of automorphisms, the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of S in this way. After that you may start to investigate symmetric configurations of elements, i.e., configurations which are invariant under a certain subgroup of the group of all automorphisms . . ."

— Hermann Weyl in Symmetry, Princeton University Press, 1952, page 144

"… any color at all can be made from three different colors, in our case, red, green, and blue lights. By suitably mixing the three together we can make anything at all, as we demonstrated . . .

Further, these laws are very interesting mathematically. For those who are interested in the mathematics of the thing, it turns out as follows. Suppose that we take our three colors, which were red, green, and blue, but label them A, B, and C, and call them our primary colors. Then any color could be made by certain amounts of these three: say an amount a of color A, an amount b of color B, and an amount c of color C makes X:

Now suppose another color Y is made from the same three colors:

Then it turns out that the mixture of the two lights (it is one of the consequences of the laws that we have already mentioned) is obtained by taking the sum of the components of X and Y:

It is just like the mathematics of the addition of vectors, where (a, b, c ) are the components of one vector, and (a', b', c' ) are those of another vector, and the new light Z is then the "sum" of the vectors. This subject has always appealed to physicists and mathematicians."

— According to the author of the Spectra site, this is Richard Feynman in Elementary Particles and the Laws of Physics, The 1986 Dirac Memorial Lectures, by Feynman and Steven Weinberg, Cambridge University Press, 1989.

These two concepts — symmetry as invariance under a group of transformations, and complicated things as linear combinations (the technical name for Feynman's sums) of simpler things — underlie much of modern mathematics, both pure and applied.      

Birthdate of Hermann Weyl

Result of a Google search.

Category:  Science > Math > Algebra > Group Theory 

Quotation from Weyl’s Symmetry:

“Symmetry is a vast subject, significant in art and nature. Mathematics lies at its root, and it would be hard to find a better one on which to demonstrate the working of the mathematical intellect.”

In honor of Princeton University, of Sylvia Nasar (see entries of Nov, 6), of the Presbyterian Church (see entry of Nov. 8), and of Professor Weyl (whose work partly inspired the website Diamond Theory), this site’s background music is now Pink Floyd’s

Updates of Friday, November 15, 2002:

In order to clarify the meaning of “Shine” and “Crazy” in the above, consult the following —

• “Shine” … The Shining, by Stephen King
• “Crazy” … The Hollow Man, by Dan Simmons 

To accompany this detailed exegesis of Pink Floyd, click here for a reading by Marlon Brando.

For a related educational experience, see pages 126-127 of The Book of Sequels, by Henry Beard, Christopher Cerf, Sarah Durkee, and Sean Kelly (Random House paperback, 1990).

Speaking of sequels, be on the lookout for Annie Dillard’s sequel to Teaching a Stone to Talk, titled Teaching a Brick to Sing.