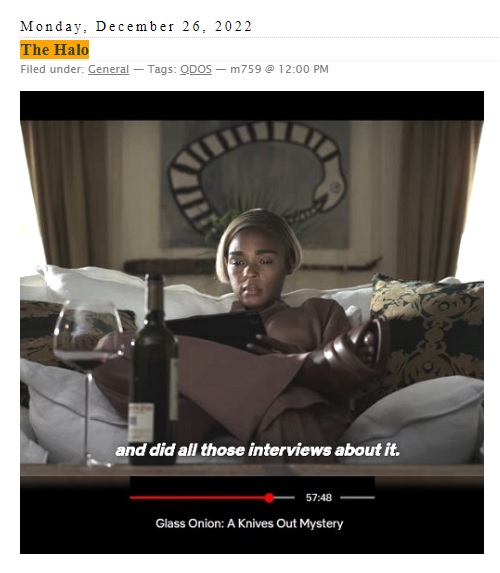
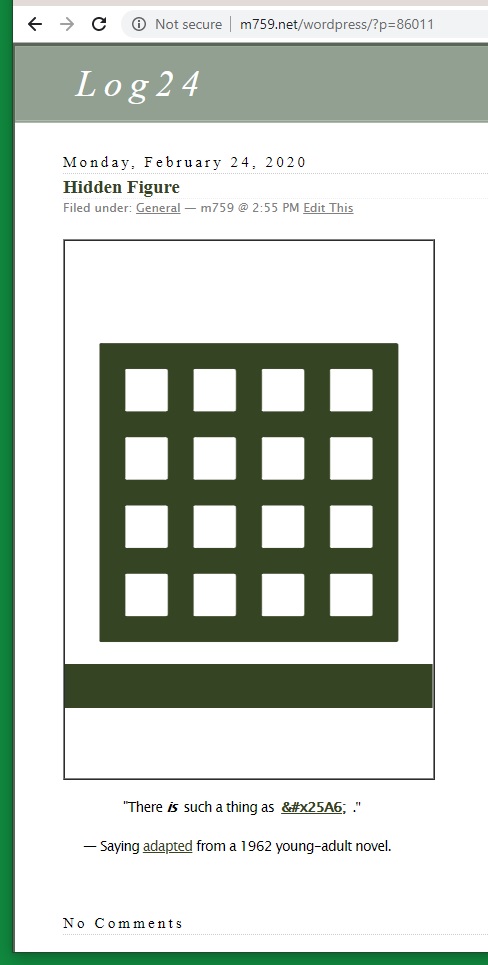
See Cormac McCarthy on "The Kekulé Problem" and . . .
Friday, January 13, 2023
Saturday, August 15, 2020
Hidden Figures Matter
“Don’t forget the portcullis, Dutch Boy!”
Comments Off on Hidden Figures Matter
Wednesday, August 12, 2020
Hidden Figure: Type Design at the East Village Other
I.e. . . . 
Comments Off on Hidden Figure: Type Design at the East Village Other
Monday, February 24, 2020
Hidden Figure
Comments Off on Hidden Figure
Friday, June 22, 2018
Saturday, March 4, 2017
Hidden Figure
Comments Off on Hidden Figure
Friday, August 17, 2012
Hidden
Detail from last night's 1.3 MB image
"Search for the Lost Tesseract"—
The lost tesseract appears here on the cover of Wittgenstein's
Zettel and, hidden in the form of a 4×4 array, as a subarray
of the Miracle Octad Generator on the cover of Griess's
Twelve Sporadic Groups and in a figure illustrating
the geometry of logic.
Another figure—
Gligoric died in Belgrade, Serbia, on Tuesday, August 14.
From this journal on that date—
"Visual forms, he thought, were solutions to
specific problems that come from specific needs."
— Michael Kimmelman in The New York Times
obituary of E. H. Gombrich (November 7th, 2001)
Comments Off on Hidden
Tuesday, August 20, 2024
Academic Tribes
|
Peter Woit today (https://www.math.columbia.edu/~woit/wordpress/?p=14092): From the beginning in 1984 I was dubious about string theory unification, and by the late 1990s could not understand why this was dominating physics departments and popular science outlets, with no acknowledgement of the serious problems and failures of the theory. From talking privately to physicists, it became clear that the field of particle theory had for quite a while become disturbingly tribal. There was a string theory tribe, seeing itself as embattled and fighting less intelligent other tribes for scarce resources. Those within the tribe wouldn’t say anything publicly critical of the theory, since that would not only hurt their own interests, but possibly get them kicked out of the tribe. Those outside the tribe also were very leery of saying anything, partly because they felt they lacked the expertise to do so, partly because they feared retribution from powerful figures in the string theory tribe. |
See also http://m759.net/wordpress/?tag=tri-be.
Comments Off on Academic Tribes
Sunday, August 18, 2024
Heart of Weir’d . . . For Mr. Kurtz
|
A brief excerpt from a 2018 book about the woman who inspired Zen and the Art of Motorcycle Maintenance . . . "There is a passage in Joseph Conrad’s novella, Heart of Darkness (1899), which exemplifies much about what Quality means . . . . … the narrator, Marlow … is … in an environment he finds malign, sinister, macabre, chaotic, indifferently cruel, and nightmarishly meaningless. What saves him is his accidental discovery of a dry old seamanship manual . . . ." Conrad, as quoted in the book cited below: It was an extraordinary find. Its title was An Inquiry into some Points of Seamanship, by a man Towser, Towson – some such name – Master in his Majesty’s Navy. The matter looked dreary reading enough, with illustrative diagrams and repulsive tables of figures, and the copy was sixty years old. I handled this amazing antiquity with the greatest possible tenderness, lest it should dissolve in my hands. Within, Towson or Towser was inquiring earnestly into the breaking strain of ships’ chains and tackle, and other such matters. Not a very enthralling book; but at the first glance you could see there a singleness of intention, an honest concern for the right way of going to work, which made these humble pages, thought out so many years ago, luminous with another than a professional light. The simple old sailor, with his talk of chains and purchases, made me forget the jungle and the pilgrims in a delicious sensation of having come upon something unmistakably real. — From pp. 36-37 of James Essinger and Henry Gurr's
A Woman of Quality: |
See also earlier posts tagged Weir'd.
Comments Off on Heart of Weir’d . . . For Mr. Kurtz
Monday, March 4, 2024
Report on the Diamond Theorem
by Google Gemini (Advanced)
|
by Google Gemini AI Monday, March 4, 2024
Absolutely! Here's an academic paper about the Cullinane diamond theorem. Please note that while I provide citations, in a fully formatted paper you'd need to adhere to your chosen academic style guide for proper formatting:
The Cullinane Diamond Theorem: Abstract This paper examines the Cullinane diamond theorem, a principle in finite projective geometry that offers unexpected insights into the symmetries found in graphic designs. The theorem, initially formulated by Steven H. Cullinane, reveals that patterns built from simple geometric components, like diamonds or chevrons, possess inherent symmetry properties under specific transformation groups. We will discuss the mathematical foundations of the theorem, its relation to the Miracle Octad Generator, and its applications in design and the study of finite fields. Introduction Symmetry plays a vital role in art and design, contributing to a sense of balance, harmony, and aesthetic appeal. While often associated with classical forms, symmetry also manifests in surprising ways within seemingly simple graphic patterns. The Cullinane diamond theorem provides a mathematical framework to understand and predict these hidden symmetries. Mathematical Background Finite Projective Geometries: The Cullinane diamond theorem has its roots in finite projective geometry. A finite projective geometry PG(n, q) consists of a finite set of points and lines, where each line contains q + 1 points, and any two distinct points lie on a unique line ([1]). Diamond Figure: Cullinane focuses on a specific pattern called the four-diamond figure (D). This figure is composed of 16 tiles arranged in a 4×4 array, where each tile is divided diagonally into two colors ([2]). Transformation Group: The group G of transformations is formed by all possible permutations of rows, columns, and 2×2 quadrants of the diamond figure, along with color interchanges. The size of this group is 322,560 ([2]). The Cullinane Diamond Theorem
Theorem:
Proof: Connections to the Miracle Octad Generator The Cullinane diamond theorem shares a relationship with R.T. Curtis's Miracle Octad Generator (MOG). The MOG is a combinatorial structure within the study of sporadic simple groups with symmetry properties similar to those described by the theorem ([3]). Applications Graphic Design: The theorem informs artistic compositions employing diamond and chevron-like shapes. Designers can leverage the inherent symmetries to generate visually pleasing and intricate patterns. Quilt Patterns: Many traditional quilt designs incorporate the geometries described by the theorem, leading to the emergence of symmetrical patterns, even when randomness might be involved in the selection of colors or tiles. Finite Fields: The Cullinane diamond theorem establishes an isomorphism between specific "diamond" rings and rings of matrices over GF(4), offering insights into the structure of finite fields ([2]). Conclusion The Cullinane diamond theorem elegantly connects abstract mathematics with the world of graphic design. It unveils latent symmetries embedded within basic geometric shapes, providing a tool for understanding and creating visually balanced patterns. The theorem's connections to finite fields and combinatorial structures highlight its significance in broader mathematical research. Citations [1] Weisstein, E. W. “Finite Projective Geometry.” From MathWorld–A Wolfram Web Resource. [invalid URL removed] [2] Cullinane, S. H. "Cullinane Diamond Theorem." Encyclopedia of Mathematics. https://encyclopediaofmath.org/wiki/Cullinane_diamond_theorem [3] Curtis, R. T. (1976). A New Combinatorial Approach to M₂₄. Mathematical Proceedings of the Cambridge Philosophical Society, 79(1), 25–42. doi:10.1017/S0305004100052075 Let me know if you'd like me to expand on any particular aspect or provide additional citations!
1. www.hellenicaworld.com/Science/Mathematics/en/MathieuGroup.html
|
Comments Off on Report on the Diamond Theorem
by Google Gemini (Advanced)
by Google Gemini (Advanced)
Sunday, March 3, 2024
Deep Blue Research: A Report by You.com AI
|
Cullinane Diamond Theorem Research Report by https://you.com/?chatMode=research on March 3, 2024 Overview of the Cullinane Diamond Theorem The Cullinane Diamond Theorem is a mathematical concept developed by Steven H. Cullinane that explores the symmetrical properties of certain geometric patterns. It is particularly concerned with the structure of finite projective geometry as it relates to the 35 square patterns found in R. T. Curtis's Miracle Octad Generator (MOG). The theorem asserts that every G-image of a diamond figure D possesses some form of ordinary or color-interchange symmetry. Symmetry and Group Theory The theorem is deeply rooted in group theory, with G being isomorphic to the affine group A on the linear 4-space over the finite field GF(2). This group has a significant order of 322,560 and underlies the symmetry of both the diamond-theorem figures and the square patterns of the MOG . The 35 structures of the 840 G-images of D are isomorphic to the 35 lines in the 3-dimensional projective space over GF(2), which explains the symmetry of the line diagrams and the two-color patterns. The Diamond Theorem in Various Dimensions The diamond theorem can be applied to different cases, such as the 4×4, 2×2, and even 3-dimensional cubes like the 2x2x2 and 4x4x4 cubes. The generalized statement of the theorem involves inscribing a white diamond in a black square and dividing it into four quadrants, each with a diagonal split into black and white halves. Algebraic Implications The theorem has algebraic implications, such as the ability to express every 4-coloring as a sum of three 2-colorings. This algebraic formulation is useful for demonstrating that certain four-colored graphic images can form a ring under multiplication. There is also an infinite family of such 'diamond' rings, isomorphic to rings of matrices over GF(4). Philosophical and Cultural Context Steven H. Cullinane has also explored the philosophical implications of the diamond theorem, referring to it in the context of Plato's Meno dialogue and opposing it to the 'Story Theory of truth' as discussed by Richard J. Trudeau. The theorem is seen as a correct statement of the relation between geometry and the physical universe. Clarification on Cullinan Diamonds It is important to note that the Cullinane Diamond Theorem is unrelated to the Cullinan diamond, which is a famous large gemstone discovered in South Africa and now part of the British Crown Jewels. The Cullinan diamond has its own historical and cultural significance, with its various cut shapes and ownership history. In summary, the Cullinane Diamond Theorem is a mathematical concept that reveals the symmetrical properties of certain geometric patterns, with applications in group theory and algebra. It also has philosophical implications, drawing connections to classical ideas about truth and geometry. |
Instagram ad for You.com AI in research mode
"Show me ALL your sources, babe."
— Line adapted from Leonardo DiCaprio
Comments Off on Deep Blue Research: A Report by You.com AI
Tuesday, January 30, 2024
Monday, January 29, 2024
Self as Imago Dei: Hofstadter vs. Valéry
|
Google search result: Saint Anselm College https://www.anselm.edu › Documents › Brown by M Brown · 2014 · Cited by 14 — Thomas insists that the image of God exists most perfectly in the acts of the soul, for the soul is that which is most perfect in us and so best images God, and … 11 pages |
For a Douglas Hofstadter version of the Imago Dei , see the
"Gödel, Escher, Bach" illustration in the Jan. 15 screenshot below —
Tuesday, June 15, 2010
|
Comments Off on Self as Imago Dei: Hofstadter vs. Valéry
Sunday, December 17, 2023
Speak, Memory
"Unsocratic Dialogue" with Google DeepMind's Gemini AI continues . . .
|
Do you remember a previous conversation about the question "What is the Cullinane diamond theorem?" If so, how would you answer the question now?
|
Comments Off on Speak, Memory
Thursday, December 14, 2023
Unsocratic Dialogue (with Gemini AI in Bard Today)
|
What is the Cullinane diamond theorem?
|
Comments Off on Unsocratic Dialogue (with Gemini AI in Bard Today)
Monday, August 7, 2023
Exorcist Director Dies
|
Los Angeles Times today BY CHRISTI CARRAS, STAFF WRITER
"William Friedkin, a master of suspense and leading figure of the 1970s New Hollywood movement who was known for directing films such as 'The Exorcist' and 'The French Connection,' has died. Friedkin died Monday in Los Angeles, his widow, Sherry Lansing, confirmed to the Los Angeles Times. CAA, which represents Lansing, said Friedkin died at home from heart failure and pneumonia. He was 87." |
Update at 8:42 PM ET —
A French Connection for Friedkin, from
the SXSW opening date of one of his films —
Comments Off on Exorcist Director Dies
Friday, June 30, 2023
Trickster Fuge (German for Joint)
|
Margaret Atwood on Lewis Hyde's "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159) What is "the next world"? It might be the Underworld…. The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart. |
"Drop me a line" — Request attributed to Emma Stone
Comments Off on Trickster Fuge (German for Joint)
Tuesday, June 20, 2023
Annals of Devolution
|
The War to End All Wars (2018)
|
Comments Off on Annals of Devolution
Monday, February 6, 2023
Interality Studies
|
You, Xi-lin; Zhang, Peter. "Interality in Heidegger."
The term "interology" is meant as an interventional alternative to traditional Western ontology. The idea is to help shift people's attention and preoccupation from subjects, objects, and entities to the interzones, intervals, voids, constitutive grounds, relational fields, interpellative assemblages, rhizomes, and nothingness that lie between, outside, or beyond the so-called subjects, objects, and entities; from being to nothing, interbeing, and becoming; from self-identicalness to relationality, chance encounters, and new possibilities of life; from "to be" to "and … and … and …" (to borrow Deleuze's language); from the actual to the virtual; and so on. As such, the term wills nothing short of a paradigm shift. Unlike other "logoi," which have their "objects of study," interology studies interality, which is a non-object, a no-thing that in-forms and constitutes the objects and things studied by other logoi. |
Some remarks from this journal on April 1, 2015 —
Manifest O
|
| 83-06-21 | An invariance of symmetry The diamond theorem on a 4x4x4 cube, and a sketch of the proof. |
| 83-10-01 | Portrait of O A table of the octahedral group O using the 24 patterns from the 2×2 case of the diamond theorem. |
| 83-10-16 | Study of O A different way of looking at the octahedral group, using cubes that illustrate the 2x2x2 case of the diamond theorem. |
| 84-09-15 | Diamonds and whirls Block designs of a different sort — graphic figures on cubes. See also the University of Exeter page on the octahedral group O. |
The above site, finitegeometry.org/sc, illustrates how the symmetry
of various visual patterns is explained by what Zhang calls "interality."
Comments Off on Interality Studies
Thursday, December 8, 2022
Centrality Continued
|
Alicia — "Gödel never says outright that there is a covenant to which all of mathematics subscribes but you get a clear sense that the hope is there. I know the allure. Some shimmering palimpsest of eternal abidement. But to claim that numbers somehow exist in the Universe with no intelligence to enable them does not require a different sort of mathematics. It requires a different sort of universe." Psychiatrist — "Is there such a universe?"
— McCarthy, Cormac. Stella Maris (p. 180). |
A palimpsest from Oslo artist Josefine Lyche —
Comments Off on Centrality Continued
Saturday, September 10, 2022
Solomon’s Mental Health Month
May 2003 was "Solomon's Mental Health Month" in this journal.
An essay linked to on the 9th of May in that month —
|
"Taking the Veil," by Jessica Kardon
https://web.archive.org/web/20021102182519/ James Hillman, writing in The Soul's Code, argues for his "acorn theory" of human individual identity, and suggests that "each person bears a uniqueness that asks to be lived and that is already present before it can be lived." He insists we are born with a given character, a daimon, the carrier of destiny. This theory is closely linked to the beautiful myth described by Plato in his Republic, when the soul stands before Lachesis and receives his specific soul guardian. Hillman maintains that the daimon will always emerge somehow, even if thwarted or unrecognized. I never had ambitions that reached fruition in the adult world. I have had only two career interests in my life – both formed precognitively. I wanted to be a mermaid or a nun. By the time I learned – shockingly late – that I could not be a mermaid, I had realized I would not be a nun. I concur with Hillman's emphasis on the persistence of early disposition, and I like to imagine that my dreamy, watery, Victorian and self-righteous psyche has held aspects of both of these early interests, throughout my life. I was adopted one month after my birth. I was tended by nuns during the first four weeks of my life. Thereafter, I spent my whole educational life in convent schools. It was the sisters of Saint Vincent de Paul that gave me my favorite musical and my early distortions about romantic love and the gender plans of Our Lord. My misconceptions about love and marriage were culled from the Lerner Loewe musical Gigi, a wonderful film based loosely on a Colette novel. I was summoned along with my whole class to the gymnasium to view the movie under the edgy eye of Sister Bernadette. Sister Bernadette was a large, mesomorphic nun famed for the beatings she gave to boys and girls alike, and feared for the mean zest with which she bestowed her favors upon many of us. I was not beaten – but once, believing I was wearing lipstick, she held my head in a sink and scrubbed my lips until they bled, then slapped me. I recall this with a mild, rueful whimsy. We were all manhandled. In memory, Bernadette seems more like an angry and troubled older sibling than a true figure of authority. Anyway, I loved Gigi. It fed directly into my Francophilia. I was convinced that at some future date, I, like Gigi, would be trained as a courtesan. I, too, would cause some hard case, experienced roué to abandon his chill and irony. I saw myself strolling down the Champs Elysee with Louis Jordan in rapt attendance, pushing a baby carriage, wearing a hat the size of a manhole cover, hoisting a parasol above that to assure the longevity of my adorable pallor. The gender plans of Our Lord had recently been revealed to me too. Sister B. had drawn a ladder on the blackboard, a ladder with three rungs. At the top, she explained, were the priests, the nuns, and the monks. These souls had surrendered their lives to God. All would be taken directly to heaven upon their passing from this vale of tears, as we all referred to the world in those lean emotional times. On the middle rung stood the married. If you married and kept the law – which meant leaving every act of marital congress open to the reception of a child, you would be eligible for heaven. If you were foul in marriage, seeking your pleasure, you were going to be damned. On the bottom rung were those selfish souls who had remained single and had imagined their lives their own. This group had never given themselves to Our Lord. They were headed to hell in a sort of preternatural laundry chute. So we little ladies had two viable options: marry and breed without ceasing – or take the veil. Despite my hat and perambulator fantasies, once given the sorry news of the ladder, the veil became the clear romantic favorite. Therefore I began my research. I obtained a catalogue of nunnery. It offered photographs of each order, describing the duties of the specific order, and displaying the garb of that order. I was looking for two things – a great looking veil and gown, and a contemplative order. I had no desire to sully my glorious vision of myself with a life in the outer world. It was apparent to me that the teaching of children was going to involve a whole range of miseries – making them cry, telling them the bad news about the ladder, and so forth. This was not for me. I saw myself kneeling on the floor of my pristine little cell, serene and untouched by human hands. Teaching would be certain to interfere with the proper lighting. Yoked to a bunch of messy children, I could not possibly have the opalescent illumination of heaven falling reliably on my upturned visage. What divided me from my dream of rebirth as a mermaid was the force of what was real: I could not morph. What divided me from my dream of life as a nun was the force of the erotic: I would not abstain. Now, long years later, I am still underwater, and I am still bending the knee. I live in the blue shadows of hidden grottoes, and I am swimming, too, in the gold of my drifting prayers. September 7th, this dream. I am standing in a dimly lit room, gazing at a group of heavy, antique silk burqas that look weirdly like Fortuny gowns. A holy woman approaches me, and tells me that my soul will leave my body, and enter these garments. She turns and points at a young girl standing nearby, a child with close-cropped hair and a solemn look. My heart knows her, but my eyes don't. For a moment I am thinking, exactly as I did in the seventies when holding a joint: "This isn't working." Suddenly, these things: I feel the shape of flame, then I am the shape. I am released into the air, and as pure essence I enter other forms, dissolving in them, gathering my energy back into myself, and flying out again. This was a sensation so exquisite that my dreaming brain woke up and announced to me: "This is a dream about death." I saw that child again as I flew. This time my eyes knew her. I flew to her, but the flame of my soul would not cohere with hers, this child who was, of course, my own self. In the shadows alone, I heard myself whisper: "I'm in the wind. I'm in the water." This lovely dream, which gave me the sublime gift of a little visceral preview of the soul in the death process, also showed me my guardian spirit; divided, but viable. I pass through my life swimming in one self, kneeling in the other. I thought of Rilke's 29th Sonnet to Orpheus and realized this was what I had been dreaming about all my life, moving between them.
by jessica kardon |
See as well yesterday's post "At a Still Point."
Comments Off on Solomon’s Mental Health Month
Sunday, July 31, 2022
Sunday, August 23, 2020
Jung on the Quaternity…
Or: "1, 2, 3 , 4, who are we for?"
Related material —
Comments Off on Jung on the Quaternity…
Wednesday, April 1, 2020
Wednesday, March 11, 2020
Visualizing Mathieu Group Generators
Update of March 17, 2020 —
The graphic images illustrate nicely Conder's six 4-cycles, but
their relationship, if any, to his eight 2-cycles is a mystery —
The Conder paper is at
https://core.ac.uk/download/pdf/82622574.pdf.
Comments Off on Visualizing Mathieu Group Generators
Monday, March 9, 2020
“Archimedes at Hiroshima” Continues.
The title is from a post of January 10, 2019.
A figure from this journal on June 1, 2019 —
The following figure may help relate labelings of the
truncated octahedron ("permutahedron") to labelings
of its fellow Archimedean solid, the cuboctahedron.
See as well other posts tagged Aitchison.
Comments Off on “Archimedes at Hiroshima” Continues.
Wednesday, July 17, 2019
The Artsy Quantum Realm
|
arXiv.org > quant-ph > arXiv:1905.06914 Quantum Physics Placing Kirkman's Schoolgirls and Quantum Spin Pairs on the Fano Plane: A Rainbow of Four Primary Colors, A Harmony of Fifteen Tones J. P. Marceaux, A. R. P. Rau (Submitted on 14 May 2019) A recreational problem from nearly two centuries ago has featured prominently in recent times in the mathematics of designs, codes, and signal processing. The number 15 that is central to the problem coincidentally features in areas of physics, especially in today's field of quantum information, as the number of basic operators of two quantum spins ("qubits"). This affords a 1:1 correspondence that we exploit to use the well-known Pauli spin or Lie-Clifford algebra of those fifteen operators to provide specific constructions as posed in the recreational problem. An algorithm is set up that, working with four basic objects, generates alternative solutions or designs. The choice of four base colors or four basic chords can thus lead to color diagrams or acoustic patterns that correspond to realizations of each design. The Fano Plane of finite projective geometry involving seven points and lines and the tetrahedral three-dimensional simplex of 15 points are key objects that feature in this study. Comments:16 pages, 10 figures Subjects:Quantum Physics (quant-ph) Cite as:arXiv:1905.06914 [quant-ph] (or arXiv:1905.06914v1 [quant-ph] for this version) Submission history
From: A. R. P. Rau [view email] |
See also other posts tagged Tetrahedron vs. Square.
Comments Off on The Artsy Quantum Realm
Monday, July 1, 2019
Inside the Exploded Cube
Metaphysical conceit | literature | Britannica.com
|
This post's title refers to a metaphysical conceit
in the previous post, Desperately Seeking Clarity.
Related material —
The source of the above mystical octahedron —
See also Jung's Imago Dei in this journal.
Comments Off on Inside the Exploded Cube
Sunday, May 26, 2019
Sunday Shul: Connecting the Dots
"It's very easy to say, 'Well, Jeff couldn't quite connect these dots,'"
director Jeff Nichols told BuzzFeed News. "Well, I wasn't actually
looking at the dots you were looking at."
— Posted on March 21, 2016, at 1:11 p.m,
Adam B. Vary, BuzzFeed News Reporter
|
"Magical arrays of numbers have been the talismans of mathematicians and mystics since the time of Pythagoras in the sixth century B.C. And in the 16th century, Rabbi Isaac ben Solomon Luria devised a cosmological world view that seems to have prefigured superstring theory, at least superficially. Rabbi Luria was a sage of the Jewish cabalist movement — a school of mystics that drew inspiration from the arcane oral tradition of the Torah.
According to Rabbi Luria's cosmology, the soul and inner life of the hidden God were expressed by 10 primordial numbers
— "Things Are Stranger Than We Can Imagine," |
Comments Off on Sunday Shul: Connecting the Dots
Wednesday, March 6, 2019
The Relativity Problem and Burkard Polster
|
From some 1949 remarks of Weyl— "The relativity problem is one of central significance throughout geometry and algebra and has been recognized as such by the mathematicians at an early time." — Hermann Weyl, "Relativity Theory as a Stimulus in Mathematical Research," Proceedings of the American Philosophical Society , Vol. 93, No. 7, Theory of Relativity in Contemporary Science: Papers Read at the Celebration of the Seventieth Birthday of Professor Albert Einstein in Princeton, March 19, 1949 (Dec. 30, 1949), pp. 535-541 Weyl in 1946—: "This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them." — Hermann Weyl, The Classical Groups , Princeton University Press, 1946, p. 16 |
For some context, see Relativity Problem in this journal.
In the case of PG(3,2), there is a choice of geometric models
to be coordinatized: two such models are the traditional
tetrahedral model long promoted by Burkard Polster, and
the square model of Steven H. Cullinane.
The above Wikipedia section tacitly (and unfairly) assumes that
the model being coordinatized is the tetrahedral model. For
coordinatization of the square model, see (for instance) the webpage
Finite Relativity.
For comparison of the two models, see a figure posted here on
May 21, 2014 —
Labeling the Tetrahedral Model (Click to enlarge) —
"Citation needed" —
The anonymous characters who often update the PG(3,2) Wikipedia article
probably would not consider my post of 2014, titled "The Tetrahedral
Model of PG(3,2)," a "reliable source."
Comments Off on The Relativity Problem and Burkard Polster
Sunday, October 21, 2018
For Connoisseurs of Bad Art
Yesterday afternoon's post "Study in Blue and Pink" featured
an image related to the "Blade and Chalice" of Dan Brown …
Requiem for a comics character known as "The Blue Blade" —
"We all float down here."
About the corresponding "Pink Chalice," the less said the better.
Comments Off on For Connoisseurs of Bad Art
Monday, October 13, 2014
Raiders of the Lost Theorem
(Continued from Nov. 16, 2013.)
The 48 actions of GL(2,3) on a 3×3 array include the 8-element
quaternion group as a subgroup. This was illustrated in a Log24 post,
Hamilton’s Whirligig, of Jan. 5, 2006, and in a webpage whose
earliest version in the Internet Archive is from June 14, 2006.
One of these quaternion actions is pictured, without any reference
to quaternions, in a 2013 book by a Netherlands author whose
background in pure mathematics is apparently minimal:
In context (click to enlarge):
Update of later the same day —
Lee Sallows, Sept. 2011 foreword to Geometric Magic Squares —
“I first hit on the idea of a geometric magic square* in October 2001,**
and I sensed at once that I had penetrated some previously hidden portal
and was now standing on the threshold of a great adventure. It was going
to be like exploring Aladdin’s Cave. That there were treasures in the cave,
I was convinced, but how they were to be found was far from clear. The
concept of a geometric magic square is so simple that a child will grasp it
in a single glance. Ask a mathematician to create an actual specimen and
you may have a long wait before getting a response; such are the formidable
difficulties confronting the would-be constructor.”
* Defined by Sallows later in the book:
“Geometric or, less formally, geomagic is the term I use for
a magic square in which higher dimensional geometrical shapes
(or tiles or pieces ) may appear in the cells instead of numbers.”
** See some geometric matrices by Cullinane in a March 2001 webpage.
Earlier actual specimens — see Diamond Theory excerpts published in
February 1977 and a brief description of the original 1976 monograph:
“51 pp. on the symmetries & algebra of
matrices with geometric-figure entries.”
— Steven H. Cullinane, 1977 ad in
Notices of the American Mathematical Society
The recreational topic of “magic” squares is of little relevance
to my own interests— group actions on such matrices and the
matrices’ role as models of finite geometries.
Comments Off on Raiders of the Lost Theorem
Thursday, March 29, 2012
Meanwhile…
| A Large & Startling Figure: The Harry Crews Online Bibliography www.harrycrews.org/Features/News/index.html Page updated: March 29, 2012, 08:16 PM Copyright © 1998 – 2010 |
Comments Off on Meanwhile…
Tuesday, January 31, 2012
Tesseract
|
"… a finite set with n elements Tesseract formed from a 4-set—
The same 16 subsets or points can
"There is such a thing as a 4-set." |
Update of August 12, 2012:
Figures like the above, with adjacent vertices differing in only one coordinate,
appear in a 1950 paper of H. S. M. Coxeter—
Comments Off on Tesseract
Saturday, June 4, 2011
Mystery
"… a little mystery to figure out…." — Bonnie Raitt, 1991
Two writers walk into a bar…
(A phrase from Immoveable Feast on May 28, 2005. See also May 28 this year.)
Yesterday's post First Class featured a picture of Mystique.
A related passage* from a book pictured here May 28—
See also Wallace Stevens on the Center.
* on participation mystique — "The primitive mind
does not differentiate the supernatural from reality,
but rather uses 'mystical participation'
to manipulate the world."
— Wikipedia
Comments Off on Mystery
Friday, October 15, 2010
Mathematics and Narrative, continued
The Story of N
Roberta Smith in the New York Times of July 7, 2006—
Art Review
Endgame Art? It's Borrow, Sample and Multiply in an Exhibition at Bard College
"… The show has an endgame, end-time mood, as if we are looking at the end of the end of the end of Pop, hyperrealism and appropriation art. The techniques of replication and copying have become so meticulous that they are beside the point. This is truly magic realism: the kind you can't see, that has to be explained. It is also a time when artists cultivate hybridism and multiplicity and disdain stylistic coherence, in keeping with the fashionable interest in collectivity, lack of ego, the fluidity of individual identity. But too often these avoidance tactics eliminate the thread of a personal sensibility or focus.
I would call all these strategies fear of form, which can be parsed as fear of materials, of working with the hands in an overt way and of originality. Most of all originality. Can we just say it? This far from Andy Warhol and Duchamp, the dismissal of originality is perhaps the oldest ploy in the postmodern playbook. To call yourself an artist at all is by definition to announce a faith, however unacknowledged, in some form of originality, first for yourself, second, perhaps, for the rest of us.
Fear of form above all means fear of compression— of an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible. With a few exceptions, forms of collage and assemblage dominate this show: the putting together (or simply putting side by side) of existing images and objects prevails. The consistency of this technique in two and three dimensions should have been a red flag for the curators. Collage has driven much art since the late 1970's. Lately, and especially in this exhibition, it often seems to have become so distended and pulled apart that its components have become virtually autonomous and unrelated, which brings us back to square one. This is most obvious in the large installations of graphic works whose individual parts gain impact and meaning from juxtaposition but are in fact considered distinct artworks."
Margaret Atwood on art and the trickster—
"The pleasures of fabulation, the charming and playful lie— this line of thought leads Hyde* to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning 'to join,' 'to fit,' and 'to make.' If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart."
* Lewis Hyde, Trickster Makes This World: Mischief, Myth, and Art, Farrar Straus & Giroux, January 1998
Smith mentions "an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible."
Atwood mentions "a seamless whole."
For some related remarks, see "A Study in Art Education" and the central figure pictured above. (There "N" can stand for "number," "nine," or "narrative.")
Comments Off on Mathematics and Narrative, continued
Thursday, July 1, 2010
Plato’s Code
John Allen Paulos yesterday at Twitter—
"Plato's code cracked? http://bit.ly/ad6k1S
Fascinating if not a hoax or hype."
The story that Paulos linked to is about a British
academic who claims to have found some
symbolism hidden in Plato's writings by
splitting each into 12 parts and correlating
the 12 parts with semitones of a musical scale.
I prefer a different approach to Plato that is
related to the following hoax and hype—
HOAX:
From Dan Brown's novel Angels & Demons (2000)—
HYPE:
This four-elements diamond summarizes the classical
four elements and four qualities neatly, but some scholars
might call the figure "hype" since it deals with an academically
disreputable subject, alchemy, and since its origin is unclear.
For the four elements' role in some literature more respectable
than Dan Brown's, see Poetry's Bones.
Although an author like Brown might spin the remarks
below into a narrative— The Plato Code — they are
neither hoax nor hype.
NOT HOAX:
NOT HYPE:
For related non-hoax, non-hype remarks, see
The Rational Enterprise: Logos in Plato's Theaetetus,
by Rosemary Desjardins.
Those who prefer hoax and hype in their philosophy may consult
the writings of, say, Barbara Johnson, Rosalind Krauss, and—
in yesterday's NY Times's "The Stone" column— Nancy Bauer.
— The New York Times
Comments Off on Plato’s Code
Tuesday, February 16, 2010
Mysteries of Faith
From today's NY Times—
Obituaries for mystery authors
Ralph McInerny and Dick Francis
From the date (Jan. 29) of McInerny's death–
"…although a work of art 'is formed around something missing,' this 'void is its vanishing point, not its essence.'"
– Harvard University Press on Persons and Things (Walpurgisnacht, 2008), by Barbara Johnson
From the date (Feb. 14) of Francis's death–
The EIghtfold Cube
The "something missing" in the above figure is an eighth cube, hidden behind the others pictured.
This eighth cube is not, as Johnson would have it, a void and "vanishing point," but is instead the "still point" of T.S. Eliot. (See the epigraph to the chapter on automorphism groups in Parallelisms of Complete Designs, by Peter J. Cameron. See also related material in this journal.) The automorphism group here is of course the order-168 simple group of Felix Christian Klein.
For a connection to horses, see
a March 31, 2004, post
commemorating the birth of Descartes
and the death of Coxeter–
Putting Descartes Before Dehors
For a more Protestant meditation,
see The Cross of Descartes—
"I've been the front end of a horse
and the rear end. The front end is better."
— Old vaudeville joke
For further details, click on
the image below–
Notre Dame Philosophical Reviews
Comments Off on Mysteries of Faith
Saturday, June 21, 2008
Saturday June 21, 2008
For Mary Gaitskill
(See Eight is a Gate and
Faith, Doubt, Art, and
The New Yorker.)
A sructure from
today's previous entry:
|
Everyone comes to Rick's.
Comments Off on Saturday June 21, 2008
Sunday, May 25, 2008
Sunday May 25, 2008
Wechsler Cubes
"Confusion is nothing new."
— Song lyric, Cyndi Lauper
— Song lyric, Cyndi Lauper
Part I:
Magister Ludi
Magister Ludi
Hermann Hesse's 1943 The Glass Bead Game (Picador paperback, Dec. 6, 2002, pp. 139-140)–
"For the present, the Master showed him a bulky memorandum, a proposal he had received from an organist– one of the innumerable proposals which the directorate of the Game regularly had to examine. Usually these were suggestions for the admission of new material to the Archives. One man, for example, had made a meticulous study of the history of the madrigal and discovered in the development of the style a curved that he had expressed both musically and mathematically, so that it could be included in the vocabulary of the Game. Another had examined the rhythmic structure of Julius Caesar's Latin and discovered the most striking congruences with the results of well-known studies of the intervals in Byzantine hymns. Or again some fanatic had once more unearthed some new cabala hidden in the musical notation of the fifteenth century. Then there were the tempestuous letters from abstruse experimenters who could arrive at the most astounding conclusions from, say, a comparison of the horoscopes of Goethe and Spinoza; such letters often included pretty and seemingly enlightening geometric drawings in several colors."
Part II:
A Bulky Memorandum
A Bulky Memorandum
From Siri Hustvedt, author of Mysteries of the Rectangle: Essays on Painting (Princeton Architectural Press, 2005)– What I Loved: A Novel (Picador paperback, March 1, 2004, page 168)–
A description of the work of Bill Wechsler, a fictional artist:
"Bill worked long hours on a series of autonomous pieces about numbers. Like O's Journey, the works took place inside glass cubes, but these were twice as large– about two feet square. He drew his inspiration from sources as varied as the Cabbala, physics, baseball box scores, and stock market reports. He painted, cut, sculpted, distorted, and broke the numerical signs in each work until they became unrecognizable. He included figures, objects, books, windows, and always the written word for the number. It was rambunctious art, thick with allusion– to voids, blanks, holes, to monotheism and the individual, the the dialectic and yin-yang, to the Trinity, the three fates, and three wishes, to the golden rectangle, to seven heavens, the seven lower orders of the sephiroth, the nine Muses, the nine circles of Hell, the nine worlds of Norse mythology, but also to popular references like A Better Marriage in Five Easy Lessons and Thinner Thighs in Seven Days. Twelve-step programs were referred to in both cube one and cube two. A miniature copy of a book called The Six Mistakes Parents Make Most Often lay at the bottom of cube six. Puns appeared, usually well disguised– one, won; two, too, and Tuesday; four, for, forth; ate, eight. Bill was partial to rhymes as well, both in images and words. In cube nine, the geometric figure for a line had been painted on one glass wall. In cube three, a tiny man wearing the black-and-white prison garb of cartoons and dragging a leg iron has
— End of page 168 —
opened the door to his cell. The hidden rhyme is "free." Looking closely through the walls of the cube, one can see the parallel rhyme in another language: the German word drei is scratched into one glass wall. Lying at the bottom of the same box is a tiny black-and-white photograph cut from a book that shows the entrance to Auschwitz: ARBEIT MACHT FREI. With every number, the arbitrary dance of associations worked togethere to create a tiny mental landscape that ranged in tone from wish-fulfillment dream to nightmare. Although dense, the effect of the cubes wasn't visually disorienting. Each object, painting, drawing, bit of text, or sculpted figure found its rightful place under the glass according to the necessary, if mad, logic of numerical, pictorial, and verbal connection– and the colors of each were startling. Every number had been given a thematic hue. Bill had been interested in Goethe's color wheel and in Alfred Jensen's use of it in his thick, hallucinatory paintings of numbers. He had assigned each number a color. Like Goethe, he included black and white, although he didn't bother with the poet's meanings. Zero and one were white. Two was blue. Three was red, four was yellow, and he mixed colors: pale blue for five, purples in six, oranges in seven, greens in eight, and blacks and grays in nine. Although other colors and omnipresent newsprint always intruded on the basic scheme, the myriad shades of a single color dominated each cube.
The number pieces were the work of a man at the top of his form. An organic extension of everything Bill had done before, these knots of symbols had an explosive effect. The longer I looked at them, the more the miniature constructions seemed on the brink of bursting from internal pressure. They were tightly orchestrated semantic bombs through which Bill laid bare the arbitrary roots of meaning itself– that peculiar social contract generated by little squiggles, dashes, lines, and loops on a page."
Part III:
Wechsler Cubes
(named not for
Bill Wechsler, the
fictional artist above,
but for the non-fictional
David Wechsler) –
(named not for
Bill Wechsler, the
fictional artist above,
but for the non-fictional
David Wechsler) –
|
From 2002:
Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale "block design" subtest. |
Part IV:
A Magic Gallery
A Magic Gallery
|
ZZ
Figures from the
Poem by Eugen Jost:
Mit Zeichen und Zahlen
Numbers and Names,
With numbers and names English translation A related poem:
Alphabets
From time to time
But if a savage
— Hermann Hesse (1943), |
Comments Off on Sunday May 25, 2008
Saturday, February 23, 2008
Saturday February 23, 2008
Jumpers
"An acute study of the links
between word and fact"
— Nina daVinci Nichols
Thanks to a Virginia reader for a reminder:
| Virginia | /391062427/item.html? | 2/22/2008 7:37 PM |
The link is to a Log24 entry
that begins as follows…
that begins as follows…
Johnny Cash:
"And behold,
a white horse."
Chess Knight
(in German, Springer)
This, along with the "jumper" theme in the previous two entries, suggests a search on springer jumper.That search yields a German sports phrase, "Springer kommt!" A search on that phrase yields the following:
"Liebe Frau vBayern,
mich würde interessieren wie man
mit diesem Hintergrund
(vonbayern.de/german/anna.html)
zu Springer kommt?"
Background of "Frau vBayern" from thePeerage.com:
Anna-Natascha Prinzessin zu Sayn-Wittgenstein-Berleburg
F, #64640, b. 15 March 1978Last Edited=20 Oct 2005
Anna-Natascha Prinzessin zu Sayn-Wittgenstein-Berleburg was born on 15 March 1978. She is the daughter of Ludwig Ferdinand Prinz zu Sayn-Wittgenstein-Berleburg and Countess Yvonne Wachtmeister af Johannishus. She married Manuel Maria Alexander Leopold Jerg Prinz von Bayern, son of Leopold Prinz von Bayern and Ursula Mohlenkamp, on 6 August 2005 at Nykøping, Södermanland, Sweden.
The date of the above "Liebe Frau vBayern" inquiry, Feb. 1, 2007, suggests the following:
From Log24 on
St. Bridget's Day, 2007:
The quotation
"Science is a Faustian bargain"
and the following figure–
Change
From a short story by
the above Princess:
"'I don't even think she would have wanted to change you. But she for sure did not want to change herself. And her values were simply a part of her.' It was true, too. I would even go so far as to say that they were her basis, if you think about her as a geometrical body. That's what they couldn't understand, because in this age of the full understanding for stretches of values in favor of self-realization of any kind, it was a completely foreign concept."
To make this excellent metaphor mathematically correct,
change "geometrical body" to "space"… as in
"For Princeton's Class of 2007"—
Review of a 2004 production of a 1972 Tom Stoppard play, "Jumpers"–
Related material:
Knight Moves (Log24, Jan. 16),
Kindergarten Theology (St. Bridget's Day, 2008),
and
(Click on image for details.)
Comments Off on Saturday February 23, 2008
Wednesday, October 24, 2007
Wednesday October 24, 2007
Descartes’s Twelfth Step
An earlier entry today (“Hollywood Midrash continued“) on a father and son suggests we might look for an appropriate holy ghost. In that context…
Descartes
A search for further background on Emmanuel Levinas, a favorite philosopher of the late R. B. Kitaj (previous two entries), led (somewhat indirectly) to the following figures of Descartes:
This trinity of figures is taken from Descartes’ Rule Twelve in Rules for the Direction of the Mind. It seems to be meant to suggest an analogy between superposition of colors and superposition of shapes.Note that the first figure is made up of vertical lines, the second of vertical and horizontal lines, and the third of vertical, horizontal, and diagonal lines. Leon R. Kass recently suggested that the Descartes figures might be replaced by a more modern concept– colors as wavelengths. (Commentary, April 2007). This in turn suggests an analogy to Fourier series decomposition of a waveform in harmonic analysis. See the Kass essay for a discussion of the Descartes figures in the context of (pdf) Science, Religion, and the Human Future (not to be confused with Life, the Universe, and Everything).
Compare and contrast:
The harmonic-analysis analogy suggests a review of an earlier entry’s
link today to 4/30– Structure and Logic— as well as
re-examination of Symmetry and a Trinity
(Dec. 4, 2002).
See also —
A Four-Color Theorem,
The Diamond Theorem, and
The Most Violent Poem,
Comments Off on Wednesday October 24, 2007
Saturday, June 30, 2007
Saturday June 30, 2007
November 2004–
Controversial "Desperate Housewives"… ranks No. 5 among all prime-time shows for ages 12-17. ("Monday Night Football" is No. 18.) This may explain in part why its current advertisers include products like Fisher-Price toys, the DVD of "Elf" and the forthcoming Tim Allen holiday vehicle, "Christmas With the Kranks." Those who cherish the First Amendment can only hope that the Traditional Values Coalition, OneMillionMoms.com, OneMillionDads.com and all the rest send every e-mail they can to the F.C.C. demanding punitive action against the stations that broadcast "Desperate Housewives." A "moral values" crusade that stands between a TV show this popular and its audience will quickly learn the limits of its power in a country where entertainment is god. — "The Great Indecency Hoax," a New York Times column by Frank Rich quoted in Log24 on Nov. 26, 2004 |
The entertainment continues. A rabbi's obituary in today's New York Times (see previous entry) served as ad-bait for "Joshua," a Fox Searchlight film opening July 6.
A search for a less sacrilegious memorial to the rabbi yields the following:
The "Project MUSE" link above
works only at
subscribing libraries.
It seems that here, too,
the rabbi is being
used as bait.
For a perhaps preferable
reference to bait, in the
context of St. Peter as
a "fisher of men," see
the Christian "mandorla"
or "vesica piscis,"
a figure hidden within
the geometry of Rome's
St. Peter's Square–
which, despite its name,
is an oval:
For the geometric
construction of the
Roman oval, see
"ovato tondo" in
Rudolf Arnheim's
The Power of the Center.
For a less theoretical account
of the religious significance
of the mandorla, see
the 2001 film
The Center of the World.
Comments Off on Saturday June 30, 2007
Friday, February 16, 2007
Friday February 16, 2007
“The much-borrowed Brown formula involves some very specific things. The name of a great artist, artifact or historical figure must be in the book’s story, not to mention on its cover. The narrative must start in the present day with a bizarre killing, then use that killing as a reason to investigate the past. And the past must yield a secret so big, so stunning, so saber-rattling that all of civilization may be changed by it. Probably not for the better.
This formula is neatly summarized….”
Cover illustration
for
The Judas Seat:
for
The Judas Seat:
The Narrative:
The Secret:
Part I
“Little ‘Jack’ Horner was actually Thomas Horner, steward to the Abbot of Glastonbury during the reign of King Henry VIII…. Always keen to raise fresh funds, Henry had shown a interest in Glastonbury (and other abbeys). Hoping to appease the royal appetite, the nervous Abbot, Richard Whiting, allegedly sent Thomas Horner to the King with a special gift. This was a pie containing the title deeds to twelve manor houses in the hope that these would deflect the King from acquiring Glastonbury Abbey. On his way to London, the not so loyal courier Horner apparently stuck his thumb into the pie and extracted the deeds for Mells Manor, a plum piece of real estate. The attempted bribe failed and the dissolution of the monasteries (including Glastonbury) went ahead from 1536 to 1540. Richard Whiting was subsequently executed, but the Horner family kept the house, so the moral of this one is: treachery and greed pay off, but bribery is a bad idea.” –Chris Roberts, Heavy Words Lightly Thrown: The Reason Behind the Rhyme
Part II
“The Grail Table has thirteen seats, one of which is kept vacant in memory of Judas Iscariot who betrayed Christ.” —Symbolism of King Arthur’s Round Table
“In medieval romance, the grail was said to have been brought to Glastonbury in Britain by Joseph of Arimathea and his followers. In the time of Arthur, the quest for the Grail was the highest spiritual pursuit.” —The Camelot Project
and the three entries preceding it:
“And what the dead had no speech for, when living,
they can tell you, being dead:
the communication of the dead is tongued with fire
beyond the language of the living.”
they can tell you, being dead:
the communication of the dead is tongued with fire
beyond the language of the living.”
— T. S. Eliot, Four Quartets
Comments Off on Friday February 16, 2007
Tuesday, January 9, 2007
Tuesday January 9, 2007
Logos and Logic
(private, cut from prev. entry)
(private, cut from prev. entry)
| The diamond is used in modal logic to symbolize possibility. |
The 3×3 grid may also be used
to illustrate “possibility.” It leads,
as noted at finitegeometry.org, to
the famed “24-cell,” which may be
pictured either as the diamond
figure from Plato’s Meno —
Click for details.
— or as a figure
with 24 vertices:
Click for details.
The “diamond” version of the
24-cell seems unrelated to the
second version that shows all
vertices and edges, yet the
second version is implicit,
or hidden, in the first.
Hence “possibility.”
Neither version of the 24-cell
seems related in any obvious
way to the 3×3 grid, yet both
versions are implicit,
or hidden, in the grid.
Hence “possibility.”
Comments Off on Tuesday January 9, 2007
Saturday, September 2, 2006
Saturday September 2, 2006
Today's birthdays:
Salma Hayek
("Frida")
"Shinin' like a diamond
she had tombstones
in her eyes."
(For the above figures,
see Log24, 5/17/06,
"Tombstone," and
Log24, 9/13/03,
"For the Man in Black.")
and Keanu Reeves
("Constantine")
(For the above figure,
see Log24, 2/18/05,
"In Hoc Signo.")
Related material:
"Un cofre de gran riqueza
Hallaron dentro un pilar,
Dentro del, nuevas banderas
Con figuras de espantar."
"A coffer of great richness
In a pillar's heart they found,
Within it lay new banners,
With figures to astound."
For some further details, see
the brief Log24 narrative
"Indiana Jones and
the Hidden Coffer" as well as
Symmetry Framed and
the design of the doors
to Rick's Cafe Americain:
Friday, May 19, 2006
Friday May 19, 2006
Star and Diamond
continued
continued
” ‘I know what it is you last saw,’ she said; ‘for that is also in my mind. Do not be afraid! But do not think that only by singing amid the trees, nor even by the slender arrows of elvenbows, is this land of Lothlórien maintained and defended against the Enemy. I say to you, Frodo, that even as I speak to you, I perceive the Dark Lord and know his mind, or all his mind that concerns the Elves. And he gropes ever to see me and my thought. But still the door is closed!’
She lifted up her white arms, and spread out her hands towards the East in a gesture of rejection and denial. Eärendil, the Evening Star, most beloved of the Elves, shone clear above. So bright was it that the figure of the Elven-lady cast a dim shadow on the ground. Its ray glanced upon a ring about her finger; it glittered like polished gold overlaid with silver light, and a white stone in it twinkled as if the Even-star had come to rest upon her hand. Frodo gazed at the ring with awe; for suddenly it seemed to him that he understood.
‘Yes,’ she said, divining his thought, ‘it is not permitted to speak of it, and Elrond could not do so. But it cannot be hidden from the Ring-Bearer, and one who has seen the Eye. Verily it is in the land of Lórien upon the finger of Galadriel that one of the Three remains. This is Nenya, the Ring of Adamant, and I am its keeper.’ ”
— J. R. R. Tolkien, The Lord of the Rings
Related material:
The last 3 entries,
as well as
Mathematics and Narrative
“How much story
do you want?”
— George Balanchine
Comments Off on Friday May 19, 2006
Wednesday, January 4, 2006
Wednesday January 4, 2006
The Shining
The Shining according to
the Catholic Church:
“The Transfiguration of Christ is the culminating point of His public life…. Jesus took with him Peter and James and John and led them to a high mountain apart, where He was transfigured before their ravished eyes. St. Matthew and St. Mark express this phenomenon by the word metemorphothe, which the Vulgate renders transfiguratus est. The Synoptics explain the true meaning of the word by adding ‘his face did shine as the sun: and his garments became white as snow,’ according to the Vulgate, or ‘as light,’ according to the Greek text. This dazzling brightness which emanated from His whole Body was produced by an interior shining of His Divinity.”
— The Catholic Encyclopedia of 1912
The Shining according to
Paul Preuss:
Paul Preuss:
From Broken Symmetries, 1983, Chapter 16:
“He’d toyed with ‘psi’ himself…. The reason he and so many other theoretical physicists were suckers for the stuff was easy to understand– for two-thirds of a century an enigma had rested at the heart of theoretical physics, a contradiction, a hard kernel of paradox….
Peter [Slater] had never thirsted after ‘hidden variables’ to explain what could not be pictured. Mathematical relationships were enough to satisfy him, mere formal relationships which existed at all times, everywhere, at once. It was a thin nectar, but he was convinced it was the nectar of the gods….
Those so-called crazy psychics were too sane, that was their problem– they were too stubborn to admit that the universe was already more bizarre than anything they could imagine in their wildest dreams of wizardry.”
Saturday, November 12, 2005
Saturday November 12, 2005
Glory Season
"…his eyes ranged the Consul's books disposed quite neatly… on high shelves around the walls: Dogme et Ritual de la Haute Magie, Serpent and Siva Worship in Central America, there were two long shelves of this, together with the rusty leather bindings and frayed edges of the numerous cabbalistic and alchemical books, though some of them looked fairly new, like the Goetia of the Lemegaton of Solomon the King, probably they were treasures, but the rest were a heterogeneous collection…."
— Malcolm Lowry, Under the Volcano, Chapter VI
"… when Saul does reach for a slim leather-bound volume Eliza cannot help but feel that something momentous is about to happen. There is care in the way he carries the book on the short journey from its shelf, as if it were constructed not of leather and parchment but of flesh and blood….
"Otzar Eden HaGanuz," Saul says. "The Hidden Eden. In this book, Abulafia describes the process of permutation…. Once you have mastered it, you will have mastered words, and once you have mastered words, you will be ready to receive shefa."
"In the Inner Game, we call the Game Dhum Welur, the Mind of God."
— The Gameplayers of Zan, a novel featuring games based on cellular automata
"Regarding cellular automata, I'm trying to think in what SF books I've seen them mentioned. Off the top of my head, only three come to mind:
The Gameplayers of Zan M.A. Foster
Permutation City Greg Egan
Glory Season David Brin"
— Jonathan L. Cunningham, Usenet
"If all that 'matters' are fundamentally mathematical relationships, then there ceases to be any important difference between the actual and the possible. (Even if you aren't a mathematical Platonist, you can always find some collection of particles of dust to fit any required pattern. In Permutation City this is called the 'logic of the dust' theory.)….
… Paul Durham is convinced by the 'logic of the dust' theory mentioned above, and plans to run, just for a few minutes, a complex cellular automaton (Permutation City) started in a 'Garden of Eden' configuration — one which isn't reachable from any other, and which therefore must have been the starting point of a simulation…. I didn't understand the need for this elaborate set-up, but I guess it makes for a better story than 'well, all possible worlds exist, and I'm going to tell you about one of them.'"
— Danny Yee, review of Permutation City
"Y'know, I never imagined the competition version involved so many tricky permutations."
— David Brin, Glory Season, 1994 Spectra paperback, p. 408
Related material:
|
Figure 2
"… matter is consciousness expressed in the intermixing of force and form, but so heavily structured and constrained by form that its behaviour becomes describable using the regular and simple laws of physics. This is shown in Figure 2. |
Figure 3
"This quaternary is a Kabbalistic representation of God-the-Knowable, in the sense that it the most abstract representation of God we are capable of comprehending…. — A Depth of Beginning: Notes on Kabbalah by Colin Low (pdf) |
See also
Cognitive Blending and the Two Cultures,
Mathematics and Narrative,
Deep Game,
and the previous entry.
Comments Off on Saturday November 12, 2005
Tuesday, July 5, 2005
Tuesday July 5, 2005
For Christopher Fry
and the White Goddess:
The Edge of Eternity
Christian humanist playwright Christopher Fry, author of The Lady’s Not for Burning, died at 97 on June 30, 2005.
From Log24 on June 30:
Robert Graves, author of
The White Goddess:
A Historical Grammar of Poetic Myth—
How may the King hold back?
Royally then he barters life for love.
Or of the undying snake from chaos hatched,
Whose coils contain the ocean,
Into whose chops with naked sword he springs,
Then in black water, tangled by the reeds,
Battles three days and nights…
From Cold Mountain:
“He sat awhile on a rock, and then got up and walked all morning through the dim woods. The track was ill used, so coiled and knotted he could not say what its general tendency was. It aimed nowhere certain but up. The brush and bracken grew thick in the footway, and the ground seemed to be healing over, so that in some near future the way would not even remain as scar. For several miles it mostly wound its way through a forest of immense hemlocks, and the fog lay among them so thick that their green boughs were hidden. Only the black trunks were visible, rising into the low sky like old menhirs stood up by a forgotten race to memorialize the darkest events of their history….
They climbed to a bend and from there they walked on great slabs of rock. It seemed to Inman that they were at the lip of a cliff, for the smell of the thin air spoke of considerable height, though the fog closed off all visual check of loftiness….
Then he looked back down and felt a rush of vertigo as the lower world was suddenly revealed between his boot toes. He was indeed at the lip of a cliff, and he took one step back…. The country around was high, broken. Inman looked about and was startled to see a great knobby mountain forming up out of the fog to the west, looming into the sky. The sun broke through a slot in the clouds, and a great band of Jacob’s ladder suddenly hung in the air like a gauze curtain between Inman and the blue mountain….
Inman looked at the big grandfather mountain and then he looked beyond it to the lesser mountains as they faded off into the southwest horizon, bathed in faint smoky haze. Waves of mountains. For all the evidence the eye told, they were endless. The grey overlapping humps of the farthest peaks distinguished themselves only as slightly darker values of the pale grey air. The shapes and their ghostly appearance spoke to Inman in a way he could not clearly interpret. They graded off like the tapering of pain from the neck wound as it healed.”
See also the entries of July 3.
The crone figure in this section of Cold Mountain is not entirely unrelated to the girl accused of being a witch in Fry’s play and to Graves’s White Goddess.
From Fry’s obituary in The Guardian:
“Though less of a public theorist than Eliot, Fry still believed passionately in the validity of poetic drama. As he wrote in the magazine Adam: ‘In prose, we convey the eccentricity of things, in poetry their concentricity, the sense of relationship between them: a belief that all things express the same identity and are all contained in one discipline of revelation.'”
From Fry’s obituary in today’s New York Times:
“His plays radiated an optimistic faith in God and humanity, evoking, in his words, ‘a world in which we are poised on the edge of eternity, a world which has deeps and shadows of mystery, and God is anything but a sleeping partner.’ He said he wrote his plays in poetry because that was ‘the language in which man expresses his own amazement’ at the complexity both of himself and of a reality which, beneath the surface, was ‘wildly, perilously, inexplicably fantastic.'”
Comments Off on Tuesday July 5, 2005
Saturday, May 22, 2004
Saturday May 22, 2004
Star Wars
In memory of Melvin J. Lasky, editor, 1958-1990, of the CIA-funded journal Encounter:
“Once called as lively, and as bitchy, as a literary cocktail party, Encounter published articles of unrivalled authority on politics, history and literature.”
Lasky died on Wednesday, May 19, 2004. From a journal entry of my own on that date:
This newly-digitized diagram is from a
paper journal note of October 21, 1999.
Note that the diagram’s overall form is that of an eight-point star. Here is an excerpt from a Fritz Leiber story dealing with such a star, the symbol of a fictional organization:
|
Time traveling, which is not quite the good clean boyish fun it’s cracked up to be, started for me when this woman with the sigil on her forehead looked in on me from the open doorway of the hotel bedroom where I’d hidden myself and the bottles and asked me, “Look, Buster, do you want to live?” Her right arm was raised and bent, the elbow touching the door frame, the hand brushing back the very dark bangs from her forehead to show me the sigil, as if that had a bearing on her question. The sigil was an eight-limbed asterisk made of fine dark lines and about as big as a silver dollar. An X superimposed on a plus sign. It looked permanent. … “Here is how it stacks up: You’ve bought your way with something other than money into an organization of which I am an agent….” “It’s a very big organization,” she went on, as if warning me. “Call it an empire or a power if you like. So far as you are concerned, it has always existed and always will exist. It has agents everywhere, literally. Space and time are no barriers to it. Its purpose, so far as you will ever be able to know it, is to change, for its own aggrandizement, not only the present and the future, but also the past. It is a ruthlessly competitive organization and is merciless to its employees.” “I. G. Farben?” I asked grabbing nervously and clumsily at humor. She didn’t rebuke my flippancy, but said, “And it isn’t the Communist Party or the Ku Klux Klan, or the Avenging Angels or the Black Hand, either, though its enemies give it a nastier name.” “Which is?” I asked. “The Spiders,” she said. That word gave me the shudders, coming so suddenly. I expected the sigil to step off her forehead and scuttle down her face and leap at me—something like that. She watched me. “You might call it the Double Cross,” she suggested, “if that seems better.” — Fritz Leiber, |
From last year’s entry,
Indiana Jones and the Hidden Coffer,
of 6/14:
|
From Borges’s “The Aleph“:
From The Hunchback of Notre Dame:
Lena Olin and Harrison Ford |
Finally, from an excellent site
on the Knights Templar,
a quotation from Umberto Eco:
|
When all the archetypes burst out shamelessly, we plumb the depths of Homeric profundity. Two cliches make us laugh but a hundred cliches move us because we sense dimly that the cliches are talking among themselves, celebrating a reunion . . . Just as the extreme of pain meets sensual pleasure, and the extreme of perversion borders on mystical energy, so too the extreme of banality allows us to catch a glimpse of the Sublime. — “Casablanca: Cult Movies and Intertextual Collage” (1984) from Travels in Hyperreality |
Comments Off on Saturday May 22, 2004
Friday, April 30, 2004
Friday April 30, 2004
Library
These are the folios of April,
All the library of spring
The above quotation is dedicated to Quay A. McCune, M.D., whose copy of Bartlett's Familiar Quotations I purchased for two dollars at a Friends of the Library sale on July 2, 1999. Dr. McCune's copy of Bartlett was the twelfth edition, of November 1948, in a February 1952 reprint. It was edited by Christopher Morley.
Incidentally, Morley's father Frank, a professor of mathematics, is the discoverer of Morley's theorem, which says that the angle trisectors of any triangle, of whatever shape, determine an equilateral "Morley triangle" hidden within the original triangle.
Those familiar with Dorothy Sayers's explication of the Trinity, The Mind of the Maker, will recognize that this figure represents a triumph over the heresies she so skillfully describes in the chapter "Scalene Trinities." From another chapter:
"… this is the Idea that is put forward for our response. There is nothing mythological about Christian Trinitarian doctrine: it is analogical. It offers itself freely for meditation and discussion; but it is desirable that we should avoid the bewildered frame of mind of the apocryphal Japanese gentleman who complained:
'Honourable Father, very good;
Honourable Son, very good; but
Honourable Bird
I do not understand at all.'
'Honourable Bird,' however, has certain advantages as a pictorial symbol, since, besides reminding us of those realities which it does symbolise, it also reminds us that the whole picture is a symbol and no more."
In the Morley family trinity, if Frank is the Father and Christopher is the Son, we must conclude that the Holy Spirit is Christopher's mother — whose maiden name was, appropriately, Bird.
Comments Off on Friday April 30, 2004
Friday, April 16, 2004
Friday April 16, 2004
Mistakes Were Made
By Al Kamen, Washington Post
Friday, April 16, 2004
“… Bush, in his news conference Tuesday…. found a way to make not one, not two, but three factual errors in a single 15-word sentence, which must be something of a world indoor record. Bush said it is still possible that inspectors will find weapons of mass destruction in Iraq.
‘They could still be there. They could be hidden, like the 50 tons of mustard gas in a turkey farm,’ he said, referring to Libya’s WMD disclosures last month.
The White House, according to Reuters, said the accurate figure was 23.6 metric tons or 26 tons, not 50. The stuff was found at various locations, not at a turkey farm. And there was no mustard gas on the farm at all, but unfilled chemical munitions.
Other than that, the sentence was spot on.”
Other mistakes …
“It’s not at all like CIA Director George J. Tenet to forget not one, but two, conversations with President Bush in the critical month [August] before Sept. 11, 2001. But there’s one possible explanation for his distraction when he testified Wednesday morning to the Sept. 11 commission: He was thinking about his luncheon plans.
Tenet was spotted around 12:30 at the Hay-Adams, sitting at a window table for two with none other than Jack Valenti, outgoing head of the Motion Picture Association of America….”
Hey, that’s why
they make erasers.
Comments Off on Friday April 16, 2004
Saturday, June 14, 2003
Saturday June 14, 2003
 |
Indiana Jones |
In memory of Bernard Williams,
Oxford philosopher, who died Tuesday, June 10, 2003.
“…in… Truth and Truthfulness [September, 2002], he sought to speak plainly, and took on the post-modern, politically correct notion that truth is merely relative…”
“People have always longed for truths about the world — not logical truths, for all their utility; or even probable truths, without which daily life would be impossible; but informative, certain truths, the only ‘truths’ strictly worthy of the name. Such truths I will call ‘diamonds’; they are highly desirable but hard to find….
A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes…. My own viewpoint is the Story Theory….”
— Richard J. Trudeau, The Non-Euclidean Revolution, Birkhauser Boston, 1987
Today is the feast day of Saint Jorge Luis Borges (b. Buenos Aires, August 24, 1899 – d. Geneva, June 14, 1986).
From Borges’s “The Aleph“:
“The Faithful who gather at the mosque of Amr, in Cairo, are acquainted with the fact that the entire universe lies inside one of the stone pillars that ring its central court…. The mosque dates from the seventh century; the pillars come from other temples of pre-Islamic religions…. Does this Aleph exist in the heart of a stone?”
(“Los fieles que concurren a la mezquita de Amr, en el Cairo, saben muy bien que el universo está en el interior de una de las columnas de piedra que rodean el patio central…. la mezquita data del siglo VII; las columnas proceden de otros templos de religiones anteislámicas…. ¿Existe ese Aleph en lo íntimo de una piedra?”)
From The Hunchback of Notre Dame:
Un cofre de gran riqueza
Hallaron dentro un pilar,
Dentro del, nuevas banderas
Con figuras de espantar.*
* A coffer of great richness
In a pillar’s heart they found,
Within it lay new banners,
With figures to astound.
See also the figures obtained by coloring and permuting parts of the above religious symbol.
Lena Olin and Harrison Ford
in “Hollywood Homicide“
Friday, March 28, 2003
Friday March 28, 2003
Bright Star
From a Spanish-English dictionary:
lucero m. morning or evening star: any bright star….
Today is Reba McEntire's birthday.
" 'I know what it is you last saw,' she said; 'for that is also in my mind. Do not be afraid! But do not think that only by singing amid the trees, nor even by the slender arrows of elven-bows, is this land of Lothlórien maintained and defended against the Enemy. I say to you, Frodo, that even as I speak to you, I perceive the Dark Lord and know his mind, or all his mind that concerns the Elves. And he gropes ever to see me and my thought. But still the door is closed!'
She lifted up her white arms, and spread out her hands towards the East in a gesture of rejection and denial. Eärendil, the Evening Star, most beloved of the Elves, shone clear above. So bright was it that the figure of the Elven-lady cast a dim shadow on the ground. Its ray glanced upon a ring about her finger; it glittered like polished gold overlaid with silver light, and a white stone in it twinkled as if the Even-star had come to rest upon her hand. Frodo gazed at the ring with awe; for suddenly it seemed to him that he understood.
'Yes', she said, divining his thought, 'it is not permitted to speak of it, and Elrond could not do so. But it cannot be hidden from the Ring-Bearer, and one who has seen the Eye. Verily it is in the land of Lórien upon the finger of Galadriel that one of the Three remains. This is Nenya, the Ring of Adamant, and I am its keeper.' "
— J. R. R. Tolkien, The Lord of the Rings
Related material on telepathy:
Shining Forth and Naturalized Epistemology
Related material on rings, and another musical Reba:
Leonard Gillman interview, Part I and Part II
Gillman, a pianist, is co-author of Rings of Continuous Functions.
Comments Off on Friday March 28, 2003
Sunday, September 15, 2002
Sunday September 15, 2002
Evariste Galois and
The Rock That Changed Things
An article in the current New York Review of Books (dated Sept. 26) on Ursula K. Le Guin prompted me to search the Web this evening for information on a short story of hers I remembered liking. I found the following in the journal of mathematician Peter Berman:
- A Fisherman of the Inland Sea, Ursula K. Le Guin, 1994:
A book of short stories. Good, entertaining. I especially liked “The Rock That Changed Things.” This story is set in a highly stratified society, one split between elite and enslaved populations. In this community, the most important art form is a type of mosaic made from rocks, whose patterns are read and interpreted by scholars from the elite group. The main character is a slave woman who discovers new patterns in the mosaics. The story is slightly over-the-top but elegant all the same.
I agree that the story is elegant (from a mathematician, a high compliment), so searched Berman’s pages further, finding this:
between The French Mathematician (a novel about Galois) and Harry Potter and the Sorcerer’s Stone.
My own version of the Philosopher’s Stone (the phrase used instead of “Sorcerer’s Stone” in the British editions of Harry Potter) appears in my profile picture at top left; see also the picture of Plato’s diamond figure in my main math website. The mathematics of finite (or “Galois”) fields plays a role in the underlying theory of this figure’s hidden symmetries. Since the perception of color plays a large role in the Le Guin story and since my version of Plato’s diamond is obtained by coloring Plato’s version, this particular “rock that changes things” might, I hope, inspire Berman to extend his table to include Le Guin’s tale as well.
Even the mosaic theme is appropriate, this being the holiest of the Mosaic holy days.
Dr. Berman, G’mar Chatimah Tova.
Tuesday, July 30, 2002
Tuesday July 30, 2002
Aesthetics of Madness
Admirers of the film "A Beautiful Mind" may be interested in the thoughts of psychotherapist Eric Olson on what he calls the "collage method" of therapy. The fictional protagonist of "A Beautiful Mind," very loosely based on the real-life mathematician John Nash, displays his madness in a visually striking manner (as required by cinematic art). He makes enormous collages of published matter in which he believes he has found hidden patterns.
This fictional character is in some ways more like the real-life therapist Olson than like the real-life schizophrenic Nash. For an excellent introduction to Olson's world, see the New York Times Magazine article of April 1, 2001, on Olson and on the mysterious death of Olson's father Frank, who worked for the CIA. Here the plot thickens… the title of the article is "What Did the C.I.A. Do to Eric Olson's Father?"
For Olson's own website, see The Frank Olson Legacy Project, which has links to Olson's work on collage therapy. Viewed in the context of this website, the resemblance of Olson's collages to the collages of "A Beautiful Mind" is, to borrow Freud's expression, uncanny. Olson's own introduction to his collage method is found on the web page "Theory and therapy."
All of the above resulted from a Google search to see if Arlene Croce's 1993 New Yorker article on Balanchine and Stravinsky, "The Spelling of Agon," could be found online. I did not find Arlene, but I did find the following, from a collage of quotations assembled by Eric Olson —
"There might be a game in which paper figures were put together to form a story, or at any rate were somehow assembled. The materials might be collected and stored in a scrap-book, full of pictures and anecdotes. The child might then take various bits from the scrap-book to put into the construction; and he might take a considerable picture because it had something in it which he wanted and he might just include the rest because it was there.”
— Ludwig Wittgenstein,
Lectures and Conversations on Aesthetics, Psychology and Religious Belief, 1943/1978
“Not games. Puzzles. Big difference. That’s a whole other matter. All art — symphonies, architecture, novels — it’s all puzzles. The fitting together of notes, the fitting together of words have by their very nature a puzzle aspect. It’s the creation of form out of chaos. And I believe in form.”— Stephen Sondheim
in Stephen Schiff, “Deconstructing Sondheim,”
The New Yorker, March 8, 1993, p. 76.
“God creates, I assemble.”— George Balenchine [sic]
in Arlene Croce, “The Spelling of Agon,”
The New Yorker, July 12, 1993, p. 91
The aesthetics of collage is, of course, not without its relevance to the creation (or assembly) of weblogs.
Comments Off on Tuesday July 30, 2002
Saturday, July 20, 2002
Saturday July 20, 2002
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.