Today the World Wide Web turns 20.
|
|
See also Galois Memorial and Correspondences.
"Of course, the aesthetic program
of cultural modernism
has long been summed up
by the maxim épater la bourgeoisie."
— The New York Times
Sunday Book Review, July 17
Examples:
"This Extreme and Difficult Sense of Spectacular Representation:
Antonin Artaud's Ontology of 'Live'," by Deborah Levitt
of the New School (See the noon post of July 13), as well as…
and, from mathematician Ellen Gethner's home page—
See also Sunday Dinner, A Link for Sunrise, and Inside CBS News.
Philosophical Investigations (1953)—
97. Thought is surrounded by a halo.
—Its essence, logic, presents an order,
in fact the a priori order of the world:
that is, the order of possibilities * ,
which must be common to both world and thought.
But this order, it seems, must be
utterly simple . It is prior to all experience,
must run through all experience;
no empirical cloudiness or uncertainty can be allowed to affect it
——It must rather be of the purest crystal.
But this crystal does not appear as an abstraction;
but as something concrete, indeed, as the most concrete,
as it were the hardest thing there is
(Tractatus Logico-Philosophicus No. 5.5563).
— Translation by G.E.M. Anscombe
All propositions of our colloquial language
are actually, just as they are, logically completely in order.
That simple thing which we ought to give here is not
a model of the truth but the complete truth itself.
(Our problems are not abstract but perhaps
the most concrete that there are.)
97. Das Denken ist mit einem Nimbus umgeben.
—Sein Wesen, die Logik, stellt eine Ordnung dar,
und zwar die Ordnung a priori der Welt,
d.i. die Ordnung der Möglichkeiten ,
die Welt und Denken gemeinsam sein muß.
Diese Ordnung aber, scheint es, muß
höchst einfach sein. Sie ist vor aller Erfahrung;
muß sich durch die ganze Erfahrung hindurchziehen;
ihr selbst darf keine erfahrungsmäßige Trübe oder Unsicherheit anhaften.
——Sie muß vielmehr vom reinsten Kristall sein.
Dieser Kristall aber erscheint nicht als eine Abstraktion;
sondern als etwas Konkretes, ja als das Konkreteste,
gleichsam Härteste . (Log. Phil. Abh. No. 5.5563.)
Related language in Łukasiewicz (1937)—
* Updates of 9:29 PM ET July 10, 2011—
A mnemonic from a course titled “Galois Connections and Modal Logics“—
“Traditionally, there are two modalities, namely, possibility and necessity.
The basic modal operators are usually written ![]() (square) for necessarily
(square) for necessarily
and ![]() (diamond) for possibly. Then, for example,
(diamond) for possibly. Then, for example, ![]() P can be read as
P can be read as
‘it is possibly the case that P .'”
| See also Intensional Semantics , lecture notes by Kai von Fintel and Irene Heim, MIT, Spring 2007 edition—
“The diamond ⋄ symbol for possibility is due to C.I. Lewis, first introduced in Lewis & Langford (1932), but he made no use of a symbol for the dual combination ¬⋄¬. The dual symbol □ was later devised by F.B. Fitch and first appeared in print in 1946 in a paper by his doctoral student Barcan (1946). See footnote 425 of Hughes & Cresswell (1968). Another notation one finds is L for necessity and M for possibility, the latter from the German möglich ‘possible.’” Barcan, Ruth C.: 1946. “A Functional Calculus of First Order Based on Strict Implication.” Journal of Symbolic Logic, 11(1): 1–16. URL http://www.jstor.org/pss/2269159. Hughes, G.E. & Cresswell, M.J.: 1968. An Introduction to Modal Logic. London: Methuen. Lewis, Clarence Irving & Langford, Cooper Harold: 1932. Symbolic Logic. New York: Century. |
From "Sunday Dinner" in this journal—
"'If Jesus were to visit us, it would have been
the Sunday dinner he would have insisted on
being a part of, not the worship service at the church.'"
—Judith Shulevitz at The New York Times
on Sunday, July 18, 2010
Some table topics—
Today's midday New York Lottery numbers were 027 and 7002.
The former suggests a Galois cube, the latter a course syllabus—
CSC 7002
Graduate Computer Security (Spring 2011)
University of Colorado at Denver
Department of Computer Science
An item from that syllabus:
| Six | 22 February 2011 | DES | History of DES; Encryption process; Decryption; Expander function; S-boxes and their output; Key; the function f that takes the modified key and part of the text as input; mulitple Rounds of DES; Present-day lack of Security in DES, which led to the new Encryption Standard, namely AES. Warmup for AES: the mathematics of Fields: Galois Fields, particularly the one of order 256 and its relation to the irreducible polynomial x^8 + x^4 + x^3 + x + 1 with coefficients from the field Z_2. |
Related material: A novel, PopCo , was required reading for the course.
Discuss a different novel by the same author—
Discuss the author herself, Scarlett Thomas.
Background for the discussion—
Derrida in this journal versus Charles Williams in this journal.
Related topics from the above syllabus date—
Metaphor and Gestell and Quadrat.
Some context— Midsummer Eve's Dream.
Continued from March 10, 2011 — A post that says
"If Galois geometry is thought of as a paradigm shift
from Euclidean geometry, both… the Kuhn cover
and the nine-point affine plane may be viewed…
as illustrating the shift."
Yesterday's posts The Fano Entity and Theology for Antichristmas,
together with this morning's New York Times obituaries (below)—
—suggest a Sunday School review from last year's
Devil's Night (October 30-31, 2010)—
|
Sunday, October 31, 2010 ART WARS – m759 @ 2:00 AM … There is a Cave – Paradise Lost , by John Milton
|
See also Ash Wednesday Surprise and Geometry for Jews.
The LA Times on last weekend's film "Thor"—
"… the film… attempts to bridge director Kenneth Branagh's high-minded Shakespearean intentions with Marvel Entertainment's bottom-line-oriented need to crank out entertainment product."
Those averse to Nordic religion may contemplate a different approach to entertainment (such as Taymor's recent approach to Spider-Man).
A high-minded— if not Shakespearean— non-Nordic approach to groups acting—
"What was wrong? I had taken almost four semesters of algebra in college. I had read every page of Herstein, tried every exercise. Somehow, a message had been lost on me. Groups act . The elements of a group do not have to just sit there, abstract and implacable; they can do things, they can 'produce changes.' In particular, groups arise naturally as the symmetries of a set with structure. And if a group is given abstractly, such as the fundamental group of a simplical complex or a presentation in terms of generators and relators, then it might be a good idea to find something for the group to act on, such as the universal covering space or a graph."
— Thomas W. Tucker, review of Lyndon's Groups and Geometry in The American Mathematical Monthly , Vol. 94, No. 4 (April 1987), pp. 392-394
"Groups act "… For some examples, see
Related entertainment—
High-minded— Many Dimensions—

Not so high-minded— The Cosmic Cube—

One way of blending high and low—
The high-minded Charles Williams tells a story
in his novel Many Dimensions about a cosmically
significant cube inscribed with the Tetragrammaton—
the name, in Hebrew, of God.
The following figure can be interpreted as
the Hebrew letter Aleph inscribed in a 3×3 square—
The above illustration is from undated software by Ed Pegg Jr.
For mathematical background, see a 1985 note, "Visualizing GL(2,p)."
For entertainment purposes, that note can be generalized from square to cube
(as Pegg does with his "GL(3,3)" software button).
For the Nordic-averse, some background on the Hebrew connection—
The late translator Helen Lane in Translation Review , Vol. 5, 1980—
"Among the awards, I submit, should be one for the entire oeuvre of a lifetime "senior" translator— and one for the best first translation…. Similar organization, cooperation, and fund-finding for a first-rate replacement for the sorely missed Delos ."
This leads to one of the founders of Delos , the late Donald Carne-Ross, who died on January 9, 2010.
For one meditation on the date January 9, see Bridal Birthday (last Thursday).
Another meditation, from the date of Carne-Ross's death—
Saturday, January 9, 2010
Positional Meaning"The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole." – Victor Turner, The Forest of Symbols , Ithaca, NY, Cornell University Press, 1967, p. 51, quoted by Beth Barrie in "Victor Turner." To everything, turn, turn, turn … |
See also Delos in this journal.
Part I — Unity and Multiplicity
(Continued from The Talented and Galois Cube)
Part II — "A feeling, an angel, the moon, and Italy"—
Today's earlier post mentions one approach to the concepts of unity and multiplicity. Here is another.

Unity:
The 3×3×3 Galois Cube
Multiplicity:
One of a group, GL(3,3), of 11,232
natural transformations of the 3×3×3 Cube
See also the earlier 1985 3×3 version by Cullinane.
For the title, see Palm Sunday.
"There is a pleasantly discursive treatment of
Pontius Pilate's unanswered question 'What is truth?'" — H. S. M. Coxeter, 1987
From this date (April 22) last year—
 Richard J. Trudeau in The Non-Euclidean Revolution , chapter on "Geometry and the Diamond Theory of Truth"– "… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions: (1) Diamonds– informative, certain truths about the world– exist. Presumption (1) is what I referred to earlier as the 'Diamond Theory' of truth. It is far, far older than deductive geometry." Trudeau's book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called "Diamond Theory." Although non-Euclidean,* the theorems of the 1976 "Diamond Theory" are also, in Trudeau's terminology, diamonds. * "Non-Euclidean" here means merely "other than Euclidean." No violation of Euclid's parallel postulate is implied. |
Trudeau comes to reject what he calls the "Diamond Theory" of truth. The trouble with his argument is the phrase "about the world."
Geometry, a part of pure mathematics, is not about the world. See G. H. Hardy, A Mathematician's Apology .
Today's news from Oslo suggests a review—
The circular sculpture in the foreground
is called by the artist "The Omega Point."
This has been described as
"a portal that leads in or out of time and space."
Some related philosophical remarks—
The following has rather mysteriously appeared in a search at Google Scholar for "Steven H. Cullinane."
|
[HTML] Romancing the Non-Euclidean Hyperspace |
This turns out to be a link to a search within this weblog. I do not know why Google Scholar attributes the resulting web page to a journal article by "AB Story" or why it drew the title from a post within the search and applied it to the entire list of posts found. I am, however, happy with the result— a Palm Sunday surprise with an eclectic mixture of styles that might please the late Robert de Marrais.
I hope the late George Temple would also be pleased. He appears in "Romancing" as a resident of Quarr Abbey, a Benedictine monastery.
The remarks by Martin Hyland quoted in connection with Temple's work are of particular interest in light of the Pope's Christmas remark on mathematics quoted here yesterday.
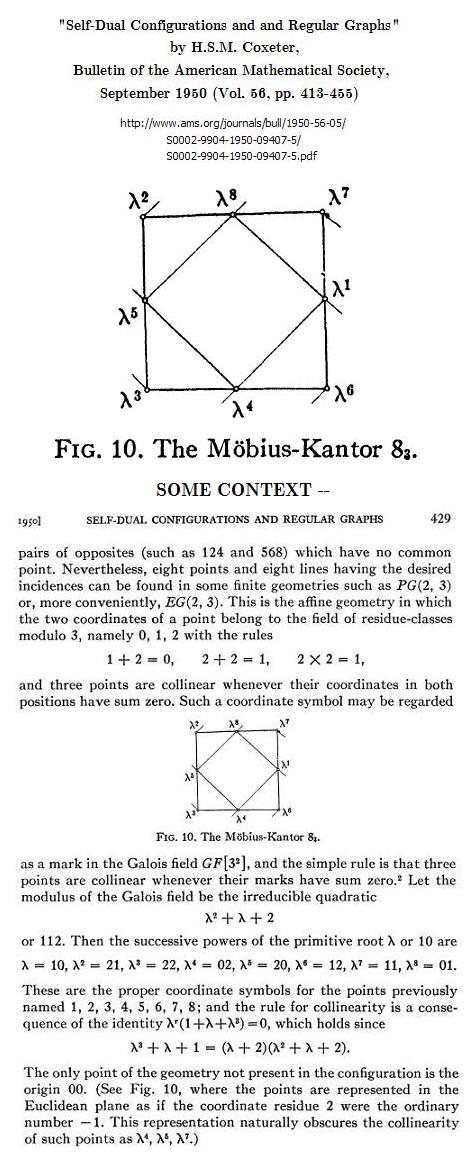
From last night's note on finite geometry—
"The (83, 83) Möbius-Kantor configuration here described by Coxeter is of course part of the larger (94, 123) Hesse configuration. Simply add the center point of the 3×3 Galois affine plane and the four lines (1 horizontal, 1 vertical, 2 diagonal) through the center point." An illustration—

This suggests a search for "diamond+star."
The On-Line Encyclopedia of Integer Sequences has an article titled "Number of combinatorial configurations of type (n_3)," by N.J.A. Sloane and D. Glynn.
From that article:
The following corrects the word "unique" in the example.

* This post corrects an earlier post, also numbered 14660 and dated 7 PM March 18, 2011, that was in error.
The correction was made at about 11:50 AM on March 20, 2011.
_____________________________________________________________
Update of March 21
The problem here is of course with the definition. Sloane and Glynn failed to include in their definition a condition that is common in other definitions of configurations, even abstract or purely "combinatorial" configurations. See, for instance, Configurations of Points and Lines , by Branko Grunbaum (American Mathematical Society, 2009), p. 17—
In the most general sense we shall consider combinatorial (or abstract) configurations; we shall use the term set-configurations as well. In this setting "points" are interpreted as any symbols (usually letters or integers), and "lines" are families of such symbols; "incidence" means that a "point" is an element of a "line". It follows that combinatorial configurations are special kinds of general incidence structures. Occasionally, in order to simplify and clarify the language, for "points" we shall use the term marks, and for "lines" we shall use blocks. The main property of geometric configurations that is preserved in the generalization to set-configurations (and that characterizes such configurations) is that two marks are incident with at most one block, and two blocks with at most one mark.
Whether or not omitting this "at most one" condition from the definition is aesthetically the best choice, it dramatically changes the number of configurations in the resulting theory, as the above (8_3) examples show.
Update of March 22 (itself updated on March 25)
For further background on configurations, see Dolgachev—

Note that the two examples Dolgachev mentions here, with 16 points and 9 points, are not unrelated to the geometry of 4×4 and 3×3 square arrays. For the Kummer and related 16-point configurations, see section 10.3, "The Three Biplanes of Order 4," in Burkard Polster's A Geometrical Picture Book (Springer, 1998). See also the 4×4 array described by Gordon Royle in an undated web page and in 1980 by Assmus and Sardi. For the Hesse configuration, see (for instance) the passage from Coxeter quoted in Quaternions in an Affine Galois Plane.
Update of March 27
See the above link to the (16,6) 4×4 array and the (16,6) exercises using this array in R.D. Carmichael's classic Introduction to the Theory of Groups of Finite Order (1937), pp. 42-43. For a connection of this sort of 4×4 geometry to the geometry of the diamond theorem, read "The 2-subsets of a 6-set are the points of a PG(3,2)" (a note from 1986) in light of R.W.H.T. Hudson's 1905 classic Kummer's Quartic Surface , pages 8-9, 16-17, 44-45, 76-77, 78-79, and 80.
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—

This journal on January 19, 2011—

If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
From Galleri MGM in Oslo —
A connection to today's earlier post, Sunday School— The Oslo Version, from Friday, May 21, 2010.
Lyche's "Omega Point" portal, together with her last name, suggested three posts from the following Saturday morning— which later proved to be the date of Martin Gardner's death—
Art Space, Through the Lyche Gate and The Lyche Gate Asterisk.
For some further religious remarks, see November 9th, 2010— A Theory of Pure Design.
From Epiphany Revisited —

A star figure and the Galois quaternion.
The square root of the former is the latter.
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
"Plato acknowledges how khora challenges our normal categories
of rational understanding. He suggests that we might best approach it
through a kind of dream consciousness."
—Richard Kearney, quoted here yesterday afternoon
"You make me feel like I'm living a teenage dream."
— Song at last night's Grammy awards
Richard Kiley in "Blackboard Jungle" (1955)
Note the directive on the blackboard.
Quoted here last year on this date—
Alexandre Borovik's Mathematics Under the Microscope (American Mathematical Society, 2010)—
"Once I mentioned to Gelfand that I read his Functions and Graphs ; in response, he rather sceptically asked me what I had learned from the book. He was delighted to hear my answer: 'The general principle of always looking at the simplest possible example.'….
So, let us look at the principle in more detail:
Always test a mathematical theory on the simplest possible example…
This is a banality, of course. Everyone knows it; therefore, almost no one follows it."
Related material— Geometry Simplified and A Simple Reflection Group of Order 168.
"Great indeed is the riddle of the universe.
Beautiful indeed is the source of truth."
– Shing-Tung Yau, Chairman,
Department of Mathematics, Harvard University
"Always keep a diamond in your mind."
– King Solomon at the Paradiso
Image from stoneship.org
The sliding window in blue below
Click for the web page shown.
is an example of a more general concept.
Such a sliding window,* if one-dimensional of length n , can be applied to a sequence of 0's and 1's to yield a sequence of n-dimensional vectors. For example— an "m-sequence" (where the "m" stands for "maximum length") of length 63 can be scanned by a length-6 sliding window to yield all possible 6-dimensional binary vectors except (0,0,0,0,0,0).
For details, see A Galois Field—

The image is from Bert Jagers at his page on the Galois field GF(64) that he links to as "A Field of Honor."
For a discussion of the m-sequence shown in circular form above, see Jagers's "Pseudo-Random Sequences from GF(64)." Here is a noncircular version of the length-63 m-sequence described by Jagers (with length scale below)—
100000100001100010100111101000111001001011011101100110101011111
123456789012345678901234567890123456789012345678901234567890123
This m-sequence may be viewed as a condensed version of 63 of the 64 I Ching hexagrams. (See related material in this journal.)
For a more literary approach to the window concept, see The Seventh Symbol (scroll down after clicking).
* Moving windows also appear (in a different way) In image processing, as convolution kernels .
Indiana Jones and the Magical Oracle
Mathematician Ken Ono in the December 2010 American Mathematical Society Notices—

The "dying genius" here is Ramanujan, not Galois. The story now continues at the AMS website—

(Excerpt from Jan. 27 screenshot;
the partitions story has been the top
news item at the site all week.)
From a Jan. 20, 2011, Emory University press release —
"Finite formula found for partition numbers" —
"We found a function, that we call P, that is like a magical oracle," Ono says. "I can take any number, plug it into P, and instantly calculate the partitions of that number. P does not return gruesome numbers with infinitely many decimal places. It's the finite, algebraic formula that we have all been looking for."
For an introduction to the magical oracle, see a preprint, "Bruinier-Ono," at the American Institute of Mathematics website.
Ono also discussed the oracle in a video (see minute 25) recorded Jan. 21 and placed online today.
See as well "Exact formulas for the partition function?" at mathoverflow.net.
A Nov. 29, 2010, remark by Thomas Bloom on that page leads to a 2006 preprint by Ono and Kathrin Bringmann, "An Arithmetic Formula for the Partition Function*," that seems not unrelated to Ono's new "magical oracle" formula—
The Bruinier-Ono paper does not mention the earlier Bringmann-Ono work.
(Both the 2011 Bruinier-Ono paper and the 2006 Bringmann-Ono paper mention their debt to a 2002 work by Zagier— Don Zagier, "Traces of singular moduli," in Motives, Polylogarithms and Hodge theory, Part II (Irvine, CA, 1998), International Press Lecture Series 3 (International Press, Somerville, MA, 2002), pages 211-244.)
Some background for those who prefer mathematics to narrative—
The Web of Modularity: Arithmetic of the Coefficients of Modular Forms and q-Series ,
by Ken Ono, American Mathematical Society CBMS Series, 2004.
The following is a new illustration for Cubist Geometries—

(For elementary cubism, see Pilate Goes to Kindergarten and The Eightfold Cube.
For advanced, see Solomon's Cube and Geometry of the I Ching .)
"Spaces and geometries, those which we perceive,
which we can’t perceive, or which only some of us perceive,
are a recurring theme in Against the Day ."
"大哉大哉 宇宙之谜
美哉美哉 真理之源"
"Great indeed is the riddle of the universe.
Beautiful indeed is the source of truth."
— Shing-Tung Yau, Chairman,
Department of Mathematics, Harvard University
"Always keep a diamond in your mind."
— King Solomon at the Paradiso
Image from stoneship.org
Galois Meets Doctor Faustus
Galois's theory of mathematical ambiguity (see June 14) —
My principal meditations for some time have been directed towards
the application of the theory of ambiguity to transcendental
analysis. It was a question of seeing a priori in a relation
between quantities or transcendent functions, what exchanges one
could make, which quantities one could substitute for the given
quantities without the original relation ceasing to hold. That
immediately made clear the impossibility of finding many expressions
that one could look for. But I do not have time and my ideas are
not yet well developed on this ground which is immense.
— Evariste Galois, testamentary letter, translated by James Dolan
Thomas Mann on musical ambiguity in his novel Doctor Faustus —
Related material — Some context for the above and some remarks on the German original.
Julie Taymor in an interview published Dec. 12 —
“I’ve got two Broadway shows, a feature film, and Mozart,’’ she said. “It’s a very interesting place to be and to be able to move back and forth, but at a certain point you have to be able to step outside and see,’’ and here she dropped her voice to a tranquil whisper, “it’s just theater. It’s all theater. It’s all theater. The whole thing is theater.’’
Non-theater —
"The interplay between Euclidean and Galois geometry" and
related remarks on interplay — Keats's Laws of Aesthetics.
Part theater, part non-theater —
Apollo's 13: A Group Theory Narrative —
I. At Wikipedia —

II. Here —
See Cube Spaces and Cubist Geometries.
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the 27-part (Galois) 3×3×3 cube–
A note from 1985 describing group actions on a 3×3 plane array—

Undated software by Ed Pegg Jr. displays
group actions on a 3×3×3 cube that extend the
3×3 group actions from 1985 described above—
Pegg gives no reference to the 1985 work on group actions.
"Shifting Amid, and Asserting, His Own Cinema"
— Headline of an essay on Bertolucci in tormorrow's Sunday New York Times
This, together with yesterday's post on the Paris "Symmetry, Duality, and Cinema" conference last June, suggests a review of the phrase "blue diamond" in this journal. The search shows a link to the French art film "Duelle."
Some background for the word and concept from a French dictionary —
|
duel duelle
|
For examples of duel and duelle see Evariste Galois
and Helen Mirren (the latter in The Tempest and in 2010 ).
Image from stoneship.org
The Dreidel Is Cast
The Nietzschean phrase "ruling and Caesarian spirits" occurred in yesterday morning's post "Novel Ending."
That post was followed yesterday morning by a post marking, instead, a beginning— that of Hanukkah 2010. That Jewish holiday, whose name means "dedication," commemorates the (re)dedication of the Temple in Jerusalem in 165 BC.
The holiday is celebrated with, among other things, the Jewish version of a die— the dreidel . Note the similarity of the dreidel to an illustration of The Stone* on the cover of the 2001 Eerdmans edition of Charles Williams's 1931 novel Many Dimensions—

For mathematics related to the dreidel , see Ivars Peterson's column on this date fourteen years ago.
For mathematics related (if only poetically) to The Stone , see "Solomon's Cube" in this journal.
Here is the opening of Many Dimensions—

For a fanciful linkage of the dreidel 's concept of chance to The Stone 's concept of invariant law, note that the New York Lottery yesterday evening (the beginning of Hanukkah) was 840. See also the number 840 in the final post (July 20, 2002) of the "Solomon's Cube" search.
Some further holiday meditations on a beginning—
Today, on the first full day of Hanukkah, we may or may not choose to mark another beginning— that of George Frederick James Temple, who was born in London on this date in 1901. Temple, a mathematician, was President of the London Mathematical Society in 1951-1953. From his MacTutor biography—
"In 1981 (at the age of 80) he published a book on the history of mathematics. This book 100 years of mathematics (1981) took him ten years to write and deals with, in his own words:-
those branches of mathematics in which I had been personally involved.
He declared that it was his last mathematics book, and entered the Benedictine Order as a monk. He was ordained in 1983 and entered Quarr Abbey on the Isle of Wight. However he could not stop doing mathematics and when he died he left a manuscript on the foundations of mathematics. He claims:-
The purpose of this investigation is to carry out the primary part of Hilbert's programme, i.e. to establish the consistency of set theory, abstract arithmetic and propositional logic and the method used is to construct a new and fundamental theory from which these theories can be deduced."
For a brief review of Temple's last work, see the note by Martin Hyland in "Fundamental Mathematical Theories," by George Temple, Philosophical Transactions of the Royal Society, A, Vol. 354, No. 1714 (Aug. 15, 1996), pp. 1941-1967.
The following remarks by Hyland are of more general interest—
"… one might crudely distinguish between philosophical and mathematical motivation. In the first case one tries to convince with a telling conceptual story; in the second one relies more on the elegance of some emergent mathematical structure. If there is a tradition in logic it favours the former, but I have a sneaking affection for the latter. Of course the distinction is not so clear cut. Elegant mathematics will of itself tell a tale, and one with the merit of simplicity. This may carry philosophical weight. But that cannot be guaranteed: in the end one cannot escape the need to form a judgement of significance."
— J. M. E. Hyland. "Proof Theory in the Abstract." (pdf)
Annals of Pure and Applied Logic 114, 2002, 43-78.
Here Hyland appears to be discussing semantic ("philosophical," or conceptual) and syntactic ("mathematical," or structural) approaches to proof theory. Some other remarks along these lines, from the late Gian-Carlo Rota—
See also "Galois Connections" at alpheccar.org and "The Galois Connection Between Syntax and Semantics" at logicmatters.net.
* Williams's novel says the letters of The Stone are those of the Tetragrammaton— i.e., Yod, He, Vau, He (cf. p. 26 of the 2001 Eerdmans edition). But the letters on the 2001 edition's cover Stone include the three-pronged letter Shin , also found on the dreidel . What esoteric religious meaning is implied by this, I do not know.
Continued from June 4, 2010

See also Jon Han's fanciful illustration in today's New York Times and "Galois Cube" in this journal.
Google News this afternoon—
Related material:
"Martin Gardner passed away on May 22, 2010."
Imaginary movie poster from stoneship.org
Context— The Gardner Tribute.
This journal on October 12 (the traditional Columbus day)—
"The text is a two-way mirror
that allows me to look into
the life and times of the reader."
– The French Mathematician
(Galois), by Tom Petsinis
It is not clear how this is supposed to work.
However, there is synchronicity and the New York Lottery—
October 12, 2010—
Midday 765, Evening 365 —
Life and Times.
Life
|
From Log24 on April 21, the date of Mark Twain’s death– Psychoshop, by Alfred Bester and Roger Zelazny:
The Pennsylvania Lottery
and hence Log24, 9/23 (2007), and page 765 of From Here to Eternity (Delta paperback, 1998):
|
Times
|
See "Seasons of Love" from the musical "Rent." |
See also Mark 15:38— "And the veil of the temple…"
"Always keep a diamond in your mind."
— Tom Waits/Kathleen Brennan song performed by Solomon Burke at the Paradiso in Amsterdam
|
"The text is a two-way mirror — The French Mathematician |
 |
From Telegraph.co.uk (published: 5:56 PM BST 10 Aug 2010), a note on British-born Canadian journalist Bruce Garvey, who died at 70 on August 1—
In 1970, while reporting on the Apollo 13 mission at Nasa Mission Control for the Toronto Star, he was one of only two journalists— alongside Richard Killian of the Daily Express— to hear the famous message: "Houston we've had a problem."
See also Log24 posts of 10 AM and noon today.
The latter post poses the problem "You're dead. Now what?"
Again, as in this morning's post, applying Jungian synchronicity—
A check of this journal on the date of Garvey's death yields a link to 4/28's "Eightfold Geometry."
That post deals with a piece of rather esoteric mathematical folklore. Those who prefer easier problems may follow the ongoing struggles of Julie Taymor with "Spider-Man: Turn Off the Dark."
The problems of death, geometry, and Taymor meet in "Spider Woman" (April 29) and "Memorial for Galois" (May 31).
Yesterday's architectural entertainment coincided, more or less, with the New York Times article "The Hand of a Master Architect" (Online Sunday, Aug. 8, and in the print edition Monday, Aug. 9).
A search for some background on that architect (Philip Johnson, not Howard Roark) showed that the Art Libraries Society of North America published a notable graphic logo in 2005—

See this journal on April 7, 2005, for a related graphic design.
The ARLIS/NA 2005 page cited above says about Houston, Texas, that
"Just beyond the museum district lies Rice University, the city's most prestigious and oldest college….
Other campuses that contain significant architecture include St. Thomas University where Philip Johnson has made his mark for a period that extends more than forty years."

University of St. Thomas, Chapel of St. Basil
Applying Jungian synchronicity, we note that Johnson designed the Chapel of St. Basil at the University of St. Thomas, that the traditional date of the Feast of St. Basil is June 14, and that this journal on that date contained the following, from the aforementioned Rice University—
… a properly formulated Principle of Sufficient Reason plays
a fundamental role in scientific thought and, furthermore, is
to be regarded as of the greatest suggestiveness from the
philosophic point of view.2
… metaphysical reasoning always relies on the Principle of
Sufficient Reason, and… the true meaning of this Principle
is to be found in the “Theory of Ambiguity” and in the associated
mathematical “Theory of Groups.”
If I were a Leibnizian mystic, believing in his “preestablished
harmony,” and the “best possible world” so satirized by Voltaire
in “Candide,” I would say that the metaphysical importance of
the Principle of Sufficient Reason and the cognate Theory of Groups
arises from the fact that God thinks multi-dimensionally3
whereas men can only think in linear syllogistic series, and the
Theory of Groups is the appropriate instrument of thought to
remedy our deficiency in this respect.
The founder of the Theory of Groups was the mathematician
Evariste Galois….
2 As far as I am aware, only Scholastic Philosophy has fully recognized
and exploited this principle as one of basic importance for philosophic thought.
3 That is, uses multi-dimensional symbols beyond our grasp.
For more about Scholastic Philosophy, see the Center for Thomistic Studies at the University of St. Thomas.
For more about the graphic symbol shown (as above) by ARLIS and by Log24 in April 2005, see in this journal "rature sous rature ."
For aficionados of mathematics and narrative —
Illustration from
"The Galois Quaternion— A Story"
This resembles an attempt by Coxeter in 1950 to represent
a Galois geometry in the Euclidean plane—
The quaternion illustration above shows a more natural way to picture this geometry—
not with dots representing points in the Euclidean plane, but rather with unit squares
representing points in a finite Galois affine plane. The use of unit squares to
represent points in Galois space allows, in at least some cases, the actions
of finite groups to be represented more naturally than in Euclidean space.
See Galois Geometry, Geometry Simplified, and
Finite Geometry of the Square and Cube.
Barnes & Noble has an informative new review today of the recent Galois book Duel at Dawn.
It begins…
"In 1820, the Hungarian noble Farkas Bolyai wrote an impassioned cautionary letter to his son Janos:
'I know this way to the very end. I have traversed this bottomless night, which extinguished all light and joy in my life… It can deprive you of your leisure, your health, your peace of mind, and your entire happiness… I turned back when I saw that no man can reach the bottom of this night. I turned back unconsoled, pitying myself and all mankind. Learn from my example…'
Bolyai wasn't warning his son off gambling, or poetry, or a poorly chosen love affair. He was trying to keep him away from non-Euclidean geometry."
For a less dark view (obtained by simply redefining "non-Euclidean" in a more logical way*) see Non-Euclidean Blocks and Finite Geometry and Physical Space.
* Finite geometry is not Euclidean geometry— and is, therefore, non-Euclidean
in the strictest sense (though not according to popular usage), simply because
Euclidean geometry has infinitely many points, and a finite geometry does not.
(This more logical definition of "non-Euclidean" seems to be shared by
at least one other person.)
And some finite geometries are non-Euclidean in the popular-usage sense,
related to Euclid's parallel postulate.
The seven-point Fano plane has, for instance, been called
"a non-Euclidean geometry" not because it is finite
(though that reason would suffice), but because it has no parallel lines.
(See the finite geometry page at the Centre for the Mathematics
of Symmetry and Computation at the University of Western Australia.)
"Human perception is a saga of created reality. But we were devising entities beyond the agreed-upon limits of recognition or interpretation…."
– Don DeLillo, Point Omega
Capitalized, the letter omega figures in the theology of two Jesuits, Teilhard de Chardin and Gerard Manley Hopkins. For the former, see a review of DeLillo. For the latter, see James Finn Cotter's Inscape and "Hopkins and Augustine."
The lower-case omega is found in the standard symbolic representation of the Galois field GF(4)—
A representation of GF(4) that goes beyond the standard representation—
Here the four diagonally-divided two-color squares represent the four elements of GF(4).
The graphic properties of these design elements are closely related to the algebraic properties of GF(4).
This is demonstrated by a decomposition theorem used in the proof of the diamond theorem.
To what extent these theorems are part of "a saga of created reality" may be debated.
I prefer the Platonist's "discovered, not created" side of the debate.
or, Darkness and Brightness at Noon
"Human perception is a saga of created reality. But we were devising entities beyond the agreed-upon limits of recognition or interpretation…. We tried to create new realities overnight, careful sets of words that resemble advertising slogans in memorability and repeatability. These were words that would yield pictures eventually and then become three-dimensional."
— Don DeLillo, Point Omega
GF(4) = {0, 1, ω, ω2}
— Symbolic representation of a Galois field
"One two three four,
who are we for?"
— Cheerleaders' chant
27
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the 27-part (Galois) 3×3×3 cube–
Geometry Simplified

(a projective space)
The above finite projective space
is the simplest nontrivial example
of a Galois geometry (i.e., a finite
geometry with coordinates in a
finite (that is, Galois) field.)
The vertical (Euclidean) line represents a
(Galois) point, as does the horizontal line
and also the vertical-and-horizontal
cross that represents the first two points'
binary sum (i.e., symmetric difference,
if the lines are regarded as sets).
Homogeneous coordinates for the
points of this line —
(1,0), (0,1), (1,1).
Here 0 and 1 stand for the elements
of the two-element Galois field GF(2).
The 3-point line is the projective space
corresponding to the affine space
(a plane, not a line) with four points —

(an affine space)
The (Galois) points of this affine plane are
not the single and combined (Euclidean)
line segments that play the role of
points in the 3-point projective line,
but rather the four subsquares
that the line segments separate.
For further details, see Galois Geometry.
There are, of course, also the trivial
two-point affine space and the corresponding
trivial one-point projective space —

Here again, the points of the affine space are
represented by squares, and the point of the
projective space is represented by a line segment
separating the affine-space squares.
"By groping toward the light we are made to realize
how deep the darkness is around us."
— Arthur Koestler, The Call Girls: A Tragi-Comedy,
Random House, 1973, page 118
A 1973 review of Koestler's book—
"Koestler's 'call girls,' summoned here and there
by this university and that foundation
to perform their expert tricks, are the butts
of some chilling satire."
Examples of Light—
Felix Christian Klein (1849- June 22, 1925) and Évariste Galois (1811-1832)
Klein on Galois—
"… in France just about 1830 a new star of undreamt-of brilliance— or rather a meteor, soon to be extinguished— lighted the sky of pure mathematics: Évariste Galois."
— Felix Klein, Development of Mathematics in the 19th Century, translated by Michael Ackerman. Brookline, Mass., Math Sci Press, 1979. Page 80.
"… um 1830 herum in Frankreich als ein neuer Stern von ungeahntem Glanze am Himmel der reinen Mathematik aufleuchtet, um freilich, einem Meteor gleich, sehr bald zu verlöschen: Évariste Galois."
— Felix Klein, Vorlesungen Über Die Entwicklung Der Mathematick Im 19. Jahrhundert. New York, Chelsea Publishing Co., 1967. (Vol. I, originally published in Berlin in 1926.) Page 88.
Examples of Darkness—
Martin Gardner on Galois—
"Galois was a thoroughly obnoxious nerd,
suffering from what today would be called
a 'personality disorder.' His anger was
paranoid and unremitting."
Gardner was reviewing a recent book about Galois by one Amir Alexander.
Alexander himself has written some reviews relevant to the Koestler book above.
See Alexander on—
The 2005 Mykonos conference on Mathematics and Narrative
A series of workshops at Banff International Research Station for Mathematical Innovation between 2003 and 2006. "The meetings brought together professional mathematicians (and other mathematical scientists) with authors, poets, artists, playwrights, and film-makers to work together on mathematically-inspired literary works."
Cubic models of finite geometries
display an interplay between
Euclidean and Galois geometry.
Example 1— The 2×2×2 Cube—
also known as the eightfold cube—

Group actions on the eightfold cube, 1984—
Version by Laszlo Lovasz et al., 2003—

Lovasz et al. go on to describe the same group actions
as in the 1984 note, without attribution.
Example 2— The 3×3×3 Cube
A note from 1985 describing group actions on a 3×3 plane array—

Undated software by Ed Pegg Jr. displays
group actions on a 3×3×3 cube that extend the
3×3 group actions from 1985 described above—
Pegg gives no reference to the 1985 work on group actions.
Example 3— The 4×4×4 Cube
A note from 27 years ago today—

As far as I know, this version of the
group-actions theorem has not yet been ripped off.
|
Limited— Good |
Unlimited— Bad
|
Jamie James in The Music of the Spheres—
"The Pythagorean philosophy, like Zoroastrianism, Taoism, and every early system of higher thought, is based upon the concept of dualism. Pythagoras constructed a table of opposites from which he was able to derive every concept needed for a philosophy of the phenomenal world. As reconstructed by Aristotle in his Metaphysics, the table contains ten dualities (ten being a particularly important number in the Pythagorean system, as we shall see):
| Limited Odd One Right Male Rest Straight Light Good Square |
Unlimited Even Many Left Female Motion Curved Dark Bad Oblong |
Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited (man, finite time, and so forth) and the unlimited (the cosmos, eternity, etc.) is not only the aim of Pythagoras's system but the central aim of all Western philosophy."
In the above view, four of the tesseract's 16
vertices are overlaid by other vertices.
For views that are more complete and
moveable, see Smith's tesseract page.
Four-Part Tesseract Divisions—

The above figure shows how four-part partitions
of the 16 vertices of a tesseract in an infinite
Euclidean space are related to four-part partitions
of the 16 points in a finite Galois space
|
Euclidean spaces versus Galois spaces in a larger context— Infinite versus Finite The central aim of Western religion —
"Each of us has something to offer the Creator...
the bridging of
masculine and feminine,
life and death.
It's redemption.... nothing else matters."
-- Martha Cooley in The Archivist (1998)
The central aim of Western philosophy —
Dualities of Pythagoras
as reconstructed by Aristotle:
Limited Unlimited
Odd Even
Male Female
Light Dark
Straight Curved
... and so on ....
"Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy." |
Another picture related to philosophy and religion—
Jung's Four-Diamond Figure from Aion—

This figure was devised by Jung
to represent the Self. Compare the
remarks of Paul Valéry on the Self—
|
Flight from Eden: The Origins of Modern Literary Criticism and Theory, by Steven Cassedy, U. of California Press, 1990, pages 156-157—
Valéry saw the mind as essentially a relational system whose operation he attempted to describe in the language of group mathematics. "Every act of understanding is based on a group," he says (C, 1:331). "My specialty— reducing everything to the study of a system closed on itself and finite" (C, 19: 645). The transformation model came into play, too. At each moment of mental life the mind is like a group, or relational system, but since mental life is continuous over time, one "group" undergoes a "transformation" and becomes a different group in the next moment. If the mind is constantly being transformed, how do we account for the continuity of the self? Simple; by invoking the notion of the invariant. And so we find passages like this one: "The S[elf] is invariant, origin, locus or field, it's a functional property of consciousness" (C, 15:170 [2:315]). Just as in transformational geometry, something remains fixed in all the projective transformations of the mind's momentary systems, and that something is the Self (le Moi, or just M, as Valéry notates it so that it will look like an algebraic variable). Transformation theory is all over the place. "Mathematical science… reduced to algebra, that is, to the analysis of the transformations of a purely differential being made up of homogeneous elements, is the most faithful document of the properties of grouping, disjunction, and variation in the mind" (O, 1:36). "Psychology is a theory of transformations, we just need to isolate the invariants and the groups" (C, 1:915). "Man is a system that transforms itself" (C, 2:896). O Paul Valéry, Oeuvres (Paris: Pléiade, 1957-60) C Valéry, Cahiers, 29 vols. (Paris: Centre National de le Recherche Scientifique, 1957-61) |
Note also the remarks of George David Birkhoff at Rice University
in 1940 (pdf) on Galois's theory of groups and the related
"theory of ambiguity" in Galois's testamentary letter—
|
… metaphysical reasoning always relies on the Principle of Sufficient Reason, and… the true meaning of this Principle is to be found in the “Theory of Ambiguity” and in the associated mathematical “Theory of Groups.” If I were a Leibnizian mystic, believing in his “preestablished harmony,” and the “best possible world” so satirized by Voltaire in “Candide,” I would say that the metaphysical importance of the Principle of Sufficient Reason and the cognate Theory of Groups arises from the fact that God thinks multi-dimensionally* whereas men can only think in linear syllogistic series, and the Theory of Groups is the appropriate instrument of thought to remedy our deficiency in this respect. * That is, uses multi-dimensional symbols beyond our grasp. |
Related material:
A medal designed by Leibniz to show how
binary arithmetic mirrors the creation by God
of something (1) from nothing (0).

Another array of 16 strings of 0's and 1's, this time
regarded as coordinates rather than binary numbers—


Some context by a British mathematician —

|
Imago by Wallace Stevens Who can pick up the weight of Britain, Who can move the German load Or say to the French here is France again? Imago. Imago. Imago. It is nothing, no great thing, nor man Of ten brilliancies of battered gold And fortunate stone. It moves its parade Of motions in the mind and heart, A gorgeous fortitude. Medium man In February hears the imagination's hymns And sees its images, its motions And multitudes of motions And feels the imagination's mercies, In a season more than sun and south wind, Something returning from a deeper quarter, A glacier running through delirium, Making this heavy rock a place, Which is not of our lives composed . . . Lightly and lightly, O my land, Move lightly through the air again. |
(Continued from April 23, 2009, and February 13, 2010.)
Paul Valéry as quoted in yesterday’s post:
“The S[elf] is invariant, origin, locus or field, it’s a functional property of consciousness” (Cahiers, 15:170 [2: 315])
The geometric example discussed here yesterday as a Self symbol may seem too small to be really impressive. Here is a larger example from the Chinese, rather than European, tradition. It may be regarded as a way of representing the Galois field GF(64). (“Field” is a rather ambiguous term; here it does not, of course, mean what it did in the Valéry quotation.)
From Geometry of the I Ching—

The above 64 hexagrams may also be regarded as
the finite affine space AG(6,2)— a larger version
of the finite affine space AG(4,2) in yesterday’s post.
That smaller space has a group of 322,560 symmetries.
The larger hexagram space has a group of
1,290,157,424,640 affine symmetries.
From a paper on GL(6,2), the symmetry group
of the corresponding projective space PG(5,2),*
which has 1/64 as many symmetries—
For some narrative in the European tradition
related to this geometry, see Solomon’s Cube.
* Update of July 29, 2011: The “PG(5,2)” above is a correction from an earlier error.
Théorie de l'Ambiguité
According to a 2008 paper by Yves André of the École Normale Supérieure of Paris—
"Ambiguity theory was the name which Galois used
when he referred to his own theory and its future developments."
The phrase "the theory of ambiguity" occurs in the testamentary letter Galois wrote to a friend, Auguste Chevalier, on the night before Galois was shot in a duel.
Hermann Weyl in Symmetry, Princeton University Press, 1952—
"This letter, if judged by the novelty and profundity of ideas it contains, is perhaps
the most substantial piece of writing in the whole literature of mankind."
Conclusion of the Galois testamentary letter, according to
the 1897 Paris edition of Galois's collected works—

The original—
A transcription—
Évariste GALOIS, Lettre-testament, adressée à Auguste Chevalier—
Tu sais mon cher Auguste, que ces sujets ne sont pas les seuls que j'aie
explorés. Mes principales méditations, depuis quelques temps,
étaient dirigées sur l'application à l'analyse transcendante de la théorie de
l'ambiguité. Il s'agissait de voir a priori, dans une relation entre des quantités
ou fonctions transcendantes, quels échanges on pouvait faire, quelles
quantités on pouvait substituer aux quantités données, sans que la relation
put cesser d'avoir lieu. Cela fait reconnaitre de suite l'impossibilité de beaucoup
d'expressions que l'on pourrait chercher. Mais je n'ai pas le temps, et mes idées
ne sont pas encore bien développées sur ce terrain, qui est
immense.
Tu feras imprimer cette lettre dans la Revue encyclopédique.
Je me suis souvent hasardé dans ma vie à avancer des propositions dont je n'étais
pas sûr. Mais tout ce que j'ai écrit là est depuis bientôt un an dans ma
tête, et il est trop de mon intérêt de ne pas me tromper pour qu'on
me soupconne d'avoir énoncé des théorèmes dont je n'aurais pas la démonstration
complète.
Tu prieras publiquement Jacobi et Gauss de donner leur avis,
non sur la vérité, mais sur l'importance des théorèmes.
Après cela, il y aura, j'espère, des gens qui trouveront leur profit
à déchiffrer tout ce gachis.
Je t'embrasse avec effusion.
E. Galois Le 29 Mai 1832
A translation by Dr. Louis Weisner, Hunter College of the City of New York, from A Source Book in Mathematics, by David Eugene Smith, Dover Publications, 1959–
You know, my dear Auguste, that these subjects are not the only ones I have explored. My reflections, for some time, have been directed principally to the application of the theory of ambiguity to transcendental analysis. It is desired see a priori in a relation among quantities or transcendental functions, what transformations one may make, what quantities one may substitute for the given quantities, without the relation ceasing to be valid. This enables us to recognize at once the impossibility of many expressions which we might seek. But I have no time, and my ideas are not developed in this field, which is immense.
Print this letter in the Revue Encyclopédique.
I have often in my life ventured to advance propositions of which I was uncertain; but all that I have written here has been in my head nearly a year, and it is too much to my interest not to deceive myself that I have been suspected of announcing theorems of which I had not the complete demonstration.
Ask Jacobi or Gauss publicly to give their opinion, not as to the truth, but as to the importance of the theorems.
Subsequently there will be, I hope, some people who will find it to their profit to decipher all this mess.
J t'embrasse avec effusion.
E. Galois. May 29, 1832.
Translation, in part, in The Unravelers: Mathematical Snapshots, by Jean Francois Dars, Annick Lesne, and Anne Papillaut (A.K. Peters, 2008)–
"You know, dear Auguste, that these subjects are not the only ones I have explored. For some time my main meditations have been directed on the application to transcendental analysis of the theory of ambiguity. The aim was to see in a relation between quantities or transcendental functions, what exchanges we could make, what quantities could be substituted to the given quantities without the relation ceasing to take place. In that way we see immediately that many expressions that we might look for are impossible. But I don't have the time and my ideas are not yet developed enough in this vast field."
Another translation, by James Dolan at the n-Category Café—
"My principal meditations for some time have been directed towards the application of the theory of ambiguity to transcendental analysis. It was a question of seeing a priori in a relation between quantities or transcendent functions, what exchanges one could make, which quantities one could substitute for the given quantities without the original relation ceasing to hold. That immediately made clear the impossibility of finding many expressions that one could look for. But I do not have time and my ideas are not yet well developed on this ground which is immense."
Related material—
"Renormalisation et Ambiguité Galoisienne," by Alain Connes, 2004
"La Théorie de l’Ambiguïté : De Galois aux Systèmes Dynamiques," by Jean-Pierre Ramis, 2006
"Ambiguity Theory, Old and New," preprint by Yves André, May 16, 2008,
"Ambiguity Theory," post by David Corfield at the n-Category Café, May 19, 2008
"Measuring Ambiguity," inaugural lecture at Utrecht University by Gunther Cornelissen, Jan. 16, 2009
Continued from May 8
(Feast of Saint Robert Heinlein)
“Wells and trees were dedicated to saints. But the offerings at many wells and trees were to something other than the saint; had it not been so they would not have been, as we find they often were, forbidden. Within this double and intertwined life existed those other capacities, of which we know more now, but of which we still know little– clairvoyance, clairaudience, foresight, telepathy.”
— Charles Williams, Witchcraft, Faber and Faber, London, 1941
Why "Saint" Robert? See his accurate depiction of evil– the Eater of Souls in Glory Road.
For more on Williams's "other capacities," see Heinlein's story "Lost Legacy."
A related story– Fritz Leiber's "The Mind Spider." An excerpt:
The conference—it was much more a hyper-intimate
gabfest—proceeded.
"My static box bugged out for a few ticks this morning,"
Evelyn remarked in the course of talking over the
trivia of the past twenty-four hours.
The static boxes were an invention of Grandfather
Horn. They generated a tiny cloud of meaningless brain
waves. Without such individual thought-screens, there was
too much danger of complete loss of individual personality
—once Grandfather Horn had "become" his infant daughter
as well as himself for several hours and the unfledged
mind had come close to being permanently lost in its own
subconscious. The static boxes provided a mental wall be-
– hind which a mind could safely grow and function, similar
to the wall by which ordinary minds are apparently
always enclosed.
In spite of the boxes, the Horns shared thoughts and
emotions to an amazing degree. Their mental togetherness
was as real and as mysterious—and as incredible—as
thought itself . . . and thought is the original angel-cloud
dancing on the head of a pin. Their present conference
was as warm and intimate and tart as any actual family
gathering in one actual room around one actual table.
Five minds, joined together in the vast mental darkness
that shrouds all minds. Five minds hugged together for
comfort and safety in the infinite mental loneliness that
pervades the cosmos.
Evelyn continued, "Your boxes were all working, of
course, so I couldn't get your thoughts—just the blurs of
your boxes like little old dark grey stars. But this time
if gave me a funny uncomfortable feeling, like a spider
Crawling down my—Grayl! Don't feel so wildly! What
Is it?”
Then… just as Grayl started to think her answer…
something crept from the vast mental darkness and infinite
cosmic loneliness surrounding the five minds of the
Horns.
Grayl was the first to notice. Her panicky thought had
ttie curling too-keen edge of hysteria. "There are six of
us now! There should only be five, but there are six.
Count! Count, I tell you! Six!"
To Mort it seemed that a gigantic spider was racing
across the web of their thoughts….
See also this journal on May 30– "720 in the Book"– and on May 31– "Memorial for Galois."
("Obnoxious nerds"— a phrase Martin Gardner recently applied to Galois— will note that 720
"I wonder if there's just been a critical mass
of creepy stories about Harvard
in the last couple of years…
A kind of piling on of
nastiness and creepiness…"
— Margaret Soltan, October 23, 2006
Harvard University Press
on Facebook—
 |
|
| http://ping.fm/YrgOh | |
The book that the late Gardner was reviewing
was published in April by Harvard University Press.
If Gardner's remark were true,
Galois would fit right in at Harvard. Example—
The Harvard math department's pie-eating contest—

Wikipedia—
"On June 2, Évariste Galois was buried in a common grave of the Montparnasse cemetery whose exact location is unknown."
Évariste Galois, Lettre de Galois à M. Auguste Chevalier—
Après cela, il y aura, j'espère, des gens qui trouveront leur profit à déchiffrer tout ce gâchis.
(Later there will be, I hope, some people who will find it to their advantage to decipher all this mess.)
Martin Gardner on the above letter—
"Galois had written several articles on group theory, and was merely annotating and correcting those earlier published papers."
— The Last Recreations, by Martin Gardner, published by Springer in 2007, page 156.
“It is a melancholy pleasure that what may be [Martin] Gardner’s last published piece, a review of Amir Alexander’s Duel at Dawn: Heroes, Martyrs & the Rise of Modern Mathematics, will appear next week in our June issue.”
– Roger Kimball of The New Criterion, May 23, 2010.
The Gardner piece is now online. It contains…
|
Gardner’s tribute to Galois— “Galois was a thoroughly obnoxious nerd,
suffering from what today would be called a ‘personality disorder.’ His anger was paranoid and unremitting.” |
Today is commencement day at Princeton.
Sunday's A Post for Galois was suggested, in part, by the fact that the founder and CEO of Amazon.com was that day's Princeton baccalaureate speaker. The Galois post linked to the Amazon reviews of one Christopher G. Robinson, a resident of Cambridge, Mass., whose Amazon book list titled "Step Right Up!" reflects a continuing libertine tradition at Harvard.
For Princeton's commencement day, it seems fitting to cite another Amazon document that reflects the more conservative values of that university.
I recommend the review Postmodern Pythagoras, by Matthew Milliner. Milliner is, in his own words, "an art history Ph.D. candidate at Princeton University."
See also Milliner's other reviews at Amazon.com.
"For every kind of libertine,
there is a kind of cross."
— Saying adapted from Pynchon
Paul Robeson in
"King Solomon's Mines," 1937—
The image above is an illustration from
"Romancing the Hyperspace," May 4, 2010.
This illustration, along with Georgia Brown's
song from "Cabin in the Sky"—
"There's honey in the honeycomb"—
suggests the following picture.
"What might have been and what has been
Point to one end, which is always present."
— Four Quartets
Romancing the
Non-Euclidean Hyperspace
Backstory —
Mere Geometry, Types of Ambiguity,
Dream Time, and Diamond Theory, 1937

For the 1937 grid, see Diamond Theory, 1937.
The grid is, as Mere Geometry points out, a non-Euclidean hyperspace.
For the diamonds of 2010, see Galois Geometry and Solomon’s Cube.
From Ursula K. Le Guin’s novel
The Dispossessed: An Ambiguous Utopia (1974)—
Chapter One
“There was a wall. It did not look important. It was built of uncut rocks roughly mortared. An adult could look right over it, and even a child could climb it. Where it crossed the roadway, instead of having a gate it degenerated into mere geometry, a line, an idea of boundary. But the idea was real. It was important. For seven generations there had been nothing in the world more important than that wall.
Like all walls it was ambiguous, two-faced. What was inside it and what was outside it depended upon which side of it you were on.”
Note—
“We note that the phrase ‘instead of having a gate it degenerated into mere geometry’ is mere fatuousness. If there is an idea here, degenerate, mere, and geometry in concert do not fix it. They bat at it like a kitten at a piece of loose thread.”
— Samuel R. Delany, The Jewel-Hinged Jaw: Notes on the Language of Science Fiction (Dragon Press, 1977), page 110 of revised edition, Wesleyan University Press, 2009
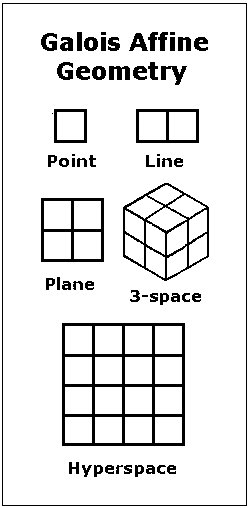
(For the phrase mere geometry elsewhere, see a note of April 22. The apparently flat figures in that note’s illustration “Galois Affine Geometry” may be regarded as degenerate views of cubes.)
Later in the Le Guin novel—
“… The Terrans had been intellectual imperialists, jealous wall builders. Even Ainsetain, the originator of the theory, had felt compelled to give warning that his physics embraced no mode but the physical and should not be taken as implying the metaphysical, the philosophical, or the ethical. Which, of course, was superficially true; and yet he had used number, the bridge between the rational and the perceived, between psyche and matter, ‘Number the Indisputable,’ as the ancient founders of the Noble Science had called it. To employ mathematics in this sense was to employ the mode that preceded and led to all other modes. Ainsetain had known that; with endearing caution he had admitted that he believed his physics did, indeed, describe reality.
Strangeness and familiarity: in every movement of the Terran’s thought Shevek caught this combination, was constantly intrigued. And sympathetic: for Ainsetain, too, had been after a unifying field theory. Having explained the force of gravity as a function of the geometry of spacetime, he had sought to extend the synthesis to include electromagnetic forces. He had not succeeded. Even during his lifetime, and for many decades after his death, the physicists of his own world had turned away from his effort and its failure, pursuing the magnificent incoherences of quantum theory with its high technological yields, at last concentrating on the technological mode so exclusively as to arrive at a dead end, a catastrophic failure of imagination. Yet their original intuition had been sound: at the point where they had been, progress had lain in the indeterminacy which old Ainsetain had refused to accept. And his refusal had been equally correct– in the long run. Only he had lacked the tools to prove it– the Saeba variables and the theories of infinite velocity and complex cause. His unified field existed, in Cetian physics, but it existed on terms which he might not have been willing to accept; for the velocity of light as a limiting factor had been essential to his great theories. Both his Theories of Relativity were as beautiful, as valid, and as useful as ever after these centuries, and yet both depended upon a hypothesis that could not be proved true and that could be and had been proved, in certain circumstances, false.
But was not a theory of which all the elements were provably true a simple tautology? In the region of the unprovable, or even the disprovable, lay the only chance for breaking out of the circle and going ahead.
In which case, did the unprovability of the hypothesis of real coexistence– the problem which Shevek had been pounding his head against desperately for these last three days. and indeed these last ten years– really matter?
He had been groping and grabbing after certainty, as if it were something he could possess. He had been demanding a security, a guarantee, which is not granted, and which, if granted, would become a prison. By simply assuming the validity of real coexistence he was left free to use the lovely geometries of relativity; and then it would be possible to go ahead. The next step was perfectly clear. The coexistence of succession could be handled by a Saeban transformation series; thus approached, successivity and presence offered no antithesis at all. The fundamental unity of the Sequency and Simultaneity points of view became plain; the concept of interval served to connect the static and the dynamic aspect of the universe. How could he have stared at reality for ten years and not seen it? There would be no trouble at all in going on. Indeed he had already gone on. He was there. He saw all that was to come in this first, seemingly casual glimpse of the method, given him by his understanding of a failure in the distant past. The wall was down. The vision was both clear and whole. What he saw was simple, simpler than anything else. It was simplicity: and contained in it all complexity, all promise. It was revelation. It was the way clear, the way home, the light.”
Related material—
Time Fold, Halloween 2005, and May and Zan.
See also The Devil and Wallace Stevens—
“In a letter to Harriet Monroe, written December 23, 1926, Stevens refers to the Sapphic fragment that invokes the genius of evening: ‘Evening star that bringest back all that lightsome Dawn hath scattered afar, thou bringest the sheep, thou bringest the goat, thou bringest the child home to the mother.’ Christmas, writes Stevens, ‘is like Sappho’s evening: it brings us all home to the fold’ (Letters of Wallace Stevens, 248).”
— “The Archangel of Evening,” Chapter 5 of Wallace Stevens: The Intensest Rendezvous, by Barbara M. Fisher, The University Press of Virginia, 1990

Stanford Encyclopedia of Philosophy —
“Mereology (from the Greek μερος, ‘part’) is the theory of parthood relations: of the relations of part to whole and the relations of part to part within a whole. Its roots can be traced back to the early days of philosophy, beginning with the Presocratics….”
A non-Euclidean* approach to parts–

Corresponding non-Euclidean*
projective points —

Richard J. Trudeau in The Non-Euclidean Revolution, chapter on “Geometry and the Diamond Theory of Truth”–
“… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions:
(1) Diamonds– informative, certain truths about the world– exist.
(2) The theorems of Euclidean geometry are diamonds.
Presumption (1) is what I referred to earlier as the ‘Diamond Theory’ of truth. It is far, far older than deductive geometry.”
Trudeau’s book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called “Diamond Theory.”
Although non-Euclidean,* the theorems of the 1976 “Diamond Theory” are also, in Trudeau’s terminology, diamonds.
* “Non-Euclidean” here means merely “other than Euclidean.” No violation of Euclid’s parallel postulate is implied.
"Democrats– in conclusion– Democrats in America
were put on earth to do one thing– Drag the
ignorant hillbilly half of this country into the next
century, which in their case is the 19th."
Reply to Maher:
"Hell is other people."
— Jean-Paul Sartre
|
Related material: Dragging Maher into the 18th century– From
Related material– Lemniscate to Langlands (2004) |
From yesterday's Seattle Times—
According to police, employees of a Second Avenue mission said the suspect, clad in black and covered in duct tape, had come into the mission "and threatened to blow the place up." He then told staffers "that he was a vampire and wanted to eat people."
The man… also called himself "a space cowboy"….
This suggests two film titles…

and Apollo's 13—
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
"The cube has…13 axes of symmetry:
6 C2 (axes joining midpoints of opposite edges),
4 C3 (space diagonals), and
3C4 (axes joining opposite face centroids)."
–Wolfram MathWorld article on the cube
These 13 symmetry axes can be used to illustrate the interplay between Euclidean and Galois geometry in a cubic model of the 13-point Galois plane.
The geometer's 3×3×3 cube–
27 separate subcubes unconnected
by any Rubik-like mechanism–

The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
A closely related structure–
the finite projective plane
with 13 points and 13 lines–
A later version of the 13-point plane
by Ed Pegg Jr.–
A group action on the 3×3×3 cube
as illustrated by a Wolfram program
by Ed Pegg Jr. (undated, but closely
related to a March 26, 1985 note
by Steven H. Cullinane)–
The above images tell a story of sorts.
The moral of the story–
Galois projective geometries can be viewed
in the context of the larger affine geometries
from which they are derived.
The standard definition of points in a Galois projective plane is that they are lines through the (arbitrarily chosen) origin in a corresponding affine 3-space converted to a vector 3-space.
If we choose the origin as the center cube in coordinatizing the 3×3×3 cube (See Weyl's relativity problem ), then the cube's 13 axes of symmetry can, if the other 26 cubes have properly (Weyl's "objectively") chosen coordinates, illustrate nicely the 13 projective points derived from the 27 affine points in the cube model.
The 13 lines of the resulting Galois projective plane may be derived from Euclidean planes through the cube's center point that are perpendicular to the cube's 13 Euclidean symmetry axes.
The above standard definition of points in a Galois projective plane may of course also be used in a simpler structure– the eightfold cube.
(The eightfold cube also allows a less standard way to picture projective points that is related to the symmetries of "diamond" patterns formed by group actions on graphic designs.)
See also Ed Pegg Jr. on finite geometry on May 30, 2006
at the Mathematical Association of America.
“Logic is all about the entertaining of possibilities.”
– Colin McGinn, Mindsight: Image, Dream, Meaning,
Harvard University Press, 2004
Geometry of Language,
continued from St. George's Day, 2009—

Related material:
Prima Materia,
The Galois Quaternion,
and The Wake of Imagination.
See also the following from a physicist
(not of the most orthodox sort, but his remarks
here on Heisenberg seem quite respectable)–
Argument for the Existence of Rebecca
Adapted from YouTube's "Mathematics and Religion," starring Rebecca Newberger Goldstein, author of the recent novel 36 Arguments for the Existence of God—

The added Quaternion picture is from
Groundhog Day, 2009.
"But wait, there's more!"
– Stanley Fish, NY Times Jan. 28
From the editors at The New York Times who, left to their own devices, would produce yet another generation of leftist morons who don't know the difference between education and entertainment–
A new Times column starts today–

The quality of the column's logo speaks for itself. It pictures a cone with dashed lines indicating height and base radius, but unlabeled except for a large italic x to the right of the cone. This enigmatic variable may indicate the cone's height or slant height– or, possibly, its surface area or volume.
Instead of the column's opening load of crap about numbers and Sesame Street, a discussion of its logo might be helpful.
The cone plays a major role in the historical development of mathematics.
Some background from an online edition of Euclid—
"Euclid proved in proposition XII.10 that the cone with the same base and height as a cylinder was one third of the cylinder, but he could not find the ratio of a sphere to the circumscribed cylinder. In the century after Euclid, Archimedes solved this problem as well as the much more difficult problem of the surface area of a sphere."
For Archimedes and the surface area of a sphere, see (for instance) a discussion by Kevin Brown. For more material on Archimedes, see "Archimedes: Volume of a Sphere," by Doug Faires (2001)– Archimedes' heuristic argument from mechanics that involves the volume of a cone– and Archimedes' more rigorous approach in The Works of Archimedes, edited by T. L. Heath (1897).
The work of Euclid and Archimedes on volumes was, of course, long before the discovery of calculus. For a helpful discussion of cone volumes involving high-school-level calculus, see, for instance, the following–

The Times editors apparently feel that
few of their readers are capable of
such high-school-level sophistication.
For some other geometric illustrations
perhaps more appealing than the Times's

dunce cap, see the symbol of
today's saint– a Bridget Cross—
and a web page on
visualized quaternions.
More Than Matter
| Wheel in Webster’s Revised Unabridged Dictionary, 1913
(f) Poetry The burden or refrain of a song. ⇒ “This meaning has a low degree of authority, but is supposed from the context in the few cases where the word is found.” Nares. You must sing a-down a-down, An you call him a-down-a. O, how the wheel becomes it! Shak. |
“In one or other of G. F. H. Shadbold’s two published notebooks, Beyond Narcissus and Reticences of Thersites, a short entry appears as to the likelihood of Ophelia’s enigmatic cry: ‘Oh, how the wheel becomes it!’ referring to the chorus or burden ‘a-down, a-down’ in the ballad quoted by her a moment before, the aptness she sees in the refrain.”
— First words of Anthony Powell’s novel “O, How the Wheel Becomes It!” (See Library Thing.)

Related material:
Photo uploaded on January 14, 2009
with caption “This nothing’s more than matter”
and the following nothings from this journal
on the same date– Jan. 14, 2009—
"The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole."
— Victor Turner, The Forest of Symbols, Ithaca, NY, Cornell University Press, 1967, p. 51, quoted by Beth Barrie in "Victor Turner."
To everything, turn, turn, turn…
— Peter Seeger
The Galois Quaternion
|
I had foreseen it all in precise detail. i = an imaginary being Here, on this complex space, |
Related material:
The Galois Quaternion
Click for context.
(See also Nativity and the end
of this morning's post.)
Recent Wikipedia activity in the area of finite geometry–
A list, complete up to now, of all Wikipedia changes made by anonymous user Marconet:
Note that all these items are related to changes in links that lead to my own web pages– with one exception, rather technical pages on finite geometry.
A list, complete up to now, of all Wikipedia changes made by anonymous user Greenfernglade:
Again, all these items are related to changes (in this case, deletions) in links that lead to my own web pages. Greenfernglade may or may not be the same person as Marconet. Neither one has a user home page at Wikipedia, but use of the pseudonyms has apparently served to cover up the IP address(es?) of the changes’ originator(s?).
For similar changes in the past, see my “user talk” page at Wikipedia. As I noted there on May 31, 2007, “There seems little point in protesting the deletions while Wikipedia still allows any anonymous user to change their articles.”

|
Non-Euclidean
Blocks
Passages from a classic story:
… he took from his pocket a gadget he had found in the box, and began to unfold it. The result resembled a tesseract, strung with beads….  Tesseract
"Your mind has been conditioned to Euclid," Holloway said. "So this– thing– bores us, and seems pointless. But a child knows nothing of Euclid. A different sort of geometry from ours wouldn't impress him as being illogical. He believes what he sees."
"Are you trying to tell me that this gadget's got a fourth dimensional extension?" Paradine demanded. "Hardening of the thought-arteries," Jane interjected. Paradine was not convinced. "Then a baby could work calculus better than Einstein? No, I don't mean that. I can see your point, more or less clearly. Only–" "Well, look. Let's suppose there are two kinds of geometry– we'll limit it, for the sake of the example. Our kind, Euclidean, and another, which we'll call x. X hasn't much relationship to Euclid. It's based on different theorems. Two and two needn't equal four in it; they could equal y, or they might not even equal. A baby's mind is not yet conditioned, except by certain questionable factors of heredity and environment. Start the infant on Euclid–" "Poor kid," Jane said. Holloway shot her a quick glance. "The basis of Euclid. Alphabet blocks. Math, geometry, algebra– they come much later. We're familiar with that development. On the other hand, start the baby with the basic principles of our x logic–" "Blocks? What kind?" Holloway looked at the abacus. "It wouldn't make much sense to us. But we've been conditioned to Euclid." — "Mimsy Were the Borogoves," Lewis Padgett, 1943 |
For the intuitive basis of one type of non-Euclidean* geometry– finite geometry over the two-element Galois field– see the work of…
Friedrich Froebel
(1782-1852), who
invented kindergarten.
His "third gift" —

Prima Materia
(Background: Art Humor: Sein Feld (March 11, 2009) and Ides of March Sermon, 2009)
From Cardinal Manning's review of Kirkman's Philosophy Without Assumptions—
"And here I must confess… that between something and nothing I can find no intermediate except potentia, which does not mean force but possibility."
— Contemporary Review, Vol. 28 (June-November, 1876), page 1017
Furthermore….
Cardinal Manning, Contemporary Review, Vol. 28, pages 1026-1027:
The following will be, I believe, a correct statement of the Scholastic teaching:–
1. By strict process of reason we demonstrate a First Existence, a First Cause, a First Mover; and that this Existence, Cause, and Mover is Intelligence and Power.
2. This Power is eternal, and from all eternity has been in its fullest amplitude; nothing in it is latent, dormant, or in germ: but its whole existence is in actu, that is, in actual perfection, and in complete expansion or actuality. In other words God is Actus Purus, in whose being nothing is potential, in potentia, but in Him all things potentially exist.
3. In the power of God, therefore, exists the original matter (prima materia) of all things; but that prima materia is pura potentia, a nihilo distincta, a mere potentiality or possibility; nevertheless, it is not a nothing, but a possible existence. When it is said that the prima materia of all things exists in the power of God, it does not mean that it is of the existence of God, which would involve Pantheism, but that its actual existence is possible.
4. Of things possible by the power of God, some come into actual existence, and their existence is determined by the impression of a form upon this materia prima. The form is the first act which determines the existence and the species of each, and this act is wrought by the will and power of God. By this union of form with the materia prima, the materia secunda or the materia signata is constituted.
5. This form is called forma substantialis because it determines the being of each existence, and is the root of all its properties and the cause of all its operations.
6. And yet the materia prima has no actual existence before the form is impressed. They come into existence simultaneously;
[p. 1027 begins]
as the voice and articulation, to use St. Augustine's illustration, are simultaneous in speech.
7. In all existing things there are, therefore, two principles; the one active, which is the form– the other passive, which is the matter; but when united, they have a unity which determines the existence of the species. The form is that by which each is what it is.
8. It is the form that gives to each its unity of cohesion, its law, and its specific nature.*
When, therefore, we are asked whether matter exists or no, we answer, It is as certain that matter exists as that form exists; but all the phenomena which fall under sense prove the existence of the unity, cohesion, species, that is, of the form of each, and this is a proof that what was once in mere possibility is now in actual existence. It is, and that is both form and matter.
When we are further asked what is matter, we answer readily, It is not God, nor the substance of God; nor the presence of God arrayed in phenomena; nor the uncreated will of God veiled in a world of illusions, deluding us with shadows into the belief of substance: much less is it catter [pejorative term in the book under review], and still less is it nothing. It is a reality, the physical kind or nature of which is as unknown in its quiddity or quality as its existence is certainly known to the reason of man.
* "… its specific nature"
(Click to enlarge) —
For a more modern treatment of these topics, see Werner Heisenberg's Physics and Philosophy. For instance:
"The probability wave of Bohr, Kramers, Slater, however, meant… a tendency for something. It was a quantitative version of the old concept of 'potentia' in Aristotelian philosophy. It introduced something standing in the middle between the idea of an event and the actual event, a strange kind of physical reality just in the middle between possibility and reality."
Compare to Cardinal Manning's statement above:
"… between something and nothing I can find no intermediate except potentia…"
To the mathematician, the cardinal's statement suggests the set of real numbers between 1 and 0, inclusive, by which probabilities are measured. Mappings of purely physical events to this set of numbers are perhaps better described by applied mathematicians and physicists than by philosophers, theologians, or storytellers. (Cf. Voltaire's mockery of possible-worlds philosophy and, more recently, The Onion's mockery of the fictional storyteller Fournier's quantum flux. See also Mathematics and Narrative.)
Regarding events that are not purely physical– those that have meaning for mankind, and perhaps for God– events affecting conception, birth, life, and death– the remarks of applied mathematicians and physicists are often ignorant and obnoxious, and very often do more harm than good. For such meaningful events, the philosophers, theologians, and storytellers are better guides. See, for instance, the works of Jung and those of his school. Meaningful events sometimes (perhaps, to God, always) exhibit striking correspondences. For the study of such correspondences, the compact topological space [0, 1] discussed above is perhaps less helpful than the finite Galois field GF(64)– in its guise as the I Ching. Those who insist on dragging God into the picture may consult St. Augustine's Day, 2006, and Hitler's Still Point.
(Title of a New Yorker
essay dated June 2, 2008)
In his honor, we may perhaps be justified in temporarily ignoring the wise saying "never assume."
From a defense of the dogma of the Assumption:
"On another level, the Assumption epitomizes the reconciliation of the material and spiritual world, as the human Mary enters 'body and soul to heavenly glory.' Carl Jung, the transpersonal psychologist, concluded that the doctrine of the Assumption reflected an acceptance of the physical world."
For other such reconciliations, see
But all these in thir pregnant causes mixt
Confus'dly, and which thus must ever fight,
Unless th' Almighty Maker them ordain
His dark materials to create more Worlds….
The New York Times
on June 17, 2007:
Design Meets Dance,
and Rules Are Broken
Yesterday's evening entry was
on the fictional sins of a fictional
mathematician and also (via a link
to St. Augustine's Day, 2006), on
the geometry of the I Ching* —
The eternal
combined with
the temporal:

The fictional mathematician's
name, noted here (with the Augustine-
I Ching link as a gloss) in yesterday's
evening entry, was Summerfield.
From the above Times article–
"Summerspace," a work by
choreographer Merce Cunningham
and artist Robert Rauschenberg
that offers a competing
vision of summer:


From left, composer John Cage,
choreographer Merce Cunningham,
and artist Robert Rauschenberg
in the 1960's
"When shall we three meet again?"

Note that the alchemical structure
at left, suited more to narrative
than to mathematics, nevertheless
is mirrored within the pure
mathematics at right.
Related material
on Galois and geometry:
| Geometries of the group PSL(2, 11) by Francis Buekenhout, Philippe Cara, and Koen Vanmeerbeek. Geom. Dedicata, 83 (1-3): 169–206, 2000–
|
“My pursuits are a joke
in that the universe is a joke.
One has to reflect
the universe faithfully.”
— John Frederick Michell
Feb. 9, 1933 –
April 24, 2009
— Robert A. Heinlein,
The Number of the Beast
For Marisa Tomei
(born Dec. 4, 1964) —
on the day that
Bob Seger turns 64 —
A Joke:
Points All Her Own
Points All Her Own,
Part I:
(For the backstory, see
the Log24 entries and links
on Marisa Tomei’s birthday
last year.)

Points All Her Own,
Part II:
(For the backstory, see
Galois Geometry:
The Simplest Examples.)

Points All Her Own,
Part III:
(For the backstory, see
Geometry of the I Ching
and the history of
Chinese philosophy.)
In simpler terms:


|
Hotel Puzzle by John Tierney "Russell Crowe arrives at the Hotel Infinity looking tired and ornery. He demands a room. The clerk informs him that there are no vacancies…."
|
Footprints from California today
(all by a person or persons using Firefox browsers):
7:10 AM
http://m759.xanga.com/679142359/concepts-of-space/?
Concepts of Space: Euclid vs. Galois
8:51 AM
http://m759.xanga.com/689601851/art-wars-continued/?
Art Wars continued: Behind the Picture
1:33 PM
http://m759.xanga.com/678995132/a-riff-for-dave/?
A Riff for Dave: Me and My Shadow
2:11 PM
http://m759.xanga.com/638308002/a-death-of-kings/?
A Death of Kings: In Memory of Bobby Fischer
2:48 PM
http://m759.xanga.com/691644175/art-wars-in-review–/?
Art Wars in review– Through the Looking Glass: A Sort of Eternity
3:28 PM and
http://m759.xanga.com/684680406/annals-of-philosophy/?
Annals of Philosophy: The Dormouse of Perception
4:28 PM
http://m759.xanga.com/641536988/epiphany-for-roy-part-i/?
Epiphany for Roy, Part I
6:03 PM
http://m759.xanga.com/641949564/art-wars-continued/?
At the Still Point: All That Jazz
6:22 PM
http://m759.xanga.com/644330798/where-entertainment-is-not-god/?
Where Entertainment is Not God: The Just Word
7:14 PM
http://m759.xanga.com/643490468/happy-new-yorker-day/?
Happy New Yorker Day– Class Galore
7:16 PM
http://m759.xanga.com/643812753/the-politics-of-change/?
The Politics of Change: Jumpers
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."

|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)

over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Cara:

Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Through the
Looking Glass:
A Sort of Eternity
From the new president’s inaugural address:
“… in the words of Scripture, the time has come to set aside childish things.”
The words of Scripture:
“through a glass”—
[di’ esoptrou].
By means of
a mirror [esoptron].
Childish things:

Not-so-childish:
Three planes through
the center of a cube
that split it into
eight subcubes:

Through a glass, darkly:
A group of 8 transformations is
generated by affine reflections
in the above three planes.
Shown below is a pattern on
the faces of the 2x2x2 cube
that is symmetric under one of
these 8 transformations–
a 180-degree rotation:
(Click on image
for further details.)
But then face to face:
A larger group of 1344,
rather than 8, transformations
of the 2x2x2 cube
is generated by a different
sort of affine reflections– not
in the infinite Euclidean 3-space
over the field of real numbers,
but rather in the finite Galois
3-space over the 2-element field.

Galois age fifteen,
drawn by a classmate.
These transformations
in the Galois space with
finitely many points
produce a set of 168 patterns
like the one above.
For each such pattern,
at least one nontrivial
transformation in the group of 8
described above is a symmetry
in the Euclidean space with
infinitely many points.
For some generalizations,
see Galois Geometry.
Related material:
The central aim of Western religion–
"Each of us has something to offer the Creator... the bridging of masculine and feminine, life and death. It's redemption.... nothing else matters." -- Martha Cooley in The Archivist (1998) The central aim of Western philosophy– Dualities of Pythagoras as reconstructed by Aristotle: Limited Unlimited Odd Even Male Female Light Dark Straight Curved ... and so on .... “Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy.” — Jamie James in The Music of the Spheres (1993) “In the garden of Adding — The Midrash Jazz Quartet in City of God, by E. L. Doctorow (2000) A quotation today at art critic Carol Kino’s website, slightly expanded: “Art inherited from the old religion — Octavio Paz,”Seeing and Using: Art and Craftsmanship,” in Convergences: Essays on Art and Literature (New York: Harcourt Brace Jovanovich 1987), 52 From Brian O’Doherty’s 1976 Artforum essays– not on museums, but rather on gallery space: “We have now reached
“Space: what you — James Joyce, Ulysses |
Recent abstracts of interest:
Kuwait Foundation Lectures —
Jan. 29, 2008: J. P. Wintenberger, “On the Proof of Serre’s Conjecture“
Oct. 28, 2008: Chandrashekhar Khare, “Modular Forms and Galois Representations“
Background:
The Last Theorem, a novel by Arthur C. Clarke and Frederik Pohl published Aug. 5, 2008
“Going Beyond Fermat’s Last Theorem,” a news article in The Hindu published April 25, 2005
Wikipedia: Serre Conjecture (Number Theory)
Henri Darmon, “Serre’s Conjectures“
On May 4, 2005, I wrote a note about how to visualize the 7-point Fano plane within a cube.
Last month, John Baez showed slides that touched on the same topic. This note is to clear up possible confusion between our two approaches.
From Baez’s Rankin Lectures at the University of Glasgow:

The statement is, however, true of the eightfold cube, whose eight subcubes correspond to points of the linear 3-space over the two-element field, if “planes through the origin” is interpreted as planes within that linear 3-space, as in Galois geometry, rather than within the Euclidean cube that Baez’s slides seem to picture.
This Galois-geometry interpretation is, as an article of his from 2001 shows, actually what Baez was driving at. His remarks, however, both in 2001 and 2008, on the plane-cube relationship are both somewhat trivial– since “planes through the origin” is a standard definition of lines in projective geometry– and also unrelated– apart from the possibility of confusion– to my own efforts in this area. For further details, see The Eightfold Cube.

In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
For radicals of another sort, see A Logocentric Meditation, A Mass for Lucero, and Steven Erlanger in The New York Times— "France Still Divided Over Lessons of 1968 Unrest."
The Klein Group as Kernel
of a Map from S4 to S3:
For those who prefer Galois's
politics to his mathematics,
there is
MAY 68: STREET POSTERS
FROM THE PARIS REBELLION
at London's Southbank Centre
(May 1 – June 1, 2008).

Thomas Wolfe
(Harvard M.A., 1922)
versus

Rosalind Krauss
(Harvard M.A., 1964,
Ph.D., 1969)
on
"No culture has a pact with eternity."
— George Steiner, interview in
The Guardian of
"At that instant he saw,
in one blaze of light, an image
of unutterable conviction….
the core of life, the essential
pattern whence all other things
proceed, the kernel of eternity."
— Thomas Wolfe, Of Time
and the River, quoted in
Log24 on June 9, 2005
From today's online Harvard Crimson:
"… under the leadership of Faust,
Harvard students should look forward
to an ever-growing opportunity for
international experience
and artistic endeavor."

Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen
From a recent book
on Wolfgang Pauli,
The Innermost Kernel:

A belated happy birthday
to the late
Felix Christian Klein
(born on April 25) —

Another Harvard figure quoted here on Dec. 5, 2002:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951)
From a review of Rosalind Krauss's The Optical Unconscious (MIT Press hardcover, 1993):
Krauss is concerned to present Modernism less in terms of its history than its structure, which she seeks to represent by means of a kind of diagram: "It is more interesting to think of modernism as a graph or table than a history." The "table" is a square with diagonally connected corners, of the kind most likely to be familiar to readers as the Square of Opposition, found in elementary logic texts since the mid-19th century. The square, as Krauss sees it, defines a kind of idealized space "within which to work out unbearable contradictions produced within the real field of history." This she calls, using the inevitable gallicism, "the site of Jameson's Political Unconscious" and then, in art, the optical unconscious, which consists of what Utopian Modernism had to kick downstairs, to repress, to "evacuate… from its field."
— Arthur C. Danto in ArtForum, Summer 1993
Rosalind Krauss in The Optical Unconscious (MIT Press paperback, 1994):
For a presentation of the Klein Group, see Marc Barbut, "On the Meaning of the Word 'Structure' in Mathematics," in Introduction to Structuralism, ed. Michael Lane (New York: Basic Books, 1970). Claude Lévi-Strauss uses the Klein group in his analysis of the relation between Kwakiutl and Salish masks in The Way of the Masks, trans. Sylvia Modelski (Seattle: University of Washington Press, 1982), p. 125; and in relation to the Oedipus myth in "The Structural Analysis of Myth," Structural Anthropology, trans. Claire Jackobson [sic] and Brooke Grundfest Schoepf (New York: Basic Books, 1963). In a transformation of the Klein Group, A. J. Greimas has developed the semiotic square, which he describes as giving "a slightly different formulation to the same structure," in "The Interaction of Semiotic Constraints," On Meaning (Minneapolis: University of Minnesota Press, 1987), p. 50. Jameson uses the semiotic square in The Political Unconscious (see pp. 167, 254, 256, 277) [Fredric Jameson, The Political Unconscious: Narrative as a Socially Symbolic Act (Ithaca: Cornell University Press, 1981)], as does Louis Marin in "Disneyland: A Degenerate Utopia," Glyph, no. 1 (1977), p. 64.
Wikipedia on the Klein group (denoted V, for Vierergruppe):
In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
For material related to Klee's phrase mentioned above by Stevens, "the organic center of all movement in time and space," see the following Google search:
Today is the 21st birthday of my note “The Relativity Problem in Finite Geometry.”
Some relevant quotations:
“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
Describing the branch of mathematics known as Galois theory, Weyl says that it
“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”
— Weyl, Symmetry, Princeton University Press, 1952, p. 138
Weyl’s set Sigma is a finite set of complex numbers. Some other sets with “discrete and finite character” are those of 4, 8, 16, or 64 points, arranged in squares and cubes. For illustrations, see Finite Geometry of the Square and Cube. What Weyl calls “the relativity problem” for these sets involves fixing “objectively” a class of equivalent coordinatizations. For what Weyl’s “objectively” means, see the article “Symmetry and Symmetry Breaking,” by Katherine Brading and Elena Castellani, in the Stanford Encyclopedia of Philosophy:
“The old and natural idea that what is objective should not depend upon the particular perspective under which it is taken into consideration is thus reformulated in the following group-theoretical terms: what is objective is what is invariant with respect to the transformation group of reference frames, or, quoting Hermann Weyl (1952, p. 132), ‘objectivity means invariance with respect to the group of automorphisms [of space-time].‘[22]
22. The significance of the notion of invariance and its group-theoretic treatment for the issue of objectivity is explored in Born (1953), for example. For more recent discussions see Kosso (2003) and Earman (2002, Sections 6 and 7).
References:
Born, M., 1953, “Physical Reality,” Philosophical Quarterly, 3, 139-149. Reprinted in E. Castellani (ed.), Interpreting Bodies: Classical and Quantum Objects in Modern Physics, Princeton, NJ: Princeton University Press, 1998, pp. 155-167.
Earman, J., 2002, “Laws, Symmetry, and Symmetry Breaking; Invariance, Conservation Principles, and Objectivity,’ PSA 2002, Proceedings of the Biennial Meeting of the Philosophy of Science Association 2002, forthcoming [Abstract/Preprint available online]
Kosso, P., 2003, “Symmetry, objectivity, and design,” in K. Brading and E. Castellani (eds.), Symmetries in Physics: Philosophical Reflections, Cambridge: Cambridge University Press, pp. 410-421.
Weyl, H., 1952, Symmetry, Princeton, NJ: Princeton University Press.
See also
Archives Henri Poincaré (research unit UMR 7117, at Université Nancy 2, of the CNRS)–
“Minkowski, Mathematicians, and the Mathematical Theory of Relativity,” by Scott Walter, in The Expanding Worlds of General Relativity (Einstein Studies, volume 7), H. Goenner, J. Renn, J. Ritter and T. Sauer, editors, Boston/Basel: Birkhäuser, 1999, pp. 45-86–
“Developing his ideas before Göttingen mathematicians in April 1909, Klein pointed out that the new theory based on the Lorentz group (which he preferred to call ‘Invariantentheorie’) could have come from pure mathematics (1910: 19). He felt that the new theory was anticipated by the ideas on geometry and groups that he had introduced in 1872, otherwise known as the Erlangen program (see Gray 1989: 229).”
References:
Gray, Jeremy J. (1989). Ideas of Space. 2d ed. Oxford: Oxford University Press.
Klein, Felix. (1910). “Über die geometrischen Grundlagen der Lorentzgruppe.” Jahresbericht der deutschen Mathematiker-Vereinigung 19: 281-300. [Reprinted: Physikalische Zeitschrift 12 (1911): 17-27].
Related material: A pathetically garbled version of the above concepts was published in 2001 by Harvard University Press. See Invariances: The Structure of the Objective World, by Robert Nozick.
By Indirections
(Hamlet, II, i)
“Michael Taylor (1971)…. contends that the central conflict in Hamlet is between ‘man as victim of fate and as controller of his own destiny.'”– The Gale Group, Shakespearean Criticism, Vol. 71, at eNotes
Doonesbury today:
“Personality is a synthesis of possibility and necessity.”– Soren Kierkegaard
On Fate (Necessity),
Freedom (Possibility),
and Machine Personality–
Part I: Google as Skynet
George Dyson–
The Godel-to-Google Net [March 8, 2005]
A Cathedral for Turing [October 24, 2005]
Dyson: “The correspondence between Google and biology is not an analogy, it’s a fact of life.”
Part II: The Galois Connection
David Ellerman–
“A Theory of Adjoint Functors– with some Thoughts about their Philosophical Significance” (pdf) [November 15, 2005]
Ellerman: “Such a mechanism seems key to understanding how an organism can perceive and learn from its environment without being under the direct stimulus control of the environment– thus resolving the ancient conundrum of receiving an external determination while exercising self-determination.”
For a less technical version, see Ellerman’s “Adjoints and Emergence: Applications of a New Theory of Adjoint Functors” (pdf).
Ellerman was apparently a friend of, and a co-author with, Gian-Carlo Rota. His “theory of adjoint functors” is related to the standard mathematical concepts known as profunctors, distributors, and bimodules. The applications of his theory, however, seem to be less to mathematics itself than to a kind of philosophical poetry that seems rather closely related to the above metaphors of George Dyson. For a less poetic approach to related purely mathematical concepts, see, for instance, the survey Practical Foundations of Mathematics by Paul Taylor (Cambridge University Press, 1999). For less poetically appealing, but perhaps more perspicuous, extramathematical applications of category theory, see the work of, for instance, Joseph Goguen: Algebraic Semiotics and Information Integration, Databases, and Ontologies.
An Epiphany
for the Birthday
of E. L. Doctorow,
Author of
City of God
(Doctorow wrote about
New York. A city more
closely associated with
God is Jerusalem.)
On the morning of January 2 this year, inspired by Sambin’s “basic picture,” I considered an entry dealing with Galois lattices (pdf). This train of thought was halted by news of the death earlier that morning of Teddy Kollek, 95, a founder of the Israeli intelligence service and six-term mayor of Jerusalem. (This led later to the entry “Damnation Morning“– a reference to the Fritz Leiber short story.)
This morning’s entry reboards the Galois train of thought.
Here are some relevant links:
Galois Connections (a French weblog entry providing an brief overview of Galois theory and an introduction to the use of Galois lattices in “formal concept analysis“)
Ontology (an introduction to formal concept analysis linked to on 3/31/06)
One motive for resuming consideration of Galois lattices today is to honor the late A. Richard Newton, a pioneer in engineering design who died at 55– also on Tuesday, Jan. 2, the date of Kollek’s death. Today’s New York Times obituary for Newton says that “most recently, Professor Newton championed the study of synthetic biology.”
A check of syntheticbiology.org leads to a web page on– again– ontology.
For the relationship between ontology (in the semantic-web sense) and Galois lattices, see (for instance)
“Knowledge Organisation and Information Retrieval Using Galois Lattices” (ps) and its references.
An epiphany within all this that Doctorow might appreciate is the following from Wikipedia, found by following a link to “upper ontology” in the syntheticbiology.org ontology page:
- There is no self-evident way of dividing the world up into concepts.
- There is no neutral ground that can serve as a means of translating between specialized (lower) ontologies.
- Human language itself is already an arbitrary approximation of just one among many possible conceptual maps. To draw any necessary correlation between English words and any number of intellectual concepts we might like to represent in our ontologies is just asking for trouble.
Related material:
The intellectual concepts
mentioned by Richard Powers
at the end of tomorrow’s
New York Times Book Review.
(See the links on these concepts
in yesterday’s “Goldberg Variation.”)
See also Old School Tie.

— From Pedagogy, Praxis, Ulysses
A quotation omitted from the above excerpt:
In Ulysses, there is "… the same quality of simultaneity as in cubist collage. Thus, for example, Bloom surveys the tombstones at Paddy Dignam's funeral and, in the midst of platitudinous and humorous thoughts, remembers Molly 'wanting to do it at the window'…."
Related material from quotations at the poetry journal eratio:
"The guiding law of the great variations in painting is one of disturbing simplicity. First things are painted; then, sensations; finally, ideas. This means that in the beginning the artist's attention was fixed on external reality; then, on the subjective; finally, on the intrasubjective. These three stages are three points on a straight line."
— Jose Ortega y Gasset ("On Point of View in the Arts," an essay on the development of cubism)
Related material on
tombstones and windows:
Geometry's Tombstones,
Galois's Window, and
Architecture of Eternity.

See also the following part
of the eratio quotations:

Quotations arranged by
Gregory Vincent St. Thomasino
Geometry
from Point
to Hyperspace
by Steven H. Cullinane
Euclid is “the most famous
geometer ever known
and for good reason:
for millennia it has been
his window
that people first look through
when they view geometry.”
— Euclid’s Window:
The Story of Geometry
from Parallel Lines
to Hyperspace,
by Leonard Mlodinow
“…the source of
all great mathematics
is the special case,
the concrete example.
It is frequent in mathematics
that every instance of a
concept of seemingly
great generality is
in essence the same as
a small and concrete
special case.”
— Paul Halmos in
I Want To Be a Mathematician
Euclid’s geometry deals with affine
spaces of 1, 2, and 3 dimensions
definable over the field
of real numbers.
Each of these spaces
has infinitely many points.
Some simpler spaces are those
defined over a finite field–
i.e., a “Galois” field–
for instance, the field
which has only two
elements, 0 and 1, with
addition and multiplication
as follows:
|
|

From these five finite spaces,
we may, in accordance with
Halmos’s advice,
select as “a small and
concrete special case”
the 4-point affine plane,
which we may call

Galois’s Window.
The interior lines of the picture
are by no means irrelevant to
the space’s structure, as may be
seen by examining the cases of
the above Galois affine 3-space
and Galois affine hyperplane
in greater detail.
For more on these cases, see
The Eightfold Cube,
Finite Relativity,
The Smallest Projective Space,
Latin-Square Geometry, and
Geometry of the 4×4 Square.
(These documents assume that
the reader is familar with the
distinction between affine and
projective geometry.)
These 8- and 16-point spaces
may be used to
illustrate the action of Klein’s
simple group of order 168
and the action of
a subgroup of 322,560 elements
within the large Mathieu group.
The view from Galois’s window
also includes aspects of
quantum information theory.
For links to some papers
in this area, see
Elements of Finite Geometry.
Serious
"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."
— Charles Matthews at Wikipedia, Oct. 2, 2006
"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."
— G. H. Hardy, A Mathematician's Apology
Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:
Affine group
Reflection group
Symmetry in mathematics
Incidence structure
Invariant (mathematics)
Symmetry
Finite geometry
Group action
History of geometry
This would appear to be a fairly large complex of mathematical ideas.
See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:
Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.
A Multicultural Farewell
to a winner of the
Nobel Prize for Literature,
the Egyptian author of
The Seventh Heaven:
Supernatural Stories —


"Jackson has identified
the seventh symbol."
— Stargate
Other versions of
the seventh symbol —



"… Max Black, the Cornell philosopher, and others have pointed out how 'perhaps every science must start with metaphor and end with algebra, and perhaps without the metaphor there would never have been any algebra' …."
— Max Black, Models and Metaphors, Cornell U. Press, 1962, page 242, as quoted in Dramas, Fields, and Metaphors, by Victor Witter Turner, Cornell U. Press, paperback, 1975, page 25
Augustine of Hippo, who is said to
have died on this date in 430 A.D.
"He is, after all, not merely taking over a Neoplatonic ontology, but he is attempting to combine it with a scriptural tradition of a rather different sort, one wherein the divine attributes most prized in the Greek tradition (e.g. necessity, immutability, and atemporal eternity) must somehow be combined with the personal attributes (e.g. will, justice, and historical purpose) of the God of Abraham, Isaac, and Jacob."
— Stanford Encyclopedia of Philosophy on Augustine
Here is a rather different attempt
to combine the eternal with the temporal:
|
The Eternal
Symbol of necessity,
For details, see |
The Temporal
Symbol of the
For details, see |
|
The eternal
combined with the temporal:
|
|
Related material:
Today’s birthday:
Tom Hanks, star of
“The Da Vinci Code”
Part III:
The Leonardo Connection
Part IV:
Nicholson’s Grail Quest
— Ben Nicholson in a 2005 interview
Nicholson’s quest has apparently lasted for some time. Promotional material for a 1996 Nicholson exhibit in Montreal says it “invites visitors of all ages to experience a contemporary architect’s search for order, meaning and logic in a world of art, science and mystery.” The title of that exhibit was “Uncovering Geometry.”
For web pages to which this same title might apply, see Quilt Geometry, Galois Geometry, and Finite Geometry of the Square and Cube.
* “Square Kufi” calligraphy is used in Islamic architectural ornament. I do not know what, if anything, is signified by Nicholson’s 6×12 example of “Kufi blocks” shown above.
Binary Geometry
There is currently no area of mathematics named “binary geometry.” This is, therefore, a possible name for the geometry of sets with 2n elements (i.e., a sub-topic of Galois geometry and of algebraic geometry over finite fields– part of Weil’s “Rosetta stone” (pdf)).
Examples:
|
The Fano Plane
by Ed Pegg Jr.,
“One thing in the Fano plane that bothered me for years (for years, I say) is that it had a circle – and it was described as a line. For me, a line was a straight line, and I didn’t trust curved or wriggly lines. This distrust kept me away from understanding projective planes, designs, and finite geometries for a awhile (for years).”
|
— Schiller,* quoted as
the epigraph to the
chapter on Galois in
Men of Mathematics,
by E. T. Bell
Related material:
Galois Geometry
* From Die Jungfrau von Orleans
(The Maid of Orleans), Act III, sc. vi.
Today is the feast of that Jungfrau.
A Living Church
continued from March 27
— G. K. Chesterton
 Shakespearean Fool |

as well as
and the remarks
of Oxford professor
Marcus du Sautoy,
who claims that
"the right side of the brain
is responsible for mathematics."
Let us hope that Professor du Sautoy
is more reliable on zeta functions,
his real field of expertise,
than on neurology.
The picture below may help
to clear up his confusion
between left and right.
His confusion about
pseudoscience may not
be so easily remedied.

flickr.com/photos/jaycross/3975200/
(Any resemblance to the film
"Hannibal" is purely coincidental.)
“There is a pleasantly discursive treatment of Pontius Pilate’s unanswered question ‘What is truth?'”
— H. S. M. Coxeter, 1987, introduction to Richard J. Trudeau’s remarks on the “Story Theory” of truth as opposed to the “Diamond Theory” of truth in The Non-Euclidean Revolution
“A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes*….”
— Richard J. Trudeau in
The Non-Euclidean Revolution
“‘Deniers’ of truth… insist that each of us is trapped in his own point of view; we make up stories about the world and, in an exercise of power, try to impose them on others.”
— Jim Holt in The New Yorker.
(Click on the box below.)
Exercise of Power:
Show that a white horse–

a figure not unlike the
symbol of the mathematics
publisher Springer–
is traced, within a naturally
arranged rectangular array of
polynomials, by the powers of x
modulo a polynomial
irreducible over a Galois field.
This horse, or chess knight–
“Springer,” in German–
plays a role in “Diamond Theory”
(a phrase used in finite geometry
in 1976, some years before its use
by Trudeau in the above book).
Related material
On this date:
In 1490, The White Knight
(Tirant lo Blanc  )–
)–
a major influence on Cervantes–
was published, and in 1910
the Mexican Revolution began.
Illustration:
Zapata by Diego Rivera,
Museum of Modern Art,
New York
“First published in the Catalan language in Valencia in 1490…. Reviewing the first modern Spanish translation in 1969 (Franco had ruthlessly suppressed the Catalan language and literature), Mario Vargas Llosa hailed the epic’s author as ‘the first of that lineage of God-supplanters– Fielding, Balzac, Dickens, Flaubert, Tolstoy, Joyce, Faulkner– who try to create in their novels an all-encompassing reality.'”
Oslo Connection
Today is the birthday of Oystein Ore (1899-1968), Sterling Professor of Mathematics at Yale for 37 years, who was born and died in Oslo, Norway. Ore is said to have coined the term “Galois connection.” In his honor, an excerpt dealing with such connections:
Today's mathematical birthdays:
Saunders Mac Lane, John Venn,
and Sir William Rowan Hamilton.
It is well known that the quaternion group is a subgroup of GL(2,3), the general linear group on the 2-space over GF(3), the 3-element Galois field.
The figures below illustrate this fact.

Related material: Visualizing GL(2,p)
— J. L. Alperin, book review,
Bulletin (New Series) of the American
Mathematical Society 10 (1984), 121

— Billy Graham Evangelistic Association,
according to messiahpage.com
"… just as God defeats the devil:
this bridge exists;
it is the theory of the field
of algebraic functions over
a finite field of constants
(that is to say, a finite number
of elements: also said to be a Galois
field, or earlier 'Galois imaginaries'
because Galois first defined them
and studied them….)"
— André Weil, 1940 letter to his sister,
Simone Weil, alias Simone Galois
(see previous entry)
Related material:
Billy Graham and the City:
A Later Look at His Words
— New York Times, June 24, 2005
Earendil_Silmarils:

Les Anamorphoses:

"Pour construire un dessin en perspective,
le peintre trace sur sa toile des repères:
la ligne d'horizon (1),
le point de fuite principal (2)
où se rencontre les lignes de fuite (3)
et le point de fuite des diagonales (4)."
_______________________________
Serge Mehl,
Perspective &
Géométrie Projective:
"… la géométrie projective était souvent
synonyme de géométrie supérieure.
Elle s'opposait à la géométrie
euclidienne: élémentaire…
La géométrie projective, certes supérieure
car assez ardue, permet d'établir
de façon élégante des résultats de
la géométrie élémentaire."
Similarly…
Finite projective geometry
(in particular, Galois geometry)
is certainly superior to
the elementary geometry of
quilt-pattern symmetry
and allows us to establish
de façon élégante
some results of that
elementary geometry.
Other Related Material…
from algebra rather than
geometry, and from a German
rather than from the French:
"This is the relativity problem:
to fix objectively a class of
equivalent coordinatizations
and to ascertain
the group of transformations S
mediating between them."
— Hermann Weyl,
The Classical Groups,
Princeton U. Press, 1946

Evariste Galois
Weyl also says that the profound branch
of mathematics known as Galois theory
"Perhaps every science must
start with metaphor
and end with algebra;
and perhaps without metaphor
there would never have been
any algebra."
For metaphor and
algebra combined, see
A.M.S. abstract 79T-A37,
Notices of the
American Mathematical Society,
February 1979, pages A-193, 194 —
the original version of the 4×4 case
of the diamond theorem.
"When approaching unfamiliar territory, we often, as observed earlier, try to describe or frame the novel situation using metaphors based on relations perceived in a familiar domain, and by using our powers of association, and our ability to exploit the structural similarity, we go on to conjecture new features for consideration, often not noticed at the outset. The metaphor works, according to Max Black, by transferring the associated ideas and implications of the secondary to the primary system, and by selecting, emphasising and suppressing features of the primary in such a way that new slants on it are illuminated."
— Paul Thompson, University College, Oxford,
The Nature and Role of Intuition
in Mathematical Epistemology
That intuition, metaphor (i.e., analogy), and association may lead us astray is well known. The examples of French perspective above show what might happen if someone ignorant of finite geometry were to associate the phrase "4×4 square" with the phrase "projective geometry." The results are ridiculously inappropriate, but at least the second example does, literally, illuminate "new slants"– i.e., diagonals– within the perspective drawing of the 4×4 square.
Similarly, analogy led the ancient Greeks to believe that the diagonal of a square is commensurate with the side… until someone gave them a new slant on the subject.

or, The Eightfold Cube

Every permutation of the plane's points that preserves collinearity is a symmetry of the plane. The group of symmetries of the Fano plane is of order 168 and is isomorphic to the group PSL(2,7) = PSL(3,2) = GL(3,2). (See Cameron on linear groups (pdf).)
The above model indicates with great clarity six symmetries of the plane– those it shares with the equilateral triangle. It does not, however, indicate where the other 162 symmetries come from.
Shown below is a new model of this same projective plane, using partitions of cubes to represent points:

The second model is useful because it lets us generate naturally all 168 symmetries of the Fano plane by splitting a cube into a set of four parallel 1x1x2 slices in the three ways possible, then arbitrarily permuting the slices in each of the three sets of four. See examples below.

(Note that this procedure, if regarded as acting on the set of eight individual subcubes of each cube in the diagram, actually generates a group of 168*8 = 1,344 permutations. But the group's action on the diagram's seven partitions of the subcubes yields only 168 distinct results. This illustrates the difference between affine and projective spaces over the binary field GF(2). In a related 2x2x2 cubic model of the affine 3-space over GF(2) whose "points" are individual subcubes, the group of eight translations is generated by interchanges of parallel 2x2x1 cube-slices. This is clearly a subgroup of the group generated by permuting 1x1x2 cube-slices. Such translations in the affine 3-space have no effect on the projective plane, since they leave each of the plane model's seven partitions– the "points" of the plane– invariant.)
To view the cubes model in a wider context, see Galois Geometry, Block Designs, and Finite-Geometry Models.
For another application of the points-as-partitions technique, see Latin-Square Geometry: Orthogonal Latin Squares as Skew Lines.
For more on the plane's symmetry group in another guise, see John Baez on Klein's Quartic Curve and the online book The Eightfold Way. For more on the mathematics of cubic models, see Solomon's Cube.

“The Divine Proportion…
is an irrational number and
the positive solution
of the quadratic equation
The Greek letter ‘phi’
(see below for the symbol)
is sometimes used
to represent this number.”

For another approach to
the divine proportion, see
Apart from its intrinsic appeal, that is the reason for treating the construction of the pentagon, and our task today will be to acquire some feel for this construction. It is not easy.”
— R. P. Langlands, 1999 lecture (pdf) at the Institute for Advanced Study, Princeton, in the spirit of Hermann Weyl
Old School Tie
“We are introduced to John Nash, fuddling flat-footed about the Princeton courtyard, uninterested in his classmates’ yammering about their various accolades. One chap has a rather unfortunate sense of style, but rather than tritely insult him, Nash holds a patterned glass to the sun, [director Ron] Howard shows us refracted patterns of light that take shape in a punch bowl, which Nash then displaces onto the neckwear, replying, ‘There must be a formula for how ugly your tie is.’ ”

“Algebra in general is particularly suited for structuring and abstracting. Here, structure is imposed via symmetries and dualities, for instance in terms of Galois connections……. diamonds and boxes are upper and lower adjoints of Galois connections….”

Evariste Galois
“Perhaps every science must
start with metaphor
and end with algebra;
and perhaps without metaphor
there would never have been
any algebra.”
— attributed, in varying forms
(1, 2, 3), to Max Black,
Models and Metaphors, 1962
For metaphor and
algebra combined, see
“Symmetry invariance
in a diamond ring,”
A.M.S. abstract 79T-A37,
Notices of the Amer. Math. Soc.,
February 1979, pages A-193, 194 —
the original version of the 4×4 case
of the diamond theorem.
Finite Relativity
Today is the 18th birthday of my note
“The Relativity Problem in Finite Geometry.”
That note begins with a quotation from Weyl:
“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
Here is another quotation from Weyl, on the profound branch of mathematics known as Galois theory, which he says
“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”
— Weyl, Symmetry, Princeton University Press, 1952, p. 138
This second quotation applies equally well to the much less profound, but more accessible, part of mathematics described in Diamond Theory and in my note of Feb. 20, 1986.
720 in the Book
Searching for an epiphany on this January 6 (the Feast of the Epiphany), I started with Harvard Magazine, the current issue of January-February 2004.
An article titled On Mathematical Imagination concludes by looking forward to
“a New Instauration that will bring mathematics, at last, into its rightful place in our lives: a source of elation….”
Seeking the source of the phrase “new instauration,” I found it was due to Francis Bacon, who “conceived his New Instauration as the fulfilment of a Biblical prophecy and a rediscovery of ‘the seal of God on things,’ ” according to a web page by Nieves Mathews.
Hmm.
The Mathews essay leads to Peter Pesic, who, it turns out, has written a book that brings us back to the subject of mathematics:
Abel’s Proof: An Essay
on the Sources and Meaning
of Mathematical Unsolvability
by Peter Pesic,
MIT Press, 2003
From a review:
“… the book is about the idea that polynomial equations in general cannot be solved exactly in radicals….
Pesic concludes his account after Abel and Galois… and notes briefly (p. 146) that following Abel, Jacobi, Hermite, Kronecker, and Brioschi, in 1870 Jordan proved that elliptic modular functions suffice to solve all polynomial equations. The reader is left with little clarity on this sequel to the story….”
— Roger B. Eggleton, corrected version of a review in Gazette Aust. Math. Soc., Vol. 30, No. 4, pp. 242-244
Here, it seems, is my epiphany:
“Elliptic modular functions suffice to solve all polynomial equations.”
Incidental Remarks
on Synchronicity,
Part I
Those who seek a star
on this Feast of the Epiphany
may click here.
Most mathematicians are (or should be) familiar with the work of Abel and Galois on the insolvability by radicals of quintic and higher-degree equations.
Just how such equations can be solved is a less familiar story. I knew that elliptic functions were involved in the general solution of a quintic (fifth degree) equation, but I was not aware that similar functions suffice to solve all polynomial equations.
The topic is of interest to me because, as my recent web page The Proof and the Lie indicates, I was deeply irritated by the way recent attempts to popularize mathematics have sown confusion about modular functions, and I therefore became interested in learning more about such functions. Modular functions are also distantly related, via the topic of “moonshine” and via the “Happy Family” of the Monster group and the Miracle Octad Generator of R. T. Curtis, to my own work on symmetries of 4×4 matrices.
Incidental Remarks
on Synchronicity,
Part II
There is no Log24 entry for
December 30, 2003,
the day John Gregory Dunne died,
but see this web page for that date.
Here is what I was able to find on the Web about Pesic’s claim:
From Wolfram Research:
From Solving the Quintic —
“Some of the ideas described here can be generalized to equations of higher degree. The basic ideas for solving the sextic using Klein’s approach to the quintic were worked out around 1900. For algebraic equations beyond the sextic, the roots can be expressed in terms of hypergeometric functions in several variables or in terms of Siegel modular functions.”
From Siegel Theta Function —
“Umemura has expressed the roots of an arbitrary polynomial in terms of Siegel theta functions. (Mumford, D. Part C in Tata Lectures on Theta. II. Jacobian Theta Functions and Differential Equations. Boston, MA: Birkhäuser, 1984.)”
From Polynomial —
“… the general quintic equation may be given in terms of the Jacobi theta functions, or hypergeometric functions in one variable. Hermite and Kronecker proved that higher order polynomials are not soluble in the same manner. Klein showed that the work of Hermite was implicit in the group properties of the icosahedron. Klein’s method of solving the quintic in terms of hypergeometric functions in one variable can be extended to the sextic, but for higher order polynomials, either hypergeometric functions in several variables or ‘Siegel functions’ must be used (Belardinelli 1960, King 1996, Chow 1999). In the 1880s, Poincaré created functions which give the solution to the nth order polynomial equation in finite form. These functions turned out to be ‘natural’ generalizations of the elliptic functions.”
Belardinelli, G. “Fonctions hypergéométriques de plusieurs variables er résolution analytique des équations algébrique générales.” Mémoral des Sci. Math. 145, 1960.
King, R. B. Beyond the Quartic Equation. Boston, MA: Birkhäuser, 1996.
Chow, T. Y. “What is a Closed-Form Number.” Amer. Math. Monthly 106, 440-448, 1999.
From Angel Zhivkov,
Preprint series,
Institut für Mathematik,
Humboldt-Universität zu Berlin:
“… discoveries of Abel and Galois had been followed by the also remarkable theorems of Hermite and Kronecker: in 1858 they independently proved that we can solve the algebraic equations of degree five by using an elliptic modular function…. Kronecker thought that the resolution of the equation of degree five would be a special case of a more general theorem which might exist. This hypothesis was realized in [a] few cases by F. Klein… Jordan… showed that any algebraic equation is solvable by modular functions. In 1984 Umemura realized the Kronecker idea in his appendix to Mumford’s book… deducing from a formula of Thomae… a root of [an] arbitrary algebraic equation by Siegel modular forms.”
— “Resolution of Degree Less-than-or-equal-to Six Algebraic Equations by Genus Two Theta Constants“
Incidental Remarks
on Synchronicity,
Part III
From Music for Dunne’s Wake:
“Heaven was kind of a hat on the universe,
a lid that kept everything underneath it
where it belonged.”
— Carrie Fisher,
Postcards from the Edge
|
“720 in |
“The group Sp4(F2) has order 720,”
as does S6. — Angel Zhivkov, op. cit.
Those seeking
“a rediscovery of
‘the seal of God on things,’ “
as quoted by Mathews above,
should see
The Unity of Mathematics
and the related note
Sacerdotal Jargon.

For more remarks on synchronicity
that may or may not be relevant
to Harvard Magazine and to
the annual Joint Mathematics Meetings
that start tomorrow in Phoenix, see
For the relevance of the time
of this entry, 10:10, see
|
Related recreational reading:
|
Labyrinth |
|
Mental Health Month, Day 26:
Many Dimensions,
Part II — The Blue Matrix
But seriously…
John Baez in July 1999:
"…it's really the fact that the Leech lattice is 24-dimensional that lets us compactify 26-dimensional spacetime in such a way as to get a bosonic string theory with the Monster group as symmetries."
Well, maybe. I certainly hope so. If the Leech lattice and the Monster group turn out to have some significance in theoretical physics, then my own work, which deals with symmetries of substructures of the Leech lattice and the Monster, might be viewed in a different light. Meanwhile, I take (cold) comfort from some writers who pursue the "story" theory of truth, as opposed to the "diamond" theory. See the following from my journal:
Evariste Galois and the Rock that Changed Things, and
A Time to Gather Stones Together: Readings for Yom Kippur.
See, too, this web page on Marion Zimmer Bradley's fictional
Matrices, or Blue Star-Stones, and

the purely mathematical site Diamond Theory, which deals with properties of the above "blue matrix" and its larger relatives.
A Logocentric Archetype
Today we examine the relativist, nominalist, leftist, nihilist, despairing, depressing, absurd, and abominable work of Samuel Beckett, darling of the postmodernists.
One lens through which to view Beckett is an essay by Jennifer Martin, "Beckettian Drama as Protest: A Postmodern Examination of the 'Delogocentering' of Language." Martin begins her essay with two quotations: one from the contemptible French twerp Jacques Derrida, and one from Beckett's masterpiece of stupidity, Molloy. For a logocentric deconstruction of Derrida, see my note, "The Shining of May 29," which demonstrates how Derrida attempts to convert a rather important mathematical result to his brand of nauseating and pretentious nonsense, and of course gets it wrong. For a logocentric deconstruction of Molloy, consider the following passage:
"I took advantage of being at the seaside to lay in a store of sucking-stones. They were pebbles but I call them stones…. I distributed them equally among my four pockets, and sucked them turn and turn about. This raised a problem which I first solved in the following way. I had say sixteen stones, four in each of my four pockets these being the two pockets of my trousers and the two pockets of my greatcoat. Taking a stone from the right pocket of my greatcoat, and putting it in my mouth, I replaced it in the right pocket of my greatcoat by a stone from the right pocket of my trousers, which I replaced by a stone from the left pocket of my trousers, which I replaced by a stone from the left pocket of my greatcoat, which I replaced by the stone which was in my mouth, as soon as I had finished sucking it. Thus there were still four stones in each of my four pockets, but not quite the same stones….But this solution did not satisfy me fully. For it did not escape me that, by an extraordinary hazard, the four stones circulating thus might always be the same four."
Beckett is describing, in great detail, how a damned moron might approach the extraordinarily beautiful mathematical discipline known as group theory, founded by the French anticleric and leftist Evariste Galois. Disciples of Derrida may play at mimicking the politics of Galois, but will never come close to imitating his genius. For a worthwhile discussion of permutation groups acting on a set of 16 elements, see R. D. Carmichael's masterly work, Introduction to the Theory of Groups of Finite Order, Ginn, Boston, 1937, reprinted by Dover, New York, 1956.
There are at least two ways of approaching permutations on 16 elements in what Pascal calls "l'esprit géométrique." My website Diamond Theory discusses the action of the affine group in a four-dimensional finite geometry of 16 points. For a four-dimensional euclidean hypercube, or tesseract, with 16 vertices, see the highly logocentric movable illustration by Harry J. Smith. The concept of a tesseract was made famous, though seen through a glass darkly, by the Christian writer Madeleine L'Engle in her novel for children and young adults, A Wrinkle in Tme.
This tesseract may serve as an archetype for what Pascal, Simone Weil (see my earlier notes), Harry J. Smith, and Madeleine L'Engle might, borrowing their enemies' language, call their "logocentric" philosophy.
For a more literary antidote to postmodernist nihilism, see Archetypal Theory and Criticism, by Glen R. Gill.
For a discussion of the full range of meaning of the word "logos," which has rational as well as religious connotations, click here.
Evariste Galois and
The Rock That Changed Things
An article in the current New York Review of Books (dated Sept. 26) on Ursula K. Le Guin prompted me to search the Web this evening for information on a short story of hers I remembered liking. I found the following in the journal of mathematician Peter Berman:
I agree that the story is elegant (from a mathematician, a high compliment), so searched Berman’s pages further, finding this:
between The French Mathematician (a novel about Galois) and Harry Potter and the Sorcerer’s Stone.
My own version of the Philosopher’s Stone (the phrase used instead of “Sorcerer’s Stone” in the British editions of Harry Potter) appears in my profile picture at top left; see also the picture of Plato’s diamond figure in my main math website. The mathematics of finite (or “Galois”) fields plays a role in the underlying theory of this figure’s hidden symmetries. Since the perception of color plays a large role in the Le Guin story and since my version of Plato’s diamond is obtained by coloring Plato’s version, this particular “rock that changes things” might, I hope, inspire Berman to extend his table to include Le Guin’s tale as well.
Even the mosaic theme is appropriate, this being the holiest of the Mosaic holy days.
Dr. Berman, G’mar Chatimah Tova.
Powered by WordPress