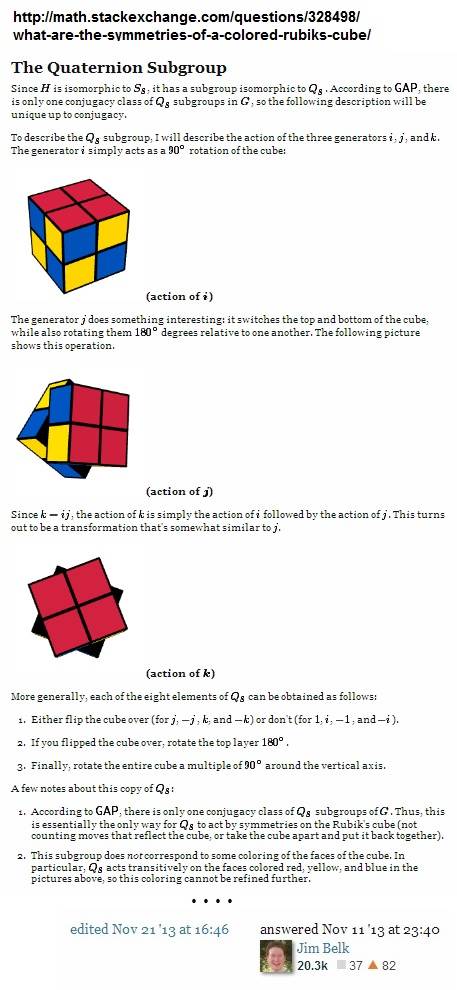
The previous post told how user58512 at math.stackexchange.com
sought in 2013 a geometric representation of Q8 , the quaternion group.
He ended up displaying an illustration that very possibly was drawn,
without any acknowledgement of its source, from my own work.
On the date that user58512 published that illustration, he further
pursued his March 1, 2013, goal of a “twisty” quaternion model.
On March 12, 2013, he suggested that the quaternion group might be
the symmetry group of the following twisty-cube coloring:
Illustration by Jim Belk
Here is part of a reply by Jim Belk from Nov. 11, 2013, elaborating on
that suggestion:
Belk argues that the colored cube is preserved under the group
of actions he describes. It is, however, also preserved under a
larger group. (Consider, say, rotation of the entire cube by 180
degrees about the center of any one of its checkered faces.) The
group Belk describes seems therefore to be a symmetry group,
not the symmetry group, of the colored cube.
I do not know if any combination puzzle has a coloring with
precisely the quaternion group as its symmetry group.
(Updated at 12:15 AM June 6 to point out the larger symmetry group
and delete a comment about an arXiv paper on quaternion group models.)