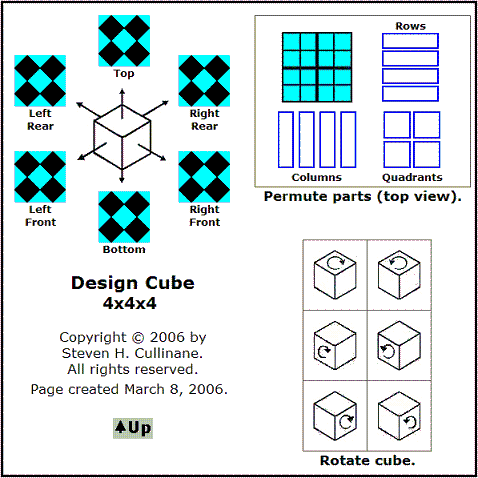
720 in the Book
Searching for an epiphany on this January 6 (the Feast of the Epiphany), I started with Harvard Magazine, the current issue of January-February 2004.
An article titled On Mathematical Imagination concludes by looking forward to
“a New Instauration that will bring mathematics, at last, into its rightful place in our lives: a source of elation….”
Seeking the source of the phrase “new instauration,” I found it was due to Francis Bacon, who “conceived his New Instauration as the fulfilment of a Biblical prophecy and a rediscovery of ‘the seal of God on things,’ ” according to a web page by Nieves Mathews.
Hmm.
The Mathews essay leads to Peter Pesic, who, it turns out, has written a book that brings us back to the subject of mathematics:
Abel’s Proof: An Essay
on the Sources and Meaning
of Mathematical Unsolvability
by Peter Pesic,
MIT Press, 2003
From a review:
“… the book is about the idea that polynomial equations in general cannot be solved exactly in radicals….
Pesic concludes his account after Abel and Galois… and notes briefly (p. 146) that following Abel, Jacobi, Hermite, Kronecker, and Brioschi, in 1870 Jordan proved that elliptic modular functions suffice to solve all polynomial equations. The reader is left with little clarity on this sequel to the story….”
— Roger B. Eggleton, corrected version of a review in Gazette Aust. Math. Soc., Vol. 30, No. 4, pp. 242-244
Here, it seems, is my epiphany:
“Elliptic modular functions suffice to solve all polynomial equations.”
Incidental Remarks
on Synchronicity,
Part I
Those who seek a star
on this Feast of the Epiphany
may click here.
Most mathematicians are (or should be) familiar with the work of Abel and Galois on the insolvability by radicals of quintic and higher-degree equations.
Just how such equations can be solved is a less familiar story. I knew that elliptic functions were involved in the general solution of a quintic (fifth degree) equation, but I was not aware that similar functions suffice to solve all polynomial equations.
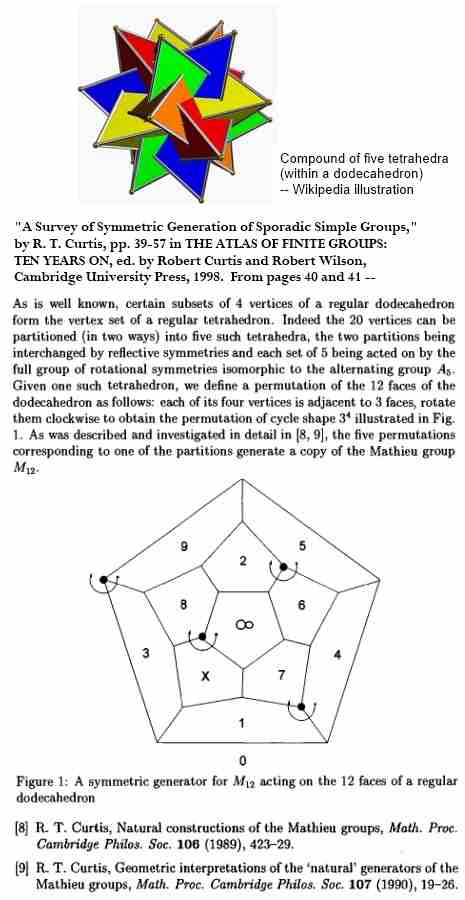
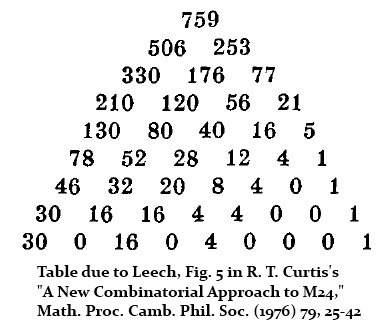
The topic is of interest to me because, as my recent web page The Proof and the Lie indicates, I was deeply irritated by the way recent attempts to popularize mathematics have sown confusion about modular functions, and I therefore became interested in learning more about such functions. Modular functions are also distantly related, via the topic of “moonshine” and via the “Happy Family” of the Monster group and the Miracle Octad Generator of R. T. Curtis, to my own work on symmetries of 4×4 matrices.
Incidental Remarks
on Synchronicity,
Part II
There is no Log24 entry for
December 30, 2003,
the day John Gregory Dunne died,
but see this web page for that date.
Here is what I was able to find on the Web about Pesic’s claim:
From Wolfram Research:
From Solving the Quintic —
“Some of the ideas described here can be generalized to equations of higher degree. The basic ideas for solving the sextic using Klein’s approach to the quintic were worked out around 1900. For algebraic equations beyond the sextic, the roots can be expressed in terms of hypergeometric functions in several variables or in terms of Siegel modular functions.”
From Siegel Theta Function —
“Umemura has expressed the roots of an arbitrary polynomial in terms of Siegel theta functions. (Mumford, D. Part C in Tata Lectures on Theta. II. Jacobian Theta Functions and Differential Equations. Boston, MA: Birkhäuser, 1984.)”
From Polynomial —
“… the general quintic equation may be given in terms of the Jacobi theta functions, or hypergeometric functions in one variable. Hermite and Kronecker proved that higher order polynomials are not soluble in the same manner. Klein showed that the work of Hermite was implicit in the group properties of the icosahedron. Klein’s method of solving the quintic in terms of hypergeometric functions in one variable can be extended to the sextic, but for higher order polynomials, either hypergeometric functions in several variables or ‘Siegel functions’ must be used (Belardinelli 1960, King 1996, Chow 1999). In the 1880s, Poincaré created functions which give the solution to the nth order polynomial equation in finite form. These functions turned out to be ‘natural’ generalizations of the elliptic functions.”
Belardinelli, G. “Fonctions hypergéométriques de plusieurs variables er résolution analytique des équations algébrique générales.” Mémoral des Sci. Math. 145, 1960.
King, R. B. Beyond the Quartic Equation. Boston, MA: Birkhäuser, 1996.
Chow, T. Y. “What is a Closed-Form Number.” Amer. Math. Monthly 106, 440-448, 1999.
From Angel Zhivkov,
Preprint series,
Institut für Mathematik,
Humboldt-Universität zu Berlin:
“… discoveries of Abel and Galois had been followed by the also remarkable theorems of Hermite and Kronecker: in 1858 they independently proved that we can solve the algebraic equations of degree five by using an elliptic modular function…. Kronecker thought that the resolution of the equation of degree five would be a special case of a more general theorem which might exist. This hypothesis was realized in [a] few cases by F. Klein… Jordan… showed that any algebraic equation is solvable by modular functions. In 1984 Umemura realized the Kronecker idea in his appendix to Mumford’s book… deducing from a formula of Thomae… a root of [an] arbitrary algebraic equation by Siegel modular forms.”
— “Resolution of Degree Less-than-or-equal-to Six Algebraic Equations by Genus Two Theta Constants“
Incidental Remarks
on Synchronicity,
Part III
From Music for Dunne’s Wake:
“Heaven was kind of a hat on the universe,
a lid that kept everything underneath it
where it belonged.”
— Carrie Fisher,
Postcards from the Edge
“The group Sp4(F2) has order 720,”
as does S6. — Angel Zhivkov, op. cit.
Those seeking
“a rediscovery of
‘the seal of God on things,’ “
as quoted by Mathews above,
should see
The Unity of Mathematics
and the related note
Sacerdotal Jargon.

For more remarks on synchronicity
that may or may not be relevant
to Harvard Magazine and to
the annual Joint Mathematics Meetings
that start tomorrow in Phoenix, see
Log24, June 2003.
For the relevance of the time
of this entry, 10:10, see
- the reference to Paradise
on the “postcard” above, and
- Storyline (10/10, 2003).
|
Related recreational reading:






























































 pure as one of Bach's inverted canons or Euler's formula for polyhedra."
pure as one of Bach's inverted canons or Euler's formula for polyhedra."