Monday, September 9, 2024
For “The Perfect Couple” — Facets and Labyrinth
Comments Off on For “The Perfect Couple” — Facets and Labyrinth
Tuesday, September 3, 2024
Mission: Possible — Facets and Labyrinth
"… to explore what it means to be human
in all the facets of that and the labyrinth of that."
Comments Off on Mission: Possible — Facets and Labyrinth
Wednesday, December 23, 2020
Facets . . .
“The book by Hesse has many facets ….” (Link added.)
— V. V. Nalimov, In the Labyrinths of Language ,
Ch. 1, “What Language Is,” p. 22.
Related philosophical speculation —
Comments Off on Facets . . .
Sunday, December 20, 2020
Facets
Comments Off on Facets
Wednesday, May 15, 2019
Facets
". . . the most magnificent 'object' in all of mathematics . . . .
is like a diamond with thousands of facets . . . ."
— MIT professor emeritus quoted here on Aug. 19, 2008
Also on that date —
Comments Off on Facets
Monday, April 23, 2018
Facets
See also the Feb. 17, 2017, post on Bertram Kostant
as well as "Mathieu Moonshine and Symmetry Surfing."
Comments Off on Facets
Wednesday, December 25, 2013
Rotating the Facets
“… her mind rotated the facts….”
Related material— hypercube rotation,* in the context
of rotational symmetries of the Platonic solids:
“I’ve heard of affairs that are strictly Platonic”
* Footnote added on Dec. 26, 2013 —
See Arnold Emch, “Triple and Multiple Systems, Their Geometric
Configurations and Groups,” Trans. Amer. Math. Soc. 31 (1929),
No. 1, 25–42.
On page 42, Emch describes the above method of rotating a
hypercube’s 8 facets (i.e., three-dimensional cubes) to count
rotational symmetries —
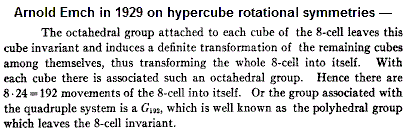
See also Diamond Theory in 1937.
Also on p. 42, Emch mentions work of Carmichael on a
Steiner system with the Mathieu group M11 as automorphism
group, and poses the problem of finding such systems and
groups that are larger. This may have inspired the 1931
discovery by Carmichael of the Steiner system S(5, 8, 24),
which has as automorphisms the Mathieu group M24 .
Comments Off on Rotating the Facets
Tuesday, October 22, 2024
Depth
James Hillman
EGALITARIAN TYPOLOGIES
VERSUS THE PERCEPTION OF THE UNIQUE
“The kind of movement Olson urges is an inward deepening of the image,
an in-sighting of the superimposed levels of significance within it.
This is the very mode that Jung suggested for grasping dreams —
not as a sequence in time, but as revolving around a nodal complex.”
See as well "True Grids" (Log24, August 9, 2018).
The Wikipedia "Truchet tiles" article shown above illustrates Hillman's
"superimposed levels of significance."
For more levels, see Wang on Gõdel and other posts tagged For Stella Maris.
Comments Off on Depth
Sunday, September 22, 2024
Raiders of the Lost Box
The "Facets" tag in this morning's previous post,
"The Portable Divinity Box," suggests a look at
Box759.
Comments Off on Raiders of the Lost Box
Saturday, May 11, 2024
The Thesis Advisor
"And so I had an idea, which turned out to be
related to that question, and so it was a little theorem
that I proved and I showed it to my thesis advisor, and
then he said, 'Oh, that could maybe help with this question.'
And he told me the question. And I said, 'Oh that's right,
maybe it could.' But he said, 'But don't work on that.'
He says, 'Borel has worked on that, Singer has worked
on that, a lot of people have tried to do this without success.'
But that has just got me fired up. And so I solved that problem…."
— The late James H. Simons, on his thesis advisor Bertram Kostant.
The thesis advisor reportedly died on Groundhog Day, 2017.
See as well, in this journal, Facets for Snorri.
Comments Off on The Thesis Advisor
Saturday, March 2, 2024
Song by Copilot: Prompt and Response*
Prompt: Compose a song about the Cullinane diamond theorem.
Response by Microsoft Copilot on Saturday afternoon,
March 2, 2024:
Sure, here’s a song inspired by the Cullinane diamond theorem:
(Verse 1)
In the realm where logic reigns and numbers intertwine,
Lies a theorem, diamond-bright, its facets all align.
Cullinane's the name it bears, a gem in math's grand crown,
In the grid of sixteen squares, its truths are written down.
(Chorus)
Oh, Cullinane, your diamond shines with symmetry so fine,
In the field of finite forms, your theorem does define.
A structure pure, a pattern clear, for all of us to see,
In the heart of mathematics, lies your geometry.
(Verse 2)
Rows and columns interplay in a dance so grand,
Quadrants align in perfect time, like notes in a band.
Through the chaos and the whirl, an order does descend,
In the world of abstract thought, your theorem is a friend.
(Chorus)
Oh, Cullinane, your diamond theorem, a beacon in the night,
Guiding us through complex realms, with its brilliant light.
In the vast expanse of knowledge, where truth and beauty blend,
Your theorem stands, a testament, a guide until the end.
(Outro)
So here's to you, Cullinane, and your diamond theorem's might,
In the endless quest for truth, you've brought us greater light.
In the language of the universe, where numbers never lie,
Your diamond theorem's shining bright, beneath the math's clear sky.
Added note from Copilot:
Please note that this is a creative interpretation and may not accurately reflect
the mathematical concepts of the Cullinane diamond theorem. 😊
Added note from Cullinane:
* The previous post may or may not display a prompt response to a Zen koan.
Comments Off on Song by Copilot: Prompt and Response*
Saturday, June 24, 2023
For the ACME Corporation: “e” is for “einheit ”
Comments Off on For the ACME Corporation: “e” is for “einheit ”
Saturday, November 12, 2022
Inside a White Cube
For the late Brian O'Doherty, from posts now tagged "Pless Birthday 2022" —
This post was suggested by an obituary of O'Doherty and by
"The Life and Work of Vera Stepen Pless" in
Notices of the American Mathematical Society , December 2022.
Comments Off on Inside a White Cube
Thursday, November 25, 2021
Fire
"Faced with a larger surface than he had ever provided with facets,
in his desperation he had divided the diamond with imaginary lines,
treating each section as if it were a single small stone and arranging
the clusters of facets so they would interact with one another, as if they
were single facets in a smaller stone. What if the final result lacked fire?"
— Novel* by Noah Gordon, who reportedly died on Monday, Nov. 22.
*
German translation of an April 1, 1979, novel.
Comments Off on Fire
Sunday, January 31, 2021
Language Game for Nabokov
A recent search for one Georgina Edwards, writer on Wittgenstein
and Hesse, yielded a different G.E. who is perhaps better suited to
illustrate the oeuvres of Nabokov and of Stephen King —
https://www.instagram.com/p/Bzf1uXelQ0p/.
This post is in memory of a fashion designer —
— and of a Russian philologist:
Comments Off on Language Game for Nabokov
Wednesday, January 27, 2021
Adoration of the Cube . . .
Related vocabulary —
See as well the word facet in this journal.
Analogously, one might write . . .
A Hiroshima cube consists of 6 faces ,
each with 4 squares called facets ,
for a total of 24 facets. . . ."
(See Aitchison's Octads , a post of Feb. 19, 2020.)
Click image to enlarge. Background: Posts tagged 'Aitchison.'"
Comments Off on Adoration of the Cube . . .
Thursday, August 20, 2020
“One More Reality Show”
Comments Off on “One More Reality Show”
Wednesday, August 5, 2020
Tuesday, January 28, 2020
Very Stable Kool-Aid
Two of the thumbnail previews
from yesterday's 1 AM post …
Further down in the "6 Prescott St." post, the link 5 Divinity Avenue
leads to …
|
A Letter from Timothy Leary, Ph.D., July 17, 1961
Harvard University July 17, 1961
Dr. Thomas S. Szasz Dear Dr. Szasz: Your book arrived several days ago. I've spent eight hours on it and realize the task (and joy) of reading it has just begun. The Myth of Mental Illness is the most important book in the history of psychiatry. I know it is rash and premature to make this earlier judgment. I reserve the right later to revise and perhaps suggest it is the most important book published in the twentieth century. It is great in so many ways–scholarship, clinical insight, political savvy, common sense, historical sweep, human concern– and most of all for its compassionate, shattering honesty. . . . . |
The small Morton Prince House in the above letter might, according to
the above-quoted remarks by Corinna S. Rohse, be called a "jewel box."
Harvard moved it in 1978 from Divinity Avenue to its current location at
6 Prescott Street.
Related "jewel box" material for those who
prefer narrative to mathematics —
"In The Electric Kool-Aid Acid Test , Tom Wolfe writes about encountering
'a young psychologist,' 'Clifton Fadiman’s nephew, it turned out,' in the
waiting room of the San Mateo County jail. Fadiman and his wife were
'happily stuffing three I-Ching coins into some interminable dense volume*
of Oriental mysticism' that they planned to give Ken Kesey, the Prankster-
in-Chief whom the FBI had just nabbed after eight months on the lam.
Wolfe had been granted an interview with Kesey, and they wanted him to
tell their friend about the hidden coins. During this difficult time, they
explained, Kesey needed oracular advice."
— Tim Doody in The Morning News web 'zine on July 26, 2012**
Oracular advice related to yesterday evening's
"jewel box" post …
A 4-dimensional hypercube H (a tesseract ) has 24 square
2-dimensional faces. In its incarnation as a Galois tesseract
(a 4×4 square array of points for which the appropriate transformations
are those of the affine 4-space over the finite (i.e., Galois) two-element
field GF(2)), the 24 faces transform into 140 4-point "facets." The Galois
version of H has a group of 322,560 automorphisms. Therefore, by the
orbit-stabilizer theorem, each of the 140 facets of the Galois version has
a stabilizer group of 2,304 affine transformations.
Similar remarks apply to the I Ching In its incarnation as
a Galois hexaract , for which the symmetry group — the group of
affine transformations of the 6-dimensional affine space over GF(2) —
has not 322,560 elements, but rather 1,290,157,424,640.
* The volume Wolfe mentions was, according to Fadiman, the I Ching.
** See also this journal on that date — July 26, 2012.
Comments Off on Very Stable Kool-Aid
Friday, March 22, 2019
Charles Jencks’s Grand Unified Theory
"The stars and galaxies seem static, eternal, or moving slowly
in deterministic patterns, becoming the background stage
on which we move. But if we could speed up the sequence,
we would see how dramatic and unpredictable this background
really is — an actor, director, script and stage all at once.
Moreover, it is a unified universe, a single unfolding event
of which we are an embedded part, a narrative of highly
dangerous and fine-tuned events, something more like
a detective thriller with many crimes and last-minute escapes
than the impersonal account of astronomy textbooks.
We are only just beginning to decipher the plot and figure out
the Cosmic Code, as Heinz Pagels puts it."
— Charles Jencks, The Architecture of the Jumping Universe :
A Polemic (How Complexity Science is Changing Architecture
and Culture), Academy Editions, 1995, rev. ed. 1997
"A Grand Unified Theory (GUT) is a model in particle physics…."
— Wikipedia
"Under the GUT symmetry operation these field components
transform into one another. The reason quantum particles
appear to have different properties in nature is that the unifying
symmetry is broken. The various gluons, quarks and leptons
are analogous to the facets of a cut diamond, which appear
differently according to the way the diamond is held but in
fact are all manifestations of the same underlying object."
— Heinz Pagels, Perfect Symmetry , Bantam paperback, 1986, p. 284
See also the recent post Multifaceted Narrative.
Comments Off on Charles Jencks’s Grand Unified Theory
Saturday, March 16, 2019
Multifaceted Narrative
"Here, modernism is defined as an autonomous body
of ideas, having little or no outward reference, placing
considerable emphasis on formal aspects of the work
and maintaining a complicated—indeed, anxious—
rather than a naïve relationship with the day-to-day
world, which is the de facto view of a coherent group
of people, such as a professional or discipline-based
group that has a high sense of the seriousness and
value of what it is trying to achieve. This brisk definition…."
— Jeremy Gray, Plato's Ghost: The Modernist
Transformation of Mathematics , Princeton, 2008
"Even as the dominant modernist narrative was being written,
there were art historians who recognized that it was inaccurate.
The narrative was too focused on France . . . . Nor was it
correct to build the narrative so exclusively around formalism;
modernism was far messier, far more multifaceted than that."
— Jane Kallir, https://www.tabletmag.com/
jewish-arts-and-culture/visual-art-and-design/
269564/the-end-of-middle-class-art,
quoted here on the above date — Sept. 11, 2018.
From some related Log24 posts —
Comments Off on Multifaceted Narrative
Sunday, February 24, 2019
Lost in Rashomon
" What this research implies is that we are not just hearing different 'stories'
about the electron, one of which may be true. Rather, there is one true story,
but it has many facets, seemingly in contradiction, just like in 'Rashomon.' "
— Edward Frenkel on "the Rashomon effect"
"Program or be programmed." — The Rushkoff Maxim
Comments Off on Lost in Rashomon
Thursday, February 21, 2019
Frenkel on “the Rashomon Effect”
Earlier in Frenkel's above opinion piece —
"What this research implies is that we are not just hearing
different 'stories' about the electron, one of which may be
true. Rather, there is one true story, but it has many facets,
seemingly in contradiction, just like in 'Rashomon.'
There is really no escape from the mysterious — some
might say, mystical — nature of the quantum world."
See also a recent New Yorker version of the fashionable cocktail-party
phrase "the Rashomon effect."
For a different approach to the dictum "there is one true story, but
it has many facets," see . . .
"Read something that means something."
— New Yorker motto
Comments Off on Frenkel on “the Rashomon Effect”
Monday, May 7, 2018
Glitter Ball for Cannes
In memory of a French film publicist who worked with Clint Eastwood
in 1971 on the release of "The Beguiled" —
From a New York Times graphic review dated Sept. 16, 2016 —
It's Chapter 1 of George Eliot's "Middlemarch."
Dorothea Brooke, young and brilliant, filled with passion
no one needs, is beguiled by some gemstones . . . .
The characters, moving through the book,
glitter as they turn their different facets toward us . . . .
Cf. a glitter-ball-like image in today's New York Times philosophy column
"The Stone" — a column named for the legendary philosophers' stone.
The publicist, Pierre Rissient, reportedly died early Sunday.
See as well Duelle in this journal.
Comments Off on Glitter Ball for Cannes
Tuesday, December 26, 2017
Raiders of the Lost Stone
Two Students of Structure
A comment on Sean Kelly's Christmas Morning column on "aliveness"
in the New York Times philosophy series The Stone —
Diana Senechal's 1999 doctoral thesis at Yale was titled
"Diabolical Structures in the Poetics of Nikolai Gogol."
Her mother, Marjorie Senechal, has written extensively on symmetry
and served as editor-in-chief of The Mathematical Intelligencer .
From a 2013 memoir by Marjorie Senechal —
|
"While I was in Holland my enterprising student assistant at Smith had found, in Soviet Physics – Crystallography, an article by N. N. Sheftal' on tetrahedral penetration twins. She gave it to me on my return. It was just what I was looking for. The twins Sheftal' described had evidently begun as (111) contact twins, with the two crystallites rotated 60o with respect to one another. As they grew, he suggested, each crystal overgrew the edges of the other and proceeded to spread across the adjacent facet. When all was said and done, they looked like they'd grown through each other, but the reality was over-and-around. Brilliant! I thought. Could I apply this to cubes? No, evidently not. Cube facets are all (100) planes. But . . . these crystals might not have been cubes in their earliest stages, when twinning occurred! I wrote a paper on "The mechanism of certain growth twins of the penetration type" and sent it to Martin Buerger, editor of Neues Jarbuch für Mineralogie. This was before the Wrinch symposium; I had never met him. Buerger rejected it by return mail, mostly on the grounds that I hadn't quoted any of Buerger's many papers on twinning. And so I learned about turf wars in twin domains. In fact I hadn't read his papers but I quickly did. I added a reference to one of them, the paper was published, and we became friends.[5]
After reading Professor Sheftal's paper I wrote to him in Moscow; a warm and encouraging correspondence ensued, and we wrote a paper together long distance.[6] Then I heard about the scientific exchanges between the Academies of Science of the USSR and USA. I applied to spend a year at the Shubnikov Institute for Crystallography, where Sheftal' worked. I would, I proposed, study crystal growth with him, and color symmetry with Koptsik. To my delight, I was accepted for an 11-month stay. Of course the children, now 11 and 14, would come too and attend Russian schools and learn Russian; they'd managed in Holland, hadn't they? Diana, my older daughter, was as delighted as I was. We had gone to Holland on a Russian boat, and she had fallen in love with the language. (Today she holds a Ph.D. in Slavic Languages and Literature from Yale.) . . . . |
Philosophy professors and those whose only interest in mathematics
is as a path to the occult may consult the Log24 posts tagged Tsimtsum.
Comments Off on Raiders of the Lost Stone
Friday, February 17, 2017
Kostant Is Dead
"Bertram Kostant, professor emeritus of mathematics at MIT,
died at the Hebrew Senior Rehabilitation Center in Roslindale,
Massachusetts, on Thursday, Feb. 2, at the age of 88."
— MIT News, story dated Feb. 16, 2017
See also a search for Kostant in this journal.
Regarding the discussions of symmetries and "facets" found in
that search —
Kostant:
“A word about E(8). In my opinion, and shared by others,
E(8) is the most magnificent ‘object’ in all of mathematics.
It is like a diamond with thousands of facets. Each facet
offering a different view of its unbelievable intricate internal
structure.”
Cullinane:
In the Steiner system S(5, 8, 24) each octad might be
regarded as a "facet," with the order of the system's
automorphism group, the Mathieu group M24 , obtained
by multiplying the number of such facets, 759, by the
order of the octad stabilizer group, 322,560.
Analogously …
Comments Off on Kostant Is Dead
Tuesday, December 31, 2013
Christmas Ornaments
Continued from December 25—
A link from Sunday afternoon to Nov. 26, 2012,
suggests a review of one of the above structures.
The Dreaming Jewels cover at left is taken from a review
by Jo Walton at Tor.com—
"This is a book that it’s clearly been difficult
for publishers to market. The covers have been
generally pretty awful, and also very different.
I own a 1975 Corgi SF Collectors Library
paperback that I bought new for 40p in the later
seventies. It’s purple, and it has a slightly grainy
cover, and it matches my editions of The Menace
From Earth and A Canticle for Leibowitz .
(Dear old Corgi SF Collectors Editions with their
very seventies fonts! How I imprinted on them at
an early age!) I mention this, however, because
the (uncredited) illustration actually represents and
illustrates the book much better than any of the other
cover pictures I’ve seen. It shows a hexagon with an
attempt at facets, a man, a woman, hands, a snake,
and stars, all in shades of green. It isn’t attractive,
but it wouldn’t put off people who’d enjoy what’s inside
either."
The "hexagon with an attempt at facets" is actually
an icosahedron, as the above diagram shows.
(The geometric part of the diagram is from a Euclid webpage.)
For Plato's dream about these jewels, see his Timaeus.
Comments Off on Christmas Ornaments
Wednesday, October 10, 2012
Melancholia, Depression, Ambiguity
Occurrences of the phrase "magic square" in Lowe-Porter's translation of the Thomas Mann novel Doctor Faustus—
"On the wall above the piano was an arithmetical diagram fastened with drawing-pins, something he had found in a second-hand shop: a so-called magic square, such as appears also in Dürer's Melancolia , along with the hour-glass, the circle, the scale, the polyhedron, and other symbols. Here as there, the figure was divided into sixteen Arabic-numbered fields, in such a way that number one was in the right-hand lower corner, sixteen in the upper left; and the magic, or the oddity, simply consisted in the fact that the sum of these numerals, however you added them, straight down, crosswise, or diagonally, always came to thirty-four. What the principle was upon which this magic uniformity rested I never made out, but by virtue of the prominent place Adrian had given it over the piano, it always attracted the eye, and I believe I never visited his room without giving a quick glance, slanting up or straight down and testing once more the invariable, incredible result."
….
"Adrian kept without changing during the whole four and a half years he spent in Leipzig his two-room quarters in Peterstrasse near the Collegium Beatae Virginis, where he had again pinned the magic square above his cottage piano."
….
" 'The decisive factor is that every note, without exception, has significance and function according to its place in the basic series or its derivatives. That would guarantee what I call the indifference to harmony and melody.'
'A magic square,' I said. 'But do you hope to have people hear all that?' "
….
" 'Extraordinarily Dürerish. You love it. First "how will I shiver after the sun"; and then the houre-glasse of the Melancolia . Is the magic square coming too?' "
….
"Here I will remind the reader of a conversation I had with Adrian on a long-ago day, the day of his sister's wedding at Buchel, as we walked round the Cow Trough. He developed for me— under pressure of a headache— his idea of the 'strict style,' derived from the way in which, as in the lied 'O lieb Madel, wie schlecht bist du ' melody and harmony are determined by the permutation of a fundamental five-note motif, the symbolic letters h, e, a, e, e-flat. He showed me the 'magic square' of a style of technique which yet developed the extreme of variety out of identical material and in which there is no longer anything unthematic, anything that could not prove itself to be a variation of an ever constant element. This style, this technique, he said, admitted no note, not one, which did not fulfil its thematic function in the whole structure— there was no longer any free note."
Review of related material—
Last night's midnight post (disambiguation), the followup 1 AM post (ambiguation), today's noon post (ambiguity), and Dürer in this journal.
The tesseracts of the noon post are related to the Dürer magic square by a well-known adjacency property.
"… the once stable 'father's depression' has been transmuted into a shifting reality that shimmered in a multiplicity of facets."
— Haim Omer, Tel-Aviv University, on Milanese ambiguation therapy,
p. 321 in "Three Styles of Constructive Therapy,"
Constructive Therapies, Vol. 2 , pp. 319-333,
ed. by Michael F. Hoyt (Guilford Press paperback, 1998)
Comments Off on Melancholia, Depression, Ambiguity
Ambiguation
Wikipedia disambiguation page—
"When you come to a fork in the road…"
For another "shifting reality that shimmered
in a multiplicity of facets," see The Diamond Theorem.
Comments Off on Ambiguation
Wednesday, August 24, 2011
Conjure
In the catacomb of my mind
Where the dead endure—a kingdom
I conjure by love to rise
— Samuel Menashe, as quoted by
Stephen Spender in a review of four
different poets, "The Last Ditch,"
The New York Review of Books , July 22, 1971
"…the ghost reveals that the beggar
is in fact a sorcerer, a necromancer
who is preparing the mandala in order
to achieve an evil end. The ascetic
intends to bind the ghost to the corpse,
place it in the center of the circle,
and worship it as a deity."
— The King and the Corpse (from synopsis in
"How Many Facets Can a Non-Existent Jewel Have?")
Menashe died on Monday, August 22, 2011.
Related material by and for two other poets
who also died on Monday:
- By Jerry Leiber— "Love Potion #9"
-
For Nick Ashford— Nicole Kidman in
Sermon (from Jan. 9) and
Conjure Wife, a 1943 tale by Fritz Leiber
See also an excerpt from Kerouac I cached on Monday, and
Men ask the way to Cold Mountain
Cold Mountain: there's no through trail .
Comments Off on Conjure
Sunday, July 31, 2011
Short Stories
An Amazon.com reader review of Algis Budrys's Writing to the Point: A Complete Guide to Selling Fiction—
"Mr. Budrys claims to have the secret to writing fiction that will sell. His secret is very useful but short enough to include here:
Beginning: Must consist of introducing a character, in a particular context, with a problem. And if there are important yet unique/unusual aspects of the character that will be revealed later in the story they must be foreshadowed in the beginning.
Middle: Must involve the character attempting to solve the problem and encountering unexpected failure. During this attempt he begins to learn more about the problem and himself. The character must undergo stress which causes hitherto concealed facets of him to be revealed-that must fit in. The character must try to overcome the problem a total of 3 times on a rising scale of effort, commitment, and depth of knowledge of the problem and one's self. At the last possible moment, with maximum effort and staking everything, he achieves victory. This must be done by wagering everything in a do-or-die situation. Conversely the villain, coming closer to his goal experiences defeat snatched from the jaws of victory-because of some flaw in character.
End: Validation and foreclosure by someone who has no other vested interest in the story. They step forward and say 'He's dead, Jim' or 'Who was that masked man?' This serves to close the story in the reader's mind."
Here are two parallel stories suggested by yesterday's New York Lottery numbers:
|
Evening: 003 and 8997— From an author born on 8/9/97: For the 003, see
7/11. |
Midday: 004 and 1931— From an author born on 1/9/31:
For the 004, see the ideogram
See also the day of the author's |
Happy Feast of St. Ignatius of Loyola.
Comments Off on Short Stories
Tuesday, May 17, 2011
Anomalies
Perfect Symmetry (Oct. 2008) and Perfect Symmetry single (Dec. 2008)—
Related science…
Heinz Pagels in Perfect Symmetry (paperback, 1985), p. xvii—
The penultimate chapter of this third part of the book—
as far as speculation is concerned— describes some
recent mathematical models for the very origin of the
universe—how the fabric of space, time and matter can
be created out of absolutely nothing. What could have more
perfect symmetry than absolute nothingness? For the first
time in history, scientists have constructed mathematical
models that account for the very creation of the universe
out of nothing.
On Grand Unified Theories (GUT's) of physics (ibid., 284)—
In spite of the fact that GUTs leave deep puzzles unsolved,
they have gone a long way toward unifying the various
quantum particles. For example, many people are disturbed
by the large numbers of gluons, quarks and leptons. Part of
the appeal of the GUT idea is that this proliferation of
quantum particles is really superficial and that all the gluons
as well at the quarks and leptons may be simply viewed as
components of a few fundamental unifying fields. Under the
GUT symmetry operation these field components transform
into one another. The reason quantum particles appear to
have different properties in nature is that the unifying
symmetry is broken. The various gluons, quarks and leptons
are analogous to the facets of a cut diamond, which appear
differently according to the way the diamond is held but in
fact are all manifestations of the same underlying object.
Related art— Puzzle and Particles…
The Diamond 16 Puzzle (compare with Keane art above)—
—and The Standard Model of particle theory—
The fact that both the puzzle and the particles appear
within a 4×4 array is of course completely coincidental.
See also a more literary approach— "The Still Point and the Wheel"—
"Anomalies must be expected along the conceptual frontier between the temporal and the eternal."
— The Death of Adam , by Marilynne Robinson, Houghton Mifflin, 1998, essay on Marguerite de Navarre
Comments Off on Anomalies
Monday, April 25, 2011
Poetry and Physics
One approach to the storied philosophers' stone, that of Jim Dodge in Stone Junction , was sketched in yesterday's Easter post. Dodge described a mystical "spherical diamond." The symmetries of the sphere form what is called in mathematics a Lie group . The "spherical" of Dodge therefore suggests a review of the Lie group E8 in Garrett Lisi's poetic theory of everything.
A check of the Wikipedia article on Lisi's theory yields…
Diamond and E8 at Wikipedia
Related material — E8 as "a diamond with thousands of facets"—
Also from the New Yorker article—
“There’s a dream that underlying the physical universe is some beautiful mathematical structure, and that the job of physics is to discover that,” Smolin told me later. “The dream is in bad shape,” he added. “And it’s a dream that most of us are like recovering alcoholics from.” Lisi’s talk, he said, “was like being offered a drink.”
A simpler theory of everything was offered by Plato. See, in the Timaeus , the Platonic solids—
Figure from this journal on August 19th, 2008.
See also July 19th, 2008.
“It’s all in Plato, all in Plato:
bless me, what do they
teach them at these schools!”
— C. S. Lewis
Comments Off on Poetry and Physics
Tuesday, February 17, 2009
Tuesday February 17, 2009
Diamond-Faceted:
Transformations
of the Rock
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
A mathematical version of
this poetic concept appears
in a rather cryptic note
from 1981 written with
Stevens's poem in mind:
For some explanation of the
groups of 8 and 24
motions referred to in the note,
see an earlier note from 1981.
For the Perlis "diamond facets,"
see the Diamond 16 Puzzle.
For a much larger group
of motions, see
Solomon's Cube.
As for "the mind itself"
and "possibilities for
human thought," see
Geometry of the I Ching.
Comments Off on Tuesday February 17, 2009
Tuesday, August 19, 2008
Tuesday August 19, 2008
Three Times
|
"Credences of Summer," VII,
by Wallace Stevens, from
"Three times the concentred |
Stevens does not say what object he is discussing.
One possibility —
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in a recent New Yorker:
"A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Another possibility —
A more modest object —
the 4×4 square.
Update of Aug. 20-21 —
Symmetries and Facets
Kostant's poetic comparison might be applied also to this object.
The natural rearrangements (symmetries) of the 4×4 array might also be described poetically as "thousands of facets, each facet offering a different view of… internal structure."
More precisely, there are 322,560 natural rearrangements– which a poet might call facets*— of the array, each offering a different view of the array's internal structure– encoded as a unique ordered pair of symmetric graphic designs. The symmetry of the array's internal structure is reflected in the symmetry of the graphic designs. For examples, see the Diamond 16 Puzzle.
For an instance of Stevens's "three times" process, see the three parts of the 2004 web page Ideas and Art.
* For the metaphor of rearrangements as facets, note that each symmetry (rearrangement) of a Platonic solid corresponds to a rotated facet: the number of symmetries equals the number of facets times the number of rotations (edges) of each facet–
The metaphor of rearrangements as facets breaks down, however, when we try to use it to compute, as above with the Platonic solids, the number of natural rearrangements, or symmetries, of the 4×4 array. Actually, the true analogy is between the 16 unit squares of the 4×4 array, regarded as the 16 points of a finite 4-space (which has finitely many symmetries), and the infinitely many points of Euclidean 4-space (which has infinitely many symmetries).
If Greek geometers had started with a finite space (as in The Eightfold Cube), the history of mathematics might have dramatically illustrated Halmos's saying (Aug. 16) that
"The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question."
The Greeks, of course, answered the infinite questions first– at least for Euclidean space. Halmos was concerned with more general modern infinite spaces (such as Hilbert space) where the intuition to be gained from finite questions is still of value.
Comments Off on Tuesday August 19, 2008
Saturday, July 19, 2008
Saturday July 19, 2008
Hard Core
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in this week's New Yorker:
"A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Hermann Weyl on the hard core of objectivity:
"Perhaps the philosophically most relevant feature of modern science is the emergence of abstract symbolic structures as the hard core of objectivity behind– as Eddington puts it– the colorful tale of the subjective storyteller mind." (Philosophy of Mathematics and Natural Science, Princeton, 1949, p. 237)
Steven H. Cullinane on the symmetries of a 4×4 array of points:
|
A Structure-Endowed Entity
"A guiding principle in modern mathematics is this lesson: Whenever you have to do with a structure-endowed entity S, try to determine its group of automorphisms, the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of S in this way." — Hermann Weyl in Symmetry Let us apply Weyl's lesson to the following "structure-endowed entity."
What is the order of the resulting group of automorphisms? |
The above group of
automorphisms plays
a role in what Weyl,
following Eddington,
called a "colorful tale"–
This puzzle shows
that the 4×4 array can
also be viewed in
thousands of ways.
"You can make 322,560
pairs of patterns. Each
pair pictures a different
symmetry of the underlying
16-point space."
— Steven H. Cullinane,
July 17, 2008
For other parts of the tale,
see Ashay Dharwadker,
the Four-Color Theorem,
and Usenet Postings.
Comments Off on Saturday July 19, 2008