Minimalism
"It's become our form of modern classicism."
— Nancy Spector in
the New York Times of April 23, 2004
Part I: Aesthetics
In honor of the current Guggenheim exhibition, "Singular Forms" — A quotation from the Guggenheim's own website:
"Minimalism refers to painting or sculpture
-
made with an extreme economy of means
-
and reduced to the essentials of geometric abstraction….
-
Minimalist art is generally characterized by precise, hard-edged, unitary geometric forms….
-
mathematically regular compositions, often based on a grid….
-
the reduction to pure self-referential form, emptied of all external references….
-
In Minimal art what is important is the phenomenological basis of the viewer’s experience, how he or she perceives the internal relationships among the parts of the work and of the parts to the whole….
-
The repetition of forms in Minimalist sculpture serves to emphasize the subtle differences in the perception of those forms in space and time as the spectator’s viewpoint shifts in time and space."
Discuss these seven points
in relation to the following:

Form,
by S. H. Cullinane
Logos and Logic
Mark Rothko's reference
to geometry as a "swamp"
and his talk of "the idea" in art
Michael Kimmelman's
remarks on ideas in art
Notes on ideas and art
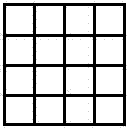
Geometry
of the 4×4 square
The Grid of Time
ART WARS:
Judgment Day
(2003, 10/07)
Part II: Theology
Today's previous entry, "Skylark," concluded with an invocation of the Lord. Of course, the Lord one expects may not be the Lord that appears.
John Barth on minimalism:
"… the idea that, in art at least, less is more.
It is an idea surely as old, as enduringly attractive and as ubiquitous as its opposite. In the beginning was the Word: only later came the Bible, not to mention the three-decker Victorian novel. The oracle at Delphi did not say, 'Exhaustive analysis and comprehension of one's own psyche may be prerequisite to an understanding of one's behavior and of the world at large'; it said, 'Know thyself.' Such inherently minimalist genres as oracles (from the Delphic shrine of Apollo to the modern fortune cookie), proverbs, maxims, aphorisms, epigrams, pensees, mottoes, slogans and quips are popular in every human century and culture–especially in oral cultures and subcultures, where mnemonic staying power has high priority–and many specimens of them are self-reflexive or self-demonstrative: minimalism about minimalism. 'Brevity is the soul of wit.' "
Another form of the oracle at Delphi, in minimalist prose that might make Hemingway proud:
"He would think about Bert. Bert was an interesting man. Bert had said something about the way a gambler wants to lose. That did not make sense. Anyway, he did not want to think about it. It was dark now, but the air was still hot. He realized that he was sweating, forced himself to slow down the walking. Some children were playing a game with a ball, in the street, hitting it against the side of a building. He wanted to see Sarah.
When he came in, she was reading a book, a tumbler of dark whiskey beside her on the end table. She did not seem to see him and he sat down before he spoke, looking at her and, at first, hardly seeing her. The room was hot; she had opened the windows, but the air was still. The street noises from outside seemed almost to be in the room with them, as if the shifting of gears were being done in the closet, the children playing in the bathroom. The only light in the room was from the lamp over the couch where she was reading.
He looked at her face. She was very drunk. Her eyes were swollen, pink at the corners. 'What's the book,' he said, trying to make his voice conversational. But it sounded loud in the room, and hard.
She blinked up at him, smiled sleepily, and said nothing.
'What's the book?' His voice had an edge now.
'Oh,' she said. 'It's Kierkegaard. Soren Kierkegaard.' She pushed her legs out straight on the couch, stretching her feet. Her skirt fell back a few inches from her knees. He looked away.
'What's that?' he said.
'Well, I don't exactly know, myself." Her voice was soft and thick.
He turned his face away from her again, not knowing what he was angry with. 'What does that mean, you don't know, yourself?'
She blinked at him. 'It means, Eddie, that I don't exactly know what the book is about. Somebody told me to read it once, and that's what I'm doing. Reading it.'
He looked at her, tried to grin at her — the old, meaningless, automatic grin, the grin that made everbody like him — but he could not. 'That's great,' he said, and it came out with more irritation than he had intended.
She closed the book, tucked it beside her on the couch. She folded her arms around her, hugging herself, smiling at him. 'I guess this isn't your night, Eddie. Why don't we have a drink?'
'No.' He did not like that, did not want her being nice to him, forgiving. Nor did he want a drink.
Her smile, her drunk, amused smile, did not change. 'Then let's talk about something else,' she said. 'What about that case you have? What's in it?' Her voice was not prying, only friendly, 'Pencils?'
'That's it,' he said. 'Pencils.'
She raised her eyebrows slightly. Her voice seemed thick. 'What's in it, Eddie?'
'Figure it out yourself.' He tossed the case on the couch."
— Walter Tevis, The Hustler, 1959,
Chapter 11
See, too, the invocation of Apollo in
A Mass for Lucero, as well as
GENERAL AUDIENCE OF JOHN PAUL II
Wednesday 15 January 2003:
"The invocation of the Lord is relentless…."
and
JOURNAL ENTRY OF S. H. CULLINANE
Wednesday 15 January 2003:
Karl Cullinane —
"I will fear no evil, for I am the
meanest son of a bitch in the valley."
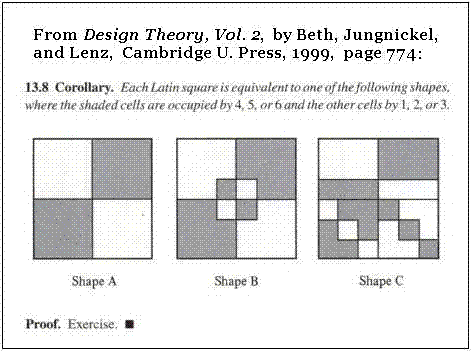















































































 22. Without using the Pythagorean Theorem prove that the hypotenuse of an isosceles right triangle will have the length
22. Without using the Pythagorean Theorem prove that the hypotenuse of an isosceles right triangle will have the length  if the equal legs have the length 1. Suggestion: Consider the similar triangles in Fig. 39.
if the equal legs have the length 1. Suggestion: Consider the similar triangles in Fig. 39.















































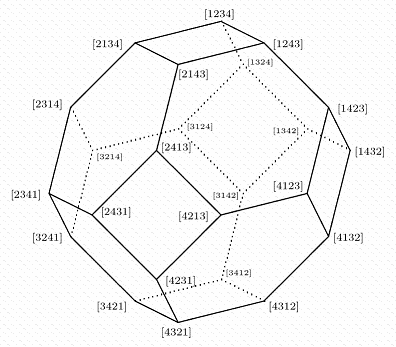
 lets us visualize the projective space PG(3,2) as well as the affine space AG(4,2). For
lets us visualize the projective space PG(3,2) as well as the affine space AG(4,2). For