Cornerstone
“In 1782, the Swiss mathematician Leonhard Euler posed a problem whose mathematical content at the time seemed about as much as that of a parlor puzzle. 178 years passed before a complete solution was found; not only did it inspire a wealth of mathematics, it is now a cornerstone of modern design theory.”
— Dean G. Hoffman, Auburn U.,
July 2001 Rutgers talk
Diagrams from Dieter Betten’s 1983 proof
of the nonexistence of two orthogonal
6×6 Latin squares (i.e., a proof
of Tarry’s 1900 theorem solving
Euler’s 1782 problem of the 36 officers):
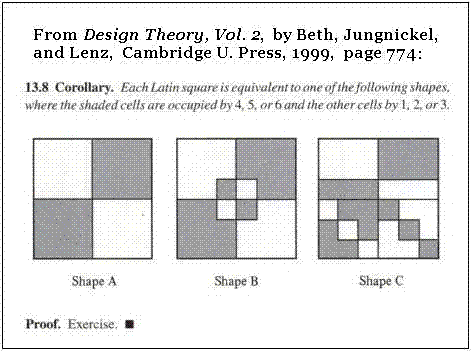
Compare with the partitions into
two 8-sets of the 4×4 Latin squares
discussed in my 1978 note (pdf).