Log24

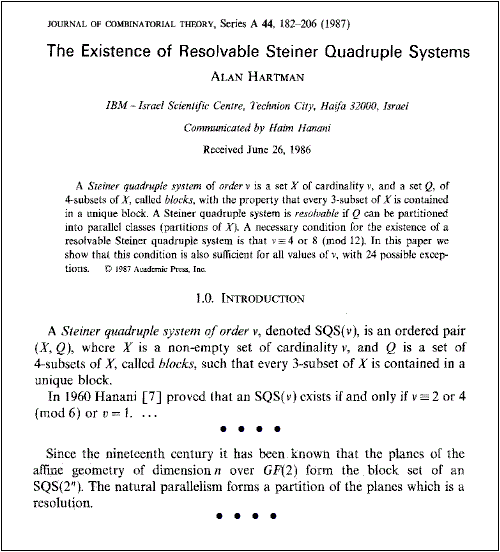
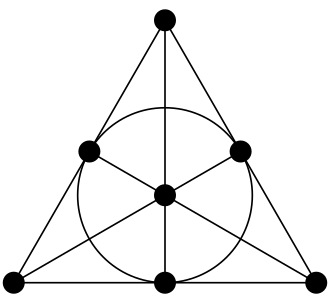
The Fano Plane —

"A balanced incomplete block design , or BIBD
with parameters b , v , r , k , and λ  is an arrangement
of b  blocks, taken from a set of v  objects (known
for historical reasons as varieties ), such that every
variety appears in exactly r  blocks, every block
contains exactly k  varieties, and every pair of
varieties appears together in exactly λ  blocks.
Such an arrangement is also called a
(b , v , r , k , λ ) design. Thus, (7, 3, 1) [the Fano plane] 
is a (7, 7, 3, 3, 1) design."

— Ezra Brown, "The Many Names of (7, 3, 1),"
     Mathematics Magazine , Vol. 75, No. 2, April 2002

W. Cherowitzo uses the notation (v, b, r, k, λ) instead of
Brown's (b , v , r , k , λ ).  Cherowitzo has described,
without mentioning its close connection with the
Fano-plane design, the following —

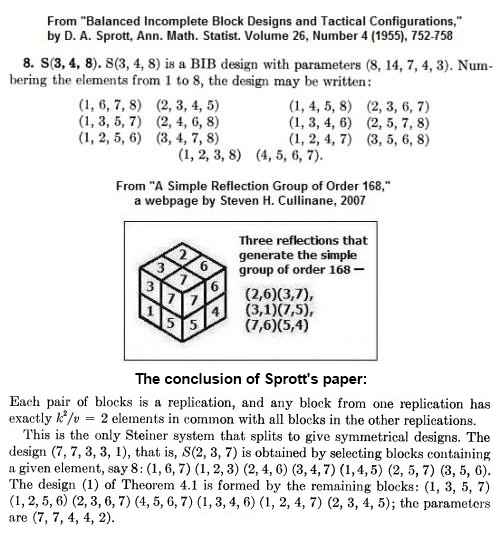
"the (8,14,7,4,3)-design on the set
X = {1,2,3,4,5,6,7,8} with blocks:

{1,3,7,8} {1,2,4,8} {2,3,5,8} {3,4,6,8} {4,5,7,8}
{1,5,6,8} {2,6,7,8} {1,2,3,6} {1,2,5,7} {1,3,4,5}
{1,4,6,7} {2,3,4,7} {2,4,5,6} {3,5,6,7}."

We can arrange these 14 blocks in complementary pairs:

{1,2,3,6} {4,5,7,8}
{1,2,4,8} {3,5,6,7}
{1,2,5,7} {3,4,6,8}
{1,3,4,5} {2,6,7,8}
{1,3,7,8} {2,4,5,6}
{1,4,6,7} {2,3,5,8}
{1,5,6,8} {2,3,4,7}.

These pairs correspond to the seven natural slicings
of the following eightfold cube —

Another representation of these seven natural slicings —

These seven slicings represent the seven
planes through the origin in the vector
3-space over the two-element field GF(2).  
In a standard construction, these seven 
planes  provide one way of defining the
seven projective lines  of the Fano plane.

A more colorful illustration —

Omega is a Greek letter, Ω , used in mathematics to denote
a set on which a group acts. 

For instance, the affine group AGL(3,2) is a group of 1,344
actions on the eight elements of the vector 3-space over the
two-element Galois field GF(2), or, if you prefer, on the Galois
field  Ω = GF(8).

Related fiction:  The Eight , by Katherine Neville.

Related non-fiction:  A remark by Werner Heisenberg
in this journal on Saturday, June 6, 2015, the eightfold cube ,
and the illustrations below —

Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—

Compare to an image of Vril muse Maria Orsitsch.

From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —

Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.

For some background, see (for instance) 
Conspiracy Theories and Secret Societies for Dummies .

(A prequel to Galois Field of Dreams)

The opening of Descartes' Dream ,
by Philip J. Davis and Reuben Hersh—

"The modern world,
our world of triumphant rationality,
began on November 10, 1619,
with a revelation and a nightmare."

For a revelation, see Battlefield Geometry.

For a nightmare, see Joyce's Nightmare.

Some later work of Descartes—

From "What Descartes knew of mathematics in 1628,"
by David Rabouin, CNRS-Univ. Paris Diderot,
Historia Mathematica , Volume 37, Issue 3,
Contexts, emergence and issues of Cartesian geometry,
August 2010, pages 428–459 —

Fig. 5. How to represent the difference between white, blue, and red
according to Rule XII [from Descartes, 1701, p. 34].

A translation —

The 4×4 array of Descartes appears also in the Battlefield Geometry posts.
For its relevance to Galois's  field of dreams, see (for instance) block designs.

An article from cnet.com tonight —

For Jobs, design is about more than aesthetics

By: Jay Greene   August 24, 2011 7:21 PM PDT

… The look of the iPhone, defined by its seamless pane of glass, its chrome border, its perfect symmetry, sparked an avalanche of copycat devices that tried to mimic its aesthetic.

Virtually all of them failed. And the reason is that Jobs understood that design wasn't merely about what a product looks like. In a 2003 interview with the New York Times' Rob Walker detailing the genesis of the iPod,  Jobs laid out his vision for product design.

''Most people make the mistake of thinking design is what it looks like,'' Jobs told Walker. "People think it's this veneer— that the designers are handed this box and told, 'Make it look good!' That's not what we think design is. It's not just what it looks like and feels like. Design is how it works.''

Related material: Open, Sesame Street  (Aug. 19) continues… Brought to you by the number 24—

"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."

— "Block Designs," by Andries E. Brouwer (Ch. 14 (pp. 693-746) of Handbook of Combinatorics , Vol. I, MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel, and László Lovász, Section 16 (p. 716))

It is well known that the seven (2² + 2 +1) points of the projective plane of order 2 correspond to 2-point subspaces (lines) of the linear 3-space over the two-element field Galois field GF(2), and may be therefore be visualized as 2-cube subsets of the 2×2×2 cube.

Similarly, recent posts* have noted that the thirteen (3² + 3 + 1) points of the projective plane of order 3 may be seen as 3-cube subsets in the 3×3×3 cube.

The twenty-one (4² + 4 +1) points of the (unique) projective plane of order 4 may also be visualized as subsets of a cube– in this case, the 4×4×4 cube. This visualization is somewhat more complicated than the 3×3×3 case, since the 4×4×4 cube has no central subcube, and each projective-plane point corresponds to four, not three, subcubes.

These three cubes, with 8, 27, and 64 subcubes, thus serve as geometric models in a straightforward way– first as models of finite linear spaces, hence as models for small Galois geometries derived from the linear spaces. (The cubes with 8 and 64 subcubes also serve in a less straightforward, and new, way as finite-geometry models– see The Eightfold Cube, Block Designs, and Solomon's Cube.)

A group of collineations** of the 21-point plane is one of two nonisomorphic simple groups of order 20,160. The other is the linear group acting on the linear 4-space over the two-element Galois field  GF(2). The 1899 paper establishing the nonisomorphism notes that "the expression Galois Field is perhaps not yet in general use."

Coordinates of the 4×4×4 cube's subcubes can, of course, be regarded as elements of the Galois field GF(64).

The preceding remarks were purely mathematical. The "dreams" of this post's title are not. See…

See also Geometry of the I Ching and a search in this journal for "Galois + Ching."

* February 27 and March 13

** G₂₀₁₆₀ in Mitchell 1910,  LF(3,2²) in Edge 1965

— Mitchell, Ulysses Grant, "Geometry and Collineation Groups
   of the Finite Projective Plane PG(2,2²),"
   Princeton Ph.D. dissertation (1910)

— Edge, W. L., "Some Implications of the Geometry of
   the 21-Point Plane," Math. Zeitschr. 87, 348-362 (1965)

“… Kirkman has established an incontestable claim to be regarded as the founding father of the theory of designs.”

— “T.P. Kirkman, Mathematician,” by N.L. Biggs, Bulletin of the London Mathematical Society, Volume 13, Number 2 (March 1981), 97-120.

This paper is now available online for $12.

For more about this subject, see Design Theory, by Beth, Jungnickel, and Lenz, Cambridge U. Press, Volume I (2nd ed., 1999, 1120 pages) and Volume II (2nd ed., 2000, 513 pages).

For an apparently unrelated subject with the same name, see Graphic Design Theory: Readings from the Field, by Helen Armstrong (Princeton Architectural Press, 2009).

For what the two subjects have in common, see Block Designs in Art and Mathematics.

Page 67 —

“… Bill and Violet were married. The wedding was held in the Bowery loft on June 16th, the same day Joyce’s Jewish Ulysses had wandered around Dublin. A few minutes before the exchange of vows, I noted that Violet’s last name, Blom, was only an o away from Bloom, and that meaningless link led me to reflect on Bill’s name, Wechsler, which carries the German root for change, changing, and making change. Blooming and changing, I thought.”

For Hustvedt’s discussion of Wechsler’s art– sculptured cubes, which she calls “tightly orchestrated semantic bombs” (p. 169)– see Log24, May 25, 2008.

Related 3×3 patterns appear
in “nine-patch” quilt blocks
and in the following–

Jared Tarbell at levitated.net, May 15, 2002: “The nine block is a common design pattern among quilters. Its construction methods and primitive building shapes are simple, yet produce millions of interesting variations. Figure A. Four 9 block patterns, arbitrarily assembled, show the grid composition of the block. Each block is composed of 9 squares, arranged in a 3 x 3 grid. Each square is composed of one of 16 primitive shapes. Shapes are arranged such that the block is radially symmetric. Color is modified and assigned arbitrarily to each new block. The basic building blocks of the nine block are limited to 16 unique geometric shapes. Each shape is allowed to rotate in 90 degree increments. Only 4 shapes are allowed in the center position to maintain radial symmetry. Figure B. The 16 possible shapes allowed for each grid space. The 4 shapes allowed in the center have bold numbers.”

   
Such designs become of mathematical interest when their size is increased slightly, from square arrays of nine blocks to square arrays of sixteen.  See Block Designs in Art and Mathematics.

(This entry was suggested by examples of 4×4 Identicons in use at Secret Blogging Seminar.)

Today’s birthday:
Tom Hanks, star of
“The Da Vinci Code”

“Kufi Blocks“*

by Ben Nicholson,
Illinois Institute of Technology

Part II:
Some Background

— Prime Curios

Classics Illustrated —

Click on picture for details.

(For some mathematics that is actually
from 1984, see Block Designs
and the 2005 followup
The Eightfold Cube.)

Mental Health Month:
Springtime for Wagner

“And now what you’ve all been waiting for…

 Wagner!“

— Colin Hay as Zac in the film “Cosi“

“When I sought those who would sympathize with my plans, I had only you, the friends of my particular art, my most personal work and creation, to turn to.”

— Wagner’s address at the ceremony for the laying of the foundation stone of the Festival Theater in Bayreuth, May 22 (Wagner’s birthday), 1872

“The new computer package DISCRETA which was created in Bayreuth is in the process of permanent development.”

— “A Computer Approach to the Enumeration of Block Designs Which Are Invariant With Respect to a Prescribed Permutation Group”

The above is a preprint from Dresden.

See, too, the work of Bierbrauer, who received his doctorate at Mainz in 1977 and taught at Heidelberg from 1977 to 1994.  Bierbrauer’s lecture notes give a particularly good background for the concepts involved in my Diamond Theory, in the tradition of Witt and Artin.  See

Introduction to Group Theory
and Applications,

by Jürgen Bierbrauer, 138 pp., PostScript

THE MONTESSORI METHOD: CHAPTER VI

HOW LESSONS SHOULD BE GIVEN

“Let all thy words be counted.”
Dante, Inf., canto X.

CONCISENESS, SIMPLICITY, OBJECTIVITY.

…Dante gives excellent advice to teachers when he says, “Let thy words be counted.” The more carefully we cut away useless words, the more perfect will become the lesson….

Another characteristic quality of the lesson… is its simplicity. It must be stripped of all that is not absolute truth…. The carefully chosen words must be the most simple it is possible to find, and must refer to the truth.

The third quality of the lesson is its objectivity. The lesson must be presented in such a way that the personality of the teacher shall disappear. There shall remain in evidence only the object to which she wishes to call the attention of the child….

Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale “block design” subtest.

Mathematicians mean something different by the phrase “block design.”

A University of London site on mathematical design theory includes a link to my diamond theory site, which discusses the mathematics of the sorts of visual designs that Professor Pope is demonstrating. For an introduction to the subject that is, I hope, concise, simple, and objective, see my diamond 16 puzzle.