Friday, April 8, 2011
Roberta Smith in today's New York Times —
"… the argument that painting may ultimately be about
little more than the communication of some quality of
light and space, however abstract or indirect."
— Review of "Rooms With a View" at the Met
Lowry —

Malcolm Lowry, author of Under the Volcano
Hollywood —

Related material —
"You've got to pick up every stitch…"
Comments Off on Windows
Tuesday, April 5, 2011
(A sequel to last night's "For Taylor")
On Joan Tewkesbury, who wrote the script for the 1975 film "Nashville"—
She urges writers to continue to generate new ideas
and new material. "Keep writing. The hardest thing
is to sell one script and not have another to follow it with."
One script— Yesterday's link titled "An Ordinary Evening in Tennessee"
Another— "A Point of Central Arrival"
Related material from last October—
"You've got to pick up every stitch…"
* A former governor of Tennessee who died at 80 yesterday in Nashville
Comments Off on For Ned*
Saturday, January 8, 2011
"Rosetta Stone" as a Metaphor
in Mathematical Narratives
For some backgound, see Mathematics and Narrative from 2005.
Yesterday's posts on mathematics and narrative discussed some properties
of the 3×3 grid (also known as the ninefold square ).
For some other properties, see (at the college-undergraduate, or MAA, level)–
Ezra Brown, 2001, "Magic Squares, Finite Planes, and Points of Inflection on Elliptic Curves."
His conclusion:
When you are done, you will be able to arrange the points into [a] 3×3 magic square,
which resembles the one in the book [5] I was reading on elliptic curves….
This result ties together threads from finite geometry, recreational mathematics,
combinatorics, calculus, algebra, and number theory. Quite a feat!
5. Viktor Prasolov and Yuri Solvyev, Elliptic Functions and Elliptic Integrals ,
American Mathematical Society, 1997.
Brown fails to give an important clue to the historical background of this topic —
the word Hessian . (See, however, this word in the book on elliptic functions that he cites.)
Investigation of this word yields a related essay at the graduate-student, or AMS, level–
Igor Dolgachev and Michela Artebani, 2009, "The Hesse Pencil of Plane Cubic Curves ."
From the Dolgachev-Artebani introduction–
In this paper we discuss some old and new results about the widely known Hesse
configuration of 9 points and 12 lines in the projective plane P2(k ): each point lies
on 4 lines and each line contains 3 points, giving an abstract configuration (123, 94).
PlanetMath.org on the Hesse configuration—

A picture of the Hesse configuration–

(See Visualizing GL(2,p), a note from 1985).
Related notes from this journal —
From last November —
From 2006 —
Also from 2006 —
|
Sunday November 26, 2006
m759 @ 7:26 AM
Rosalind Krauss
in "Grids," 1979:
"If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.
Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
checking strange correspondences between them."
– The Club Dumas ,1993
"And it's whispered that soon if we all call the tune
Then the piper will lead us to reason."
– Robert Plant ,1971
The nine engravings of The Club Dumas
(filmed as "The Ninth Gate") are perhaps more
an example of the concrete than of the universal.
An example of the universal*– or, according to Krauss,
a "staircase" to the universal– is the ninefold square:

"This is the garden of Apollo, the field of Reason…."
– John Outram, architect
For more on the field of reason, see
Log24, Oct. 9, 2006.
A reasonable set of "strange correspondences"
in the garden of Apollo has been provided by
Ezra Brown in a mathematical essay (pdf).
Unreason is, of course, more popular.
* The ninefold square is perhaps a "concrete universal" in the sense of Hegel:
"Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
– Hegel, Lectures on the History of Philosophy ,
"Idea of a Concrete Universal Unity"
"For every kind of vampire,
there is a kind of cross."
– Thomas Pynchon
|
And from last October —
"You've got to pick up every stitch…"
Comments Off on True Grid (continued)
Friday, January 7, 2011
(A continuation of this morning's Coxeter and the Aleph)
"You've got to pick up every stitch… Must be the season of the witch."
— Donovan song at the end of Nicole Kidman's "To Die For"
"As is well known, the Aleph is the first letter of the Hebrew alphabet.
Its use for the strange sphere in my story may not be accidental.
For the Kabbala, the letter stands for the En Soph ,
the pure and boundless godhead; it is also said that it takes
the shape of a man pointing to both heaven and earth, in order to show
that the lower world is the map and mirror of the higher; for Cantor's
Mengenlehre , it is the symbol of transfinite numbers,
of which any part is as great as the whole."
— Borges, "The Aleph"
Ein Sof
Ein Soph or Ayn Sof (Hebrew אין סוף, literally "without end", denoting "boundlessness" and/or "nothingness"), is a Kabbalistic term that usually refers to an abstract state of existence preceding God's Creation of the limited universe. This Ein Sof , typically referred to figuratively as the "light of Ein Sof " ("Or Ein Sof "), is the most fundamental emanation manifested by God. The Ein Sof is the material basis of Creation that, when focused, restricted, and filtered through the sefirot , results in the created, dynamic universe.
….
Cultural impact
Mathematician Georg Cantor labeled different sizes of infinity using the Aleph. The smallest size of infinity is aleph-null (ℵ0), the second size is aleph-one (ℵ1), etc. One theory about why Cantor chose to use the aleph is because it is the first letter of Ein-Sof. (See Aleph number)
|
"Infinite Jest… now stands as the principal contender
for what serious literature can aspire to
in the late twentieth and early twenty-first centuries."
— All Things Shining, a work of pop philosophy published January 4th

"You're gonna need a bigger boat." — Roy Scheider in "Jaws"
"We're gonna need more holy water." — "Season of the Witch," a film opening tonight
See also, with respect to David Foster Wallace, infinity, nihilism,
and the above reading of "Ayn Sof" as "nothingness,"
the quotations compiled as "Is Nothing Sacred?"
Comments Off on Ayn Sof
Saturday, December 18, 2010
Comments Off on Logic Tale
Tuesday, November 23, 2010
A reviewer says Steve Martin finds in his new novel An Object of Beauty "a sardonic morality tale."
From this journal on the day The Cube was published (see today's Art Object ) —
|
m759 @ 12:00 AM
The Past Revisited
From Log24 a year ago on this date, a quote from Many Dimensions (1931), by Charles Williams:
“Lord Arglay had a suspicion that the Stone would be purely logical. Yes, he thought, but what, in that sense, were the rules of its pure logic?”
For the rest of the story, see the downloadable version at Project Gutenberg of Australia.
|
See also a post on Mathematics and Narrative from Nov. 14, 2009.
That post compares characters in Many Dimensions to those in Logicomix—

Comments Off on Tale
Saturday, November 13, 2010
From the December 2010 American Mathematical Society Notices—

Related material from this journal—
Mathematics and Narrative and
Consolation Prize (August 19, 2010)
Comments Off on Story
Monday, October 25, 2010
A New York Times "The Stone" post from yesterday (5:15 PM, by John Allen Paulos) was titled—
Stories vs. Statistics
Related Google searches—
"How to lie with statistics"— about 148,000 results
"How to lie with stories"— 2 results
What does this tell us?
Consider also Paulos's phrase "imbedding the God character." A less controversial topic might be (with the spelling I prefer) "embedding the miraculous." For an example, see this journal's "Mathematics and Narrative" entry on 5/15 (a date suggested, coincidentally, by the time of Paulos's post)—
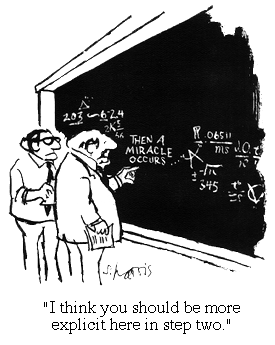
Cartoon by S.Harris
* Not directly related to the novel The Embedding discussed at Tenser, said the Tensor on April 23, 2006 ("Quasimodo Sunday"). An academic discussion of that novel furnishes an example of narrative as more than mere entertainment. See Timothy J. Reiss, "How can 'New' Meaning Be Thought? Fictions of Science, Science Fictions," Canadian Review of Comparative Literature , Vol. 12, No. 1, March 1985, pp. 88-126. Consider also on this, Picasso's birthday, his saying that "Art is a lie that makes us realize truth…."
Comments Off on The Embedding*
Thursday, October 21, 2010
Mathematics and Narrative continued
A search for Ursula in this journal yields a story…
“The main character is a slave woman who discovers new patterns in the mosaics.”
Other such stories: Plato’s Meno and Changing Woman —
Philosophical postscript—
“That Lévi-Strauss should have been able to transmute the romantic passion of Tristes Tropiques into the hypermodern intellectualism of La Pensée Sauvage is surely a startling achievement. But there remain the questions one cannot help but ask. Is this transmutation science or alchemy? Is the ‘very simple transformation’ which produced a general theory out of a personal disappointment real or a sleight of hand? Is it a genuine demolition of the walls which seem to separate mind from mind by showing that the walls are surface structures only, or is it an elaborately disguised evasion necessitated by a failure to breach them when they were directly encountered? Is Lévi-Strauss writing, as he seems to be claiming in the confident pages of La Pensée Sauvage, a prolegomenon to all future anthropology? Or is he, like some uprooted neolithic intelligence cast away on a reservation, shuffling the debris of old traditions in a vain attempt to revivify a primitive faith whose moral beauty is still apparent but from which both relevance and credibility have long since departed?”
— Clifford Geertz, conclusion of “The Cerebral Savage: On the Work of Claude Lévi-Strauss“
Comments Off on St. Ursula’s Day
Friday, October 8, 2010
… and Finishing Up at Noon
This post was suggested by last evening’s post on mathematics and narrative
and by Michiko Kakutani on Vargas Llosa in this morning’s New York Times.
“One must proceed cautiously, for this road— of truth and falsehood in the realm of fiction— is riddled with traps and any enticing oasis is usually a mirage.”
— “Is Fiction the Art of Lying?”* by Mario Vargas Llosa, New York Times essay of October 7, 1984
My own adventures in that realm— as reader, not author— may illustrate Llosa’s remark.
A nearby stack of paperbacks I haven’t touched for some months (in order from bottom to top)—
- Pale Rider by Alan Dean Foster
- Franny and Zooey by J. D. Salinger
- The Hobbit by J. R. R. Tolkien
- Le Petit Prince by Antoine de Saint Exupéry
- Literary Reflections by James A. Michener
- The Ninth Configuration by William Peter Blatty
- A Streetcar Named Desire by Tennessee Williams
- Nine Stories by J. D. Salinger
- A Midsummer Night’s Dream by William Shakespeare
- The Tempest by William Shakespeare
- Being There by Jerzy Kosinski
- What Dreams May Come by Richard Matheson
- Zen and the Art of Motorcycle Maintenance by Robert M. Pirsig
- A Gathering of Spies by John Altman
- Selected Poems by Robinson Jeffers
- Hook— Tinkerbell’s Challenge by Tristar Pictures
- Rising Sun by Michael Crichton
- Changewar by Fritz Leiber
- The Painted Word by Tom Wolfe
- The Hustler by Walter Tevis
- The Natural by Bernard Malamud
- Truly Tasteless Jokes by Blanche Knott
- The Man Who Was Thursday by G. K. Chesterton
- Under the Volcano by Malcolm Lowry
What moral Vargas Llosa might draw from the above stack I do not know.
Generally, I prefer the sorts of books in a different nearby stack. See Sisteen, from May 25. That post the fanciful reader may view as related to number 16 in the above list. The reader may also relate numbers 24 and 22 above (an odd couple) to By Chance, from Thursday, July 22.
* The Web version’s title has a misprint— “living” instead of “lying.”
Comments Off on Starting Out in the Evening
Wednesday, October 6, 2010
Notes on Mathematics and Narrative, continued
"the Citizen Kane of horror films"
— Sarah Lawless quoting other reviews
in Saga of the Wicker Man,
cited here on September 7
"Frivolous as a willow on a tombstone"
— Robert Stone on "our secret culture" in A Flag for Sunrise
"world's wildfire, leave but ash"
— Gerard Manley Hopkins, S.J.,
quoted here on October 4
Happy birthday, Britt.
Comments Off on The Rising…
Tuesday, September 21, 2010
"I know no writing— except perhaps Henry James's introductory essays— which conveys so clearly and with such an absence of fuss the excitement of the creative artist."
— Graham Greene on A Mathematician's Apology , review in The Spectator , 20 December 1940
"The mere quality and play of an ironic consciousness in the designer left wholly alone, amid a chattering unperceiving world, with the thing he has most wanted to do, with the design more or less realised— some effectual glimpse of that might, by itself, for instance, reward one's experiment."
— Henry James, "Prefaces to the New York Edition," in The Figure in the Carpet and Other Stories, Penguin Books, 1986, with introduction and notes by Frank Kermode
"What? You've found a pattern?"
— Greg Egan, "Wang's Carpets"
See also Notes on Mathematics and Narrative, with its discussion of the tiles of the creative artist Patrick Blackburn in the recent (August 2010) Pythagorean novel The Thousand and the discussion of Wang tiles in Modal Logic, a book from November 2002 whose author also happens to be named Patrick Blackburn.
(Credit for the Greene bibliographic information is due to Janelle Robyn Humphreys, whose doctoral thesis, Shadows of Another Dimension, was published in 2009 by the University of Wollongong.)
Comments Off on Patterns in the Carpets
Friday, September 17, 2010
From Peter J. Cameron's web journal today—
|
… Eliot’s Four Quartets has been one of my favourite works of poetry since I was a student….
Of course, a poem doesn’t have a single meaning, especially one as long and complex as Four Quartets. But to me the primary meaning of the poem is about the relationship between time and eternity, which is something maybe of interest to mathematicians as well as to mystics.
Curiously, the clearest explanation of what Eliot is saying that I have found is in a completely different work, Pilgrimage of Dreams by the artist Thetis Blacker, in which she describes a series of dreams she had which stood out as being completely different from the confusion of normal dreaming. In one of these dreams, “Mr Goad and the Cathedral”, we find the statements
“Eternity isn’t a long time”
and
“Eternity is always now, but …”
“Now isn’t always eternity”.
In other words, eternity is not the same as infinity; it is not the time line stretched out to infinity. Rather, it is an intimation of a different dimension, which we obtain only because we are aware of the point at which that dimension intersects the familiar dimension of time. In a recurring motif in the second Quartet, “East Coker”, Eliot says,
Time future and time past
Are both somehow contained in time present
and, in “Little Gidding”,
… to apprehend
The point of intersection of the timeless
With time, is an occupation for the saint
|
From this journal on the date of Blacker's death—
what would, if she were a Catholic saint, be called her dies natalis—
|
m759 @ 7:20 AM
Fade to Black:
Martin Gardner in the Notices of the American Mathematical Society, June/July 2005 (pdf):
“I did a column in Scientific American on minimal art, and I reproduced one of Ed Rinehart’s [sic ] black paintings. Of course, it was just a solid square of pure black.”

Click on picture for details.
The Notices of the American Mathematical Society, January 2007 (pdf):
“This was just one of the many moments in this sad tale when there were no whistle-blowers. As a result the entire profession has received a very public and very bad black mark.”
– Joan S. Birman
Professor Emeritus of Mathematics
Barnard College and
Columbia University
|
Comments Off on Fade to Blacker
Thursday, September 9, 2010
Notes on Mathematics and Narrative, continued
Patrick Blackburn, meet Gideon Summerfield…
From a summary of a politically correct 1995 feminist detective novel about quilts, A Piece of Justice—
The story deals with “one Gideon Summerfield, deceased.” Summerfield, a former tutor at (the fictional) St. Agatha’s College, Cambridge University, “is about to become the recipient of the Waymark prize. This prize is awarded in Mathematics and has the same prestige as the Nobel. Summerfield had a rather lackluster career at St. Agatha’s, with the exception of one remarkable result that he obtained. It is for this result that he is being awarded the prize, albeit posthumously.” Someone is apparently trying to prevent a biography of Summerfield from being published.
The following page contains a critical part of the solution to the mystery:

Compare and contrast with an episode from the resume of a real Gideon Summerfield—
Head of Strategy, Designer City (May 1999 — January 2002)
Secured Web agency business from new and existing clients with compelling digital media strategies and oversaw delivery of creative, production and technical teams…. Clients included… Greenfingers and Lord of the Dance .
For material related to Greenfingers and Lord of the Dance , see Castle Kennedy Gardens at Wicker Man Locations.
Comments Off on Building a Mystery
Tuesday, September 7, 2010
Notes on Mathematics and Narrative
Background—
-
The Burning Man in Bester's classic The Stars My Destination,
-
The not-so-classic Hitler Plans Burning Man, and
-
The cult film The Wicker Man
Commentary on The Wicker Man—
Originally The Wicker Man was not well-received by critics in the UK. It was considered
to be bizarre, disturbing, and uncomfortable, with the hasty editing making the story confusing
and out of order…. Today this movie is considered a cult classic and has been called
the “Citizen Kane of horror films” by some reviewers. How did this film become a cult classic?
Real estate motto— Location, Location, Location.
Illustration— The fire leap scene from Wicker Man, filmed at Castle Kennedy—

From August 27—
In today's New York Times, Michiko Kakutani reviews a summer thriller
by Kevin Guilfoile. The Thousand is in the manner of Dan Brown's
2003 The Da Vinci Code or of Katherine Neville's 1988 The Eight .
From the review—
What connects these disparate events, it turns out, is a sinister organization
called the Thousand, made up of followers of the ancient Greek mathematician
and philosopher Pythagoras (yes, the same Pythagoras associated with
the triangle theorem that we learned in school).
As Mr. Guilfoile describes it, this organization is part Skull and Bones,
part Masonic lodge, part something much more twisted and nefarious….
The plot involves, in part,
… an eccentric artist’s mysterious masterwork, made up of thousands of
individually painted tiles that may cohere into an important message….
Not unlike the tiles in the Diamond Theory cover (see yesterday's post)
or, more aptly, the entries in this journal.

A brief prequel to the above dialogue—

In lieu of songs, here is a passage by Patrick Blackburn
more relevant to the art of The Thousand—

See also the pagan fire leaping in Dancing at Lughnasa.
Comments Off on Burning Patrick —
Saturday, August 7, 2010
For aficionados of mathematics and narrative —
Illustration from
"The Galois Quaternion— A Story"

This resembles an attempt by Coxeter in 1950 to represent
a Galois geometry in the Euclidean plane—
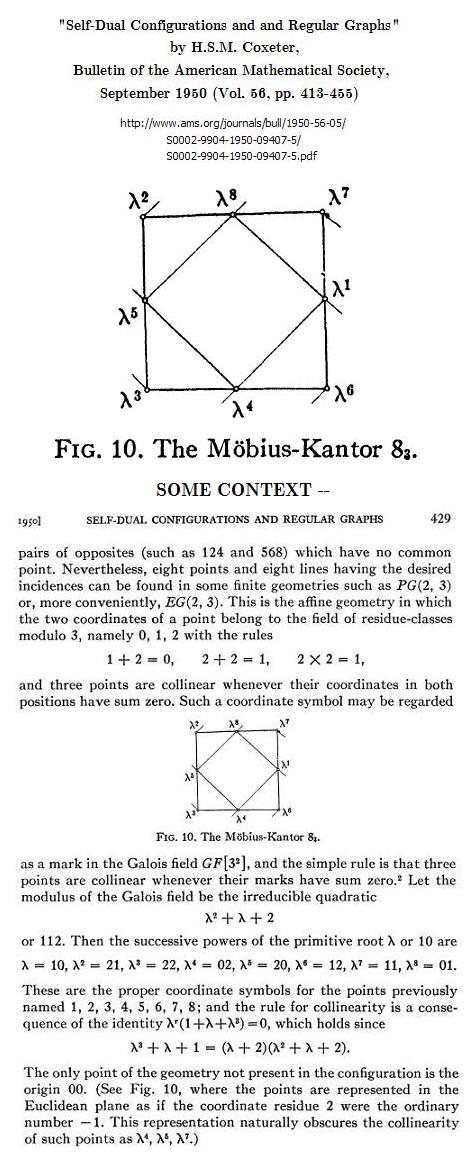
The quaternion illustration above shows a more natural way to picture this geometry—
not with dots representing points in the Euclidean plane, but rather with unit squares
representing points in a finite Galois affine plane. The use of unit squares to
represent points in Galois space allows, in at least some cases, the actions
of finite groups to be represented more naturally than in Euclidean space.
See Galois Geometry, Geometry Simplified, and
Finite Geometry of the Square and Cube.
Comments Off on The Matrix Reloaded
Saturday, June 5, 2010
The history of mathematics continues…
|
PRESS RELEASE
The Academician Professor Nicolaos Artemiadis will give a speech entitled "The Exploration of the Universe through the Mathematical Science" during a public session of the Academy of Athens (the speech will be in Greek).
The public session will be held on Tuesday, January 26th, 2010, at 19:00 at the Academy of Athens.
|
|
For some background on Professor Artemiadis, see two notes of July 2005 (the month an international conference on "Mathematics and Narrative" was held in Greece).
A post related by synchronicity to Artemiadis's Jan. 26 speech— Symbology.
Other philosophical remarks— "The Pediment of Appearance."
Comments Off on Academy Award
Tuesday, May 25, 2010
Darkness Visible
The inevitable tribute to Martin Gardner
has now appeared at the AMS website—

Related Imagery—
The following is an image from Saturday morning—

See also Art Wars and
Mathematics and Narrative.
Comments Off on ART WARS continued
Thursday, April 29, 2010
Mathematics and Narrative
(continued from April 26 and 28):
The Web

See also
Leiber's Big Time, Spider Woman, and The Eight.
Comments Off on Spider Woman
Thursday, December 3, 2009
Mathematics and Narrative, continued…
Out of What Chaos, a novel by Lee Oser—
"This book is more or less what one would expect if Walker Percy wrote about a cynical rock musician who converts to Catholicism, and then Nabokov added some of his verbal pyrotechnics, and then Buster Keaton and the Marquis de Sade and Lionel Trilling inserted a few extra passages. It is a loving and yet appalled description of the underground music scene in the Pacific Northwest. And it is a convincing representation of someone very, very smart."
—Matt Greenfield in The Valve
"If Evelyn Waugh had lived amid the American Northwest rock music scene, he might have written a book like this."
–Anonymous Amazon.com reviewer
A possible source for Oser's title–
"…Lytton Strachey described Pope's theme as 'civilization illumined by animosity; such was the passionate and complicated material from which he wove his patterns of balanced precision and polished clarity.' But out of what chaos did that clarity and precision come!"
—Authors at Work, by Herman W. Liebert and Robert H. Taylor, New York, Grolier Club, 1957, p. 16
Related material:
Unthought Known

and the

White Cube Gallery, 2002
Comments Off on Out of What Chaos
Saturday, September 5, 2009
Comments Off on Saturday September 5, 2009
Tuesday, August 25, 2009
Comments Off on Tuesday August 25, 2009
Tuesday, August 18, 2009
Prima Materia
(Background: Art Humor: Sein Feld (March 11, 2009) and Ides of March Sermon, 2009)
From Cardinal Manning's review of Kirkman's Philosophy Without Assumptions—
"And here I must confess… that between something and nothing I can find no intermediate except potentia, which does not mean force but possibility."
— Contemporary Review, Vol. 28 (June-November, 1876), page 1017
Furthermore….
Cardinal Manning, Contemporary Review, Vol. 28, pages 1026-1027:
The following will be, I believe, a correct statement of the Scholastic teaching:–
1. By strict process of reason we demonstrate a First Existence, a First Cause, a First Mover; and that this Existence, Cause, and Mover is Intelligence and Power.
2. This Power is eternal, and from all eternity has been in its fullest amplitude; nothing in it is latent, dormant, or in germ: but its whole existence is in actu, that is, in actual perfection, and in complete expansion or actuality. In other words God is Actus Purus, in whose being nothing is potential, in potentia, but in Him all things potentially exist.
3. In the power of God, therefore, exists the original matter (prima materia) of all things; but that prima materia is pura potentia, a nihilo distincta, a mere potentiality or possibility; nevertheless, it is not a nothing, but a possible existence. When it is said that the prima materia of all things exists in the power of God, it does not mean that it is of the existence of God, which would involve Pantheism, but that its actual existence is possible.
4. Of things possible by the power of God, some come into actual existence, and their existence is determined by the impression of a form upon this materia prima. The form is the first act which determines the existence and the species of each, and this act is wrought by the will and power of God. By this union of form with the materia prima, the materia secunda or the materia signata is constituted.
5. This form is called forma substantialis because it determines the being of each existence, and is the root of all its properties and the cause of all its operations.
6. And yet the materia prima has no actual existence before the form is impressed. They come into existence simultaneously;
[p. 1027 begins]
as the voice and articulation, to use St. Augustine's illustration, are simultaneous in speech.
7. In all existing things there are, therefore, two principles; the one active, which is the form– the other passive, which is the matter; but when united, they have a unity which determines the existence of the species. The form is that by which each is what it is.
8. It is the form that gives to each its unity of cohesion, its law, and its specific nature.*
When, therefore, we are asked whether matter exists or no, we answer, It is as certain that matter exists as that form exists; but all the phenomena which fall under sense prove the existence of the unity, cohesion, species, that is, of the form of each, and this is a proof that what was once in mere possibility is now in actual existence. It is, and that is both form and matter.
When we are further asked what is matter, we answer readily, It is not God, nor the substance of God; nor the presence of God arrayed in phenomena; nor the uncreated will of God veiled in a world of illusions, deluding us with shadows into the belief of substance: much less is it catter [pejorative term in the book under review], and still less is it nothing. It is a reality, the physical kind or nature of which is as unknown in its quiddity or quality as its existence is certainly known to the reason of man.
* "… its specific nature"
(Click to enlarge) —
The Catholic physics expounded by Cardinal Manning above is the physics of Aristotle.
For a more modern treatment of these topics, see Werner Heisenberg's Physics and Philosophy. For instance:
"The probability wave of Bohr, Kramers, Slater, however, meant… a tendency for something. It was a quantitative version of the old concept of 'potentia' in Aristotelian philosophy. It introduced something standing in the middle between the idea of an event and the actual event, a strange kind of physical reality just in the middle between possibility and reality."
Compare to Cardinal Manning's statement above:
"… between something and nothing I can find no intermediate except potentia…"
To the mathematician, the cardinal's statement suggests the set of real numbers between 1 and 0, inclusive, by which probabilities are measured. Mappings of purely physical events to this set of numbers are perhaps better described by applied mathematicians and physicists than by philosophers, theologians, or storytellers. (Cf. Voltaire's mockery of possible-worlds philosophy and, more recently, The Onion's mockery of the fictional storyteller Fournier's quantum flux. See also Mathematics and Narrative.)
Regarding events that are not purely physical– those that have meaning for mankind, and perhaps for God– events affecting conception, birth, life, and death– the remarks of applied mathematicians and physicists are often ignorant and obnoxious, and very often do more harm than good. For such meaningful events, the philosophers, theologians, and storytellers are better guides. See, for instance, the works of Jung and those of his school. Meaningful events sometimes (perhaps, to God, always) exhibit striking correspondences. For the study of such correspondences, the compact topological space [0, 1] discussed above is perhaps less helpful than the finite Galois field GF(64)– in its guise as the I Ching. Those who insist on dragging God into the picture may consult St. Augustine's Day, 2006, and Hitler's Still Point.
Comments Off on Tuesday August 18, 2009
Sunday, August 16, 2009
Return to Paradise
(Title of a New Yorker
essay dated June 2, 2008)
Kenneth Bacon, an advocate for
refugees, died yesterday
at 64 on the Feast of the Assumption.
In his honor, we may perhaps be justified in temporarily ignoring the wise saying "never assume."
From a defense of the dogma of the Assumption:
"On another level, the Assumption epitomizes the reconciliation of the material and spiritual world, as the human Mary enters 'body and soul to heavenly glory.' Carl Jung, the transpersonal psychologist, concluded that the doctrine of the Assumption reflected an acceptance of the physical world."
For other such reconciliations, see
Comments Off on Sunday August 16, 2009
Sunday, August 2, 2009
Spider Girl
"The 'magico-religious' tarantella
is a solo dance performed
supposedly to cure…
the delirium and contortions
attributed to the bite of a spider
at harvest (summer) time."
— Wikipedia

Moral:
Life's a dance
(and Jersey girls
are tough).
 For Mira Sorvino, star of "Tarantella,"
For Mira Sorvino, star of "Tarantella,"
who was raised in Tenafly, New Jersey–
Bull on Sacred Cows:
"Poor late nineteenth-century, poor early twentieth-century! Oh, brave new world that had such people in it: people like Charles Darwin, Karl Marx, Friedrich Nietzsche, Sigmund Freud, Albert Einstein, Werner Heisenberg, Kurt Gödel. Seven people who did more than all the machine-guns and canons of the Somme Valley or the Panzer divisions of Hitler to end the old world and to create– if not the answers– at least the questions that started off the new, each one of them killing one of the sacred cows on which Western consciousness had fed for so long…."
— Apostolos Doxiadis, "Writing Incompleteness-– the Play" (pdf).
See also Mathematics and Narrative.
Comments Off on Sunday August 2, 2009
Tuesday, July 21, 2009
Today's Readings:
-
The White Itself
Plato and the "concrete universal"--
Log24 on Thursday, July 16, 2009
-
Edged with Brown
Context for a Log24 entry of July 16:
"So we moved, and they, in a formal pattern,
Along the empty alley, into the box circle,
To look down into the drained pool.
Dry the pool, dry concrete, brown edged,
And the pool was filled with water out of sunlight,
And the lotos rose, quietly, quietly,
The surface glittered out of heart of light...."
-
Signifying Nothing
An essay on Harvard professor
Henry Louis Gates, Jr.
-
Professor Gates Arrested
A racial incident in Cambridge
on Thursday, July 16, 2009
-
New England White
Race relations in Academia
-
Notes on Mathematics
and Narrative
"... the glue that binds the brotherhood
is ultimately made not of
love and interracial harmony,
but of something stronger and more
enduring: shame, fear, and greed."
-- Review of New England White
-
Don't Forget Hate
Cf. Eugene Burdick and The Word, 1966.
More recently, Tom Wolfe and The Word
and Pig and Rat Get Lost.
Comments Off on Tuesday July 21, 2009
Tuesday, July 14, 2009
For Galois on Bastille Day Elements
of Finite Geometry
Some fans of the alchemy in
Katherine Neville’s novel
The Eight and in Dan Brown’s
novel Angels & Demons may
enjoy the following analogy–

Note that the alchemical structure
at left, suited more to narrative
than to mathematics, nevertheless
is mirrored within the pure
mathematics at right.
Related material
on Galois and geometry:
Geometries of the group PSL(2, 11)
by Francis Buekenhout, Philippe Cara, and Koen Vanmeerbeek. Geom. Dedicata, 83 (1-3): 169–206, 2000–
|
Comments Off on Tuesday July 14, 2009
Thursday, July 9, 2009
Comments Off on Thursday July 9, 2009
Wednesday, June 3, 2009
Epigraphs
to Four Quartets:

The Dissertations of Maximus Tyrius, translated from the Greek by Thomas Taylor, printed by C. Whittingham, London, for the translator, 1804, Vol. II, p. 55:
"You see the mutation of bodies, and the transition of generation, a path upwards and downwards according to Heraclitus; and again, as he says, one thing living the death, but dying the life of another. Thus fire lives the death of earth, and air lives the death of fire; water lives the death of air, and earth lives the death of water. You see a succession of life, and a mutation of bodies, both of which are the renovation of the whole."

For an interpretation
of the above figure
in terms of the classical
four elements discussed
in Four Quartets,
in Dissertations, and
in Angels & Demons,
see
Notes on Mathematics
and Narrative.
For a more entertaining
interpretation, see Fritz Leiber's
classic story "Damnation Morning."
Comments Off on Wednesday June 3, 2009
Thursday, March 19, 2009
Two-Face

[Note: Janus is Roman, not Greek, and
the photo is from one “Fubar Obfusco”]  Click on image for details.
From January 8:
Click on image for details.
From January 8:
Religion and Narrative, continued:
| A Public Square
In memory of
Richard John Neuhaus,
who died today at 72:
“It seems, as one becomes older,
That the past has another pattern,
and ceases to be a mere sequence….”
— T. S. Eliot, Four Quartets

Click on image for details.
See also The Folding.
Posted 1/8/2009 7:00 PM |
|
Context:
Notes on Mathematics and Narrative
(entries in chronological order,
March 13 through 19)
Comments Off on Thursday March 19, 2009
Tuesday, March 17, 2009
"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."
— Edward Buckner at The Logic Museum
From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:

The Semiotic Square
"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."
* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter
Another version of the semiotic square:

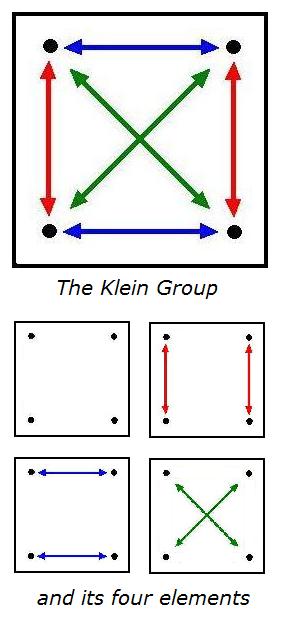
Krauss says that her figure "is, of course, a Klein Group."
Here is a more explicit figure representing the Klein group:
There is also the logical
diamond of opposition —

A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
a Marxist literary theorist:
|
"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–
The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.)
Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1):

As Jameson explains in the Foreword to Greimas' On Meaning, "-s 1 and -s 2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.
* Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.
|
"The Game in the Ship cannot be approached as a job, a vocation, a career, or a recreation. To the contrary, it is Life and Death itself at work there. In the Inner Game, we call the Game Dhum Welur, the Mind of God."
— The Gameplayers of Zan, by M.A. Foster
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
Gravity's Rainbow
Crosses used by semioticians
to baffle their opponents
are illustrated above.
Some other kinds of crosses,
and another kind of opponent:
Monday, July 11, 2005
Logos
for St. Benedict's Day
Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org.
 
Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth. Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion. I personally prefer the "diamond theory" of truth, represented by the logo below.

See also the previous entry
(below) and the entries
of 7/11, 2003.
Sunday, July 10, 2005
|
From Artemiadis's website:
|
|
1986:
|
Elected Regular Member
of the Academy of Athens
|
|
1999:
|
Vice President
of the Academy of Athens
|
|
2000:
|
President
of the Academy of Athens
|

"First of all, I'd like to
thank the Academy…"
— Remark attributed to Plato
|
Comments Off on Tuesday March 17, 2009
Monday, March 9, 2009
Humorism

"Always with a
little humor."
— Dr. Yen Lo

From Temperament: A Brief Survey
For other interpretations
of the above shape, see
The Illuminati Diamond.
from Jung's Aion:
"From the circle and quaternity motif is derived the symbol of the geometrically formed crystal and the wonder-working stone. From here analogy formation leads on to the city, castle, church, house, room, and vessel. Another variant is the wheel. The former motif emphasizes the ego’s containment in the greater dimension of the self; the latter emphasizes the rotation which also appears as a ritual circumambulation. Psychologically, it denotes concentration on and preoccupation with a centre…." –Jung,
Collected Works, Vol. 9, Part II, paragraph 352
As for rotation, see the ambigrams in Dan Brown's Angels & Demons (to appear as a film May 15) and the following figures:
Comments Off on Monday March 9, 2009
Saturday, February 28, 2009
Mathematics
and Narrative
continued
Narrative:
xxx
Mathematics:
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference, (July 2000), Springer, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Mathematics:
"Regular graphs are considered, whose automorphism groups are permutation representations P of the orthogonal groups in various dimensions over GF(2). Vertices and adjacencies are defined by quadratic forms, and after graphical displays of the trivial isomorphisms between the symmetric groups S2, S3, S5, S6 and corresponding orthogonal groups, a 28-vertex graph is constructed that displays the isomorphism between S8 and O6 + (2)."
— J. Sutherland Frame in "Orthogonal Groups over GF(2) and Related Graphs," Springer Lecture Notes in Mathematics vol. 642, Theory and Applications of Graphs (Proceedings, Michigan, May 11–15, 1976), edited by Y. Alavi and D. R. Lick, pp. 174-185
"One has O+(6) ≅ S8, the symmetric group of order 8!…."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint. This paper gives some context in superstring theory for the following work of Frame:
[F1] J.S. Frame, The classes and representations of the group of 27 lines and 28 bitangents, Annali
di Mathematica Pura ed Applicata, 32 (1951) 83–119.
[F2] J.S. Frame, Some characters of orthogonal groups over the field of two elements, In: Proc. of the
Second Inter. Conf. on the Theory of Groups, Lecture Notes in Math., Vol. 372, pp. 298–314,
Springer, 1974.
[F3] J. S. Frame, Degree polynomials for the orthogonal groups over GF(2), C. R. Math. Rep. Acad.
Sci. Canada 2 (1980) 253–258.
Comments Off on Saturday February 28, 2009
Tuesday, February 24, 2009
Hollywood Nihilism
Meets
Pantheistic Solipsism
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."

|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer…
A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination.
|
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
Heinlein:
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
Stevens:
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in
The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
This interpretation might appeal to Joan Didion, who, as author of the classic novel
Play It As It Lays, is perhaps the world's leading expert on
Hollywood nihilism.
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)

over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Heinlein should perhaps have had in mind
the Klein correspondence when he discussed "some way to project six dimensions into three." While such a projection is of course trivial for anyone who has taken an undergraduate course in linear algebra, the following remarks by Philippe Cara present a much more meaningful mapping, using the Klein correspondence, of structures in six (affine) dimensions to structures in three.
Cara:

Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Comments Off on Tuesday February 24, 2009
Sunday, February 15, 2009
From April 28, 2008:
Religious Art
The black monolith of
Kubrick's 2001 is, in
its way, an example
of religious art.

One artistic shortcoming
(or strength– it is, after
all, monolithic) of
that artifact is its
resistance to being
analyzed as a whole
consisting of parts, as
in a Joycean epiphany.
The following
figure does
allow such
an epiphany.

One approach to
the epiphany:
"Transformations play
a major role in
modern mathematics."
– A biography of
Felix Christian Klein
See 4/28/08 for examples
of such transformations.
|
Related material:
|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, pp. 117-118:
"… his point of origin is external nature, the fount to which we come seeking inspiration for our fictions. We come, many of Stevens's poems suggest, as initiates, ritualistically celebrating the place through which we will travel to achieve fictive shape. Stevens's 'real' is a bountiful place, continually giving forth life, continually changing. It is fertile enough to meet any imagination, as florid and as multifaceted as the tropical flora about which the poet often writes. It therefore naturally lends itself to rituals of spring rebirth, summer fruition, and fall harvest. But in Stevens's fictive world, these rituals are symbols: they acknowledge the real and thereby enable the initiate to pass beyond it into the realms of his fictions.
Two counter rituals help to explain the function of celebration as Stevens envisions it. The first occurs in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer. A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. For in 'Notes Toward a Supreme Fiction' he tells us that
... the first idea was not to shape the clouds
In imitation. The clouds preceded us.
There was a muddy centre before we breathed.
There was a myth before the myth began,
Venerable and articulate and complete.
From this the poem springs: that we live in a place
That is not our own and, much more, not ourselves
And hard it is in spite of blazoned days.
We are the mimics.
(Collected Poems, 383-84)
Believing that they are the life and not the mimics thereof, the world and not its fiction-forming imitators, these young men cannot find the savage transparence for which they are looking. In its place they find the pediment, a scowling rock that, far from being life's source, is symbol of the human delusion that there exists a 'form alone,' apart from 'chains of circumstance.'
A far more productive ritual occurs in 'Sunday Morning.'…."
|
For transformations of a more
specifically religious nature,
see the remarks on
Richard Strauss,
"Death and Transfiguration,"
(Tod und Verklärung, Opus 24)
in Mathematics and Metaphor
on July 31, 2008, and the entries
of August 3, 2008, related to the
death of Alexander Solzhenitsyn.
Comments Off on Sunday February 15, 2009
Friday, January 23, 2009
Mathematics:
Happy Hilbert’s Birthday.
Today is the birthday
of mathematician
David Hilbert (1862-1943).
Narrative:
See a different Hilbert– namely Jules, a fictional professor of literary theory at the University of Illinois at Chicago played by Dustin Hoffman in “Stranger than Fiction” (cf. yesterday’s entry). See also, in today’s previous entry, Stanley Fish– a non-fictional literary theorist and former dean at the same institution.
Related material:
“The Gift in ‘Stranger than Fiction’,” by Eric Austin Lee (June 1, 2007).
Lee’s essay might please another mathematician whose name appears in the film. The clever but heartless Professor Hilbert is opposed, indirectly, in “Stranger than Fiction” by a Harvard Law dropout, Ana, who dispenses eucharistic blessings, in the form of cookies, at her Chicago bakery/café– filmed at a real Chicago location, Catedral Café. Her last name is Pascal.
Comments Off on Friday January 23, 2009
Friday, December 12, 2008
On the Symmetric Group S8
Wikipedia on Rubik's 2×2×2 "Pocket Cube"–
"Any permutation of the 8 corner cubies is possible (8! positions)."
Some pages related to this claim–
Simple Groups at Play
Analyzing Rubik's Cube with GAP
Online JavaScript Pocket Cube.
The claim is of course trivially true for the unconnected subcubes of Froebel's Third Gift:

 © 2005 The Institute for Figuring
© 2005 The Institute for Figuring
Comments Off on Friday December 12, 2008
Friday, November 21, 2008
Gatsby Starts Over:
Cleaning Up the
St. Olaf Mess
St. Olaf College,
Northfield, Minnesota —
From The MSCS Mess
(Dept. of Mathematics, Statistics,
and Computer Science)
November 14, 2008
Volume 37, Number 9—
Math Film Festival 2008
The MSCS Department is sponsoring the second of two film-discussion evenings this Wednesday, November 19. Come to RNS 390 at 7:00 PM to see watch [sic] two short [sic]— Whatchu Know 'bout Math and Just a Finite Simple Group of Order Two— and our feature film, Good Will Hunting. Will Hunting is a mathematical genius who's living a rough life in South Boston, while being employed at a prestigious college in Boston, he's [sic] discovered by a Fields Medal winning mathematics Professor [sic] who eventually tries to get Will to turn his life around but becomes haunted by his own professional inadequacies when compared with Will. Professor Garrett will explain the “impossible problem” and its solution after the film.
Background:
Log24 entries of Wednesday, November 19, the day "Good Will Hunting" was shown:
Damnation Morning revisited and
Mathematics and Narrative continued
From
a story in the November 21
Chronicle of Higher Education
on a recent St. Olaf College
reading of
Paradise Lost:
" Of man's first disobedience,
and the fruit
Of that forbidden tree,
whose mortal taste
Brought death into the World,
and all our woe….
A red apple made the rounds,
each reader tempting the next." |
Comments Off on Friday November 21, 2008
Wednesday, November 19, 2008
"Through the unknown,
remembered gate…."
— Four Quartets
(Epigraph to the introduction,
Parallelisms of Complete Designs
by Peter J. Cameron,
Merton College, Oxford)
"It's still the same old story…."
— Song lyric
The Great Gatsby, Chapter 6:
"An instinct toward his future glory had led him, some months before, to the small Lutheran college of St. Olaf in southern Minnesota. He stayed there two weeks, dismayed at its ferocious indifference to the drums of his destiny, to destiny itself, and despising the janitor’s work with which he was to pay his way through."
There is a link to an article on St. Olaf College in Arts & Letters Daily today:
"John Milton, boring? Paradise Lost has a little bit of something for everybody. Hot sex! Hellfire! Some damned good poetry, too…" more»
The "more" link is to The Chronicle of Higher Education.
For related material on Paradise Lost and higher education, see Mathematics and Narrative.
Comments Off on Wednesday November 19, 2008
Wednesday, August 6, 2008
From the last link within the last link of yesterday’s entry:
“Review the concepts of integritas, consonantia, and claritas in Aquinas….”
Review also the properties of the number six that appears in today’s date.
For such properties, see the page of Log24 entries that end on September 6, 2006, with “Hamlet’s Transformation.”
Happy Feast of the Transfiguration.
Comments Off on Wednesday August 6, 2008
Sunday, August 3, 2008
 "Russian writer Alexander Solzhenitsyn, who exposed Stalin's prison system in his novels and spent 20 years in exile, has died near Moscow at the age of 89.
"Russian writer Alexander Solzhenitsyn, who exposed Stalin's prison system in his novels and spent 20 years in exile, has died near Moscow at the age of 89.
The author of The Gulag Archipelago and One Day In The Life Of Ivan Denisovich, who returned to Russia in 1994, died of either a stroke or heart failure.
The Nobel laureate had suffered from high blood pressure in recent years.
After returning to Russia, Solzhenitsyn wrote several polemics on Russian history and identity.
His son Stepan was quoted by one Russian news agency as saying his father died of heart failure, while another agency quoted literary sources as saying he had suffered a stroke.
He died in his home in the Moscow area, where he had lived with his wife Natalya, at 2345 local time (1945 GMT) [3:45 PM EDT], Stepan told Itar-Tass.
Russian President Dmitry Medvedev sent his condolences to the writer's family, a Kremlin spokesperson said…."
Related material:
Today's 3 PM (EDT) entry.
Comments Off on Sunday August 3, 2008
Tuesday, June 24, 2008
Plato’s Cave, continued:
… we know that we use
Only the eye as faculty, that the mind
Is the eye, and that this landscape of the mind
Is a landscape only of the eye; and that
We are ignorant men incapable
Of the least, minor, vital metaphor….
— Wallace Stevens, “Crude Foyer”
… So, so,
O son of man, the ignorant night, the travail
Of early morning, the mystery of the beginning
Again and again,
while history is unforgiven.
— Delmore Schwartz,
“In the Naked Bed, in Plato’s Cave“
Comments Off on Tuesday June 24, 2008
Comments Off on Tuesday June 24, 2008
Friday, May 9, 2008
Comments Off on Friday May 9, 2008
Tuesday, April 29, 2008
Sacerdotal Jargon
at Harvard:

Thomas Wolfe
(Harvard M.A., 1922)
versus

Rosalind Krauss
(Harvard M.A., 1964,
Ph.D., 1969)
on
The Kernel of Eternity
"No culture has a pact with eternity."
— George Steiner, interview in
The Guardian of April 19
"At that instant he saw,
in one blaze of light, an image
of unutterable conviction….
the core of life, the essential
pattern whence all other things
proceed, the kernel of eternity."
— Thomas Wolfe, Of Time
and the River, quoted in
Log24 on June 9, 2005
From today's online Harvard Crimson:
"… under the leadership of Faust,
Harvard students should look forward
to an ever-growing opportunity for
international experience
and artistic endeavor."

Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen
From a recent book
on Wolfgang Pauli,
The Innermost Kernel:

A belated happy birthday
to the late
Felix Christian Klein
(born on April 25) —

Another Harvard figure quoted here on Dec. 5, 2002:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951)
From a review of Rosalind Krauss's The Optical Unconscious (MIT Press hardcover, 1993):
Krauss is concerned to present Modernism less in terms of its history than its structure, which she seeks to represent by means of a kind of diagram: "It is more interesting to think of modernism as a graph or table than a history." The "table" is a square with diagonally connected corners, of the kind most likely to be familiar to readers as the Square of Opposition, found in elementary logic texts since the mid-19th century. The square, as Krauss sees it, defines a kind of idealized space "within which to work out unbearable contradictions produced within the real field of history." This she calls, using the inevitable gallicism, "the site of Jameson's Political Unconscious" and then, in art, the optical unconscious, which consists of what Utopian Modernism had to kick downstairs, to repress, to "evacuate… from its field."
— Arthur C. Danto in ArtForum, Summer 1993
Rosalind Krauss in The Optical Unconscious (MIT Press paperback, 1994):
For a presentation of the Klein Group, see Marc Barbut, "On the Meaning of the Word 'Structure' in Mathematics," in Introduction to Structuralism, ed. Michael Lane (New York: Basic Books, 1970). Claude Lévi-Strauss uses the Klein group in his analysis of the relation between Kwakiutl and Salish masks in The Way of the Masks, trans. Sylvia Modelski (Seattle: University of Washington Press, 1982), p. 125; and in relation to the Oedipus myth in "The Structural Analysis of Myth," Structural Anthropology, trans. Claire Jackobson [sic] and Brooke Grundfest Schoepf (New York: Basic Books, 1963). In a transformation of the Klein Group, A. J. Greimas has developed the semiotic square, which he describes as giving "a slightly different formulation to the same structure," in "The Interaction of Semiotic Constraints," On Meaning (Minneapolis: University of Minnesota Press, 1987), p. 50. Jameson uses the semiotic square in The Political Unconscious (see pp. 167, 254, 256, 277) [Fredric Jameson, The Political Unconscious: Narrative as a Socially Symbolic Act (Ithaca: Cornell University Press, 1981)], as does Louis Marin in "Disneyland: A Degenerate Utopia," Glyph, no. 1 (1977), p. 64.
For related non-sacerdotal jargon, see…
Wikipedia on the Klein group (denoted V, for Vierergruppe):
In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
Comments Off on Tuesday April 29, 2008
Sunday, April 13, 2008
The Echo
in Plato’s Cave
“It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy.”
— Simon Blackburn, Think (Oxford, 1999)
Michael Harris, mathematician at the University of Paris:
“… three ‘parts’ of tragedy identified by Aristotle that transpose to fiction of all types– plot (mythos), character (ethos), and ‘thought’ (dianoia)….”
— paper (pdf) to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.
Mythos —
A visitor from France this morning viewed the entry of Jan. 23, 2006: “In Defense of Hilbert (On His Birthday).” That entry concerns a remark of Michael Harris.
A check of Harris’s website reveals a new article:
“Do Androids Prove Theorems in Their Sleep?” (slighly longer version of article to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.) (pdf).
From that article:
“The word ‘key’ functions here to structure the reading of the article, to draw the reader’s attention initially to the element of the proof the author considers most important. Compare E.M. Forster in Aspects of the Novel:
[plot is] something which is measured not be minutes or hours, but by intensity, so that when we look at our past it does not stretch back evenly but piles up into a few notable pinnacles.”
Ethos —
“Forster took pains to widen and deepen the enigmatic character of his novel, to make it a puzzle insoluble within its own terms, or without. Early drafts of A Passage to India reveal a number of false starts. Forster repeatedly revised drafts of chapters thirteen through sixteen, which comprise the crux of the novel, the visit to the Marabar Caves. When he began writing the novel, his intention was to make the cave scene central and significant, but he did not yet know how:
When I began a A Passage to India, I knew something important happened in the Malabar (sic) Caves, and that it would have a central place in the novel– but I didn’t know what it would be… The Malabar Caves represented an area in which concentration can take place. They were to engender an event like an egg.”
— E. M. Forster: A Passage to India, by Betty Jay
Dianoia —
Flagrant Triviality
or Resplendent Trinity?
“Despite the flagrant triviality of the proof… this result is the key point in the paper.”
— Michael Harris, op. cit., quoting a mathematical paper
Online Etymology Dictionary:
flagrant c.1500, “resplendent,” from L. flagrantem (nom. flagrans) “burning,” prp. of flagrare “to burn,” from L. root *flag-, corresponding to PIE *bhleg– (cf. Gk. phlegein “to burn, scorch,” O.E. blæc “black”). Sense of “glaringly offensive” first recorded 1706, probably from common legalese phrase in flagrante delicto “red-handed,” lit. “with the crime still blazing.”
A related use of “resplendent”– applied to a Trinity, not a triviality– appears in the Liturgy of Malabar:
— The Liturgies of SS. Mark, James, Clement, Chrysostom, and Basil, and the Church of Malabar, by the Rev. J.M. Neale and the Rev. R.F. Littledale, reprinted by Gorgias Press, 2002
On Universals and
A Passage to India:
“”The universe, then, is less intimation than cipher: a mask rather than a revelation in the romantic sense. Does love meet with love? Do we receive but what we give? The answer is surely a paradox, the paradox that there are Platonic universals beyond, but that the glass is too dark to see them. Is there a light beyond the glass, or is it a mirror only to the self? The Platonic cave is even darker than Plato made it, for it introduces the echo, and so leaves us back in the world of men, which does not carry total meaning, is just a story of events.”
Comments Off on Sunday April 13, 2008
Friday, July 13, 2007
Today’s birthday:
Harrison Ford is 65.

“Three times the concentred
self takes hold, three times
The thrice concentred self,
having possessed
The object, grips it
in savage scrutiny,
Once to make captive,
once to subjugate
Or yield to subjugation,
once to proclaim
The meaning of the capture,
this hard prize,
Fully made, fully apparent,
fully found.”
— “Credences of Summer,” VII,
by Wallace Stevens, from
Transport to Summer (1947) |
“It was Plato who best expressed– who veritably embodied– the tension between the narrative arts and mathematics….
Plato clearly loved them both, both mathematics and poetry. But he approved of mathematics, and heartily, if conflictedly, disapproved of poetry. Engraved above the entrance to his Academy, the first European university, was the admonition: Oudeis ageometretos eiseto. Let none ignorant of geometry enter. This is an expression of high approval indeed, and the symbolism could not have been more perfect, since mathematics was, for Plato, the very gateway for all future knowledge. Mathematics ushers one into the realm of abstraction and universality, grasped only through pure reason. Mathematics is the threshold we cross to pass into the ideal, the truly real.”
Comments Off on Friday July 13, 2007
Thursday, July 12, 2007
On Interpenetration,
or Coinherence, of Souls
The August 2007 issue of Notices of the American Mathematical Society contains a review of a new book by Douglas Hofstadter, I Am a Strange Loop. (2007, Basic Books, New York. $26.95, 412 pages.)
A better review, in the Los Angeles Times of March 18, 2007, notes an important phrase in the book, "interpenetration of souls," that the AMS Notices review ignores.
Here is an Amazon.com search on "interpenetration" in the Hofstadter book:
|
1.
|
on Page 217:
|
|
"… described does not create a profound blurring of two people's identities. Tennis and driving do not give rise to deep interpenetrations of souls. …"
|
|
2.
|
on Page 237:
|
|
"… What seems crucial here is the depth of interpenetration of souls the sense of shared goals, which leads to shared identity. Thus, for instance, Carol always had a deep, …"
|
|
3.
|
on Page 270:
|
|
"… including the most private feelings and the most confidential confessions, then the interpenetration of our worlds becomes so great that our worldviews start to fuse. Just as I could jump to California when …"
|
|
4.
|
on Page 274:
|
|
"… we choose to downplay or totally ignore the implications of the everyday manifestations of the interpenetration of souls. Consider how profoundly wrapped up you can become in a close friend's successes and failures, in their very …"
|
|
5.
|
on Page 276:
|
|
"… Interpenetration of National Souls Earlier in this chapter, I briefly offered the image of a self as analogous to a country …"
|
|
6.
|
from Index:
|
|
"… birthday party for, 350 "bachelor", elusiveness of concept, 178 bad-breath analogy, 150 bandwidth of communication as determinant of degree of interpenetration, 212 213, 220, …"
|
|
7.
|
from Index:
|
|
"… phrases denying interpenetration of souls, 270 271; physical phenomena that lack consciousness, 281 282; physical structures lacking hereness, 283; potential personal attributes, 183; …"
|
The American Mathematical Society editors and reviewer seem to share Hofstadter's ignorance of Christian doctrine; they might otherwise have remembered a rather famous remark: "This is not mathematics, it is theology."
For more on the theology of interpenetration, see Log24 on "Perichoresis, or Coinherence" (Jan. 22, 2004).
For a more mathematical approach to this topic, see Spirituality Today, Spring 1991:
"… the most helpful image is perhaps the ellipse often used to surround divine figures in ancient art, a geometrical figure resulting from the overlapping, greater or lesser, of two independent circles, an interpenetration or coinherence which will, in some sense, reunify divided humanity, thus restoring to some imperfect degree the original image of God."
See also the trinitarian doctrine implicit in related Log24 entries of July 1, 2007, which include the following illustration of the geometrical figure described, in a somewhat confused manner, above:
Comments Off on Thursday July 12, 2007
Thursday, June 7, 2007
Masters of Chaos
From the May 6, 2007,
New York Times,
Charles McGrath on
Philip K. Dick:
His early novels, written in two weeks or less, were published in double-decker Ace paperbacks that included two books in one, with a lurid cover for each. "If the Holy Bible was printed as an Ace Double," an editor once remarked, "it would be cut down to two 20,000-word halves with the Old Testament retitled as 'Master of Chaos' and the New Testament as 'The Thing With Three Souls.'"

Click to enlarge.
As for "the thing with
three souls"–
Part I:
"Educate, Empower, Entertain"
— Motto of Yolanda King
Part II:
Three universities
(but not those of
Martin Myerson)–
Princeton, Harvard, Cambridge
Comments Off on Thursday June 7, 2007
Wednesday, May 23, 2007
Strong Emergence Illustrated:
The Beauty Test
"There is no royal road
to geometry"
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):

The illustration (and definition) is
of
regular tessellations of the plane.
This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:

One might argue that such beauty is
strongly emergent because of the "harmonious way" the parts fit together: the regularity (or fitting together) of the whole is not reducible to the regularity of the parts. (Regular triangles, squares, and hexagons fit together, but regular pentagons do not.)
The symmetries of these regular tessellations of the plane are less well suited as illustrations of emergence, since they are tied rather closely to symmetries of the component parts.
But the symmetries of regular tessellations of the
sphere— i.e., of the five Platonic solids–
do emerge strongly, being apparently independent of symmetries of the component parts.
Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
Comments Off on Wednesday May 23, 2007
Sunday, April 22, 2007
Built
continued from
March 25, 2006
In honor of Scarlett Johansson's recent London films "Match Point" and "Scoop," here is a link to an entry of Women's History Month, 2006, with a discussion of an exhibition of the works of artist Liza Lou at London's White Cube Gallery. That entry includes the following illustrations:
Comments Off on Sunday April 22, 2007
Friday, April 20, 2007
Icons
Part I
|
The Library of Congress
Today in History, April 20:
“American sculptor Daniel Chester French was born in Exeter, New Hampshire on April 20, 1850. His colossal seated figure of Abraham Lincoln presides over the Lincoln Memorial.
Reared in Cambridge and Concord, Massachusetts, he was embraced by members of the Transcendentalist community including Ralph Waldo Emerson. Author and fellow Concord resident Louisa May Alcott encouraged young French to pursue a career as an artist. Louisa’s sister, artist May Alcott, was his early teacher.
French studied in Boston and New York prior to receiving his first commission for the 1875 statue The Minute Man. Standing near the North Bridge in Concord, in the Minute Man National Historical Park, this work commemorates events at the North Bridge, the site of ‘the shot heard ’round the world.’ An American icon, images derivative of The Minute Man statue appeared on defense bonds, stamps, and posters during World War II.”
|
|
Saturday, April 14, 2007 4:30 AM
The Sun Also Sets, or…
This Way to
the Egress
Continued from April 12:
“I have only come here
seeking knowledge,
Things they would not
teach me of in college….”
— Synchronicity lyrics
Quoted in Log24,
Time’s Labyrinth continued:
“The sacred axe was used to kill the King. The ritual had been the same since the beginning of time. The game of chess was merely a reenactment. Why hadn’t I recognized it before?”
— Katherine Neville,
The Eight,
Ballantine reprint, 1990,
|
“Know the one about
the Demiurge and the
Abridgment of Hope?”
— Robert Stone,
A Flag for Sunrise,
Knopf, 1981,
the final page
|
Comments Off on Friday April 20, 2007
Monday, April 9, 2007
Symmetry
for Beavis and Butt-Head

(An illustration from
Mathematics and Narrative;
the “Book” is
The Gospel
According to St. Matthew.)
“
Is Beauty Truth and Truth Beauty?,”
a review by famed vulgarizer
Martin Gardner of the new book
by his fellow vulgarizer Ian Stewart
in the April 2007
Scientific American:
“Associated with every kind of symmetry is a ‘group.’ Stewart explains the group concept in a simple way by considering operations on an equilateral triangle. Rotate it 60 degrees in either direction, and it looks the same. Every operation has an ‘inverse,’ that cancels the operation. Imagine the corners of the triangle labeled A, B and C. A 60-degree clockwise rotation alters the corners’ positions. If this is followed by a similar rotation the other way, the original positions are restored. If you do nothing to the triangle, this is called the ‘identity’ operation. The set of all symmetry transformations of the triangle constitutes its group.”
“Is Beauty Truth?”
asked jesting Gardner…
The reasoned reply of
Beavis and Butt-Head:
“Sixty degrees, a hundred
and twenty degrees, who
gives a rat’s ass?”
Tuesday, January 9, 2007
For Balanchine's Birthday
(continued from
January 9, 2003)
George Balanchine
Encyclopædia Britannica Article
born January 22
[January 9, Old Style], 1904,
St. Petersburg, Russia
died April 30, 1983, New York,
New York, U.S.

George Balanchine.
©1983 Martha Swope
original name
Georgy Melitonovich Balanchivadze
most influential choreographer of classical ballet in the United States in the 20th century. His works, characterized by a cool neoclassicism, include The Nutcracker (1954) and Don Quixote (1965), both pieces choreographed for the New York City Ballet, of which he was a founder (1948), the artistic director, and the…
Balanchine, George… (75 of 1212 words)
|
"What on earth is
a concrete universal?"
— Robert M. Pirsig
Review:
From Wikipedia's
"Upper Ontology"
and
Epiphany 2007:
"There is no neutral ground
that can serve as
a means of translating between
specialized (lower) ontologies."
There is, however,
"the field of reason"–
the 3×3 grid:

Click on grid
for details.
As Rosalind Krauss
has noted, some artists
regard the grid as
"a staircase to
the Universal."
Other artists regard
Epiphany itself as an
approach to
the Universal:
"Epiphany signals the traversal
of the finite by the infinite,
of the particular by the universal,
of the mundane by the mystical,
of time by eternity."
— Richard Kearney, 2005,
in The New Arcadia Review

Kearney (right) with
Martin Scorsese (left)
and Gregory Peck
in 1997.
"… one of the things that worried me about traditional metaphysics, at least as I imbibed it in a very Scholastic manner at University College Dublin in the seventies, is that philosophy was realism and realism was truth. What disturbed me about that was that everything was already acquired; truth was always a systematic given and it was there to be learned from Creation onwards; it was spoken by Jesus Christ and then published by St. Thomas Aquinas: the system as perfect synthesis. Hence, my philosophy grew out of
a hunger for the 'possible' and it was definitely a reaction to my own philosophical formation. Yet that wasn't my only reaction. I was also reacting to what I considered to be the deep pessimism, and even at times 'nihilism' of the postmodern turn."
— Richard Kearney, interview (pdf) in The Leuven Philosophy Newsletter, Vol. 14, 2005-2006
For more on "the possible," see Kearney's The God Who May Be, Diamonds Are Forever, and the conclusion of Mathematics and Narrative:
"We symbolize
logical necessity
with the box ( ) )
and logical possibility
with the diamond ( )." )."
— Keith Allen Korcz

"The possibilia that exist,
and out of which
the Universe arose,
are located in
a necessary being…."
— Michael Sudduth,
Notes on
God, Chance, and Necessity
by Keith Ward,
Regius Professor of Divinity,
Christ Church College, Oxford
(the home of Lewis Carroll)
|
Friday, December 29, 2006
Tools
of Christ Church
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon

Click on picture for details.
Today is the feast
of St. Thomas Becket.
In his honor, a meditation
on tools and causation:
"Lewis Wolpert, an eminent developmental biologist at University College London, has just published
Six Impossible Things Before Breakfast, a pleasant, though rambling, look at the biological basis of belief. While the book focuses on our ability to form causal beliefs about everyday matters (the wind moved the trees, for example), it spends considerable time on the origins of religious and moral beliefs. Wolpert defends the unusual idea that causal thinking is an adaptation required for tool-making. Religious beliefs can thus be seen as
an odd extension of causal thinking about technology to more mysterious matters. Only a species that can reason causally could assert that 'this storm was sent by God because we sinned.' While Wolpert's attitude toward religion is tolerant, he's an atheist who seems to find religion more puzzling than absorbing."
— Review by H. Allen Orr in
The New York Review of Books,
Vol. 54, No. 1, January 11, 2007
"An odd extension"–
Wolpert's title is, of course,
from Lewis Carroll.
Related material:
"It's a poor sort of memory
that only works backwards."
— Through the Looking-Glass
An event at the Kennedy Center
broadcast on
December 26, 2006
(St. Steven's Day):
"Conductor John Williams, a 2004 Honoree, says, 'Steven, sharing our 34-year collaboration has been a great privilege for me. It's been an inspiration to watch you dream your dreams, nurture them and make them grow. And, in the process, entertain and edify billions of people around the world. Tonight we'd like to salute you, musically, with a piece that expresses that spirit beautifully … It was written by Leonard Bernstein, a 1980 Kennedy Center Honoree who was, incidentally, the first composer to be performed in this hall.' Backed by The United States Army Chorus and The Choral Arts Society, soprano Harolyn Blackwell and tenor Gregory Turay sing the closing number for Spielberg's tribute and the gala itself. It's the finale to the opera 'Candide,' 'Make Our Garden Grow,' and Williams conducts."
— CBS press release
See also the following,
from the conclusion to
"Mathematics and Narrative"
(Log24, Aug. 22, 2005):

"At times, bullshit can
only be countered
with superior bullshit."
— Norman Mailer
Many Worlds and Possible Worlds in Literature and Art, in Wikipedia:
"The concept of possible worlds dates back to at least Leibniz who in his Théodicée tries to justify the apparent imperfections of the world by claiming that it is optimal among all possible worlds. Voltaire satirized this view in his picaresque novel Candide….
Borges' seminal short story El jardín de senderos que se bifurcan ("The Garden of Forking Paths") is an early example of many worlds in fiction."
"Il faut cultiver notre jardin."
— Voltaire
"We symbolize
logical necessity
with the box ( )
)
and logical possibility
with the diamond ( )."
)."
— Keith Allen Korcz

"The possibilia that exist,
and out of which
the Universe arose,
are located in
a necessary being…."
— Michael Sudduth,
Notes on
God, Chance, and Necessity
by Keith Ward,
Regius Professor of Divinity,
Christ Church College, Oxford
(the home of Lewis Carroll)
For further details,
click on the
Christ Church diamond.
Comments Off on Friday December 29, 2006
Monday, December 18, 2006
Fade to Black:
Martin Gardner in the Notices of the American Mathematical Society, June/July 2005 (pdf):
“I did a column in Scientific American on minimal art, and I reproduced one of Ed Rinehart’s [sic] black paintings. Of course, it was just a solid square of pure black.”

Click on picture
for details.
The Notices of the American Mathematical Society, January 2007 (pdf):
“This was just one of the many moments in this sad tale when there were no whistle-blowers. As a result the entire profession has received a very public and very bad black mark.”
— Joan S. Birman
Professor Emeritus of Mathematics
Barnard College and
Columbia University
Comments Off on Monday December 18, 2006
Wednesday, December 6, 2006
Tompkins was found dead
on December 1, 2006.
From Log24 on that date:

That entry contained an excerpt from
Tom Wolfe’s The Painted Word—
“What I saw before me was the critic-in-chief of The New York Times saying: In looking at a painting today, ‘to lack a persuasive theory is to lack something crucial.’ I read it again. It didn’t say ‘something helpful’ or ‘enriching’ or even ‘extremely valuable.’ No, the word was crucial….”
The story deals with “one Gideon Summerfield, deceased.” Summerfield, a former tutor at (the fictional) St. Agatha’s College, Cambridge University, “is about to become the recipient of the Waymark prize. This prize is awarded in Mathematics and has the same prestige as the Nobel. Summerfield had a rather lackluster career at St. Agatha’s, with the exception of one remarkable result that he obtained. It is for this result that he is being awarded the prize, albeit posthumously.” Someone is apparently trying to prevent a biography of Summerfield from being published.
The following page contains
a critical part of the solution
to the mystery:
Comments Off on Wednesday December 6, 2006
Tuesday, October 24, 2006
Here is an interpretation
of those numbers:
"The geometrization conjecture, also known as Thurston's geometrization conjecture, concerns the geometric structure of compact 3-manifolds.
The geometrization conjecture can be considered an analogue for 3-manifolds of the uniformization theorem for surfaces. It was proposed by William Thurston in the late 1970s. It 'includes' other conjectures, such as the Poincaré conjecture and the Thurston elliptization conjecture."
The second sentence, in bold type, was added on 8/21 by yours truly. No deep learning or original thought was required to make this important improvement in the article; the sentence was simply copied from the then-current version of the article on Grigori Perelman (who has, it seems, proved the geometrization conjecture).
This may serve as an example of the "mathematics" part of the above phrase "Mathematics and Narrative" — a phrase which served, with associated links, as the Log24 entry for 8/21.
7/23 — Narrative:
"Each step in the story is a work of art, and the story as a whole is a sequence of episodes of rare beauty, a drama built out of nothing but numbers and imagination." –Freeman Dyson
This quotation appeared in the Log24 entry for 7/23, "Dance of the Numbers." What Dyson calls a "story" or "drama" is in fact mathematics. (Dyson calls the "steps" in the story "works of art," so it is clear that Dyson (a former student of G. H. Hardy) is discussing mathematical steps, not paragraphs in someone's account– perhaps a work of art, perhaps not– of mathematical history.) I personally regard the rhetorical trick of calling the steps leading to a mathematical result a "story" as contemptible vulgarization, but Dyson, as someone whose work (pdf) led to the particular result he is discussing, is entitled to dramatize it as he pleases.
For related material on mathematics, narrative, and vulgarization, click here.
The art of interpretation (applied above to a lottery) is relevant to narrative and perhaps also, in some sense, to the arts of mathematical research and exposition (if not to mathematics itself). This art is called hermeneutics.
For more on the subject, see the Stanford Encyclopedia of Philosophy article on Hans-Georg Gadamer, "the decisive figure in the development of twentieth-century hermeneutics."
"Foreword" in Gian-Carlo Rota,
Indiscrete Thoughts,
Boston: Birkhäuser Verlag,
1996, xiii-xvii, and
"Gadamer's Theory of Hermeneutics" in
The Philosophy of Hans-Georg Gadamer,
edited by Lewis E. Hahn,
The Library of Living Philosophers, Vol. 24,
Chicago: Open Court Publishers,
1997, 223-34.
Comments Off on Tuesday October 24, 2006
Wednesday, September 13, 2006
ART WARS continued:
The Krauss Cross

Rosalind Krauss in "Grids":
"If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World, for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.
Or, to take a more up-to-date example, we could think about Ad Reinhardt who, despite his repeated insistence that 'Art is art,' ended up by painting a series of black nine-square grids in which the motif that inescapably emerges is a Greek cross. There is no painter in the West who can be unaware of the symbolic power of the cruciform shape and the Pandora's box of spiritual reference that is opened once one uses it."
Rebecca Goldstein on
Mathematics and Narrative:
"I don't write exclusively on Jewish themes or about Jewish characters. My collection of short stories, Strange Attractors, contained nine pieces, five of which were, to some degree, Jewish, and this ratio has provided me with a precise mathematical answer (for me, still the best kind of answer) to the question of whether I am a Jewish writer. I am five-ninths a Jewish writer."
Jacques Maritain,
October 1941:
"The passion of Israel
today is taking on
more and more distinctly
the form of the Cross."
E. L. Doctorow,
City of God:
"In the garden of Adding,
Live Even and Odd."
Comments Off on Wednesday September 13, 2006
Thursday, August 31, 2006
Ingrid Thulin and
Glenn Ford in
“The 4 Horsemen
of the Apocalypse”:

A sneering review from TIME Magazine, March 23, 1962:
“Hero Ford, a playboy from Argentina, falls pampassionately in love with Heroine Thulin, a Parisienne married to a patriotic editor. When the editor joins the Resistance, the hero realizes his duty and secretly does the same. Unaware of his decision, the heroine decides that he is merely a lightweight, and goes back to her husband. At the fade, while the violins soar among the bomb bursts, the poor misunderstood playboy dies heroically in an attempt to weaken the Wehrmacht’s defenses in Normandy.
The tale is trite, the script clumsy, and the camera work grossly faked. Though the lovers wander all over Paris, the Cathedral of Notre Dame turns up in the background practically everywhere they go, almost as if it were following them around like a little dog.”
TIME Magazine is still wearing the Ivy League sneer it displayed so impressively in 1962.
A less dismissive summary from Answers.com:
“The World War I setting of the original Blasco-Ibanez novel has been updated to World War II, but the basic plot remains the same. A well-to-do Argentinian family, rent asunder by the death of patriarch Lee J. Cobb, scatters to different European countries in the late 1930s. Before expiring, Cobb had warned his nephew Carl Boehm that the latter’s allegiance to the Nazis would bring down the wrath of the titular Four Horsemen: War, Conquest, Famine and Death. Ford, Cobb’s grandson, has promised to honor his grandfather’s memory by thwarting the plans of Boehm. At the cost of his own life, Ford leads allied bombers to Boehm’s Normandy headquarters.”
In memory of Glenn Ford, a talented character actor who died at 90 yesterday, the opening paragraphs of an obituary in The Scotsman:

Screen icon Glenn Ford
dies at 90
RHIANNON EDWARD
GLENN Ford, one of the most enduring stars of the silver screen, has died at the age of 90.
Ford, who appeared in more than 200 films in a career spanning five decades, died at his home in Beverly Hills.
The actor’s health had been in decline for a number of years after he suffered a series of strokes.
Although he never achieved the superstardom he craved, Ford was widely acclaimed as one of the best character actors in the business.
The business of narrative:
From a narrative suggested by the name of The Scotsman‘s reporter and related, if only by association with Normandy, to Ford’s “Four Horsemen” film:
“The Vandaleurs are a family of Norman nobles with a heritable version of the mages’ Gift. They have been using magic covertly for what appears to have been a very long time…. Another branch of the family is known to hold a fief in Normandy, but it is not yet known if they are covert magicians as well.”
The Vandaleur narrative may be of interest to fans of The Da Vinci Code. (Ford is said to have been a Freemason, a charter member of Riviera Lodge No. 780, Pacific Palisades, California.)
For Catholics and others who prefer more traditional narratives:

Illuminated parchment,
1047 A.D.,
The Four Horsemen
of the Apocalypse
Related material:
Yesterday’s entries, and
an entry from April 7. 2003,
that they link to:

Comments Off on Thursday August 31, 2006
Monday, August 21, 2006
Comments Off on Monday August 21, 2006
Thursday, August 17, 2006
Special Topics
From a review by Liesl Schillinger in the Aug. 13 New York Times of a new novel by Marisha Pessl:
“… Special Topics in Calamity Physics tells the story of a wise newcomer who joins a circle of students who orbit a charismatic teacher with a tragic secret. The newcomer, a motherless waif named Blue van Meer, spent most of her life driving between college towns with her genius poli-sci professor father, Gareth…. Gareth is fond of making oracular statements, which his daughter laps up as if they were Churchill’s: ‘Everyone is responsible for the page-turning tempo of his or her Life Story,’ he tells her. And, he cautions, ‘never try to change the narrative structure of someone else’s story.’
…. Heeding Gareth van Meer’s dictum that the most page-turning read known to man is the collegiate curriculum, with its ‘celestial, sweet set of instructions, culminating in the scary wonder of the Final Exam,’ Pessl structures Blue’s mystery like a kind of Great Books class…. A professor is all-powerful, Gareth liked to tell his daughter, he puts ‘a veritable frame around life,’ and ‘organizes the unorganizable. Nimbly partitions it into modern and postmodern, renaissance, baroque, primitivism, imperialism and so on. Splice that up with Research Papers, Vacation, Midterms. All that order– simply divine.’ Blue’s syllabus also includes a murder or two. Her book’s last pages are a final exam. You will be relieved to learn it is mostly multiple choice, and there is no time limit.”
Multiple choice:
The examination below, taken from a page by a scholar at a Jesuit university, is on the Borges story “The Garden of Forking Paths”– a classic of multiple choice.
No time limit:
See the first question.
Examination on
“The Garden of Forking Paths“

“What is the meaning of the idea expressed by Yu Tsun that ‘everything happens to a man precisely, precisely now. Centuries of centuries and only in the present do things happen’? What is the significance of the emphasis on the present moment, the here and now? Is this related to the carpe diem (‘seize the day’) idea? How? How is the present effectively connected to the past and the future? How is the present associated simultaneously to choices, actions, and consequences? How is the present moment relevant to the idea of the ‘forking paths’? What is the symbolic meaning of forking paths when understood as a crossroads? What is a person confronted with when standing at a crossroads? What are the implications of a choice of road? May this be connected to the myth of Oedipus and its concerns with human choices and supposed predestination? What is suggested by the idea that ‘in all fictional works, each time a man is confronted with several alternatives, he chooses one and eliminates the others; in the fiction of Ts’ui Pen, he chooses– simultaneously– all of them. He creates, in this way, diverse futures, diverse times which themselves also proliferate and fork’? What does it mean to make all choices at once? What view of life do such beliefs embody?”
Saturday, July 29, 2006
Big Rock
Thanks to Ars Mathematica, a link to everything2.com:
“In mathematics, a big rock is a result which is vastly more powerful than is needed to solve the problem being considered. Often it has a difficult, technical proof whose methods are not related to those of the field in which it is applied. You say ‘I’m going to hit this problem with a big rock.’ Sard’s theorem is a good example of a big rock.”
Another example:
Properties of the Monster Group of R. L. Griess, Jr., may be investigated with the aid of the Miracle Octad Generator, or MOG, of R. T. Curtis. See the MOG on the cover of a book by Griess about some of the 20 sporadic groups involved in the Monster:
The MOG, in turn, illustrates (via Abstract 79T-A37, Notices of the American Mathematical Society, February 1979) the fact that the group of automorphisms of the affine space of four dimensions over the two-element field is also the natural group of automorphisms of an arbitrary 4×4 array.
This affine group, of order 322,560, is also the natural group of automorphisms of a family of graphic designs similar to those on traditional American quilts. (See the diamond theorem.)
This top-down approach to the diamond theorem may serve as an illustration of the “big rock” in mathematics.
For a somewhat simpler, bottom-up, approach to the theorem, see Theme and Variations.
For related literary material, see Mathematics and Narrative and The Diamond as Big as the Monster.
“The rock cannot be broken.
It is the truth.”
— Wallace Stevens,
“Credences of Summer”
Comments Off on Saturday July 29, 2006
Thursday, July 27, 2006
Number Sense
The NY lottery numbers for yesterday, 7/26, Jung's birthday, were 726 (mid-day) and 970 (evening).
We may view these numbers as representing the Jungian "sheep" and Freudian "goats" of yesterday's entry Partitions.
For the Jungian coincidence of 726 with 7/26, recall the NY lottery number 911 that was drawn on 9/11 exactly a year after the destruction of the World Trade Center. For more on this coincidence, see For Hemingway's Birthday: Mathematics and Narrative Continued (July 21, 2006).
For 970, Google reveals a strictly skeptical (i.e., like Freud, not Jung) meaning: 970 is the first page of the article "Sources of Mathematical Thinking," in Science, 7 May 1999: Vol. 284. no. 5416, pp. 970 – 974.
That article has been extensively cited in the scholarly literature on the psychology of mathematics. Its lead author, Stanislas Dehaene, has written a book, The Number Sense.
What sense, if any, is made by 726 and 970?
The mid-day number again (see Hemingway's birthday) illustrates the saying
"Time and chance happeneth to them all."
The evening number again illustrates the saying
"Though truth may be very hard to find in the pages of most books, the page numbers are generally reliable."
— Steven H. Cullinane,
Zen and Language Games
These sayings may suit the religious outlook of Susan Blackmore, source (along with Matthew 25:31-46) of the sheep/goats partition in yesterday's entry on that topic. She herself, apparently a former sheep, is now a goat practicing Zen.
Update of later the same evening–
On Space, Time, Life, the Universe, and Everything:
Note that the "sheep" number 726 has a natural interpretation as a date– i.e., in terms of time, while the "goat" number 970 has an interpretation as a page number– i.e., in terms of space. Rooting, like Jesus and St. Matthew, for the sheep, we may interpret both of today's NY lottery results as dates, as in the next entry, Real Numbers. That entry may (or may not) pose (and/or answer) The Ultimate Question. Selah.
Comments Off on Thursday July 27, 2006
Monday, July 24, 2006
Discourse Analysis
Edward Rothstein in today’s New York Times, reviewing Evil Incarnate (Princeton University Press):
“… the most decisive aspect of the myth is that it is, literally, a myth. Every single example of evil he gives turns out to be evil imagined: there is, he says, no evidence for any of it. Evil, he argues, is not something real, it is a ‘discourse,’ a ‘way of representing things and shaping our experience, not some force in itself.'”
Related material:
A review (pdf) by Steven G. Krantz of Charles Wells’s A Handbook of Mathematical Discourse (Notices of the American Mathematical Society, September 2004):
“Ambrose Bierce’s Devil’s Dictionary is a remarkable and compelling piece of writing because of its searing wit and sardonic take on life. Bierce does not define any new words. He instead gives deadly interpretations of very familiar words. Wells’s book does not fit into the same category of literary effort.”
For literary efforts perhaps more closely related to Bierce’s, see Mathematics and Narrative and the five Log24 entries ending on this date last year.
Comments Off on Monday July 24, 2006
Friday, July 21, 2006
For Hemingway’s birthday:
Mathematics and Narrative, continued
“We know many little things about the relation between mathematics and narrative, but lack one big comprehensive insight.”
— John Allen Paulos (pdf)
“On Wednesday, Sept. 11, 2002– 9/11/02– the New York State lottery numbers were 911, an eerie coincidence that set many people to thinking or, perhaps more accurately, to not thinking.”
— John Allen Paulos
“Time and chance happeneth to them all.”
— Ecclesiastes 9:11
Comments Off on Friday July 21, 2006
Sunday, July 16, 2006
Mathematics and Narrative
continued…
“Now, at the urging of the UC Berkeley cognitive linguist George Lakoff, liberal America’s guru of the moment, progressive Democrats are practicing to get their own reluctant mouths around some magical new vocabulary, in the hope of surviving and eventually overcoming the age of Bush.”
— Marc Cooper in The Atlantic Monthly, April 2005, “Thinking of Jackasses: The Grand Delusions of the Democratic Party”
Cooper’s “now” is apparently still valid. In today’s New York Times, the leftist Stanley Fish reviews Talking Right, by leftist Geoffrey Nunberg:
“… the right’s language is now the default language for everyone.
On the way to proposing a counterstrategy (it never really arrives), Nunberg pauses to engage in a polite disagreement with his fellow linguist George Lakoff, who has provided a rival account of the conservative ascendancy. Lakoff argues that Republicans have articulated– first for themselves and then for others– a conceptual framework that allows them to unite apparently disparate issues in a single coherent worldview … woven together not in a philosophically consistent framework but in a narrative ‘that creates an illusion of coherence.’
Once again, the Republicans have such a narrative– ‘declining patriotism and moral standards, the out-of-touch media and the self-righteous liberal elite … minorities demanding special privileges … disrespect for religious faith, a swollen government’– but ‘Democrats and liberals have not offered compelling narratives that could compete’ with it. Eighty pages later he is still saying the same thing. ‘The Democrats need a compelling narrative of their own.'”
Lakoff is the co-author of a book on the philosophy of mathematics, Where Mathematics Comes From: How the Embodied Mind Brings Mathematics into Being. From Wikipedia’s article on Lakoff:
“According to Lakoff, even mathematics itself is subjective to the human species and its cultures: thus ‘any question of math’s being inherent in physical reality is moot, since there is no way to know whether or not it is.’ Lakoff and Rafael E. Nunez (2000) argue at length that mathematical and philosophical ideas are best understood in light of the embodied mind. The philosophy of mathematics ought therefore to look to the current scientific understanding of the human body as a foundation ontology, and abandon self-referential attempts to ground the operational components of mathematics in anything other than ‘meat.'”
For a long list of related leftist philosophy, see The Thinking Meat Project.
Democrats seeking narratives may also consult The Carlin Code and The Prime Cut Gospel.
Comments Off on Sunday July 16, 2006
Sunday, July 2, 2006
The Rock and the Serpent
In a search for a title to express
the contrast between truth and lies,
an analogy between the phrases
“Crystal and Dragon” and
“Mathematics and Narrative“
suggests a similar phrase,
“The Rock and the Serpent.”
A web search for related titles leads to a book by Alice Thomas Ellis:
Serpent on the Rock: A Personal View of Christianity. (See a review.)
(This in turn leads to an article on Ellis’s husband, the late Colin Haycraft, publisher.)
For an earlier discussion of Ellis in this weblog, see Three Eleanors (March 12, 2005).
That entry brings us back to the theme of truth and lies with its link to an article from the Catholic publication Commonweal:
Getting to Truth by Lying.
Christians who wish to lie more effectively may consult a book by the author of the Commonweal article:

For a more sympathetic view of
suffering stemming from
Christian narrative,
see

(Click on cover
for details. See also Log24
entries on Guy Davenport,
who wrote the foreword.)
Comments Off on Sunday July 2, 2006
Monday, June 19, 2006
Snippets:
A Reply to John Updike
See Updike on digitized snippets.
The following four snippets were pirated from the end of MathPages Quotations, compiled by Kevin Brown.
They are of synchronistic interest in view of the previous two Log24 entries, which referred (implicitly) to a Poe story and (explicitly) to Pascal.
"That is another of your odd notions,"
said the Prefect, who had the fashion
of calling everything 'odd' that was
beyond his comprehension, and thus
lived amid an absolute legion of 'oddities.'
Edgar Allan Poe
I knew when seven justices could not
take up a quarrel, but when the parties
were met themselves, one of them
thought but of an If, as, 'If you said so,
then I said so'; and they shook hands
and swore brothers. Your If is the only
peacemaker; much virtue in If.
Shakespeare
I have made this letter longer than usual
because I lack the time to make it shorter.
Blaise Pascal
S'io credessi che mia risposta fosse
a persona che mai tornasse al mondo,
questa fiamma staria senza piu scosse.
Ma per cio che giammai di questo fondo
non torno vivo alcun, s'i'odo il vero,
senza tema d'infamia ti rispondo.
Dante, 1302
For translations of the Dante (including one by Dorothy Sayers), see everything2.com.
An anonymous author there notes that Dante describes a flame in which is encased a damned soul. The flame vibrates as the soul speaks:
If I thought that I were making
Answer to one that might return to view
The world, this flame should evermore
cease shaking.
But since from this abyss, if I hear true,
None ever came alive, I have no fear
Of infamy, but give thee answer due.
-- Dante, Inferno, Canto 27, lines 61-66,
translated by Dorothy Sayers
Updike says,
“Yes, there is a ton of information on the web but much of it is grievously inaccurate, unedited, unattributed and juvenile. The electronic marvels that abound around us serve, I have the impression, to inflame what is most informally and non-critically human about us. Our computer screens stare back at us with a kind of giant, instant aw-shucks, disarming in its modesty.”
Note Updike’s use of “inflame.”
For an aw-shucks version of “what is most informally and non-critically human about us,” as well as a theological flame, see both the previous entry and the above report from Hell.
Note that the web serves also to correct material that is inaccurate, unedited, unattributed, and juvenile. For examples, see Mathematics and Narrative. The combination of today’s entry for Pascal’s birthday with that web page serves both to light one candle and to curse the darkness.
Comments Off on Monday June 19, 2006
Monday, May 29, 2006
For John F. Kennedy's birthday:
Comments Off on Monday May 29, 2006
Monday, May 22, 2006
A Kind of Cross
Google Maps image
of the isle of Delos,
birthplace of Apollo:

“I faced myself that day with
the nonplused apprehension
of someone who has
come across a vampire
and has no crucifix in hand.”
— Joan Didion, “On Self-Respect,”
in Slouching Towards Bethlehem
“For every kind of vampire,
there is a kind of cross.”
— Thomas Pynchon,
Gravity’s Rainbow

Related material:
Mathematics and Narrative,
Secret Passages
Comments Off on Monday May 22, 2006
Friday, May 19, 2006
Star and Diamond
continued
” ‘I know what it is you last saw,’ she said; ‘for that is also in my mind. Do not be afraid! But do not think that only by singing amid the trees, nor even by the slender arrows of elvenbows, is this land of Lothlórien maintained and defended against the Enemy. I say to you, Frodo, that even as I speak to you, I perceive the Dark Lord and know his mind, or all his mind that concerns the Elves. And he gropes ever to see me and my thought. But still the door is closed!’
She lifted up her white arms, and spread out her hands towards the East in a gesture of rejection and denial. Eärendil, the Evening Star, most beloved of the Elves, shone clear above. So bright was it that the figure of the Elven-lady cast a dim shadow on the ground. Its ray glanced upon a ring about her finger; it glittered like polished gold overlaid with silver light, and a white stone in it twinkled as if the Even-star had come to rest upon her hand. Frodo gazed at the ring with awe; for suddenly it seemed to him that he understood.
‘Yes,’ she said, divining his thought, ‘it is not permitted to speak of it, and Elrond could not do so. But it cannot be hidden from the Ring-Bearer, and one who has seen the Eye. Verily it is in the land of Lórien upon the finger of Galadriel that one of the Three remains. This is Nenya, the Ring of Adamant, and I am its keeper.’ ”
— J. R. R. Tolkien, The Lord of the Rings
Comments Off on Friday May 19, 2006
Friday, May 5, 2006
Comments Off on Friday May 5, 2006
Thursday, May 4, 2006
First of all…

Comments Off on Thursday May 4, 2006
Tuesday, April 25, 2006
“There is a pleasantly discursive treatment
of Pontius Pilate’s unanswered question
‘What is truth?'”
— H. S. M. Coxeter, 1987, introduction to
Richard J. Trudeau’s remarks on
the “Story Theory” of truth
as opposed to
the “Diamond Theory” of truth
in The Non-Euclidean Revolution
A Serious Position
“‘Teitelbaum,’ in German,
is ‘date palm.'”
— Generations, Jan. 2003
“In Hasidism, a mystical brand
of Orthodox Judaism, the grand rabbi
is revered as a kinglike link to God….”
— Today’s New York Times obituary
of Rabbi Moses Teitelbaum,
who died on April 24, 2006
(Easter Monday in the
Orthodox Church)
NEW BOOKS: 02.16.05 Proofs and Paradoxes Alfred Teitelbaum changed his name to Tarski in the early 20s, the same time he changed religions, but when the Germans invaded his native Poland, the mathematician was in California, where he remained. His “great achievement was his
audacious assault on the notion of
truth,” says Martin Davis, focusing on the
semantics and syntax of scientific language.
Alfred Tarski: Life and Logic, co-written by a former student,
Solomon Feferman, offers “remarkably intimate information,” such as abusive teaching and “extensive amorous involvements.”
From Wikipedia, an unsigned story:
“In 1923 Alfred Teitelbaum and his brother Wacław changed their surnames to Tarski, a name they invented because it sounded very Polish, was simple to spell and pronounce, and was unused. (Years later, he met another Alfred Tarski in northern California.) The Tarski brothers also converted to Roman Catholicism, the national religion of the Poles. Alfred did so, even though he was an avowed atheist, because he was about to finish his Ph.D. and correctly anticipated that it would be difficult for a Jew to obtain a serious position in the new Polish university system.”
Comments Off on Tuesday April 25, 2006
Friday, March 31, 2006
Women's History Month continues…
Ontology Alignment
"He had with him a small red book of Mao's poems, and as he talked he squared it on the table, aligned it with the table edge first vertically and then horizontally. To understand who Michael Laski is you must have a feeling for that kind of compulsion."
— Joan Didion in the
Saturday Evening Post,
Nov. 18, 1967 (reprinted in
Slouching Towards Bethlehem)
"Or were you," I said.
He said nothing.
"Raised a Catholic," I said.
He aligned a square crystal paperweight with the edge of his desk blotter.
— Joan Didion in
The Last Thing He Wanted,
Knopf, 1996
"It was Plato who best expressed– who veritably embodied– the tension between the narrative arts and mathematics….
Plato clearly loved them both, both mathematics and poetry. But he approved of mathematics, and heartily, if conflictedly, disapproved of poetry. Engraved above the entrance to his Academy, the first European university, was the admonition: Oudeis ageometretos eiseto. Let none ignorant of geometry enter. This is an expression of high approval indeed, and the symbolism could not have been more perfect, since mathematics was, for Plato, the very gateway for all future knowledge. Mathematics ushers one into the realm of abstraction and universality, grasped only through pure reason. Mathematics is the threshold we cross to pass into the ideal, the truly real."
— Rebecca Goldstein,
Mathematics and
the Character of Tragedy
Comments Off on Friday March 31, 2006
Sunday, March 12, 2006
A Circle of Quiet
From the Harvard Math Table page:
“No Math table this week. We will reconvene next week on March 14 for a special Pi Day talk by Paul Bamberg.”
Some friends of mine are in this band.
They’re playing in a bar on Diversey,
way down the bill, around…
I said I’d be there.
Great.
They’re all in the math department.
They’re good.
They have this song called “i.”
You’d like it. Lowercase i.
They just stand there.
They don’t play anything for three minutes.
Imaginary number?
It’s a math joke.
You see why they’re way down the bill. |
From the April 2006 Notices of the American Mathematical Society, a footnote in a review by Juliette Kennedy (pdf) of Rebecca Goldstein’s Incompleteness:
4 There is a growing literature in the area of postmodern commentaries of [sic] Gödel’s theorems. For example, Régis Debray has used Gödel’s theorems to demonstrate the logical inconsistency of self-government. For a critical view of this and related developments, see Bricmont and Sokal’s Fashionable Nonsense [13]. For a more positive view see Michael Harris’s review of the latter, “I know what you mean!” [9]….
[9] MICHAEL HARRIS, “I know what you mean!,” http://www.math.jussieu.fr/~harris/Iknow.pdf.
[13] ALAN SOKAL and JEAN BRICMONT, Fashionable Nonsense, Picador, 1999.
Following the trail marked by Ms. Kennedy, we find the following in Harris’s paper:
“Their [Sokal’s and Bricmont’s] philosophy of mathematics, for instance, is summarized in the sentence ‘A mathematical constant like  doesn’t change, even if the idea one has about it may change.’ ( p. 263). This claim, referring to a ‘crescendo of absurdity’ in Sokal’s original hoax in Social Text, is criticized by anthropologist Joan Fujimura, in an article translated for IS*. Most of Fujimura’s article consists of an astonishingly bland account of the history of non-euclidean geometry, in which she points out that the ratio of the circumference to the diameter depends on the metric. Sokal and Bricmont know this, and Fujimura’s remarks are about as helpful as FN’s** referral of Quine’s readers to Hume (p. 70). Anyway, Sokal explicitly referred to “Euclid’s pi”, presumably to avoid trivial objections like Fujimura’s — wasted effort on both sides.32 If one insists on making trivial objections, one might recall that the theorem
doesn’t change, even if the idea one has about it may change.’ ( p. 263). This claim, referring to a ‘crescendo of absurdity’ in Sokal’s original hoax in Social Text, is criticized by anthropologist Joan Fujimura, in an article translated for IS*. Most of Fujimura’s article consists of an astonishingly bland account of the history of non-euclidean geometry, in which she points out that the ratio of the circumference to the diameter depends on the metric. Sokal and Bricmont know this, and Fujimura’s remarks are about as helpful as FN’s** referral of Quine’s readers to Hume (p. 70). Anyway, Sokal explicitly referred to “Euclid’s pi”, presumably to avoid trivial objections like Fujimura’s — wasted effort on both sides.32 If one insists on making trivial objections, one might recall that the theorem
that p is transcendental can be stated as follows: the homomorphism Q[X] –> R taking X to  is injective. In other words,
is injective. In other words,  can be identified algebraically with X, the variable par excellence.33
can be identified algebraically with X, the variable par excellence.33

More interestingly, one can ask what kind of object  was before the formal definition of real numbers. To assume the real numbers were there all along, waiting to be defined, is to adhere to a form of Platonism.34 Dedekind wouldn’t have agreed.35 In a debate marked by the accusation that postmodern writers deny the reality of the external world, it is a peculiar move, to say the least, to make mathematical Platonism a litmus test for rationality.36 Not that it makes any more sense simply to declare Platonism out of bounds, like Lévy-Leblond, who calls Stephen Weinberg’s gloss on Sokal’s comment ‘une absurdité, tant il est clair que la signification d’un concept quelconque est évidemment affectée par sa mise en oeuvre dans un contexte nouveau!’37 Now I find it hard to defend Platonism with a straight face, and I prefer to regard the formula
was before the formal definition of real numbers. To assume the real numbers were there all along, waiting to be defined, is to adhere to a form of Platonism.34 Dedekind wouldn’t have agreed.35 In a debate marked by the accusation that postmodern writers deny the reality of the external world, it is a peculiar move, to say the least, to make mathematical Platonism a litmus test for rationality.36 Not that it makes any more sense simply to declare Platonism out of bounds, like Lévy-Leblond, who calls Stephen Weinberg’s gloss on Sokal’s comment ‘une absurdité, tant il est clair que la signification d’un concept quelconque est évidemment affectée par sa mise en oeuvre dans un contexte nouveau!’37 Now I find it hard to defend Platonism with a straight face, and I prefer to regard the formula

as a creation rather than a discovery. But Platonism does correspond to the familiar experience that there is something about mathematics, and not just about other mathematicians, that precisely doesn’t let us get away with saying ‘évidemment’!38
32 There are many circles in Euclid, but no pi, so I can’t think of any other reason for Sokal to have written ‘Euclid’s pi,’ unless this anachronism was an intentional part of the hoax. Sokal’s full quotation was ‘the  of Euclid and the G of Newton, formerly thought to be constant and universal, are now perceived in their ineluctable historicity.’ But there is no need to invoke non-Euclidean geometry to perceive the historicity of the circle, or of pi: see Catherine Goldstein’s ‘L’un est l’autre: pour une histoire du cercle,’ in M. Serres, Elements d’histoire des sciences, Bordas, 1989, pp. 129-149.
of Euclid and the G of Newton, formerly thought to be constant and universal, are now perceived in their ineluctable historicity.’ But there is no need to invoke non-Euclidean geometry to perceive the historicity of the circle, or of pi: see Catherine Goldstein’s ‘L’un est l’autre: pour une histoire du cercle,’ in M. Serres, Elements d’histoire des sciences, Bordas, 1989, pp. 129-149.
33 This is not mere sophistry: the construction of models over number fields actually uses arguments of this kind. A careless construction of the equations defining modular curves may make it appear that pi is included in their field of scalars.
34 Unless you claim, like the present French Minister of Education [at the time of writing, i.e. 1999], that real numbers exist in nature, while imaginary numbers were invented by mathematicians. Thus  would be a physical constant, like the mass of the electron, that can be determined experimentally with increasing accuracy, say by measuring physical circles with ever more sensitive rulers. This sort of position has not been welcomed by most French mathematicians.
would be a physical constant, like the mass of the electron, that can be determined experimentally with increasing accuracy, say by measuring physical circles with ever more sensitive rulers. This sort of position has not been welcomed by most French mathematicians.
35 Cf. M. Kline, Mathematics The Loss of Certainty, p. 324.
36 Compare Morris Hirsch’s remarks in BAMS April 94.
37 IS*, p. 38, footnote 26. Weinberg’s remarks are contained in his article “Sokal’s Hoax,” in the New York Review of Books, August 8, 1996.
38 Metaphors from virtual reality may help here.”
* Earlier defined by Harris as “Impostures Scientifiques (IS), a collection of articles compiled or commissioned by Baudouin Jurdant and published simultaneously as an issue of the journal Alliage and as a book by La Découverte press.”
** Earlier defined by Harris as “Fashionable Nonsense (FN), the North American translation of Impostures Intellectuelles.”
What is the moral of all this French noise?
Perhaps that, in spite of the contemptible nonsense at last summer’s Mykonos conference on mathematics and narrative, stories do have an important role to play in mathematics — specifically, in the history of mathematics.
Despite his disdain for Platonism, exemplified in his remarks on the noteworthy connection of pi with the zeta function in the formula given above, Harris has performed a valuable service to mathematics by pointing out the excellent historical work of Catherine Goldstein. Ms. Goldstein has demonstrated that even a French nominalist can be a first-rate scholar. Her essay on circles that Harris cites in a French version is also available in English, and will repay the study of those who, like Barry Mazur and other Harvard savants, are much too careless with the facts of history. They should consult her “Stories of the Circle,” pp. 160-190 in A History of Scientific Thought, edited by Michel Serres, Blackwell Publishers (December 1995).
For the historically-challenged mathematicians of Harvard, this essay would provide a valuable supplement to the upcoming “Pi Day” talk by Bamberg.
For those who insist on limiting their attention to mathematics proper, and ignoring its history, a suitable Pi Day observance might include becoming familiar with various proofs of the formula, pictured above, that connects pi with the zeta function of 2. For a survey, see Robin Chapman, Evaluating Zeta(2) (pdf). Zeta functions in a much wider context will be discussed at next May’s politically correct “Women in Mathematics” program at Princeton, “Zeta Functions All the Way” (pdf).
Comments Off on Sunday March 12, 2006
Thursday, February 23, 2006
Cubist Epiphany

“In The Painted Word, a rumination on the state of American painting in the 1970s, Tom Wolfe described an epiphany….”
— Peter Berkowitz, “Literature in Theory”
“I had an epiphany.”
— Apostolos Doxiadis, organizer of last summer’s conference on mathematics and narrative. See the Log24 entry of 1:06 PM last August 23 and the four entries that preceded it.
“… das Durchleuchten des ewigen Glanzes des ‘Einen’ durch die materielle Erscheinung“
— A definition of beauty from Plotinus, via Werner Heisenberg
“By groping toward the light we are made to realize how deep the darkness is around us.”
— Arthur Koestler, The Call Girls: A Tragi-Comedy, Random House, 1973, page 118, quoted in The Shining of May 29
“Perhaps we are meant to see the story as a cubist retelling of the crucifixion….”
— Adam White Scoville, quoted in Cubist Crucifixion, on Iain Pears’s novel, An Instance of the Fingerpost
Related material:
Log24 entries of
Feb. 20, 21, and 22.
Comments Off on Thursday February 23, 2006
Thursday, February 16, 2006
Monolith
From James A. Michener‘s The Source:
“Trouble started in a quarter that neither Uriel nor Zadok could have foreseen. For many generations the wiser men of Zadok’s clan had worshipped El-Shaddai with the understanding that whereas Canaanites and Egyptians could see their gods directly, El-Shaddai was invisible and inhabited no specific place. Unequivocally the Hebrew patriarchs had preached this concept and the sager men of the clans accepted it, but to the average Hebrew who was not a philosopher the theory of a god who lived nowhere, who did not even exist in corporeal form, was not easy to comprehend. Such people were willing to agree with Zadok that their god did not live on this mountain– the one directly ahead– but they suspected that he did live on some mountain nearby, and when they said this they pictured an elderly man with a white beard who lived in a proper tent and whom they might one day see and touch. If questioned, they would have said that they expected El-Shaddai to look much like their father Zadok, but with a longer beard, a stronger voice, and more penetrating eyes.
Now, as these simpler-minded Hebrews settled down outside the walls of Makor, they began to see Canaanite processions leave the main gate and climb the mountain to the north, seeking the high place where Baal lived, and they witnessed the joy which men experienced when visiting their god, and the Hebrews began in subtle ways and easy steps to evolve the idea that Baal, who obviously lived in a mountain, and El-Shaddai, who was reported to do so, must have much in common. Furtively at first, and then openly, they began to climb the footpath to the place of Baal, where they found a monolith rising from the highest point of rock. Here was a tangible thing they could comprehend, and after much searching along the face of the mountain, a group of Hebrew men found a straight rock of size equal to the one accorded Baal, and with much effort they dragged it one starless night to the mountain top, where they installed it not far from the home of Baal….”
Rabbi Chitrik died on
Valentine’s Day, 2006,
having had a heart attack
on Feb. 8, 2006–

Grammy Night.

The above monolith is perhaps more
closely related to El-Shaddai than to
Madonna, Grammy Night, and Baal.
It reflects my own interests
(Mathematics and Narrative)
and those of Martin Buber
(Jews on Fiction):
“Among Buber’s early philosophical influences were Kant’s
Prolegomena, which he read at the age of fourteen, and Nietzsche’s
Zarathustra. Whereas Kant had a calming influence on the young mind troubled by the
aporia of infinite versus finite time, Nietzsche’s doctrine of ‘the eternal recurrence of the same’ constituted a powerful negative seduction. By the time Buber graduated from Gymnasium he felt he had overcome this seduction, but Nietzsche’s prophetic tone and aphoristic style are evident in Buber’s subsequent writings.”
Monday, February 6, 2006
The Diamond Theory of Truth
“Legend says that when the stones
are brought together the diamonds
inside of them will glow.”
— Harrison Ford in
“Indiana Jones and the
Temple of Doom”
In today’s online New York Times:
(1) A review of pop-archaeology TV,
“Digging for the Truth,”
(2) a Sunday news story,
“Looking for the Lie,”
(3) and a profile,
“Storyteller in the Family.”
From (1):
“The season premiere ‘Digging for the Truth: The Real Temple of Doom,’ showed Mr. Bernstein in South America, exploring tunnels….”
From (2):
“… scientists are building a cognitive theory of deception to show what lying looks like….”
From (3):
“I did feel one had to get not just the facts, but the emotional underpinnings.”
Comments Off on Monday February 6, 2006
Sunday, January 22, 2006
Comments Off on Sunday January 22, 2006
Saturday, January 21, 2006
Jews on Fiction
See Tony Kushner and E.L. Doctorow in today’s New York Times, Rebecca Goldstein’s talk from last summer’s Mykonos conference on mathematics and narrative, and Martin Buber on the Bible.
Comments Off on Saturday January 21, 2006
Monday, January 16, 2006
Mathematics and Narrative
Rebecca Goldstein, Mathematics and the Character of Tragedy:
“It was Plato who best expressed– who veritably embodied– the tension between the narrative arts and mathematics.”
Veritably.
Comments Off on Monday January 16, 2006
Friday, December 16, 2005
Jesus vs. the Goddess:
A Brief Chronology
In 1946, Robert Graves published “King Jesus, an historical novel based on the theory and Graves’ own historical conjecture that Jesus was, in fact, the rightful heir to the Israelite throne… written while he was researching and developing his ideas for The White Goddess.”
In 1948, C. S. Lewis finished the first draft of The Lion, The Witch, and The Wardrobe, a novel in which one of the main characters is “the White Witch.”
In 1948, Robert Graves published The White Goddess.
In 1949, Robert Graves published Seven Days in New Crete [also titled Watch the North Wind Rise], “a novel about a social distopia in which Goddess worship is (once again?) the dominant religion.”
Lewis died on November 22, 1963, the day John F. Kennedy was killed.
Related material:
Log24, December 10, 2005
Graves died on December 7 (Pearl Harbor Day), 1985.
Related material:
Log24, December 7, 2005, and
Log24, December 11, 2005
Jesus died, some say, on April 7 in the year 30 A.D.
Related material:
Art Wars, April 7, 2003:
Geometry and Conceptual Art,
Eight is a Gate, and

Plato’s Diamond

— Motto of
Plato’s Academy
“Plato is wary of all forms of rapture other than reason’s. He is most deeply leery of, because himself so susceptible to, the literary imagination. He speaks of it as a kind of holy madness or intoxication and goes on to link it to Eros, another derangement that joins us, but very dangerously, with the gods.”
“It’s all in Plato, all in Plato;
bless me, what do they
teach them at these schools?”
— C. S. Lewis in
the Narnia Chronicles
“How much story do you want?”
— George Balanchine
Sunday, December 11, 2005
Intelligence/
Counterintelligence
continued:
Intelligence: A file on James Jesus Angleton at namebase.org, a site run by Daniel Brandt.
Counterintelligence: Hollywood on James Jesus Angleton–
"From a screenplay by 'Forrest Gump' screenwriter Eric Roth, 'The Good Shepherd' tells the mostly true story of James Wilson (a character reported to be based on legendary CIA spymaster James Jesus Angleton, and played in the film by Matt Damon), one of the founding members of the Central Intelligence Agency. Beginning as an scholar at Yale, the film follows Wilson as he is recruited to join the secret Skull and Bones fraternity, a brotherhood and breeding ground for future world leaders, where his acute mind, spotless reputation and sincere belief in the American way of life render him a prime candidate for a career in intelligence."
— Edward Havens, FilmJerk.com, 8/30/2005
The Forrest Gump Award goes to Good Will Hunting* for this choice of roles.
Counterintelligence
illustrated:

Forrest Gump (l.)
and JFK (r.)
* See Log24, April 4, 2003, Mathematics Awareness Month. For some related material, see Mathematics and Narrative.
Comments Off on Sunday December 11, 2005
Wednesday, November 30, 2005
For St. Andrew’s Day
“The miraculous enters…. When we investigate these problems, some fantastic things happen….”
— John H. Conway and N. J. A. Sloane, Sphere Packings, Lattices and Groups, preface to first edition (1988)
The remarkable Mathieu group M24, a group of permutations on 24 elements, may be studied by picturing its action on three interchangeable 8-element “octads,” as in the “Miracle Octad Generator” of R. T. Curtis.
A picture of the Miracle Octad Generator, with my comments, is available online.
Comments Off on Wednesday November 30, 2005
Friday, November 18, 2005
It’s still the same old story,
a fight for love and…
Glory
Wikipedia on the tesseract:
“Glory Road (1963) included the
foldbox, a hyperdimensional packing case that was bigger inside than outside.”
Robert A. Heinlein in Glory Road:
“Rufo’s baggage turned out to be a little black box about the size and shape of a portable typewriter. He opened it.
And opened it again.
And kept on opening it– And kept right on unfolding its sides and letting them down until the durn thing was the size of a small moving van and even more packed….
… Anyone who has studied math knows that the inside does not have to be smaller than the outside, in theory…. Rufo’s baggage just carried the principle further.”
Johnny Cash: “And behold, a white horse.”
On The Last Battle, a book in the Narnia series by C. S. Lewis:
“… there is much glory in this wonderfully written apocalypse. Tirian, looking into the stable through the hole in the door, says, ‘The stable seen from within and the stable seen from without are two different places.’ Digory answers, ‘Its inside is bigger than its outside.’ It is the perceptive Lucy who voices the hope that is in us, ‘In our world, too, a stable once had something inside it that was bigger than our whole world.'”
Lewis said in “The Weight of Glory”—
“Do you think I am trying to weave a spell? Perhaps I am; but remember your fairy tales. Spells are used for breaking enchantments as well as for inducing them.”
On enchantments that need to be broken:
See the description of the Eater of Souls in Glory Road and of Scientism in
Comments Off on Friday November 18, 2005
Saturday, November 12, 2005
Glory Season
"…his eyes ranged the Consul's books disposed quite neatly… on high shelves around the walls: Dogme et Ritual de la Haute Magie, Serpent and Siva Worship in Central America, there were two long shelves of this, together with the rusty leather bindings and frayed edges of the numerous cabbalistic and alchemical books, though some of them looked fairly new, like the Goetia of the Lemegaton of Solomon the King, probably they were treasures, but the rest were a heterogeneous collection…."
— Malcolm Lowry, Under the Volcano, Chapter VI
"… when Saul does reach for a slim leather-bound volume Eliza cannot help but feel that something momentous is about to happen. There is care in the way he carries the book on the short journey from its shelf, as if it were constructed not of leather and parchment but of flesh and blood….
"Otzar Eden HaGanuz," Saul says. "The Hidden Eden. In this book, Abulafia describes the process of permutation…. Once you have mastered it, you will have mastered words, and once you have mastered words, you will be ready to receive shefa."
— Bee Season: A Novel
"In the Inner Game, we call the Game Dhum Welur, the Mind of God."
— The Gameplayers of Zan, a novel featuring games based on cellular automata
"Regarding cellular automata, I'm trying to think in what SF books I've seen them mentioned. Off the top of my head, only three come to mind:
The Gameplayers of Zan M.A. Foster
Permutation City Greg Egan
Glory Season David Brin"
— Jonathan L. Cunningham, Usenet
"If all that 'matters' are fundamentally mathematical relationships, then there ceases to be any important difference between the actual and the possible. (Even if you aren't a mathematical Platonist, you can always find some collection of particles of dust to fit any required pattern. In Permutation City this is called the 'logic of the dust' theory.)….
… Paul Durham is convinced by the 'logic of the dust' theory mentioned above, and plans to run, just for a few minutes, a complex cellular automaton (Permutation City) started in a 'Garden of Eden' configuration — one which isn't reachable from any other, and which therefore must have been the starting point of a simulation…. I didn't understand the need for this elaborate set-up, but I guess it makes for a better story than 'well, all possible worlds exist, and I'm going to tell you about one of them.'"
— Danny Yee, review of Permutation City
"Y'know, I never imagined the competition version involved so many tricky permutations."
— David Brin, Glory Season, 1994 Spectra paperback, p. 408
Related material:

|
"… matter is consciousness expressed in the intermixing of force and form, but so heavily structured and constrained by form that its behaviour becomes describable using the regular and simple laws of physics. This is shown in Figure 2.
The glyph in Figure 2 is the basis for a kabbalistic diagram called the Etz Chaiim, or Tree of Life. The first principle of being or consciousness is called Keter, which means Crown. The raw energy of consciousness is called Chokhmah or Wisdom, and the capacity to give form to the energy of consciousness is called Binah, which is sometimes translated as Understanding, and sometimes as Intelligence. The outcome of the interaction of force and form, the physical world, is called Malkhut or Kingdom. This is shown… in Figure 3."
|
Figure 3
"This quaternary is a Kabbalistic representation of God-the-Knowable, in the sense that it the most abstract representation of God we are capable of comprehending….
God-the-Knowable has four aspects, two male and two female: Keter and Chokhmah are both represented as male, and Binah and Malkhut are represented as female. One of the titles of Chokhmah is Abba, which means Father, and one of the titles of Binah is Imma, which means Mother, so you can think of Chokhmah as God-the-Father, and Binah as God-the-Mother. Malkhut is the daughter, the female spirit of God-as-Matter, and it would not be wildly wrong to think of her as Mother Earth. And what of God-the-Son? Is there also a God-the-Son in Kabbalah? There is…."
— A Depth of Beginning: Notes on Kabbalah by Colin Low (pdf)
|
See also
Cognitive Blending and the Two Cultures,
Mathematics and Narrative,
Deep Game,
and the previous entry.
Comments Off on Saturday November 12, 2005
Wednesday, September 28, 2005
Mathematical Narrative,
continued:
There is a pleasantly discursive treatment
of Pontius Pilate’s unanswered question
“What is truth?”
— H. S. M. Coxeter, introduction to
Richard J. Trudeau’s
The Non-Euclidean Revolution
“People have always longed for truths about the world — not logical truths, for all their utility; or even probable truths, without which daily life would be impossible; but informative, certain truths, the only ‘truths’ strictly worthy of the name. Such truths I will call ‘diamonds’; they are highly desirable but hard to find….The happy metaphor is Morris Kline’s in Mathematics in Western Culture (Oxford, 1953), p. 430.”
— Richard J. Trudeau,
The Non-Euclidean Revolution,
Birkhauser Boston,
1987, pages 114 and 117
“A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes…. My own viewpoint is the Story Theory…. I concluded long ago that each enterprise contains only stories (which the scientists call ‘models of reality’). I had started by hunting diamonds; I did find dazzlingly beautiful jewels, but always of human manufacture.”
— Richard J. Trudeau,
The Non-Euclidean Revolution,
Birkhauser Boston,
1987, pages 256 and 259
An example of
the story theory of truth:

Actress Gwyneth Paltrow (“Proof”) was apparently born on either Sept. 27, 1972, or Sept. 28, 1972. Google searches yield “about 193” results for the 27th and “about 610” for the 28th.
Those who believe in the “story theory” of truth may therefore want to wish her a happy birthday today. Those who do not may prefer the contents of yesterday’s entry, from Paltrow’s other birthday.
Comments Off on Wednesday September 28, 2005
Tuesday, September 27, 2005
Mathematical Narrative
Gwyneth Paltrow is said to be 33 today.
Tuesday, August 30, 2005
Comments Off on Tuesday August 30, 2005
Monday, August 22, 2005
The Hole
Part I: Mathematics and Narrative

Apostolos Doxiadis on last month's conference on "mathematics and narrative"–
Doxiadis is describing how talks by two noted mathematicians were related to
"… a sense of a 'general theory bubbling up' at the meeting… a general theory of the deeper relationship of mathematics to narrative…. "
Doxiadis says both talks had "a big hole in the middle."
"Both began by saying something like: 'I believe there is an important connection between story and mathematical thinking. So, my talk has two parts. [In one part] I’ll tell you a few things about proofs. [And in the other part] I’ll tell you about stories.' …. And in both talks it was in fact implied by a variation of the post hoc propter hoc, the principle of consecutiveness implying causality, that the two parts of the lectures were intimately related, the one somehow led directly to the other."
"And the hole?"
"This was exactly at the point of the link… [connecting math and narrative]… There is this very well-known Sidney Harris cartoon… where two huge arrays of formulas on a blackboard are connected by the sentence ‘THEN A MIRACLE OCCURS.’ And one of the two mathematicians standing before it points at this and tells the other: ‘I think you should be more explicit here at step two.’ Both… talks were one half fascinating expositions of lay narratology– in fact, I was exhilarated to hear the two most purely narratological talks at the meeting coming from number theorists!– and one half a discussion of a purely mathematical kind, the two parts separated by a conjunction roughly synonymous to ‘this is very similar to this.’ But the similarity was not clearly explained: the hole, you see, the ‘miracle.’ Of course, both [speakers]… are brilliant men, and honest too, and so they were very clear about the location of the hole, they did not try to fool us by saying that there was no hole where there was one."
Part II: Possible Worlds
"At times, bullshit can only be countered with superior bullshit."
— Norman Mailer
Many Worlds and Possible Worlds in Literature and Art, in Wikipedia:
"The concept of possible worlds dates back to a least Leibniz who in his Théodicée tries to justify the apparent imperfections of the world by claiming that it is optimal among all possible worlds. Voltaire satirized this view in his picaresque novel Candide….
Borges' seminal short story El jardín de senderos que se bifurcan ("The Garden of Forking Paths") is an early example of many worlds in fiction."
Background:
Modal Logic in Wikipedia
Possible Worlds in Wikipedia
Possible-Worlds Theory, by Marie-Laure Ryan
(entry for The Routledge Encyclopedia of Narrative Theory)
The God-Shaped Hole
Part III: Modal Theology
"'What is this Stone?' Chloe asked….
'…It is told that, when the Merciful One made the worlds, first of all He created that Stone and gave it to the Divine One whom the Jews call Shekinah, and as she gazed upon it the universes arose and had being.'"
— Many Dimensions, by Charles Williams, 1931 (Eerdmans paperback, April 1979, pp. 43-44)
"The lapis was thought of as a unity and therefore often stands for the prima materia in general."
— Aion, by C. G. Jung, 1951 (Princeton paperback, 1979, p. 236)
"Its discoverer was of the opinion that he had produced the equivalent of the primordial protomatter which exploded into the Universe."
"We symbolize
logical necessity
with the box ( ) )
and logical possibility
with the diamond ( ). )."
— Keith Allen Korcz

"The possibilia that exist,
and out of which
the Universe arose,
are located in
a necessary being…."
— Michael Sudduth,
Notes on
God, Chance, and Necessity
by Keith Ward,
Regius Professor of Divinity
at Christ Church College, Oxford
(the home of Lewis Carroll)
|
Comments Off on Monday August 22, 2005
Friday, August 19, 2005
Mathematics and Narrative
continued
"There is a pleasantly discursive treatment of Pontius Pilate's unanswered question 'What is truth?'"
— H. S. M. Coxeter, 1987, introduction to Richard J. Trudeau's remarks on the "Story Theory" of truth as opposed to the "Diamond Theory" of truth " in The Non-Euclidean Revolution
"I had an epiphany: I thought 'Oh my God, this is it! People are talking about elliptic curves and of course they think they are talking mathematics. But are they really? Or are they talking about stories?'"
— An organizer of last month's "Mathematics and Narrative" conference
"A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the 'Story Theory' of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called 'true.' The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes*…."
— Richard J. Trudeau in The Non-Euclidean Revolution
"'Deniers' of truth… insist that each of us is trapped in his own point of view; we make up stories about the world and, in an exercise of power, try to impose them on others."
— Jim Holt in this week's New Yorker magazine. Click on the box below.

* Many stripes —
"What disciplines were represented at the meeting?"
"Apart from historians, you mean? Oh, many: writers, artists, philosophers, semioticians, cognitive psychologists – you name it."
— An organizer of last month's "Mathematics and Narrative" conference
Comments Off on Friday August 19, 2005
Thursday, August 18, 2005
Comments Off on Thursday August 18, 2005
Tuesday, August 16, 2005
Narrative and Latin Squares
From The Independent, 15 August 2005:
“Millions of people now enjoy Sudoku puzzles. Forget the pseudo-Japanese baloney: sudoku grids are a version of the Latin Square created by the great Swiss mathematician Leonhard Euler in the late 18th century.”
The Independent was discussing the conference on “Mathematics and Narrative” at Mykonos in July.
From the Wikipedia article on Latin squares:
“The popular Sudoku puzzles are a special case of Latin squares; any solution to a Sudoku puzzle is a Latin square. Sudoku imposes the additional restriction that 3×3 subgroups must also contain the digits 1–9 (in the standard version).
The Diamond 16 Puzzle illustrates a generalized concept of Latin-square orthogonality: that of “orthogonal squares” (Diamond Theory, 1976) or “orthogonal matrices”– orthogonal, that is, in a combinatorial, not a linear-algebra sense (A. E. Brouwer, 1991).”
This last paragraph, added to Wikipedia on Aug. 14, may or may not survive the critics there.
Comments Off on Tuesday August 16, 2005
Friday, July 29, 2005
Anatomy of a Death
From today's New York Times:

From the Washington Post:
"Al Held, an American artist who painted large-scale abstract works… was found dead July 27, floating in a swimming pool at his villa…. The cause of death was not reported, but Italian police said he died of natural causes. He was 76."
From the Associated Press,
filed at 4:34 PM ET July 27, 2005:
"Held once described his work this way: 'Historically, the priests and wise men believed that it was the artist's job to make images of heaven and hell believable, even though nobody had experienced these places.'
'Today,' he went on, 'scientists talk about vast worlds and universes that the senses cannot experience. The purpose of the nonobjective artist is to create these images.'"
Another view:
"Most modern men do not believe in hell because they have not been there."
— Review of Malcolm Lowry's novel Under the Volcano (1947)
Related material:
The Four Last Things.
Hollywood images:

Comments Off on Friday July 29, 2005
Wednesday, July 27, 2005
Comments Off on Wednesday July 27, 2005
Friday, July 22, 2005
By Their Fruits
Today's birthdays:
Don Henley and Willem Dafoe

Related material:
Mathematics and Narrative,
Crankbuster.
"And the fruit is rotten;
the serpent's eyes shine
as he wraps around the vine
in the Garden of Allah."
Comments Off on Friday July 22, 2005
Wednesday, July 20, 2005
Beaming Scotty Up
From crankbuster, July 18:
“Do not underestimate Evil Cullinane’s plan for World Domination! http://www.log24.com now shows that he has crossed over to the dark side, making sacrifices to the Ancient Hindu Goddess ‘Kalli’ to ward off our attacks! ‘Kalli’-nane will soon appear as the top result on every Google search.

July 20 illustration of
crankbuster’s remarks Soon, all young mathematicians will be hypnotised by his dark diamonds of falsehood. At least, that’s his plan. But wait, who’s that brilliant mathematician who shines the light right through Cullinane’s fraud and exposes him to the whole world?! Crankbuster saves the day! (applause)”
From Log24, July 18:
Is Beauty the Beast?
(
Headline in Christianity Today)
“In Hindu mythology,
Kali, the Divine Mother, is the symbol for the infinite diversity of experience.
Kali represents the entire physical plane. She is the drama, tragedy, humor, and sorrow of life. She is the brother, father, sister, mother, lover, and friend. She is the fiend, monster, beast, and brute.”
— Gary Zukav, Harvard ’64
Star Trek’s “Scotty,” who died
at 5:30 AM PDT July 20, was “a veteran of the D-Day landings who managed to hide a war injury on screen. As an artillery lieutenant in the Canadian army, he was hit by six machine-gun bullets, one of which removed his middle right finger.”

Comments Off on Wednesday July 20, 2005
Sunday, July 10, 2005
| From Artemiadis’s website: |
| 1986: |
Elected Regular Member
of the Academy of Athens |
| 1999: |
Vice President
of the Academy of Athens |
| 2000: |
President
of the Academy of Athens |

“First of all, I’d like to
thank the Academy…”
— remark attributed to Plato
Comments Off on Sunday July 10, 2005
Friday, June 24, 2005
Geometry for Jews
continued:

People have tried in many ways
to
bridge the gap
between themselves and God….
No bridge reaches God, except one…
God's
Bridge: The Cross
— Billy Graham Evangelistic Association,
according to messiahpage.com
"… just as God defeats the devil:
this bridge exists;
it is the theory of the field
of algebraic functions over
a finite field of constants
(that is to say, a finite number
of elements: also said to be a Galois
field, or earlier 'Galois imaginaries'
because Galois first defined them
and studied them….)"
— André Weil, 1940 letter to his sister,
Simone Weil, alias Simone Galois
(see previous entry)
Related material:
Billy Graham and the City:
A Later Look at His Words
— New York Times, June 24, 2005
Geometry for Jews
and other art notes
Comments Off on Friday June 24, 2005
Thursday, June 23, 2005
Mathematics and Metaphor
The current (June/July) issue of the Notices of the American Mathematical Society has two feature articles. The first, on the vulgarizer Martin Gardner, was dealt with here in a June 19 entry, Darkness Visible. The second is related to a letter of André Weil (pdf) that is in turn related to mathematician Barry Mazur’s attempt to rewrite mathematical history and to vulgarize other people’s research by using metaphors drawn, it would seem, from the Weil letter.
A Mathematical Lie conjectures that Mazur’s revising of history was motivated by a desire to dramatize some arcane mathematics, the Taniyama conjecture, that deals with elliptic curves and modular forms, two areas of mathematics that have been known since the nineteenth century to be closely related.
Mazur led author Simon Singh to believe that these two areas of mathematics were, before Taniyama’s conjecture of 1955, completely unrelated —
“Modular forms and elliptic equations live in completely different regions of the mathematical cosmos, and nobody would ever have believed that there was the remotest link between the two subjects.” — Simon Singh, Fermat’s Enigma, 1998 paperback, p. 182
This is false. See Robert P. Langlands, review of Elliptic Curves, by Anthony W. Knapp, Bulletin of the American Mathematical Society, January 1994.
It now appears that Mazur’s claim was in part motivated by a desire to emulate the great mathematician André Weil’s manner of speaking; Mazur parrots Weil’s “bridge” and “Rosetta stone” metaphors —
From Peter Woit’s weblog, Feb. 10, 2005:
“The focus of Weil’s letter is the analogy between number fields and the field of algebraic functions of a complex variable. He describes his ideas about studying this analogy using a third, intermediate subject, that of function fields over a finite field, which he thinks of as a ‘bridge‘ or ‘Rosetta stone.'”
In “A 1940 Letter of André Weil on Analogy in Mathematics,” (pdf), translated by Martin H. Krieger, Notices of the A.M.S., March 2005, Weil writes that
“The purely algebraic theory of algebraic functions in any arbitrary field of constants is not rich enough so that one might draw useful lessons from it. The ‘classical’ theory (that is, Riemannian) of algebraic functions over the field of constants of the complex numbers is infinitely richer; but on the one hand it is too much so, and in the mass of facts some real analogies become lost; and above all, it is too far from the theory of numbers. One would be totally obstructed if there were not a bridge between the two. And just as God defeats the devil: this bridge exists; it is the theory of the field of algebraic functions over a finite field of constants….
On the other hand, between the function fields and the ‘Riemannian’ fields, the distance is not so large that a patient study would not teach us the art of passing from one to the other, and to profit in the study of the first from knowledge acquired about the second, and of the extremely powerful means offered to us, in the study of the latter, from the integral calculus and the theory of analytic functions. That is not to say that at best all will be easy; but one ends up by learning to see something there, although it is still somewhat confused. Intuition makes much of it; I mean by this the faculty of seeing a connection between things that in appearance are completely different; it does not fail to lead us astray quite often. Be that as it may, my work consists in deciphering a trilingual text {[cf. the Rosetta Stone]}; of each of the three columns I have only disparate fragments; I have some ideas about each of the three languages: but I know as well there are great differences in meaning from one column to another, for which nothing has prepared me in advance. In the several years I have worked at it, I have found little pieces of the dictionary. Sometimes I worked on one column, sometimes under another.”
Here is another statement of the Rosetta-stone metaphor, from Weil’s translator, Martin H. Krieger, in the A.M.S. Notices of November 2004, “Some of What Mathematicians Do” (pdf):
“Weil refers to three columns, in analogy with the Rosetta Stone’s three languages and their arrangement, and the task is to ‘learn to read Riemannian.’ Given an ability to read one column, can you find its translation in the other columns? In the first column are Riemann’s transcendental results and, more generally, work in analysis and geometry. In the second column is algebra, say polynomials with coefficients in the complex numbers or in a finite field. And in the third column is arithmetic or number theory and combinatorial properties.”
For greater clarity, see Armand Borel (pdf) on Weil’s Rosetta stone, where the three columns are referred to as Riemannian (transcendental), Italian (“algebraico-geometric,” over finite fields), and arithmetic (i.e., number-theoretic).
From Fermat’s Enigma, by Simon Singh, Anchor paperback, Sept. 1998, pp. 190-191:
Barry Mazur: “On the one hand you have the elliptic world, and on the other you have the modular world. Both these branches of mathematics had been studied intensively but separately…. Than along comes the Taniyama-Shimura conjecture, which is the grand surmise that there’s a bridge between these two completely different worlds. Mathematicians love to build bridges.”
Simon Singh: “The value of mathematical bridges is enormous. They enable communities of mathematicians who have been living on separate islands to exchange ideas and explore each other’s creations…. The great potential of the Taniyama-Shimura conjecture was that it would connect two islands and allow them to speak to each other for the first time. Barry Mazur thinks of the Taniyama-Shimura conjecture as a translating device similar to the Rosetta stone…. ‘It’s as if you know one language and this Rosetta stone is going to give you an intense understanding of the other language,’ says Mazur. ‘But the Taniyama-Shimura conjecture is a Rosetta stone with a certain magical power.'”
If Mazur, who is scheduled to speak at a conference on Mathematics and Narrative this July, wants more material on stones with magical powers, he might consult The Blue Matrix and The Diamond Archetype.
Comments Off on Thursday June 23, 2005
Sunday, June 12, 2005
Comments Off on Sunday June 12, 2005
Saturday, June 11, 2005
Birthday Links
Today’s birthdays:
Gene Wilder and Adrienne Barbeau.
For Gene:
A discussion of Frankenstein as
The Modern Prometheus at
Mathematics and Narrative.
For Adrienne:
Chinese Arithmetic.
Comments Off on Saturday June 11, 2005
Thursday, May 26, 2005
Drama of the Diagonal
"The beautiful in mathematics
resides in contradiction.
Incommensurability, logoi alogoi, was
the first splendor in mathematics."
— Simone Weil, Oeuvres Choisies,
éd. Quarto , Gallimard, 1999, p. 100

Logos Alogos
by S. H. Cullinane
"To a mathematician, mathematical entities have their own existence, they habitate spaces created by their intention. They do things, things happen to them, they relate to one another. We can imagine on their behalf all sorts of stories, providing they don't contradict what we know of them. The drama of the diagonal, of the square…"
— Dennis Guedj, abstract of "The Drama of Mathematics," a talk to be given this July at the Mykonos conference on mathematics and narrative.
For the drama of the diagonal of the square, see
Comments Off on Thursday May 26, 2005
Wednesday, May 25, 2005
The Turning
Readers who have an Amazon.com account may view book pages relevant to the previous entry. See page 77 of The Way We Think, by Fauconnier and Turner (Amazon search term = Meno). This page discusses both the Pythagorean theorem and Plato's diamond figure in the Meno, but fails to "blend" these two topics. See also page 53 of The History of Mathematics, by Roger Cooke (first edition), where these two topics are in fact blended (Amazon search term = Pythagorean). The illustration below is drawn from the Cooke book.
Cooke demonstrates how the Pythagorean theorem might have been derived by "blending" Plato's diamond (left) with the idea of moving the diamond's corners (right).
The previous entry dealt with a conference on mathematics and narrative. Above is an example I like of mathematics…. Here is an example I like of narrative:
Kate felt quite dizzy. She didn't know exactly what it was
that had just happened, but she felt pretty damn certain that
it was the sort of experience that her mother would not have
approved of on a first date.
"Is this all part of what we have to do to go to Asgard?"
she said. "Or are you just fooling around?"
"We will go to Asgard...now," he said.
At that moment he raised his hand as if to pluck an apple,
but instead of plucking he made a tiny, sharp turning movement.
The effect was as if he had twisted the entire world through a
billionth part of a billionth part of a degree. Everything
shifted, was for a moment minutely out of focus, and then
snapped back again as a suddenly different world.
— Douglas Adams, The Long Dark Tea-Time of the Soul
And here is a blend of the concepts "Asgard" and "conference":
"Asgard
During the Interuniverse Society conference,
a bridge was opened to Valhalla…."
Bifrost
In Norse myth, the rainbow bridge
that connected Earth to Asgard,
home of the gods. It was extended
to Tellus Tertius during the
Interuniverse Society conference"
— From A Heinlein Concordance

— Front page picture from a
local morning newspaper published
today, Wednesday, May 25, 2005
As George Balanchine once asked,
"How much story do you want?"
Comments Off on Wednesday May 25, 2005
“Poetry is a satisfying of
the desire for resemblance….
If resemblance is described as
a partial similarity between
two dissimilar things,
it complements and reinforces
that which the two dissimilar things
have in common.
It makes it brilliant.”
— Wallace Stevens,
“Three Academic Pieces” in
The Necessary Angel (1951)
Two dissimilar things:
1. A talk to be given at a conference on “Mathematics and Narrative” in Mykonos in July:
Mark Turner,
“The Role of Narrative Imagining in Blended Mathematical Concepts” —
Abstract:
“The Way We Think (Gilles Fauconnier and Mark Turner; Basic Books, 2002) presents a theory of conceptual integration, or “blending,” as a basic mental operation. See http://blending.stanford.edu. This talk will explore some ways in which narrative imagining plays a role in blended mathematical concepts.”
2. An application of the “conceptual blending” of Fauconnier and Turner to some journal entries of 2004: Cognitive Blending and the Two Cultures.
« Newer Posts







































 For Mira Sorvino, star of "Tarantella,"
For Mira Sorvino, star of "Tarantella,"
































 "Russian writer Alexander Solzhenitsyn, who exposed Stalin's prison system in his novels and spent 20 years in exile, has died near Moscow at the age of 89.
"Russian writer Alexander Solzhenitsyn, who exposed Stalin's prison system in his novels and spent 20 years in exile, has died near Moscow at the age of 89.













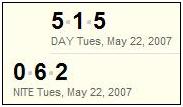



































 doesn’t change, even if the idea one has about it may change.’ ( p. 263). This claim, referring to a ‘crescendo of absurdity’ in Sokal’s original hoax in Social Text, is criticized by anthropologist Joan Fujimura, in an article translated for IS*. Most of Fujimura’s article consists of an astonishingly bland account of the history of non-euclidean geometry, in which she points out that the ratio of the circumference to the diameter depends on the metric. Sokal and Bricmont know this, and Fujimura’s remarks are about as helpful as FN’s** referral of Quine’s readers to Hume (p. 70). Anyway, Sokal explicitly referred to “Euclid’s pi”, presumably to avoid trivial objections like Fujimura’s — wasted effort on both sides.32 If one insists on making trivial objections, one might recall that the theorem
doesn’t change, even if the idea one has about it may change.’ ( p. 263). This claim, referring to a ‘crescendo of absurdity’ in Sokal’s original hoax in Social Text, is criticized by anthropologist Joan Fujimura, in an article translated for IS*. Most of Fujimura’s article consists of an astonishingly bland account of the history of non-euclidean geometry, in which she points out that the ratio of the circumference to the diameter depends on the metric. Sokal and Bricmont know this, and Fujimura’s remarks are about as helpful as FN’s** referral of Quine’s readers to Hume (p. 70). Anyway, Sokal explicitly referred to “Euclid’s pi”, presumably to avoid trivial objections like Fujimura’s — wasted effort on both sides.32 If one insists on making trivial objections, one might recall that the theorem
































