A Ghost Ship —

Related tales for the Church of Synchronology —
See excerpts from an RSS feed this evening.
Earlier related material — Peregrine in this journal.
A Ghost Ship —

Related tales for the Church of Synchronology —
See excerpts from an RSS feed this evening.
Earlier related material — Peregrine in this journal.
A meditation suggested by yesterday's evening fashion news . . .

* A discussion of Stevens's late poem "The Rock" (1954) in
Wallace Stevens: A World of Transforming Shapes,
by Alan D. Perlis, Bucknell University Press, 1976, p. 120 —
"For Stevens, the poem 'makes meanings of the rock.'
In the mind, 'its barrenness becomes a thousand things/
And so exists no more.' In fact, in a peculiar irony that
only a poet with Stevens's particular notion of the imagination's
function could develop, the rock becomes the mind itself,
shattered into such diamond-faceted brilliance that it
encompasses all possibilities for human thought:
The rock is the gray particular of man's life, The stone from which he rises, up—and—ho, The step to the bleaker depths of his descents ... The rock is the stern particular of the air, The mirror of the planets, one by one, But through man's eye, their silent rhapsodist, Turquoise the rock, at odious evening bright With redness that sticks fast to evil dreams; The difficult rightness of half-risen day. The rock is the habitation of the whole, Its strength and measure, that which is near, point A In a perspective that begins again At B: the origin of the mango's rind. (Collected Poems, 528)"
Related reading: "Back in Action."


|
From http://www.pynchon.pomona.edu/v/eigen.html — Perhaps history this century, thought Eigenvalue, is rippled with gathers in its fabric such that if we are situated, as Stencil seemed to be, at the bottom of a fold, it's impossible to determine warp, woof, or pattern anywhere else. By virtue, however, of existing in one gather it is assumed there are others, compartmented off into sinuous cycles each of which come to assume greater importance than the weave itself and destroy any continuity. Thus it is that we are charmed by funny looking automobiles of the '30's, the curious fashions of the '20's, the peculiar moral habits of our grandparents … We are accordingly lost to any sense of continuous tradition. Perhaps if we lived on a crest, things would be different. We could at least see. (Pp. 155-6, Harper Perennial ed.) |
|
THE PHILOSOPHY OF RUDOLF CARNAP
EDITED BY PAUL ARTHUR SCHILPP
. . . . |
See also . . .
The extraordinary consequences of Einstein’s universe:
Relativity shatters our experience of time
9th January 2023
By Michael David Silberstein
"Professor of Philosophy at Elizabethtown College
and co-athor [sic] of Emergence in Context:
A treatise of twentry [sic] first-century natural philosophy
(Oxford University Press, 2022)."
"… the experience that there is something special about
the character of the present moment. This is what presumably
lead [sic] Einstein to say that
'there is something essential about the Now
which is just outside the realm of science.' "
Silberstein does not give any source for his quotation.
But see the passage from Carnap above.
I do not recommend taking Carnap's — or Silberstein's —
word for anything.
The source of Silberstein's remarks is a publication of an
organization called "Institute of Art and Ideas," or IAI.
Wikipedia on that organization:
"The IAI is responsible for organising the bi-annual festival
HowTheLightGetsIn, the biggest philosophy and music
festival in the world* aimed at 'tackling the dearth of philosophy
in daily life,' in addition to monthly IAI Live events."
* Maya Oppenheim (7 September 2021):
"HowTheLightGetsIn: The world's largest philosophy
and music festival to ask life's big questions."
The Independent.
"We all know the song . . ."
— Neil Diamond, "Love on the Rocks"
|
From "The Case Against the Trauma Plot" by Parul Sehgal, "With the trauma plot, the logic goes: Evoke the wound and we will believe that a body, a person, has borne it. Such belief can be difficult to sustain. The invocation of trauma promises access to some well-guarded bloody chamber; increasingly, though, we feel as if we have entered a rather generic motel room, with all the signs of heavy turnover. The second-season revelation of Ted Lasso’s childhood trauma only reduces him; his peculiar, almost sinister buoyancy is revealed to be merely a coping mechanism. He opens up about his past to his therapist just as another character does to her mother—their scenes are intercut—and it happens that both of their traumatic incidents occurred on the same day. The braided revelations make familiar points about fathers (fallible), secrecy (bad), and banked resentments (also bad), but mostly expose the creakiness of a plot mechanism." |
In context —
Hans Freudenthal in 1962 on the axiomatic approach to geometry
of Fano and Hilbert —
"The bond with reality is cut."

Some philosophical background —

For Weyl's "few isolated relational concepts," see (for instance)
Projective Geometries over Finite Fields , by
J. W. P. Hirschfeld (first published by Oxford University Press in 1979).
Weyl in 1932 —
|
Mathematics is the science of the infinite , its goal the symbolic comprehension of the infinite with human, that is finite, means. It is the great achievement of the Greeks to have made the contrast between the finite and the infinite fruitful for the cognition of reality. The intuitive feeling for, the quiet unquestioning acceptance of the infinite, is peculiar to the Orient; but it remains merely an abstract consciousness, which is indifferent to the concrete manifold of reality and leaves it unformed, unpenetrated. Coming from the Orient, the religious intuition of the infinite, the apeiron , takes hold of the Greek soul in the Dionysiac-Orphic epoch which precedes the Persian wars. Also in this respect the Persian wars mark the separation of the Occident from the Orient. This tension between the finite and the infinite and its conciliation now become the driving motive of Greek investigation; but every synthesis, when it has hardly been accomplished, causes the old contrast to break through anew and in a deepened sense. In this way it determines the history of theoretical cognition to our day. — "The Open World: Three Lectures on the Metaphysical Implications of Science," 1932 |
Twelves (in memory of Robert de Marrais) —
Receipt date for the above article —
Synchronicity check —
Related reading —
http://www.universityreaders.com/pdf/
Incarnations-of-the-Blaring-Bluesblinger_sneak_preview.pdf
(Continued from yesterday's Sunday School Lesson Plan for Peculiar Children)
Novelist George Eliot and programming pioneer Ada Lovelace —

For an image that suggests a resurrected multifaceted
(specifically, 759-faceted) Osterman Omega (as in Sunday's afternoon
Log24 post), behold a photo from today's NY Times philosophy
column "The Stone" that was reproduced here in today's previous post —
For a New York Times view of George Eliot data, see a Log24 post
of September 20, 2016, on the diamond theorem as the Middlemarch
"key to all mythologies."
The Institute for Advanced Study (IAS) at Princeton in its Fall 2015 Letter
revived "Beautiful Mathematics" as a title:
This ugly phrase was earlier used by Truman State University
professor Martin Erickson as a book title. See below.
In the same IAS Fall 2015 Letter appear the following remarks
by Freeman Dyson —
". . . a special case of a much deeper connection that Ian Macdonald
discovered between two kinds of symmetry which we call modular and affine.
The two kinds of symmetry were originally found in separate parts of science,
modular in pure mathematics and affine in physics. Modular symmetry is
displayed for everyone to see in the drawings of flying angels and devils
by the artist Maurits Escher. Escher understood the mathematics and got the
details right. Affine symmetry is displayed in the peculiar groupings of particles
created by physicists with high-energy accelerators. The mathematician
Robert Langlands was the first to conjecture a connection between these and
other kinds of symmetry. . . ." (Wikipedia link added.)
The adjective "modular" might aptly be applied to . . .

The adjective "affine" might aptly be applied to . . .
The geometry of the 4×4 square combines modular symmetry
(i.e., related to theta functions) with the affine symmetry above.
Hudson's 1905 discussion of modular symmetry (that of Rosenhain
tetrads and Göpel tetrads) in the 4×4 square used a parametrization
of that square by the digit 0 and the fifteen 2-subsets of a 6-set, but
did not discuss the 4×4 square as an affine space.
For the connection of the 15 Kummer modular 2-subsets with the 16-
element affine space over the two-element Galois field GF(2), see my note
of May 26, 1986, "The 2-subsets of a 6-set are the points of a PG(3,2)" —
— and the affine structure in the 1979 AMS abstract
"Symmetry invariance in a diamond ring" —
For some historical background on the symmetry investigations by
Dyson and Macdonald, see Dyson's 1972 article "MIssed Opportunities."
For Macdonald's own use of the words "modular" and "affine," see
Macdonald, I. G., "Affine Lie algebras and modular forms,"
Séminaire N. Bourbaki , Vol. 23 (1980-1981), Talk no. 577, pp. 258-276.
Box Office Report —
"Only a peculiar can enter a time loop."
A post from Halloween season seven years ago last Saturday —
Related material — This morning's "Highway 61 Revisited."
I was sidetracked by this peculiar Tweet after a search for
fictions titled "The Weaver's Tale."
A version of the tale that I liked had led to the author's Twitter account
and the above remarks, dated 1 Feb. 2017.
That Tweet date led in turn to Log24 posts now tagged Heinlein Lottery.
8777.
"On a Saturday night" — Johnny Thunder, 1962
"Only a peculiar can enter a time loop." — Tim Burton film, 2016
Highly qualified —
Quotations by and for an artist who reportedly died
on Sunday, January 15, 2017 —
"What drives my vision is a need to locate
a 'genetically felt' devotional space
in which a simultaneous multiplicity
of disparate realities coexists."
— The late Ciel Bergman, in her webpage
"Artist's Statement"
"Once a registered nurse who worked in a hospital
psychiatric ward, Ms. Bergman was a struggling
single mom of two when she couldn’t resist the pull
of her art. In 1969, she entered a painting in the
Jack London Invitational, an art contest in Oakland,
and won first prize. This compelled her to enroll at
the San Francisco Art Institute, where she earned
her master of fine arts with honors in painting."
— Sam Whiting in the San Francisco Chronicle
See also Oakland in this journal and
"Only a peculiar can enter a time loop."
"The peculiar kind of 'identity' that is attributed to
apparently altogether heterogeneous figures
in virtue of their being transformable into one another
by means of certain operations defining a group,
is thus seen to exist also in the domain of perception."
— Ernst Cassirer, quoted here on
Midsummer Eve (St. John's Eve), 2010
Wikipedia on The Exorcist III (1990),
written and directed by William Peter Blatty —
"Kinderman takes his friend, a priest named Father Dyer,
out to see their mutually favorite film It's a Wonderful Life ."
Related material from an RSS feed at noon —

From "Night Moves," by Bob Seger —
And oh, the wonder
Felt the lightning
Yeah, and we waited on the thunder
Waited on the thunder
I woke last night to the sound of thunder
How far-off, I sat and wondered
Started humming a song from 1962
Ain't it funny* how the night moves?
See as well Johnny Thunder on Diamond Records in 1962 —
Recent posts have featured the Tim Burton films
"Miss Peregrine's Home for Peculiar Children"
(and the Ghost Ship), as well as "Ed Wood" (and Plan 9).
Related material —
The previous post suggests a Log24 search for
Stevens + Sorbonne. This yields …
|
Michael Bryson in an essay on Stevens’s The Quest for the Fiction of the Absolute: Canto nine considers the movement of the poem between the particular and the general, the immanent and the transcendent: “The poem goes from the poet’s gibberish to / The gibberish of the vulgate and back again. / Does it move to and fro or is it of both / At once?” The poet, the creator-figure, the shadowy god-figure, is elided, evading us, “as in a senseless element.” The poet seeks to find the transcendent in the immanent, the general in the particular, trying “by a peculiar speech to speak / The peculiar potency of the general.” In playing on the senses of “peculiar” as particular and strange or uncanny , these lines play on the mystical relation of one and many, of concrete and abstract. |
"The poem goes from the poet’s gibberish to
The gibberish of the vulgate and back again.
Does it move to and fro or is it of both
At once?”
— Stevens, “Notes Toward a Supreme Fiction" (1942)
Par exemple , the previous post's title: "Space Case."
A check of the New York Times 's version of the
philosophers' stone yields…
"And as Du Bois, a student of Hegel’s, reminds us,
the one who is in the dominated position is aware
of the perspective of the master: She is conscious
of herself as being seen by the other. It is this
double-consciousness that we must learn to understand."
— Seyla Benhabib, quoted by the Times
on Oct. 29, 2015, 3:30 AM ET
A check of Benhabib's words yields…
"It is a peculiar sensation, this double-consciousness,
this sense of always looking at one's self through
the eyes of others, of measuring one's soul by the tape
of a world that looks on in amused contempt and pity."
That, for example, looks on Harvard in amused contempt and pity…
See Con Vocation.
A review describes the main character in the 1981 novel
The Knife in My Hands —
"His other peculiar characteristic is a fascination, developed during
his university years, with the Cabala and, like a miniature Lowry,*
he spends much of his time wandering drunkenly through its
labyrinthine mysteries."
AA motto: "Principles before personalities."

* Related material: Back to the Real.
From "Entertainment," a 1981 story by M. A. Foster—
|
"For some time, Cormen had enjoyed a peculiar suspicion, which he had learned from his wanderings around the city, and cultivated with a little notebook, in which he had made a detailed series of notes and jottings, as well as crude, but effective, charts and maps of certain districts. 'Cormen's Problem,' as it was known, was familiar to the members of the circle in which he moved; in fact, if he had not been so effective with his productions and so engaging in his personality, they might have considered him a bore. It seemed, so the suspicion went, that the city was slowly shrinking, as evidenced by abandoned districts along the city edges. Beyond the empty houses were ruins, and beyond that, traces of foundations and street lines. Moreover, it had recently dawned on him that there were no roads out of the city, although there were no restraints. One hardly noticed this—it was the norm. But like many an easy assumption, once broken it became increasingly obvious. Cormen's acquaintances were tolerant of his aberration, but generally unsympathetic. What he needed was proof, something he could demonstrate in black and white—and color if required. But the city was reluctant, so it appeared, to give up its realities so easily. The Master Entertainment Center, MEC, would not answer direct queries about this, even though it would obediently show him presentations, pictorial or symbolic as he required, of the areas in question. But it was tiresome detail work, in which he had to proceed completely on his own." |
Lily Collins in City of Bones (2013)—
American Folk Art (see August 23, 2011) —

See as well Ballet Blanc .

Meanwhile…
|
Log24 on Sunday, October 5, 2008— “In a game of chess, the knight’s move is unique because it alone goes around corners. In this way, it combines the continuity of a set sequence with the discontinuity of an unpredictable turn in the middle. This meaningful combination of continuity and discontinuity in an otherwise linear set of possibilities has led some to refer to the creative act of discovery in any field of research as a ‘knight’s move’ in intelligence.” Related material: Terence McKenna: “Schizophrenia is not a psychological disorder peculiar to human beings. Schizophrenia is not a disease at all but rather a localized traveling discontinuity of the space time matrix itself. It is like a travelling whirl-wind of radical understanding that haunts time. It haunts time in the same way that Alfred North Whitehead said that the color dove grey ‘haunts time like a ghost.’” Anonymous author: “‘Knight’s move thinking’ is a psychiatric term describing a thought disorder where in speech the usual logical sequence of ideas is lost, the sufferer jumping from one idea to another with no apparent connection. It is most commonly found in schizophrenia.” |
Related journalism—

"What's the 'S' stand for?" — Amy Adams
Random thoughts from A Story (September 13th)—
|
797 April 24, 2003 |
451 August 26, 2002 |
|
1734 April 24, 2005 |
3276 March 24, 2008 |
A September 24th story from The Washington Post —
"James R. Adams was author or co-author of seven books,
beginning in 1971 with 'The Sting of Death.'"
Adams, an Episcopal priest, died on September 13th.
The September 13th Log24 post from which the above numbered links were
taken was in memory of film producer John Calley, who also died on that day.
A quote from Adams on the resurrected body of Christ:
"This new body had peculiar powers."
"Let me put you in this unit."
For Norway's Niels Henrik Abel (1802-1829)
on his birthday, August Fifth
(6 PM Aug. 4, Eastern Time, is 12 AM Aug. 5 in Oslo.)
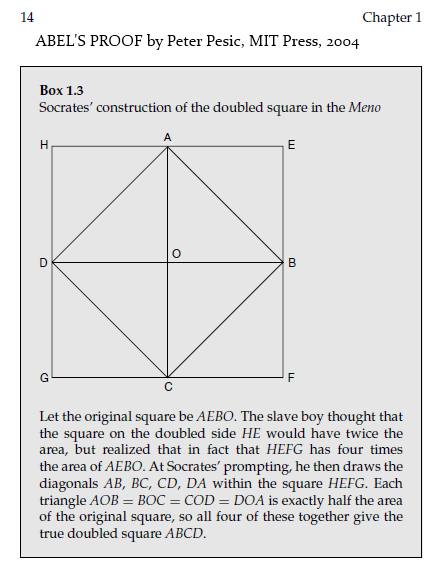
Plato's Diamond
The above version by Peter Pesic is from Chapter I of his book Abel's Proof , titled "The Scandal of the Irrational." Plato's diamond also occurs in a much later mathematical story that might be called "The Scandal of the Noncontinuous." The story—
Paradigms"These passages suggest that the Form is a character or set of characters common to a number of things, i.e. the feature in reality which corresponds to a general word. But Plato also uses language which suggests not only that the forms exist separately (χωριστά ) from all the particulars, but also that each form is a peculiarly accurate or good particular of its own kind, i.e. the standard particular of the kind in question or the model (παράδειγμα ) [i.e. paradigm ] to which other particulars approximate…. … Both in the Republic and in the Sophist there is a strong suggestion that correct thinking is following out the connexions between Forms. The model is mathematical thinking, e.g. the proof given in the Meno that the square on the diagonal is double the original square in area." – William and Martha Kneale, The Development of Logic , Oxford University Press paperback, 1985 Plato's paradigm in the Meno—
Changed paradigm in the diamond theorem (2×2 case) —
Aspects of the paradigm change— Monochrome figures to Areas to Continuous transformations to Euclidean geometry to Euclidean quantities to The 24 patterns resulting from the paradigm change—
Each pattern has some ordinary or color-interchange symmetry. This is the 2×2 case of a more general result. The patterns become more interesting in the 4×4 case. For their relationship to finite geometry and finite fields, see the diamond theorem. |
Related material: Plato's Diamond by Oslo artist Josefine Lyche.
“Plato’s Ghost evokes Yeats’s lament that any claim to worldly perfection inevitably is proven wrong by the philosopher’s ghost….”
— Princeton University Press on Plato’s Ghost: The Modernist Transformation of Mathematics (by Jeremy Gray, September 2008)
"Remember me to her."
— Closing words of the Algis Budrys novel Rogue Moon .
Background— Some posts in this journal related to Abel or to random thoughts from his birthday.
For St. Peter's Day
"For Stevens, the poem 'makes meanings of the rock.'
In the mind, 'its barrenness becomes a thousand things/
And so exists no more.' In fact, in a peculiar irony
that only a poet with Stevens's particular notion
of the imagination's function could develop,
the rock becomes the mind itself, shattered
into such diamond-faceted brilliance
that it encompasses all possibilities for human thought…."
—A discussion of Stevens's late poem "The Rock" (1954)
in Wallace Stevens: A World of Transforming Shapes,
by Alan D. Perlis, Bucknell University Press, 1976, p. 120
Related material on transforming shapes:
Today's previous post suggests the following—

Bester on bedrock and "the bottom line of all existence" suggests
a review of Wallace Stevens's "The Rock." Some background:
See Succor, May 11, and But Seriously, May 12.
See also Waiting for Benjamin, May 15.
Larry McMurtry famously wrote of reading Walter Benjamin
at the Dairy Queen. I never read Benjamin there, but I did
read at least some of the Bester book quoted above.
The bottom lines of this peculiar meditation—
It is not enough to cover the rock with leaves.
We must be cured of it by a cure of the ground
Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And yet the leaves, if they broke into bud,
If they broke into bloom, if they bore fruit,
And if we ate the incipient colorings
Of their fresh culls might be a cure of the ground.
— "The Rock," a poem by Wallace Stevens from
a section with the same title in the Collected Poems .
"These passages suggest that the Form is a character or set of characters
common to a number of things, i.e. the feature in reality which corresponds
to a general word. But Plato also uses language which suggests not only
that the forms exist separately (χωριστά ) from all the particulars, but also
that each form is a peculiarly accurate or good particular of its own kind,
i.e. the standard particular of the kind in question or the model (παράδειγμα )
[i.e. paradigm ] to which other particulars approximate….
… Both in the Republic and in the Sophist there is a strong suggestion
that correct thinking is following out the connexions between Forms.
The model is mathematical thinking, e.g. the proof given in the Meno
that the square on the diagonal is double the original square in area."
— William and Martha Kneale, The Development of Logic,
Oxford University Press paperback, 1985
Plato's paradigm in the Meno —

Changed paradigm in the diamond theorem (2×2 case) —

Aspects of the paradigm change* —
Monochrome figures to
colored figures
Areas to
transformations
Continuous transformations to
non-continuous transformations
Euclidean geometry to
finite geometry
Euclidean quantities to
finite fields
Some pedagogues may find handling all of these
conceptual changes simultaneously somewhat difficult.
* "Paradigm shift " is a phrase that, as John Baez has rightly pointed out,
should be used with caution. The related phrase here was suggested by Plato's
term παράδειγμα above, along with the commentators' specific reference to
the Meno figure that serves as a model. (For "model" in a different sense,
see Burkard Polster.) But note that Baez's own beloved category theory
has been called a paradigm shift.
Excerpts from "The Concept of Group and the Theory of Perception,"
by Ernst Cassirer, Philosophy and Phenomenological Research,
Volume V, Number 1, September, 1944.
(Published in French in the Journal de Psychologie, 1938, pp. 368-414.)
The group-theoretical interpretation of the fundaments of geometry is,
from the standpoint of pure logic, of great importance, since it enables us to
state the problem of the "universality" of mathematical concepts in simple
and precise form and thus to disentangle it from the difficulties and ambigui-
ties with which it is beset in its usual formulation. Since the times of the
great controversies about the status of universals in the Middle Ages, logic
and psychology have always been troubled with these ambiguities….
Our foregoing reflections on the concept of group permit us to define more
precisely what is involved in, and meant by, that "rule" which renders both
geometrical and perceptual concepts universal. The rule may, in simple
and exact terms, be defined as that group of transformations with regard to
which the variation of the particular image is considered. We have seen
above that this conception operates as the constitutive principle in the con-
struction of the universe of mathematical concepts….
…Within Euclidean geometry,
a "triangle" is conceived of as a pure geometrical "essence," and this
essence is regarded as invariant with respect to that "principal group" of
spatial transformations to which Euclidean geometry refers, viz., displace-
ments, transformations by similarity. But it must always be possible to
exhibit any particular figure, chosen from this infinite class, as a concrete
and intuitively representable object. Greek mathematics could not
dispense with this requirement which is rooted in a fundamental principle
of Greek philosophy, the principle of the correlatedness of "logos" and
"eidos." It is, however, characteristic of the modern development of
mathematics, that this bond between "logos" and "eidos," which was indis-
soluble for Greek thought, has been loosened more and more, to be, in the
end, completely broken….
…This process has come to its logical
conclusion and systematic completion in the development of modern group-
theory. Geometrical figures are no longer regarded as fundamental, as
date of perception or immediate intuition. The "nature" or "essence" of a
figure is defined in terms of the operations which may be said to
generate the figure. The operations in question are, in turn, subject to
certain group conditions….
…What we
find in both cases are invariances with respect to variations undergone by
the primitive elements out of which a form is constructed. The peculiar
kind of "identity" that is attributed to apparently altogether heterogen-
eous figures in virtue of their being transformable into one another by means
of certain operations defining a group, is thus seen to exist also in the
domain of perception. This identity permits us not only to single out ele-
ments but also to grasp "structures" in perception. To the mathematical
concept of "transformability" there corresponds, in the domain of per-
ception, the concept of "transposability." The theory of the latter con-
cept has been worked out step by step and its development has gone through
various stages….
…By the acceptance of
"form" as a primitive concept, psychological theory has freed it from the
character of contingency which it possessed for its first founders. The inter-
pretation of perception as a mere mosaic of sensations, a "bundle" of simple
sense-impressions has proved untenable….
…In the domain of mathematics this state of affairs mani-
fests itself in the impossibility of searching for invariant properties of a
figure except with reference to a group. As long as there existed but one
form of geometry, i.e., as long as Euclidean geometry was considered as the
geometry kat' exochen this fact was somehow concealed. It was possible
to assume implicitly the principal group of spatial transformations that lies
at the basis of Euclidean geometry. With the advent of non-Euclidean
geometries, however, it became indispensable to have a complete and sys-
tematic survey of the different "geometries," i.e., the different theories of
invariancy that result from the choice of certain groups of transformation.
This is the task which F. Klein set to himself and which he brought to a
certain logical fulfillment in his Vergleichende Untersuchungen ueber neuere
geometrische Forschungen….
…Without discrimination between the
accidental and the substantial, the transitory and the permanent, there
would be no constitution of an objective reality.
This process, unceasingly operative in perception and, so to speak, ex-
pressing the inner dynamics of the latter, seems to have come to final per-
fection, when we go beyond perception to enter into the domain of pure
thought. For the logical advantage and peculiar privilege of the pure con –
cept seems to consist in the replacement of fluctuating perception by some-
thing precise and exactly determined. The pure concept does not lose
itself in the flux of appearances; it tends from "becoming" toward "being,"
from dynamics toward statics. In this achievement philosophers have
ever seen the genuine meaning and value of geometry. When Plato re-
gards geometry as the prerequisite to philosophical knowledge, it is because
geometry alone renders accessible the realm of things eternal; tou gar aei
ontos he geometrike gnosis estin. Can there be degrees or levels of objec-
tive knowledge in this realm of eternal being, or does not rather knowledge
attain here an absolute maximum? Ancient geometry cannot but answer
in the affirmative to this question. For ancient geometry, in the classical
form it received from Euclid, there was such a maximum, a non plus ultra.
But modern group theory thinking has brought about a remarkable change
In this matter. Group theory is far from challenging the truth of Euclidean
metrical geometry, but it does challenge its claim to definitiveness. Each
geometry is considered as a theory of invariants of a certain group; the
groups themselves may be classified in the order of increasing generality.
The "principal group" of transformations which underlies Euclidean geome-
try permits us to establish a number of properties that are invariant with
respect to the transformations in question. But when we pass from this
"principal group" to another, by including, for example, affinitive and pro-
jective transformations, all that we had established thus far and which,
from the point of view of Euclidean geometry, looked like a definitive result
and a consolidated achievement, becomes fluctuating again. With every
extension of the principal group, some of the properties that we had taken
for invariant are lost. We come to other properties that may be hierar-
chically arranged. Many differences that are considered as essential
within ordinary metrical geometry, may now prove "accidental." With
reference to the new group-principle they appear as "unessential" modifica-
tions….
… From the point of view of modern geometrical systematization,
geometrical judgments, however "true" in themselves, are nevertheless not
all of them equally "essential" and necessary. Modern geometry
endeavors to attain progressively to more and more fundamental strata of
spatial determination. The depth of these strata depends upon the com-
prehensiveness of the concept of group; it is proportional to the strictness of
the conditions that must be satisfied by the invariance that is a universal
postulate with respect to geometrical entities. Thus the objective truth
and structure of space cannot be apprehended at a single glance, but have to
be progressively discovered and established. If geometrical thought is to
achieve this discovery, the conceptual means that it employs must become
more and more universal….
Alyssa is Wonderland
Manohla Dargis in The New York Times yesterday—
“Of course the character of Carroll’s original Alice is evident in each outrageous creation she dreams up in ‘Wonderland’ and in the sequel, ‘Through the Looking-Glass,’ which means that she’s a straight man to her own imagination. (She is Wonderland.)”
From Inside the White Cube—
“The sacramental nature of the space becomes clear, and so does one of the great projective laws of modernism: as modernism gets older, context becomes content. In a peculiar reversal, the object introduced into the gallery ‘frames’ the gallery and its laws.”
From Yogi Berra–
“When you come to a fork in the road, take it.”
Related material: For Baron Samedi and…
Where Entertainment is God, continued—
The following paragraphs are from a review by Piotr Siemion of Infinite Jest, a novel by David Foster Wallace. Illustrations have been added.
"Wallace was somehow able to twist together three yarns…. …there's a J.D Salinger for those who like J.D. Salinger. There's William Burroughs for those hardy souls who like some kick in their prose. And there's a dash of Kurt Vonnegut too. All three voices, though, are amplified in Infinite Jest beyond mere distortion and then projected onto Wallace's peculiar own three-ring circus….
… there's entertainment. Make it a capital E.
Illustration by Clint Eastwood
from Log24 post "E is for Everlast"
Infinite Jest revolves, among its many gyrations, around the story of the Entertainment, a film-like creation going by the title of 'Infinite Jest' and created shortly before his suicidal death by the young tennis star's father. The Entertainment's copies are now being disseminated clandestinely all over Wallace's funny America. Problem is, of course, that the film is too good. Anybody who gets to watch it becomes hooked instantly and craves only to watch it again, and again, and again, until the audience drops dead of exhaustion and hunger. Why eat when you're entertained by such a good movie? Wallace's premise brings you back to that apocryphal lab experiment in which rats were treated to a similar choice. When the rat pushed one button, marked FOOD, it would get a food pellet. The other button, marked FUN, would fire up an electrode rigged right into the orgasm center somewhere in the rat's cortex. Needless to add, one rat after another would drop dead from hunger, still twitching luridly and trying to finesse one last push of the button. Same thing in Wallace's story, especially that even those characters who have not seen the Entertainment yet, keep on entertaining themselves by different means."
The title of the Entertainment, "Infinite Jest," might also be applied to a BBC program featuring mathematician Peter J. Cameron. The program's actual title was "To Infinity and Beyond." It was broadcast the night of Feb. 10 (the date of this journal's previous post).
Few, however, are likely to find the Infinity program addictive. For closer approaches to Wallace's ideal Entertainment, see instead Dante (in the context of this journal's Feb. 4 posts on Cameron and the afterlife) and the BBC News.
Begettings of
the Broken Bold
Thanks for the following
quotation (“Non deve…
nella testa“) go to the
weblog writer who signs
himself “Conrad H. Roth.”
|
… Yesterday I took leave of my Captain, with a promise of visiting him at Bologna on my return. He is a true A PAPAL SOLDIER’S IDEAS OF PROTESTANTS 339 representative of the majority of his countrymen. Here, however, I would record a peculiarity which personally distinguished him. As I often sat quiet and lost in thought he once exclaimed “Che pensa? non deve mai pensar l’uomo, pensando s’invecchia;” which being interpreted is as much as to say, “What are you thinking about: a man ought never to think; thinking makes one old.” And now for another apophthegm of his; “Non deve fermarsi l’uomo in una sola cosa, perche allora divien matto; bisogna aver mille cose, una confusione nella testa;” in plain English, “A man ought not to rivet his thoughts exclusively on any one thing, otherwise he is sure to go mad; he ought to have in his head a thousand things, a regular medley.” Certainly the good man could not know that the very thing that made me so thoughtful was my having my head mazed by a regular confusion of things, old and new. The following anecdote will serve to elucidate still more clearly the mental character of an Italian of this class. Having soon discovered that I was a Protestant, he observed after some circumlocution, that he hoped I would allow him to ask me a few questions, for he had heard such strange things about us Protestants that he wished to know for a certainty what to think of us. |
Notes for Roth:

The title of this entry,
“Begettings of the Broken Bold,”
is from Wallace Stevens’s
“The Owl in the Sarcophagus”–
This was peace after death, the brother of sleep, The inhuman brother so much like, so near, Yet vested in a foreign absolute, Adorned with cryptic stones and sliding shines, An immaculate personage in nothingness, With the whole spirit sparkling in its cloth, Generations of the imagination piled In the manner of its stitchings, of its thread, In the weaving round the wonder of its need, And the first flowers upon it, an alphabet By which to spell out holy doom and end, A bee for the remembering of happiness. Peace stood with our last blood adorned, last mind, Damasked in the originals of green, A thousand begettings of the broken bold. This is that figure stationed at our end, Always, in brilliance, fatal, final, formed Out of our lives to keep us in our death.... |
Related material:
Some further context:
Roth’s entry of Nov. 3, 2006–
“Why blog, sinners?“–
and Log24 on that date:
“First to Illuminate.”
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."

|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)

over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Cara:

Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Diamond-Faceted:
Transformations
of the Rock
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|

For some explanation of the
groups of 8 and 24
motions referred to in the note,
see an earlier note from 1981.
For the Perlis "diamond facets,"
see the Diamond 16 Puzzle.
For a much larger group
of motions, see
Solomon's Cube.
As for "the mind itself"
and "possibilities for
human thought," see
Geometry of the I Ching.
Last night's entry presented a
short story summarized by
four lottery numbers.
Today's mid-day lotteries
and associated material:
Pennsylvania, 201– i.e., 2/01:
Kindergarten Theology —
"In a game of chess, the knight's move is unique because it alone goes around corners. In this way, it combines the continuity of a set sequence with the discontinuity of an unpredictable turn in the middle. This meaningful combination of continuity and discontinuity in an otherwise linear set of possibilities has led some to refer to the creative act of discovery in any field of research as a 'knight's move' in intelligence."
"One must join forces with friends of like mind"
Related material:
"Schizophrenia is not a psychological disorder peculiar to human beings. Schizophrenia is not a disease at all but rather a localized traveling discontinuity of the space time matrix itself. It is like a travelling whirl-wind of radical understanding that haunts time. It haunts time in the same way that Alfred North Whitehead said that the color dove grey 'haunts time like a ghost.'"
"'Knight's move thinking' is a psychiatric term describing a thought disorder where in speech the usual logical sequence of ideas is lost, the sufferer jumping from one idea to another with no apparent connection. It is most commonly found in schizophrenia."

I know more than Apollo,
For oft when he lies sleeping
I see the stars at mortal wars
In the wounded welkin weeping.
For more on the sleep of Apollo,
see the front page of today's
New York Times Book Review.
Garrison Keillor's piece there,
"Dying of the Light," is
about the fear of death felt
by an agnostic British twit.
For relevant remarks by
a British non-twit, see
William Dunbar–
Hermann Hesse's 1943 The Glass Bead Game (Picador paperback, Dec. 6, 2002, pp. 139-140)–
"For the present, the Master showed him a bulky memorandum, a proposal he had received from an organist– one of the innumerable proposals which the directorate of the Game regularly had to examine. Usually these were suggestions for the admission of new material to the Archives. One man, for example, had made a meticulous study of the history of the madrigal and discovered in the development of the style a curved that he had expressed both musically and mathematically, so that it could be included in the vocabulary of the Game. Another had examined the rhythmic structure of Julius Caesar's Latin and discovered the most striking congruences with the results of well-known studies of the intervals in Byzantine hymns. Or again some fanatic had once more unearthed some new cabala hidden in the musical notation of the fifteenth century. Then there were the tempestuous letters from abstruse experimenters who could arrive at the most astounding conclusions from, say, a comparison of the horoscopes of Goethe and Spinoza; such letters often included pretty and seemingly enlightening geometric drawings in several colors."
From Siri Hustvedt, author of Mysteries of the Rectangle: Essays on Painting (Princeton Architectural Press, 2005)– What I Loved: A Novel (Picador paperback, March 1, 2004, page 168)–
A description of the work of Bill Wechsler, a fictional artist:
"Bill worked long hours on a series of autonomous pieces about numbers. Like O's Journey, the works took place inside glass cubes, but these were twice as large– about two feet square. He drew his inspiration from sources as varied as the Cabbala, physics, baseball box scores, and stock market reports. He painted, cut, sculpted, distorted, and broke the numerical signs in each work until they became unrecognizable. He included figures, objects, books, windows, and always the written word for the number. It was rambunctious art, thick with allusion– to voids, blanks, holes, to monotheism and the individual, the the dialectic and yin-yang, to the Trinity, the three fates, and three wishes, to the golden rectangle, to seven heavens, the seven lower orders of the sephiroth, the nine Muses, the nine circles of Hell, the nine worlds of Norse mythology, but also to popular references like A Better Marriage in Five Easy Lessons and Thinner Thighs in Seven Days. Twelve-step programs were referred to in both cube one and cube two. A miniature copy of a book called The Six Mistakes Parents Make Most Often lay at the bottom of cube six. Puns appeared, usually well disguised– one, won; two, too, and Tuesday; four, for, forth; ate, eight. Bill was partial to rhymes as well, both in images and words. In cube nine, the geometric figure for a line had been painted on one glass wall. In cube three, a tiny man wearing the black-and-white prison garb of cartoons and dragging a leg iron has
— End of page 168 —
opened the door to his cell. The hidden rhyme is "free." Looking closely through the walls of the cube, one can see the parallel rhyme in another language: the German word drei is scratched into one glass wall. Lying at the bottom of the same box is a tiny black-and-white photograph cut from a book that shows the entrance to Auschwitz: ARBEIT MACHT FREI. With every number, the arbitrary dance of associations worked togethere to create a tiny mental landscape that ranged in tone from wish-fulfillment dream to nightmare. Although dense, the effect of the cubes wasn't visually disorienting. Each object, painting, drawing, bit of text, or sculpted figure found its rightful place under the glass according to the necessary, if mad, logic of numerical, pictorial, and verbal connection– and the colors of each were startling. Every number had been given a thematic hue. Bill had been interested in Goethe's color wheel and in Alfred Jensen's use of it in his thick, hallucinatory paintings of numbers. He had assigned each number a color. Like Goethe, he included black and white, although he didn't bother with the poet's meanings. Zero and one were white. Two was blue. Three was red, four was yellow, and he mixed colors: pale blue for five, purples in six, oranges in seven, greens in eight, and blacks and grays in nine. Although other colors and omnipresent newsprint always intruded on the basic scheme, the myriad shades of a single color dominated each cube.
The number pieces were the work of a man at the top of his form. An organic extension of everything Bill had done before, these knots of symbols had an explosive effect. The longer I looked at them, the more the miniature constructions seemed on the brink of bursting from internal pressure. They were tightly orchestrated semantic bombs through which Bill laid bare the arbitrary roots of meaning itself– that peculiar social contract generated by little squiggles, dashes, lines, and loops on a page."
|
From 2002:
Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale "block design" subtest. |
|
ZZ
Figures from the
Poem by Eugen Jost:
Mit Zeichen und Zahlen
Numbers and Names,
With numbers and names English translation A related poem:
Alphabets
From time to time
But if a savage
— Hermann Hesse (1943), |
Haunting Time
"Macquarrie remains one of the most
important commentators [on] …
Heidegger's work. His co-translation
of Being and Time into English is
considered the canonical version."
— Wikipedia

The Rev. Macquarrie died on
May 28. The Log24 entry
for that date contains the
following illustration:

The part of the illustration
relevant to the death of
Macquarrie is the color.
From my reply to
a comment on the
May 28 entry:
From McKenna's afterword:
My own remarks on the hippie
scene seem appropriate as a
response to media celebration
of today's 40th anniversary of
the beginning of the 1967
"summer of love."
Hopkins on Parallelism
“The structure of poetry is that of continuous parallelism, ranging from the technical so-called Parallelism of Hebrew Poetry and the antiphons of Church music up to the intricacy of Greek or Italian or English verse. But parallelism is of two kinds necessarily – where the opposition is clearly marked, and where it is transitional rather or chromatic. Only the first kind, that of marked parallelism is concerned with the structure of verse — in rhythm, the recurrence of a certain sequence of rhythm, in alliteration, in assonance and in rhyme. Now the force of this recurrence is to beget a recurrence or parallelism answering to it in the words or thought and, speaking roughly and rather for the tendency than the invariable result, the more marked parallelism in structure whether of elaboration or of emphasis begets more marked parallelism in the words and sense. And moreover parallelism in expression tends to beget or passes into parallelism in thought. This point reached we shall be able to see and account for the peculiarities of poetic diction. To the marked or abrupt kind of parallelism belong metaphor, simile, parable, and so on, where the effect is sought in likeness of things, and antithesis, contrast, and so on, where it is sought in unlikeness. To the chromatic parallelism belong gradation, intensity, climax, tone, expression (as the word is used in music), chiaroscuro, perhaps emphasis: while the faculties of Fancy and Imagination might range widely over both kinds, Fancy belonging more especially to the abrupt than to the transitional class.”
— From Gerard Manley Hopkins, “Poetic Diction,” 1865
For an application to Hopkins’s poetry, see an excerpt from Stephen Prickett, Words and the Word: Language, Poetics and Biblical Interpretation (Cambridge: Cambridge University Press, 1986).
See also the publisher’s description of Maria R. Lichtmann’s The Contemplative Poetry of Gerard Manley Hopkins, Princeton University Press, 1989.
A Circle of Quiet
From the Harvard Math Table page:
“No Math table this week. We will reconvene next week on March 14 for a special Pi Day talk by Paul Bamberg.”
| Some friends of mine are in this band. They’re playing in a bar on Diversey, way down the bill, around… I said I’d be there. Great. Imaginary number? It’s a math joke. |
From the April 2006 Notices of the American Mathematical Society, a footnote in a review by Juliette Kennedy (pdf) of Rebecca Goldstein’s Incompleteness:
4 There is a growing literature in the area of postmodern commentaries of [sic] Gödel’s theorems. For example, Régis Debray has used Gödel’s theorems to demonstrate the logical inconsistency of self-government. For a critical view of this and related developments, see Bricmont and Sokal’s Fashionable Nonsense [13]. For a more positive view see Michael Harris’s review of the latter, “I know what you mean!” [9]….
[9] MICHAEL HARRIS, “I know what you mean!,” http://www.math.jussieu.fr/~harris/Iknow.pdf.
[13] ALAN SOKAL and JEAN BRICMONT, Fashionable Nonsense, Picador, 1999.
Following the trail marked by Ms. Kennedy, we find the following in Harris’s paper:
“Their [Sokal’s and Bricmont’s] philosophy of mathematics, for instance, is summarized in the sentence ‘A mathematical constant like
doesn’t change, even if the idea one has about it may change.’ ( p. 263). This claim, referring to a ‘crescendo of absurdity’ in Sokal’s original hoax in Social Text, is criticized by anthropologist Joan Fujimura, in an article translated for IS*. Most of Fujimura’s article consists of an astonishingly bland account of the history of non-euclidean geometry, in which she points out that the ratio of the circumference to the diameter depends on the metric. Sokal and Bricmont know this, and Fujimura’s remarks are about as helpful as FN’s** referral of Quine’s readers to Hume (p. 70). Anyway, Sokal explicitly referred to “Euclid’s pi”, presumably to avoid trivial objections like Fujimura’s — wasted effort on both sides.32 If one insists on making trivial objections, one might recall that the theorem
that p is transcendental can be stated as follows: the homomorphism Q[X] –> R taking X tois injective. In other words,
can be identified algebraically with X, the variable par excellence.33
More interestingly, one can ask what kind of object
was before the formal definition of real numbers. To assume the real numbers were there all along, waiting to be defined, is to adhere to a form of Platonism.34 Dedekind wouldn’t have agreed.35 In a debate marked by the accusation that postmodern writers deny the reality of the external world, it is a peculiar move, to say the least, to make mathematical Platonism a litmus test for rationality.36 Not that it makes any more sense simply to declare Platonism out of bounds, like Lévy-Leblond, who calls Stephen Weinberg’s gloss on Sokal’s comment ‘une absurdité, tant il est clair que la signification d’un concept quelconque est évidemment affectée par sa mise en oeuvre dans un contexte nouveau!’37 Now I find it hard to defend Platonism with a straight face, and I prefer to regard the formula

as a creation rather than a discovery. But Platonism does correspond to the familiar experience that there is something about mathematics, and not just about other mathematicians, that precisely doesn’t let us get away with saying ‘évidemment’!38
32 There are many circles in Euclid, but no pi, so I can’t think of any other reason for Sokal to have written ‘Euclid’s pi,’ unless this anachronism was an intentional part of the hoax. Sokal’s full quotation was ‘the
of Euclid and the G of Newton, formerly thought to be constant and universal, are now perceived in their ineluctable historicity.’ But there is no need to invoke non-Euclidean geometry to perceive the historicity of the circle, or of pi: see Catherine Goldstein’s ‘L’un est l’autre: pour une histoire du cercle,’ in M. Serres, Elements d’histoire des sciences, Bordas, 1989, pp. 129-149.
33 This is not mere sophistry: the construction of models over number fields actually uses arguments of this kind. A careless construction of the equations defining modular curves may make it appear that pi is included in their field of scalars.
34 Unless you claim, like the present French Minister of Education [at the time of writing, i.e. 1999], that real numbers exist in nature, while imaginary numbers were invented by mathematicians. Thuswould be a physical constant, like the mass of the electron, that can be determined experimentally with increasing accuracy, say by measuring physical circles with ever more sensitive rulers. This sort of position has not been welcomed by most French mathematicians.
35 Cf. M. Kline, Mathematics The Loss of Certainty, p. 324.
36 Compare Morris Hirsch’s remarks in BAMS April 94.
37 IS*, p. 38, footnote 26. Weinberg’s remarks are contained in his article “Sokal’s Hoax,” in the New York Review of Books, August 8, 1996.
38 Metaphors from virtual reality may help here.”
* Earlier defined by Harris as “Impostures Scientifiques (IS), a collection of articles compiled or commissioned by Baudouin Jurdant and published simultaneously as an issue of the journal Alliage and as a book by La Découverte press.”
** Earlier defined by Harris as “Fashionable Nonsense (FN), the North American translation of Impostures Intellectuelles.”
What is the moral of all this French noise?
Perhaps that, in spite of the contemptible nonsense at last summer’s Mykonos conference on mathematics and narrative, stories do have an important role to play in mathematics — specifically, in the history of mathematics.
Despite his disdain for Platonism, exemplified in his remarks on the noteworthy connection of pi with the zeta function in the formula given above, Harris has performed a valuable service to mathematics by pointing out the excellent historical work of Catherine Goldstein. Ms. Goldstein has demonstrated that even a French nominalist can be a first-rate scholar. Her essay on circles that Harris cites in a French version is also available in English, and will repay the study of those who, like Barry Mazur and other Harvard savants, are much too careless with the facts of history. They should consult her “Stories of the Circle,” pp. 160-190 in A History of Scientific Thought, edited by Michel Serres, Blackwell Publishers (December 1995).
For the historically-challenged mathematicians of Harvard, this essay would provide a valuable supplement to the upcoming “Pi Day” talk by Bamberg.
For those who insist on limiting their attention to mathematics proper, and ignoring its history, a suitable Pi Day observance might include becoming familiar with various proofs of the formula, pictured above, that connects pi with the zeta function of 2. For a survey, see Robin Chapman, Evaluating Zeta(2) (pdf). Zeta functions in a much wider context will be discussed at next May’s politically correct “Women in Mathematics” program at Princeton, “Zeta Functions All the Way” (pdf).
Balance

"An asymmetrical balance is sought since it possesses more movement. This is achieved by the imaginary plotting of the character upon a nine-fold square, invented by some ingenious writer of the Tang dynasty. If the square were divided in half or in four, the result would be symmetrical, but the nine-fold square permits balanced asymmetry."
— Chiang Yee, Chinese Calligraphy, quoted in Aspen no. 10, item 8
"'Burnt Norton' opens as a meditation on time. Many comparable and contrasting views are introduced. The lines are drenched with reminiscences of Heraclitus' fragments on flux and movement…. the chief contrast around which Eliot constructs this poem is that between the view of time as a mere continuum, and the difficult paradoxical Christian view of how man lives both 'in and out of time,' how he is immersed in the flux and yet can penetrate to the eternal by apprehending timeless existence within time and above it. But even for the Christian the moments of release from the pressures of the flux are rare, though they alone redeem the sad wastage of otherwise unillumined existence. Eliot recalls one such moment of peculiar poignance, a childhood moment in the rose-garden– a symbol he has previously used, in many variants, for the birth of desire. Its implications are intricate and even ambiguous, since they raise the whole problem of how to discriminate between supernatural vision and mere illusion. Other variations here on the theme of how time is conquered are more directly apprehensible. In dwelling on the extension of time into movement, Eliot takes up an image he had used in 'Triumphal March': 'at the still point of the turning world.' This notion of 'a mathematically pure point' (as Philip Wheelwright has called it) seems to be Eliot's poetic equivalent in our cosmology for Dante's 'unmoved Mover,' another way of symbolising a timeless release from the 'outer compulsions' of the world. Still another variation is the passage on the Chinese jar in the final section. Here Eliot, in a conception comparable to Wallace Stevens' 'Anecdote of the Jar,' has suggested how art conquers time:
Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness."
— F. O. Matthiessen, The Achievement of T.S. Eliot,
Oxford University Press, 1958, as quoted in On "Burnt Norton"
Ideas and Art, Part III
The first idea was not our own. Adam
In Eden was the father of Descartes…
— Wallace Stevens, from
Notes Toward a Supreme Fiction
"Quaedam ex his tanquam rerum imagines sunt, quibus solis proprie convenit ideae nomen: ut cùm hominem, vel Chimaeram, vel Coelum, vel Angelum, vel Deum cogito."
— Descartes, Meditationes III, 5
"Of my thoughts some are, as it were, images of things, and to these alone properly belongs the name idea; as when I think [represent to my mind] a man, a chimera, the sky, an angel or God."
— Descartes, Meditations III, 5
Begin, ephebe, by perceiving the idea
Of this invention, this invented world,
The inconceivable idea of the sun.
You must become an ignorant man again
And see the sun again with an ignorant eye
And see it clearly in the idea of it.
— Wallace Stevens, from
Notes Toward a Supreme Fiction
"… Quinimo in multis saepe magnum discrimen videor deprehendisse: ut, exempli causâ, duas diversas solis ideas apud me invenio, unam tanquam a sensibus haustam, & quae maxime inter illas quas adventitias existimo est recensenda, per quam mihi valde parvus apparet, aliam verò ex rationibus Astronomiae desumptam, hoc est ex notionibus quibusdam mihi innatis elicitam, vel quocumque alio modo a me factam, per quam aliquoties major quàm terra exhibetur; utraque profecto similis eidem soli extra me existenti esse non potest, & ratio persuadet illam ei maxime esse dissimilem, quae quàm proxime ab ipso videtur emanasse."
— Descartes, Meditationes III, 11
"… I have observed, in a number of instances, that there was a great difference between the object and its idea. Thus, for example, I find in my mind two wholly diverse ideas of the sun; the one, by which it appears to me extremely small draws its origin from the senses, and should be placed in the class of adventitious ideas; the other, by which it seems to be many times larger than the whole earth, is taken up on astronomical grounds, that is, elicited from certain notions born with me, or is framed by myself in some other manner. These two ideas cannot certainly both resemble the same sun; and reason teaches me that the one which seems to have immediately emanated from it is the most unlike."
— Descartes, Meditations III, 11
"Et quamvis forte una idea ex aliâ nasci possit, non tamen hîc datur progressus in infinitum, sed tandem ad aliquam primam debet deveniri, cujus causa sit in star archetypi, in quo omnis realitas formaliter contineatur, quae est in ideâ tantùm objective."
— Descartes, Meditationes III, 15
"And although an idea may give rise to another idea, this regress cannot, nevertheless, be infinite; we must in the end reach a first idea, the cause of which is, as it were, the archetype in which all the reality [or perfection] that is found objectively [or by representation] in these ideas is contained formally [and in act]."
— Descartes, Meditations III, 15
Michael Bryson in an essay on Stevens's "Notes Toward a Supreme Fiction,"
The Quest for the Fiction of the Absolute:
"Canto nine considers the movement of the poem between the particular and the general, the immanent and the transcendent: "The poem goes from the poet's gibberish to / The gibberish of the vulgate and back again. / Does it move to and fro or is it of both / At once?" The poet, the creator-figure, the shadowy god-figure, is elided, evading us, "as in a senseless element." The poet seeks to find the transcendent in the immanent, the general in the particular, trying "by a peculiar speech to speak / The peculiar potency of the general." In playing on the senses of "peculiar" as particular and strange or uncanny, these lines play on the mystical relation of one and many, of concrete and abstract."
Brian Cronin in Foundations of Philosophy:
"The insight is constituted precisely by 'seeing' the idea in the image, the intelligible in the sensible, the universal in the particular, the abstract in the concrete. We pivot back and forth between images and ideas as we search for the correct insight."
— From Ch. 2, Identifying Direct Insights
Michael Bryson in an essay on Stevens's "Notes Toward a Supreme Fiction":
"The fourth canto returns to the theme of opposites. 'Two things of opposite natures seem to depend / On one another . . . . / This is the origin of change.' Change resulting from a meeting of opposities is at the root of Taoism: 'Tao produced the One. / The One produced the two. / The two produced the three. / And the three produced the ten thousand things' (Tao Te Ching 42) …."
|
From an entry of March 7, 2004:
From the web page
Introduction to the I Ching– "He who has perceived the meaning of change fixes his attention no longer on transitory individual things but on the immutable, eternal law at work in all change. This law is the tao of Lao-tse, the course of things, the principle of the one in the many. That it may become manifest, a decision, a postulate, is necessary. This fundamental postulate is the 'great primal beginning' of all that exists, t'ai chi — in its original meaning, the 'ridgepole.' Later Chinese philosophers devoted much thought to this idea of a primal beginning. A still earlier beginning, wu chi, was represented by the symbol of a circle. Under this conception, t'ai chi was represented by the circle divided into the light and the dark, yang and yin,
This symbol has also played a significant part in India and Europe. However, speculations of a gnostic-dualistic character are foreign to the original thought of the I Ching; what it posits is simply the ridgepole, the line. With this line, which in itself represents oneness, duality comes into the world, for the line at the same time posits an above and a below, a right and left, front and back-in a word, the world of the opposites." The t'ai chi symbol is also illustrated on the web page Cognitive Iconology, which says that
"W.J.T. Mitchell calls 'iconology' A variation on the t'ai chi symbol appears in a log24.net entry for March 5:
The Line, See too my web page Logos and Logic, which has the following:
Logos Alogos, In the conclusion of Section 3, Canto X, of "Notes," Stevens says
This is the logoi alogoi of Simone Weil. |
In "Notes toward a Supreme Fiction,"
Wallace Stevens lists three criteria
for a work of the imagination:
It Must Be Abstract

The Line,
by S.H. Cullinane
It Must Change
It Must Give Pleasure
Related material:
Quid Pro Quack
(Headline of today’s
Maureen Dowd column)
The essence, nature, or distinctive peculiarity, of a thing; that which answers the question, Quid est? or, What is it?
-Webster’s Revised Unabridged Dictionary (1913)
|
Quid |
|
|
Pro |
|
|
Quo |
The above rather cryptic sequence of pictures may be regarded as a memorial to Queen Juliana of the Netherlands, who died at about the time I found the central picture, “Royal Palm Student.” For further details, click on the individual pictures, each of which is taken from a past log24 entry. Also of some relevance: the palm at the end of A Mass for Lucero and the Stevens poem on The Palm at the End of the Mind.
Transcendental Meditation
|
Transcendental Man |
The bicentennial of Ralph Waldo Emerson was on May 25, 2003. For a commemoration of Emerson on that date, click on the picture below of Harvard University’s Room 305, Emerson Hall.
This will lead you to a discussion of the properties of a 5×5 array, or matrix, with a symbol of mystical unity at its center. Although this symbol of mystical unity, the number “1,” is not, pace the Shema, a transcendental number, the matrix is, as perhaps a sort of Emersonian compensation, what postmodernists would call phallologocentric. It is possible that Emerson is a saint; if so, his feast day (i.e., date of death), April 27, might reveal to us the sort of miraculous fact hoped for by Fritz Leiber in my previous entry. A check of my April 27 notes shows us, lo and behold, another phallologocentric 5×5 array, this one starring Warren Beatty. This rather peculiar coincidence is, perhaps, the sort of miracle appropriate to a saint who is, as this week’s politically correct New Yorker calls him, a Big Dead White Male.
in my previous entry. A check of my April 27 notes shows us, lo and behold, another phallologocentric 5×5 array, this one starring Warren Beatty. This rather peculiar coincidence is, perhaps, the sort of miracle appropriate to a saint who is, as this week’s politically correct New Yorker calls him, a Big Dead White Male.
 Leiber’s fiction furnishes “a behind-the-scenes view of the time change wars.”
Leiber’s fiction furnishes “a behind-the-scenes view of the time change wars.”
“It’s quarter to three…” — St. Frank Sinatra
Powered by WordPress