“His daughter, the poet Jorie Graham, confirmed the death.”
From an artist on Oct. 3, 2013:
“‘This is St. Francis country,’ she says of Umbria.”
Friday, April 4, 2014
Monday, October 14, 2013
Dream of the Expanded Field
Comments Off on Dream of the Expanded Field
Sunday, November 7, 2010
Dream of the Expanded Field
Continued from July 26, 2010 —
Comments Off on Dream of the Expanded Field
Wednesday, September 2, 2015
Expanding the Field
See The Nordic Journal of Aesthetics ,
Vol. 23, No. 42 (2012), pp. 14-31,
"Art in an Expanded Field: Wittgenstein and Aesthetics,"
by Noël Carroll.
Abstract:
"This article reviews the various ways in which the later writings
of Ludwig Wittgenstein have been employed to address the question
'What is Art?' These include the family resemblance model, the
cluster concept model and the form of life model. The article defends
a version of the form of life approach. Also, addressed the charge that
it would have been more profitable had aestheticians explored what
Wittgenstein actually said about art instead of trying to extrapolate from
his writings an approach to what Nigel Warburton calls the art question."
Comments Off on Expanding the Field
Wednesday, June 30, 2010
Field Dream
In memory of Wu Guanzhong, Chinese artist who died in Beijing on Friday—
"Once Knecht confessed to his teacher that he wished to learn enough to be able to incorporate the system of the I Ching into the Glass Bead Game. Elder Brother laughed. 'Go ahead and try,' he exclaimed. 'You'll see how it turns out. Anyone can create a pretty little bamboo garden in the world. But I doubt that the gardener would succeed in incorporating the world in his bamboo grove.'"
— Hermann Hesse, The Glass Bead Game, translated by Richard and Clara Winston
"The Chinese painter Wu Tao-tzu was famous because he could paint nature in a unique realistic way that was able to deceive all who viewed the picture. At the end of his life he painted his last work and invited all his friends and admirers to its presentation. They saw a wonderful landscape with a romantic path, starting in the foreground between flowers and moving through meadows to high mountains in the background, where it disappeared in an evening fog. He explained that this picture summed up all his life’s work and at the end of his short talk he jumped into the painting and onto the path, walked to the background and disappeared forever."
— Jürgen Teichmann. Teichmann notes that "the German poet Hermann Hesse tells a variation of this anecdote, according to his own personal view, as found in his 'Kurzgefasster Lebenslauf,' 1925."
Comments Off on Field Dream
Saturday, October 19, 2024
A Seven-Eleven for Mystics: October 7 . . . 11 Years Ago
Comments Off on A Seven-Eleven for Mystics: October 7 . . . 11 Years Ago
Saturday, September 3, 2022
1984 Revisited
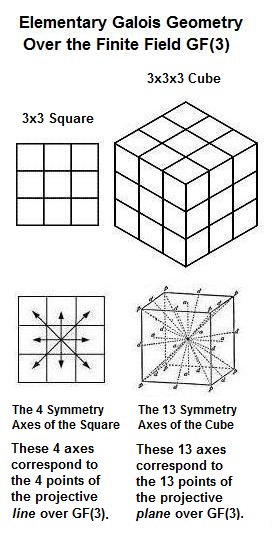
Note the three quadruplets of parallel edges in the 1984 figure above.
The above Gates article appeared earlier, in the June 2010 issue of
Physics World , with bigger illustrations. For instance —
Exercise: Describe, without seeing the rest of the article,
the rule used for connecting the balls above.
Wikipedia offers a much clearer picture of a (non-adinkra) tesseract —
And then, more simply, there is the Galois tesseract —
For parts of my own world in June 2010, see this journal for that month.
The above Galois tesseract appears there as follows:
See also the Klein correspondence in a paper from 1968
in yesterday's 2:54 PM ET post.
Comments Off on 1984 Revisited
Monday, August 1, 2022
Review
From Log24 posts tagged Art Space —
From a paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
“The Universal Kummer Threefold,” by
Qingchun Ren, Steven V Sam, Gus Schrader,
and Bernd Sturmfels —
Two such considerations —
Comments Off on Review
Friday, March 18, 2022
The Dog Far Hence, or Ekphrasis for Anubis
The above image suggests a review of Sigaud in this journal and of . . .
Related material from the Web —
"Anubis, easily recognizable as an anthropomorphized jackal or dog,
was the Egyptian god of the afterlife and mummification. He helped
judge souls after their death and guided lost souls into the afterlife.
So, was he evil? No, and in fact just the opposite. In ancient Egyptian
mythology the ultimate evil was chaos. Nearly all of Egyptian mythology
was focused around maintaining the cycles of cosmic order that kept
chaos at bay. Few things were as significant in this goal as the rituals
maintaining the cycle of life, death, and afterlife. Therefore, Anubis was
not evil but rather one of the most important gods who kept evil out of Egypt."
— Christopher Muscato at Study.com
Comments Off on The Dog Far Hence, or Ekphrasis for Anubis
Sunday, February 20, 2022
Tuesday, November 5, 2019
Non-Woo
Comments Off on Non-Woo
Monday, March 11, 2019
Ant-Man Meets Doctor Strange
The 4×4 square may also be called the Galois Tesseract .
By analogy, the 4x4x4 cube may be called the Galois Hexeract .
Comments Off on Ant-Man Meets Doctor Strange
Sunday, July 1, 2018
Friday, June 29, 2018
For St. Stanley
The phrase "Blue Dream" in the previous post
suggests a Web search for Traumnovelle .
That search yields an interesting weblog post
from 2014 commemorating the 1999 dies natalis
(birth into heaven) of St. Stanley Kubrick.
Related material from March 7, 2014,
in this journal —
That 2014 post was titled "Kummer Varieties." It is now tagged
"Kummerhenge." For some backstory, see other posts so tagged.
Comments Off on For St. Stanley
Wednesday, March 29, 2017
Art Space, Continued
"And as the characters in the meme twitch into the abyss
that is the sky, this meme will disappear into whatever
internet abyss swallowed MySpace."
—Staff writer Kamila Czachorowski, Harvard Crimson today
From Log24 posts tagged Art Space —
From a recent paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
“The Universal Kummer Threefold,” by
Qingchun Ren, Steven V Sam, Gus Schrader, and
Bernd Sturmfels —
Two such considerations —
Comments Off on Art Space, Continued
Monday, October 26, 2015
Expanding the Spielraum
Halloween meditation on the Tummelplatz at Innsbruck —
"Die Ritter und Knappen des nahegelegenen Ambras
pflegten hier ihre Rosse zu tummeln, woher sich auch
der Name Tummelplatz schreibt."
"The knights and squires of nearby Ambras used to let their
steeds romp here, whence came the name Tummelplatz ."
— Quelle: Ludwig von Hörmann, "Der Tummelplatz bei Amras,"
in: Der Alpenfreund , 1. Band, Gera 1870, S. 72 – 73.
See as well Sigmund Freud, Erinnern, Wiederholen und Durcharbeiten
(1914) —
"Wir eröffnen ihm die Übertragung als den Tummelplatz ,
auf dem ihm gestattet wird, sich in fast völliger Freiheit
zu entfalten, und auferlegt ist, uns alles vorzuführen,
was sich an pathogenen Trieben im Seelenleben des
Analysierten verborgen hat."
"We admit it into the transference as a playground
in which it is allowed to expand in almost complete freedom
and in which it is expected to display to us everything in the
way of pathogenic instincts that is hidden in the patient's mind."
This passage has been discussed by later psychotherapists,
notably Russell Meares. Dr. Meares, working from a translation
that has "playground" for Freud's Tummelplatz , uses Spielraum
in place of Freud's own word.
For related material in this journal, see Expanding the Spielraum.
An illustration from that search —
Comments Off on Expanding the Spielraum
Tuesday, February 3, 2015
Expanding the Spielraum
A short poem by several authors:
"The role of
the 16 singular points
on the Kummer surface
is now played by
the 64 singular points
on the Kummer threefold."
— From Remark 2.4 on page 9 of
"The Universal Kummer Threefold,"
by Qingchun Ren, Steven V Sam,
Gus Schrader, and Bernd Sturmfels,
http://arxiv.org/abs/1208.1229v3,
August 6, 2012 — June 12, 2013.
See also "Expanded Field" in this journal.
Illustration from "Sunday School," July 20, 2014.
Comments Off on Expanding the Spielraum
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Comments Off on Sunday School
Sunday, July 6, 2014
Sticks and Stones
The title is from this morning's previous post.
From a theater review in that post—
… "all flying edges and angles, a perpetually moving and hungry soul"
… "a formidably centered presence, the still counterpoint"
A more abstract perspective:
See also Desargues via Galois (August 6, 2013).
Comments Off on Sticks and Stones
Friday, March 7, 2014
Kummer Varieties
The Dream of the Expanded Field continues…
From Klein's 1893 Lectures on Mathematics —
"The varieties introduced by Wirtinger may be called Kummer varieties…."
— E. Spanier, 1956
From this journal on March 10, 2013 —
From a recent paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
"The Universal Kummer Threefold," by
Qingchun Ren, Steven V Sam, Gus Schrader, and Bernd Sturmfels —
Two such considerations —
Update of 10 PM ET March 7, 2014 —
The following slides by one of the "Kummer Threefold" authors give
some background related to the above 64-point vector space and
to the Weyl group of type E7, W (E7):
The Cayley reference is to "Algorithm for the characteristics of the
triple ϑ-functions," Journal für die Reine und Angewandte
Mathematik 87 (1879): 165-169. <http://eudml.org/doc/148412>.
To read this in the context of Cayley's other work, see pp. 441-445
of Volume 10 of his Collected Mathematical Papers .
Comments Off on Kummer Varieties
Friday, November 9, 2012
Ideas
(Continued from Deconstructing Alice)
"Somehow it seems to fill my head
with ideas— only I don't exactly know
what they are!"
See also Deep Play.
Comments Off on Ideas
Friday, September 9, 2011
Galois vs. Rubik
(Continued from Abel Prize, August 26)
The situation is rather different when the
underlying Galois field has two rather than
three elements… See Galois Geometry.
The coffee scene from "Bleu"
Related material from this journal:
The Dream of
the Expanded Field
Comments Off on Galois vs. Rubik
Tuesday, November 23, 2010
Art Object
There is more than one way
to look at a cube.
From Cambridge U. Press on Feb. 20, 2006 —
and from this journal on June 30, 2010 —
In memory of Wu Guanzhong, Chinese artist
who died in Beijing on June 25, 2010 —
See also this journal on Feb. 20, 2006
(the day The Cube was published).
Comments Off on Art Object
Sunday, July 18, 2010
Du Sucre
http://passionforcinema.com/sapphire/ on "Bleu" — Jan. 9, 2010 —
"An extremely long lens on an insert of a sugar cube, dipped just enough, in a small cup of coffee, so that it gradually seeps in the dark beverage. Four and a half seconds of unadulterated cinematic bliss."
Related material from this journal:
The Dream of
the Expanded Field
Comments Off on Du Sucre
Friday, June 25, 2010
ART WARS continued
Comments Off on ART WARS continued
Friday, July 5, 2024
Monday, April 18, 2022
Iconic Simplicity
Comments Off on Iconic Simplicity
Monday, October 23, 2017
Plan 9 Continues
Click for some background —
Another approach, for Dan Brown fans —
In the following passage, Brown claims that an eight-ray star
with arrowheads at the rays' ends is "the mathematical symbol for
entropy." Brown may have first encountered this symbol at a
questionable "Sacred Science" website. Wikipedia discusses
some even less respectable uses of the symbol.
Comments Off on Plan 9 Continues
Saturday, October 18, 2014
Educational Series
Barron's Educational Series (click to enlarge):
The Tablet of Ahkmenrah:
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Another educational series (this journal):
Art theorist Rosalind Krauss and The Ninefold Square
Comments Off on Educational Series
Thursday, October 10, 2013
Continued
|
"… the walkway between here and there would be colder than a witch’s belt buckle. Or a well-digger’s tit. Or whatever the saying was. Vera had been hanging by a thread for a week now, comatose, in and out of Cheyne-Stokes respiration, and this was exactly the sort of night the frail ones picked to go out on. Usually at 4 a.m. He checked his watch. Only 3:20, but that was close enough for government work."
— King, Stephen (2013-09-24). |
From Space.com, the death of an astronaut this morning —
"Carpenter passed at 5:30 a.m. MDT (7:30 a.m. EDT; 1130 GMT)."
A link, "Continued," in this journal at 3:26 a.m. EDT today led to…
Comments Off on Continued
Thursday, February 5, 2009
Thursday February 5, 2009
Through the
Looking Glass:
A Sort of Eternity
From the new president’s inaugural address:
“… in the words of Scripture, the time has come to set aside childish things.”
The words of Scripture:
“through a glass”—
[di’ esoptrou].
By means of
a mirror [esoptron].
Childish things:
© 2005 The Institute for Figuring
Photo by Norman Brosterman
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
Not-so-childish:
Three planes through
the center of a cube
that split it into
eight subcubes:
Through a glass, darkly:
A group of 8 transformations is
generated by affine reflections
in the above three planes.
Shown below is a pattern on
the faces of the 2x2x2 cube
that is symmetric under one of
these 8 transformations–
a 180-degree rotation:
(Click on image
for further details.)
But then face to face:
A larger group of 1344,
rather than 8, transformations
of the 2x2x2 cube
is generated by a different
sort of affine reflections– not
in the infinite Euclidean 3-space
over the field of real numbers,
but rather in the finite Galois
3-space over the 2-element field.
Galois age fifteen,
drawn by a classmate.
These transformations
in the Galois space with
finitely many points
produce a set of 168 patterns
like the one above.
For each such pattern,
at least one nontrivial
transformation in the group of 8
described above is a symmetry
in the Euclidean space with
infinitely many points.
For some generalizations,
see Galois Geometry.
Related material:
The central aim of Western religion–
"Each of us has something to offer the Creator... the bridging of masculine and feminine, life and death. It's redemption.... nothing else matters." -- Martha Cooley in The Archivist (1998) The central aim of Western philosophy– Dualities of Pythagoras as reconstructed by Aristotle: Limited Unlimited Odd Even Male Female Light Dark Straight Curved ... and so on .... “Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy.” — Jamie James in The Music of the Spheres (1993) “In the garden of Adding — The Midrash Jazz Quartet in City of God, by E. L. Doctorow (2000) A quotation today at art critic Carol Kino’s website, slightly expanded: “Art inherited from the old religion — Octavio Paz,”Seeing and Using: Art and Craftsmanship,” in Convergences: Essays on Art and Literature (New York: Harcourt Brace Jovanovich 1987), 52 From Brian O’Doherty’s 1976 Artforum essays– not on museums, but rather on gallery space: “We have now reached
“Space: what you — James Joyce, Ulysses |
Comments Off on Thursday February 5, 2009
Saturday, July 20, 2002
Saturday July 20, 2002
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.