Today's earlier post mentions one approach to the concepts of unity and multiplicity. Here is another.
Unity:
The 3×3×3 Galois Cube
Multiplicity:
One of a group, GL(3,3), of 11,232
natural transformations of the 3×3×3 Cube
See also the earlier 1985 3×3 version by Cullinane.
Apollo and the Tricksters
From The Story of N (Oct. 15, 2010)—
Roberta Smith on what she calls "endgame art"—
"Fear of form above all means fear of compression— of an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible."
Margaret Atwood on tricksters and art—
"If it’s a seamless whole you want, pray to Apollo."
Here is some related material In memory of CIA officer Clare Edward Petty, who died at 90 on March 18—
A review of a sort of storyteller's MacGuffin — the 3×3 grid. This is, in Smith's terms, an "artistic focus" that appears to be visually comprehensible but is not as simple as it seems.
The Hesse configuration can serve as more than a sort of Dan Brown MacGuffin. As a post of January 14th notes, it can (rather fancifullly) illustrate the soul—
" … I feel I understand
Existence, or at least a minute part
Of my existence, only through my art,
In terms of combinational delight…."
— Vladimir Nabokov, Pale Fire
The On-Line Encyclopedia of Integer Sequences has an article titled "Number of combinatorial configurations of type (n_3)," by N.J.A. Sloane and D. Glynn.
From that article:
The following corrects the word "unique" in the example.
* This post corrects an earlier post, also numbered 14660 and dated 7 PM March 18, 2011, that was in error.
The correction was made at about 11:50 AM on March 20, 2011.
_____________________________________________________________
Update of March 21
The problem here is of course with the definition. Sloane and Glynn failed to include in their definition a condition that is common in other definitions of configurations, even abstract or purely "combinatorial" configurations. See, for instance, Configurations of Points and Lines , by Branko Grunbaum (American Mathematical Society, 2009), p. 17—
In the most general sense we shall consider combinatorial (or abstract) configurations; we shall use the term set-configurations as well. In this setting "points" are interpreted as any symbols (usually letters or integers), and "lines" are families of such symbols; "incidence" means that a "point" is an element of a "line". It follows that combinatorial configurations are special kinds of general incidence structures. Occasionally, in order to simplify and clarify the language, for "points" we shall use the term marks, and for "lines" we shall use blocks. The main property of geometric configurations that is preserved in the generalization to set-configurations (and that characterizes such configurations) is that two marks are incident with at most one block, and two blocks with at most one mark.
Whether or not omitting this "at most one" condition from the definition is aesthetically the best choice, it dramatically changes the number of configurations in the resulting theory, as the above (8_3) examples show.
Update of March 22 (itself updated on March 25)
For further background on configurations, see Dolgachev—
Note that the two examples Dolgachev mentions here, with 16 points and 9 points, are not unrelated to the geometry of 4×4 and 3×3 square arrays. For the Kummer and related 16-point configurations, see section 10.3, "The Three Biplanes of Order 4," in Burkard Polster's A Geometrical Picture Book (Springer, 1998). See also the 4×4 array described by Gordon Royle in an undated web page and in 1980 by Assmus and Sardi. For the Hesse configuration, see (for instance) the passage from Coxeter quoted in Quaternions in an Affine Galois Plane.
Update of March 27
See the above link to the (16,6) 4×4 array and the (16,6) exercises using this array in R.D. Carmichael's classic Introduction to the Theory of Groups of Finite Order (1937), pp. 42-43. For a connection of this sort of 4×4 geometry to the geometry of the diamond theorem, read "The 2-subsets of a 6-set are the points of a PG(3,2)" (a note from 1986) in light of R.W.H.T. Hudson's 1905 classic Kummer's Quartic Surface , pages 8-9, 16-17, 44-45, 76-77, 78-79, and 80.
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—
This journal on January 19, 2011—
If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
"Rosetta Stone" as a Metaphor
in Mathematical Narratives
For some backgound, see Mathematics and Narrative from 2005.
Yesterday's posts on mathematics and narrative discussed some properties
of the 3×3 grid (also known as the ninefold square ).
For some other properties, see (at the college-undergraduate, or MAA, level)–
Ezra Brown, 2001, "Magic Squares, Finite Planes, and Points of Inflection on Elliptic Curves."
His conclusion:
When you are done, you will be able to arrange the points into [a] 3×3 magic square,
which resembles the one in the book [5] I was reading on elliptic curves….
This result ties together threads from finite geometry, recreational mathematics,
combinatorics, calculus, algebra, and number theory. Quite a feat!
5. Viktor Prasolov and Yuri Solvyev, Elliptic Functions and Elliptic Integrals ,
American Mathematical Society, 1997.
Brown fails to give an important clue to the historical background of this topic —
the word Hessian . (See, however, this word in the book on elliptic functions that he cites.)
Investigation of this word yields a related essay at the graduate-student, or AMS, level–
Igor Dolgachev and Michela Artebani, 2009, "The Hesse Pencil of Plane Cubic Curves ."
From the Dolgachev-Artebani introduction–
In this paper we discuss some old and new results about the widely known Hesse
configuration of 9 points and 12 lines in the projective plane P2(k ): each point lies
on 4 lines and each line contains 3 points, giving an abstract configuration (123, 94).
PlanetMath.org on the Hesse configuration—
A picture of the Hesse configuration–
(See Visualizing GL(2,p), a note from 1985).
Related notes from this journal —
From last November —
|
From the December 2010 American Mathematical Society Notices—
Related material from this journal— Consolation Prize (August 19, 2010) |
From 2006 —
|
Sunday December 10, 2006
“Function defined form, expressed in a pure geometry
– J. G. Ballard on Modernism
“The greatest obstacle to discovery is not ignorance –
— Daniel J. Boorstin, |
Also from 2006 —
|
Sunday November 26, 2006
Rosalind Krauss "If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete. Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
"And it's whispered that soon if we all call the tune
The nine engravings of The Club Dumas
An example of the universal*– or, according to Krauss,
"This is the garden of Apollo, the field of Reason…."
For more on the field of reason, see
A reasonable set of "strange correspondences" Unreason is, of course, more popular. * The ninefold square is perhaps a "concrete universal" in the sense of Hegel: "Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
– Hegel, Lectures on the History of Philosophy ,
"For every kind of vampire, |
And from last October —
|
Friday, October 8, 2010
Starting Out in the Evening This post was suggested by last evening's post on mathematics and narrative and by Michiko Kakutani on Vargas Llosa in this morning's New York Times .
"One must proceed cautiously, for this road— of truth and falsehood in the realm of fiction— is riddled with traps and any enticing oasis is usually a mirage."
– "Is Fiction the Art of Lying?"* by Mario Vargas Llosa,
* The Web version's title has a misprint— |
Leading today's New York Times obituaries —
— is that of Nassos Daphnis, a painter of geometric abstractions
who in 1995 had an exhibition at a Leo Castelli gallery
titled "Energies in Outer Space." (See pictures here.)
Daphnis died, according to the Times, on November 23.
See Art Object, a post in this journal on that date—
There is more than one way
to look at a cube.
Some context— this morning's previous post (Apollo's 13,
on the geometry of the 3×3×3 cube), yesterday's noon post
featuring the 3×3 square grid (said to be a symbol of Apollo),
and, for connoisseurs of the Ed Wood school of cinematic art,
a search in this journal for the phrase "Plan 9."
You can't make this stuff up.
Apollo's 13: A Group Theory Narrative —
I. At Wikipedia —
II. Here —
See Cube Spaces and Cubist Geometries.
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the 27-part (Galois) 3×3×3 cube–
A note from 1985 describing group actions on a 3×3 plane array—
Undated software by Ed Pegg Jr. displays
group actions on a 3×3×3 cube that extend the
3×3 group actions from 1985 described above—
Pegg gives no reference to the 1985 work on group actions.
A picture one might view as
related to the novel An Object of Beauty
and the film "The Object of Beauty" —
"If it's a seamless whole you want,
pray to Apollo." — Margaret Atwood
The Turning
"To everything, turn, turn, turn…
… there is a season, turn, turn, turn…"
For less turning and more seasons, see a search in this journal for
fullness + multitude + "cold mountain."
From Doonesbury today—
From this journal (September 20, 2009)—
|
scheinen
Quine, Pursuit of Truth,
Google search:
|
For Kathrin Bringmann, who has been mentioned as a possible candidate for a Fields Medal.
The four Fields medal winners were announced today at the International Congress of Mathematicians in Hyderabad, India. Bringmann was not among them.
Bringmann was, however, the winner of the 2009 SASTRA Ramanujan Prize—
See The Hindu of September 30, 2009 and this journal on that date—
For more about Bringmann's work, see an article on what has been called Ramanujan's "final problem."
For another problem with a claim to this title, see "Mathematician Untangles Legendary Problem" and search in this journal for Dyson + crank.
Cubic models of finite geometries
display an interplay between
Euclidean and Galois geometry.
Example 1— The 2×2×2 Cube—
also known as the eightfold cube—
Group actions on the eightfold cube, 1984—
Version by Laszlo Lovasz et al., 2003—
Lovasz et al. go on to describe the same group actions
as in the 1984 note, without attribution.
Example 2— The 3×3×3 Cube
A note from 1985 describing group actions on a 3×3 plane array—
Undated software by Ed Pegg Jr. displays
group actions on a 3×3×3 cube that extend the
3×3 group actions from 1985 described above—
Pegg gives no reference to the 1985 work on group actions.
Example 3— The 4×4×4 Cube
A note from 27 years ago today—
As far as I know, this version of the
group-actions theorem has not yet been ripped off.
“What on earth is a 'concrete universal'?"
— Said to be an annotation (undated)
by Robert M. Pirsig of A History of Philosophy,
by Frederick Copleston, Society of Jesus.
From Aaron Urbanczyk's 2005 review of Christ and Apollo by William Lynch, S.J., a book first published in 1960—
"Lynch's use of analogy vis-a-vis literature provides, in a sense, a philosophical basis to the theoretical paradox popularized by W. K. Wimsatt (1907-1975), which contends that literature is a sort of 'concrete universal.'"
The following figure has often been
offered in this journal as a symbol of Apollo—
Arguments that it is, rather, a symbol of Christ
may be left to the Society of Jesus.
One possible approach—
Urbanczyk's review says that
"Christianity offers the critic
a privileged ontological window…."
"The world was warm and white when I was born:
Beyond the windowpane the world was white,
A glaring whiteness in a leaded frame,
Yet warm as in the hearth and heart of light."
天鈞
Made famous by Ursula K. Le Guin
as the book title "Lathe of Heaven,"
this Chinese phrase, tianjun, apparently
means something more like "Scales of Heaven"–
an appropriate image for Law Day 2010.
An anonymous forum user says that
"…if you switch the two characters around,
you get: 鈞天, which is one of
the nine heavens, more specifically,
the middle heaven."
This is supported by a
non-anonymous source:
"I follow A.C. Graham’s translation of
Juntian as 'Level Heaven (the innermost
of the nine divisions of heaven)';
he renders Juntian guangyue as
'the mighty music of the innermost heaven.'"
— "Music in the World of Su Shi (1037-1101):
Terminology," by Stuart H. Sargent,
Colorado State University,
Journal of Sung-Yuan Studies 32 (2002), 39-81
The Nine Divisions of Heaven–
Some context–
"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
Some historians consider today's date, April 7, to be the date of the Crucifixion in the Roman calendar (a solar calendar, as opposed to the Jewish lunar scheme).
Since the ninefold square has been called both a symbol of Apollo and the matrix of a cross, it will serve as an icon for today–
Adapted from
Ad Reinhardt
"The cube has…13 axes of symmetry:
6 C2 (axes joining midpoints of opposite edges),
4 C3 (space diagonals), and
3C4 (axes joining opposite face centroids)."
–Wolfram MathWorld article on the cube
These 13 symmetry axes can be used to illustrate the interplay between Euclidean and Galois geometry in a cubic model of the 13-point Galois plane.
The geometer's 3×3×3 cube–
27 separate subcubes unconnected
by any Rubik-like mechanism–
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
A closely related structure–
the finite projective plane
with 13 points and 13 lines–
A later version of the 13-point plane
by Ed Pegg Jr.–
A group action on the 3×3×3 cube
as illustrated by a Wolfram program
by Ed Pegg Jr. (undated, but closely
related to a March 26, 1985 note
by Steven H. Cullinane)–
The above images tell a story of sorts.
The moral of the story–
Galois projective geometries can be viewed
in the context of the larger affine geometries
from which they are derived.
The standard definition of points in a Galois projective plane is that they are lines through the (arbitrarily chosen) origin in a corresponding affine 3-space converted to a vector 3-space.
If we choose the origin as the center cube in coordinatizing the 3×3×3 cube (See Weyl's relativity problem ), then the cube's 13 axes of symmetry can, if the other 26 cubes have properly (Weyl's "objectively") chosen coordinates, illustrate nicely the 13 projective points derived from the 27 affine points in the cube model.
The 13 lines of the resulting Galois projective plane may be derived from Euclidean planes through the cube's center point that are perpendicular to the cube's 13 Euclidean symmetry axes.
The above standard definition of points in a Galois projective plane may of course also be used in a simpler structure– the eightfold cube.
(The eightfold cube also allows a less standard way to picture projective points that is related to the symmetries of "diamond" patterns formed by group actions on graphic designs.)
See also Ed Pegg Jr. on finite geometry on May 30, 2006
at the Mathematical Association of America.
| “Zhu Xi maintained that all things are brought into being by the union of two universal aspects of reality: qi, sometimes translated as vital (or physical, material) force; and li, sometimes translated as rational principle (or law).” —Wikipedia |
| “Drop off the key, Lee” — Paul Simon
Reference frame (Click for details.) According to Chu Hsi [Zhu Xi],
“Li” is “the principle or coherence or order or pattern underlying the cosmos.” – Smith, Bol, Adler, and Wyatt, Sung Dynasty Uses of the I Ching, Related material: Dynasty and |
|
January 06, 2007 Picture of Nothing
“Varnedoe’s lectures were ultimately about faith, about his faith in the power of abstraction, and abstraction as a kind of anti-religious faith in itself….” Related material: The more industrious scholars will derive considerable pleasure from describing how the art-history professors and journalists of the period 1945-75, along with so many students, intellectuals, and art tourists of every sort, actually struggled to see the paintings directly, in the old pre-World War II way, like Plato’s cave dwellers watching the shadows, without knowing what had projected them, which was the Word.” — Tom Wolfe, The Painted Word “Concept (scholastics’ verbum mentis)– theological analogy of Son’s procession as Verbum Patris, 111-12″ — Index to Joyce and Aquinas, by William T. Noon, S.J., Yale University Press 1957, second printing 1963, page 162
“So did God cause the big bang? Overcome by metaphysical lassitude, I finally reach over to my bookshelf for The Devil’s Bible. Turning to Genesis I read: ‘In the beginning there was nothing. And God said, ‘Let there be light!’ And there was still nothing, but now you could see it.'”
— Jim Holt, Big-Bang Theology, from Slate‘s “High Concept” department  “Bang.” “…Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal….” For properties of the “nothing” represented by the 3×3 grid, see The Field of Reason. For religious material related to the above and to Epiphany, a holy day observed by some, see Plato, Pegasus, and the Evening Star and Shining Forth.
|
Some Context:
See also Nativity.
This morning’s New York Times reports the deaths of Nuremberg interrogator Richard W. Sonnenfeldt and of avant-garde novelist and Beckett scholar Raymond Federman.
Symbols from this journal on the dates of their deaths:
— James Joyce, Finnegans Wake
"…strict grids of nine pictures
establish an egalitarian
framework…."
Some are more
egalitarian
than others.
Midnight in the Garden, Autumn 2009
Review:
|
From this journal on the following day, Sept. 21:
Happy birthday, Stephen King.
Today's previous entry is based on a song, "Unthought Known,"
from the above album; the cover of the album uses the 3×3 grid
shown in Sept. 20's midnight review. For related material
on the unconscious, see June 13-15, 2005.
I know more than Apollo,
For oft when he lies sleeping
I see the stars at mortal wars
In the wounded welkin weeping.
“Music and mathematics are among the pre-eminent wonders of the race. Levi-Strauss sees in the invention of melody ‘a key to the supreme mystery’ of man– a clue, could we but follow it, to the singular structure and genius of the species. The power of mathematics to devise actions for reasons as subtle, witty, manifold as any offered by sensory experience and to move forward in an endless unfolding of self-creating life is one of the strange, deep marks man leaves on the world. Chess, on the other hand, is a game in which thirty-two bits of ivory, horn, wood, metal, or (in stalags) sawdust stuck together with shoe polish, are pushed around on sixty-four alternately coloured squares. To the addict, such a description is blasphemy. The origins of chess are shrouded in mists of controversy, but unquestionably this very ancient, trivial pastime has seemed to many exceptionally intelligent human beings of many races and centuries to constitute a reality, a focus for the emotions, as substantial as, often more substantial than, reality itself. Cards can come to mean the same absolute. But their magnetism is impure. A mania for whist or poker hooks into the obvious, universal magic of money. The financial element in chess, where it exists at all, has always been small or accidental.
To a true chess player, the pushing about of thirty-two counters on 8×8 squares is an end in itself, a whole world next to which that of a mere biological or political or social life seems messy, stale, and contingent. Even the patzer, the wretched amateur who charges out with his knight pawn when the opponent’s bishop decamps to R4, feels this daemonic spell. There are siren moments when quite normal creatures otherwise engaged, men such as Lenin and myself, feel like giving up everything– marriage, mortgages, careers, the Russian Revolution– in order to spend their days and nights moving little carved objects up and down a quadrate board. At the sight of a set, even the tawdriest of plastic pocket sets, one’s fingers arch and a coldness as in a light sleep steals over one’s spine. Not for gain, not for knowledge or reknown, but in some autistic enchantment, pure as one of Bach’s inverted canons or Euler’s formula for polyhedra.”
— George Steiner in “A Death of Kings,” The New Yorker, issue dated September 7, 1968, page 133
“Examples are the stained-glass windows of knowledge.” —Nabokov
Click above images for some context.
“Inside the church, the grief was real. Sen. Edward Kennedy’s voice caught as he read his lovely eulogy, and when he was done, Caroline Kennedy Schlossberg stood up and hugged him. She bravely read from Shakespeare’s ‘The Tempest‘ (‘Our revels now are ended. We are such stuff as dreams are made on‘). Many of the 315 mourners, family and friends of the Kennedys and Bessettes, swallowed hard through a gospel choir’s rendition of ‘Amazing Grace,’ and afterward, they sang lustily as Uncle Teddy led the old Irish songs at the wake.”
— Newsweek magazine, issue dated August 2, 1999
The Ba gua (Chinese….) are eight diagrams used in Taoist cosmology to represent a range of interrelated concepts. Each consists of three lines, each either ‘broken’ or ‘unbroken,’ representing a yin line or a yang line, respectively. Due to their tripartite structure, they are often referred to as ‘trigrams’ in English. —Wikipedia
Background:
| 1. "Jesus's Response to Dishonor" 2. For Stephen King, August 11, 2009 3. Art and Man at Yale |
A note from 1985 leads,
via today’s earlier entry,
to an article from 1993:
See also
Arnold’s Cat Map.
From a 1984 book review:
"After three decades of intensive research by hundreds of group theorists, the century old problem of the classification of the finite simple groups has been solved and the whole field has been drastically changed. A few years ago the one focus of attention was the program for the classification; now there are many active areas including the study of the connections between groups and geometries, sporadic groups and, especially, the representation theory. A spate of books on finite groups, of different breadths and on a variety of topics, has appeared, and it is a good time for this to happen. Moreover, the classification means that the view of the subject is quite different; even the most elementary treatment of groups should be modified, as we now know that all finite groups are made up of groups which, for the most part, are imitations of Lie groups using finite fields instead of the reals and complexes. The typical example of a finite group is
— Jonathan L. Alperin,
review of books on group theory,
Bulletin (New Series) of the American
Mathematical Society 10 (1984) 121, doi:
10.1090/S0273-0979-1984-15210-8
The same example
at Wolfram.com:
Citation data from Wolfram.com:
"GL(2,p) and GL(3,3) Acting on Points"
from The Wolfram Demonstrations Project,
http://demonstrations.wolfram.com/GL2PAndGL33ActingOnPoints/,
Contributed by: Ed Pegg Jr"
As well as displaying Cullinane's 48 pictures of group actions from 1985, the Pegg program displays many, many more actions of small finite general linear groups over finite fields. It illustrates Cullinane's 1985 statement:
"Actions of GL(2,p) on a p×p coordinate-array have the same sorts of symmetries, where p is any odd prime."
Pegg's program also illustrates actions on a cubical array– a 3×3×3 array acted on by GL(3,3). For some other actions on cubical arrays, see Cullinane's Finite Geometry of the Square and Cube.
Images from Log24 on
December 10, 2006 —
Nobel Prize Day, and
the day after
Kirk Douglas’s birthday—
Kirk Douglas ad for
the film “Diamonds”
(2000)
Images from
Google News
at noon today —
“The serpent’s eyes shine
as he wraps around the vine…”
Just What We Need
Thomas Pynchon's new novel
Inherent Vice comes out today.
Title of a review in
The New York Times:
Another Doorway to the
Paranoid Pynchon Dimension
More interesting doorways:
An Aleph for Pynchon (July 9)
Click on the doorway for details.
|
|
|
The Aleph (July 8)
Click on the aleph for details.
mentioned in
this afternoon's entry
"Emblematizing the Modern"
was the object of a recent
cinematic chase sequence
(successful and inspiring)
starring Mira Sorvino
at the Metropolitan
Museum of Art.
In memory of
Dr. Hunter S. Thompson,
dead by his own hand
on this date
four years ago —
There is
another sort of object
we may associate with a
different museum and with
a modern Constantine …
See "Art Wars for MoMA"
(Dec. 14, 2008).
This object, modern
rather than medieval,
is the ninefold square:
It may suit those who,
like Rosalind Krauss
(see "Emblematizing"),
admire the grids of modern art
but view any sort of Christian
cross with fear and loathing.
For some background that
Dr. Thompson might appreciate,
see notes on Geometry and Death
in this journal, June 1-15, 2007,
and the five Log24 entries
ending at 9 AM Dec. 10. 2006,
which include this astute
observation by J. G. Ballard:
Selah.
A Sunrise
for Sunrise
“If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World, for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.”
Yesterday’s entry featured a rather simple-minded example from Krauss of how the ninefold square (said to be a symbol of Apollo)
may be used to create a graphic design– a Greek cross, which appears also in crossword puzzles:
Illustration by
Paul Rand
(born Peretz Rosenbaum)
A more sophisticated example
of the ninefold square
in graphic design:
“That old Jew
gave me this here.”
— A Flag for Sunrise
Against the Day
is a novel by Thomas Pynchon
published on Nov. 21, 2006, in
hardcover, and in paperback on
Oct. 30, 2007 (Devil's Night).
Perhaps the day the title
refers to is one of the above
dates… or perhaps it is–
|
The Candlebrow Conference in Pynchon's Against the Day: The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–"  Quaternion by S. H. Cullinane "Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
Introduction
For details of the story,
click on the images.
Chapter I:
Chapter II:
Chapter III:
and the following quotation:
"There is no landing fee in Avalon,
or anywhere else in Catalina."
A figure from
Nobel Prize day, December 10,
and from Eugene Wigner‘s
birthday, November 17:
Also on December 10:
the death of Constantine–
(Click for details.)
Related material:
Tina Modotti: A Fragile Life,
Photos by Tina Modotti,
Art Wars for Trotsky’s Birthday,
as well as
Art Wars, June 1-15, 2007:
“Ay que bonito es volar
A las dos de la mañana….”
— “La Bruja“
“If it’s a seamless whole you want,
pray to Apollo, who sets the limits
within which such a work can exist.”
— Margaret Atwood,
quoted here on
November 17, 2008
Related material:
A web page by
Nick Wedd at Oxford
with a neater version
of pictures I drew on
March 26, 1985
(Recall that Apollo is the god
of, among other things, reason.)
From the previous entry:
“If it’s a seamless whole you want,
pray to Apollo, who sets the limits
within which such a work can exist.”
— Margaret Atwood,
author of Cat’s Eye
Happy birthday
to the late
Eugene Wigner
… and a belated
Merry Christmas
to Paul Newman:
— Eugene Wigner, Nobel Prize Lecture, December 12, 1963
(continued from
June 15, 2007)
Today is the anniversary
of the 1955 death of poet
Wallace Stevens.
Related material:
an essay on the
relationships between
poets and philosophers —
“Bad Blood,” by
Leonard Michaels —
and
"Hancock" Powers to the Top
of July Fourth Box Office
— This evening's online
New York Times
|
New York Lottery
Sunday, July 6, 2008:
Mid-day 307
Evening 921 |
|
Symbols:
|
|
"The consolations of form,
the clean crystalline work"
— Iris Murdoch,
"Against Dryness" |
Will Smith
on Chess
Will Smith
"A devoted father, Smith passes on his philosophy of life to his children through chess, among other things.
'My father taught me how to play chess at seven and introduced beautiful concepts that I try to pass on to my kids. The elements and concepts of life are so perfectly illustrated on a chess board. The ability to accurately assess your position is the key to chess, which I also think is the key to life.'
He pauses, searching for an example. 'Everything you do in your life is a move. You wake up in the morning, you strap on a gun, and you walk out on the street– that's a move. You've made a move and the universe is going to respond with its move.
'Whatever move you're going to make in your life to be successful, you have to accurately access the next couple of moves– like what's going to happen if you do this? Because once you've made your move, you can't take it back. The universe is going to respond.'
Smith has just finished reading The Alchemist, by the Brazilian writer Paulo Coelho: 'It says the entire world is contained in one grain of sand, and you can learn everything you need to learn about the entire universe from that one grain of sand. That is the kind of concept I'm teaching my kids.'"
| CHANGE FEW CAN BELIEVE IN |
Page 67 —
“… Bill and Violet were married. The wedding was held in the Bowery loft on June 16th, the same day Joyce’s Jewish Ulysses had wandered around Dublin. A few minutes before the exchange of vows, I noted that Violet’s last name, Blom, was only an o away from Bloom, and that meaningless link led me to reflect on Bill’s name, Wechsler, which carries the German root for change, changing, and making change. Blooming and changing, I thought.”
For Hustvedt’s discussion of Wechsler’s art– sculptured cubes, which she calls “tightly orchestrated semantic bombs” (p. 169)– see Log24, May 25, 2008.
(after David Wechsler,
1896-1981, chief
psychologist at Bellevue)
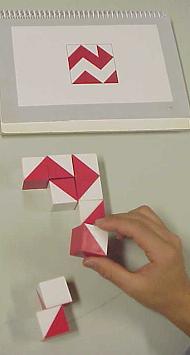
These cubes are used to
make 3×3 patterns for
psychological testing.
Related 3×3 patterns appear
in “nine-patch” quilt blocks
and in the following–
| Don Park at docuverse.com, Jan. 19, 2007: “How to draw an Identicon
A 9-block is a small quilt using only 3 types of patches, out of 16 available, in 9 positions. Using the identicon code, 3 patches are selected: one for center position, one for 4 sides, and one for 4 corners. Positions and Rotations For center position, only a symmetric patch is selected (patch 1, 5, 9, and 16). For corner and side positions, patch is rotated by 90 degree moving clock-wise starting from top-left position and top position respectively.” |
| From a weblog by Scott Sherrill-Mix:
“… Don Park came up with the original idea for representing users with geometric shapes….” Claire | 20-Dec-07 at 9:35 pm | Permalink “This reminds me of a flash demo by Jarred Tarbell |
| Jared Tarbell at levitated.net, May 15, 2002:
“The nine block is a common design pattern among quilters. Its construction methods and primitive building shapes are simple, yet produce millions of interesting variations.  Figure A. Four 9 block patterns,
arbitrarily assembled, show the grid composition of the block. Each block is composed of 9 squares, arranged in a 3 x 3 grid. Each square is composed of one of 16 primitive shapes. Shapes are arranged such that the block is radially symmetric. Color is modified and assigned arbitrarily to each new block.
The basic building blocks of the nine block are limited to 16 unique geometric shapes. Each shape is allowed to rotate in 90 degree increments. Only 4 shapes are allowed in the center position to maintain radial symmetry.
 Figure B. The 16 possible shapes allowed |
Such designs become of mathematical interest when their size is increased slightly, from square arrays of nine blocks to square arrays of sixteen. See Block Designs in Art and Mathematics.
(This entry was suggested by examples of 4×4 Identicons in use at Secret Blogging Seminar.)
"But not, perhaps,
in the Garden of Apollo":
— "Garden Party" —
Log24, April 9, 2007
Related material:
"When, on the last day of February 1953 Francis told her excitedly of the double helix discovery, she took no notice: 'He was always saying that kind of thing.' But when nine years later she heard the news of the Nobel Prize while out shopping, she immediately rushed to the fishmonger for ice to fill the bath and cool the champagne: a party was inevitable."
— Matt Ridley on Odile Crick (The Independent, July 20, 2007), who drew what "may be the most famous [scientific] drawing of the 20th century, in that it defines modern biology," according to Terrence J. Sejnowski, a neuroscientist at the Salk Institute for Biological Studies in La Jolla quoted by Adam Bernstein in The Washington Post, July 21, 2007
See also "Game Boy"
(Log24 on the Feast
of the Transfiguration–
August 6, 2006):
Rudolf Arnheim, a student of Gestalt psychology (which, an obituary notes, emphasizes "the perception of forms as organized wholes") was the first Professor of the Psychology of Art at Harvard. He died at 102 on Saturday, June 9, 2007.
The conclusion of yesterday's New York Times obituary of Arnheim:
"… in The New York Times Book Review in 1986, Celia McGee called Professor Arnheim 'the best kind of romantic,' adding, 'His wisdom, his patient explanations and lyrical enthusiasm are those of a teacher.'"
A related quotation:
"And you are teaching them a thing or two about yourself. They are learning that you are the living embodiment of two timeless characterizations of a teacher: 'I say what I mean, and I mean what I say' and 'We are going to keep doing this until we get it right.'"
Here, yet again, is an illustration that has often appeared in Log24– notably, on the date of Arnheim's death:
Related quotations:
"We have had a gutful of fast art and fast food. What we need more of is slow art: art that holds time as a vase holds water: art that grows out of modes of perception and whose skill and doggedness make you think and feel; art that isn't merely sensational, that doesn't get its message across in 10 seconds, that isn't falsely iconic, that hooks onto something deep-running in our natures. In a word, art that is the very opposite of mass media. For no spiritually authentic art can beat mass media at their own game."
— Robert Hughes, speech of June 2, 2004
"Whether the 3×3 square grid is fast art or slow art, truly or falsely iconic, perhaps depends upon the eye of the beholder."
If the beholder is Rudolf Arnheim, whom we may now suppose to be viewing the above figure in the afterlife, the 3×3 square is apparently slow art. Consider the following review of his 1982 book The Power of the Center:
"Arnheim deals with the significance of two kinds of visual organization, the concentric arrangement (as exemplified in a bull's-eye target) and the grid (as exemplified in a Cartesian coordinate system)….
It is proposed that the two structures of grid and target are the symbolic vehicles par excellence for two metaphysical/psychological stances. The concentric configuration is the visual/structural equivalent of an egocentric view of the world. The self is the center, and all distances exist in relation to the focal spectator. The concentric arrangement is a hermetic, impregnable pattern suited to conveying the idea of unity and other-worldly completeness. By contrast, the grid structure has no clear center, and suggests an infinite, featureless extension…. Taking these two ideal types of structural scaffold and their symbolic potential (cosmic, egocentric vs. terrestrial, uncentered) as given, Arnheim reveals how their underlying presence organizes works of art."
— Review of Rudolf Arnheim's The Power of the Center: A Study of Composition in the Visual Arts (Univ. of Calif. Press, 1982). Review by David A. Pariser, Studies in Art Education, Vol. 24, No. 3 (1983), pp. 210-213
Arnheim himself says in this book (pp. viii-ix) that "With all its virtues, the framework of verticals and horizontals has one grave defect. It has no center, and therefore it has no way of defining any particular location. Taken by itself, it is an endless expanse in which no one place can be distinguished from the next. This renders it incomplete for any mathematical, scientific, and artistic purpose. For his geometrical analysis, Descartes had to impose a center, the point where a pair of coordinates [sic] crossed. In doing so he borrowed from the other spatial system, the centric and cosmic one."
Students of art theory should, having read the above passages, discuss in what way the 3×3 square embodies both "ideal types of structural scaffold and their symbolic potential."
We may imagine such a discussion in an afterlife art class– in, perhaps, Purgatory rather than Heaven– that now includes Arnheim as well as Ernst Gombrich and Kirk Varnedoe.
Such a class would be one prerequisite for a more advanced course– Finite geometry of the square and cube.
June 9, the birthday of
Aaron Sorkin, a writer
mentioned in recent
Log24 entries, was also
the birthday of writer
Patricia Cornwell.
An illustration
from that date:
Cornwell's first book was
a biography of
Ruth Bell Graham,
A Time for Remembering.
"Seven is heaven,
Eight is a gate,
Nine is a vine."
The above scene from
The Best of Riverdance
furnishes an exercise in
what Victor Turner has called
“comparative symbology.”
The circular symbol at top
may be seen as representing
the solar deity Apollo,
Leader of the Muses.
The nine female dancers
may be seen as
the nine muses,
with Jean Butler
at the center
as Terpsichore,
Muse of Dance.
Related Material —
“This is the garden of Apollo,
the field of Reason….”
John Outram, architect
For another look at
Terpsichore in action,
see Jean Butler at
CRC Irish Dance Camp.
For those who prefer
a different sort of camp
there is of course
Xanadu.
I prefer Butler.
“And the fruit is rotten;
the serpent’s eyes shine
as he wraps around the vine
in the Garden of Allah.“
— Don Henley
But not, perhaps,
in the Garden of Apollo:
Click on the image
for further details.
The Logic of Dreams
From A Beautiful Mind–
“How could you,” began Mackey, “how could you, a mathematician, a man devoted to reason and logical proof…how could you believe that extraterrestrials are sending you messages? How could you believe that you are being recruited by aliens from outer space to save the world? How could you…?”
Nash looked up at last and fixed Mackey with an unblinking stare as cool and dispassionate as that of any bird or snake. “Because,” Nash said slowly in his soft, reasonable southern drawl, as if talking to himself, “the ideas I had about supernatural beings came to me the same way that my mathematical ideas did. So I took them seriously.”
Ideas:
A link in the 7/11 entry leads to a remark of Noel Gray on Plato’s Meno and “graphic austerity as the tool to bring to the surface, literally and figuratively, the inherent presence of geometry in the mind of the slave.”
Also Friday: an example of graphic austerity– indeed, Gray graphic austerity– in Log24:
(Related material: the Harvard Gazette of April 6, 2006, “Mathematician George W. Mackey, 90: Obituary“– “A memorial service will be held at Harvard’s Memorial Church on April 29 at 2 p.m.“)
Friday’s Pennsylvania evening number 038 tells two other parts of the story involving Mackey…
As Mackey himself might hope, the number may be regarded as a reference to the 38 impressive pages of Varadarajan’s “Mackey Memorial Lecture” (pdf).
More in the spirit of Nash, 38 may also be taken as a reference to Harvard’s old postal address, Cambridge 38, and to the year, 1938, that Mackey entered graduate study at Harvard, having completed his undergraduate studies at what is now Rice University.
Returning to the concept of graphic austerity, we may further simplify the already abstract chessboard figure above to obtain an illustration that has been called both “the field of reason” and “the Garden of Apollo” by an architect, John Outram, discussing his work at Mackey’s undergraduate alma mater:
Let us hope that Mackey,
a devotee of reason,
is now enjoying the company
of Apollo rather than that of
Tom O’Bedlam:
For John Nash on his birthday:
I know more than Apollo,
For oft when he lies sleeping
I see the stars at mortal wars
In the wounded welkin weeping.
and
“The False Artaxerxes:
Borges and the
Dream of Chess“
This entry was inspired by
Xanga footprints yesterday
from Virginia:
| 1. Virginia Weblog ART WARS: Time and the Grid |
3/6/2007 9:48 AM |
| 2. Virginia Weblog Sequel |
3/6/2007 11:38 AM |
| 3. Virginia Weblog Games and Truth |
3/6/2007 1:25 PM |
| 4. Virginia /item.aspx?user=m759&ta… The Transcendent Signified |
3/6/2007 5:15 PM |
| 5. Virginia /item.aspx?user=m759&ta… Zen and Language Games |
3/6/2007 5:16 PM |
| 6. Virginia /item.aspx?user=m759&ta… Balanchine’s Birthday |
3/6/2007 6:12 PM |
| 7. Virginia /item.aspx?user=m759&ta… The Agony and the Ya-Ya |
3/6/2007 6:12 PM |
| 8. Virginia /item.aspx?user=m759&ta… Directions Out |
3/6/2007 6:13 PM |
| 9. Virginia /item.aspx?user=m759&ta… The Four Last Things |
3/6/2007 6:13 PM |
Semantic Transparency
"… semantic transparency … would allow disparate systems to share some understanding of the actual concepts that are represented…"
— IBM Developer Works on October 7, 2003
"There is no neutral ground
that can serve as
a means of translating between
specialized (lower) ontologies."
There is, however,
"the field of reason"–
the 3×3 grid:
Click on grid
for details.
From a Log24 entry of January 7, 2007:
"One of the primary critiques of modernism that Learning from Las Vegas was engaged in, as Frederic [sic] Jameson clearly noted, was the dialectic between inside and outside and the assumption that the outside expressed the interior. Let's call this the modernist drive for 'expressive transparency.'"
— Aron Vinegar of Ohio State U., "Skepticism and the Ordinary: From Burnt Norton to Las Vegas"
From this week's New Yorker (issue dated Jan. 22, 2007)–
"A Life," by Zbigniew Herbert
(translated from the Polish by Alissa Vales):
I was a quiet boy a little sleepy and–amazingly–
unlike my peers–who were fond of adventures–
I didn't expect much–didn't look out the window
At school more diligent than able–docile stable
For the rest of the poem, click here.
From the Wikipedia article on Zbigniew Herbert:
"In modern poetry, Herbert advocated semantic transparence. In a talk given at a conference organized by the journal Odra he said: 'So not having pretensions to infallibility, but stating only my predilections, I would like to say that in contemporary poetry the poems that appeal to me the most are those in which I discern something I would call a quality of semantic transparency (a term borrowed from Husserl's logic). This semantic transparency is the characteristic of a sign consisting in this: that during the time when the sign is used, attention is directed towards the object denoted, and the sign itself does not hold the attention. The word is a window onto reality.'"
(Wikipedia cites as the source–
Herbert's talk at the meeting "Poet in face of the present day," organized by the "Odra" journal. Print version: Preface to: Zbigniew Herbert "Poezje," Panstwowy Instytut Wydawniczy, Warszawa 1998, ISBN 83-06-02667-5.)
Fom Nabokov's Transparent Things (pdf):
"Its ultimate vision was the incandescence of a book or a box grown completely transparent and hollow. This is, I believe, it: not the crude anguish of physical death but the incomparable pangs of the mysterious mental maneuver needed to pass from one state of being to another. Easy, you know, does it, son."
Related material:
| The diamond is used in modal logic to symbolize possibility. |
The 3×3 grid may also be used
to illustrate “possibility.” It leads,
as noted at finitegeometry.org, to
the famed “24-cell,” which may be
pictured either as the diamond
figure from Plato’s Meno —
Click for details.
— or as a figure
with 24 vertices:
Click for details.
The “diamond” version of the
24-cell seems unrelated to the
second version that shows all
vertices and edges, yet the
second version is implicit,
or hidden, in the first.
Hence “possibility.”
Neither version of the 24-cell
seems related in any obvious
way to the 3×3 grid, yet both
versions are implicit,
or hidden, in the grid.
Hence “possibility.”
(continued from
January 9, 2003)
George Balanchine
|
"What on earth is
a concrete universal?"
— Robert M. Pirsig
Review:
From Wikipedia's
"Upper Ontology"
and
Epiphany 2007:
"There is no neutral ground
that can serve as
a means of translating between
specialized (lower) ontologies."
There is, however,
"the field of reason"–
the 3×3 grid:
Click on grid
for details.
As Rosalind Krauss
has noted, some artists
regard the grid as
"a staircase to
the Universal."
Other artists regard
Epiphany itself as an
approach to
the Universal:
— Richard Kearney, 2005,
in The New Arcadia Review
Kearney (right) with
Martin Scorsese (left)
and Gregory Peck
in 1997.
— Richard Kearney, interview (pdf) in The Leuven Philosophy Newsletter, Vol. 14, 2005-2006
For more on "the possible," see Kearney's The God Who May Be, Diamonds Are Forever, and the conclusion of Mathematics and Narrative:
|
"We symbolize
logical necessity with the box  )and logical possibility with the diamond  )."
"The possibilia that exist,
— Michael Sudduth, |
Picture of Nothing
On Kirk Varnedoe’s
2003 Mellon Lectures,
“Pictures of Nothing“–
“Varnedoe’s lectures were ultimately
about faith, about his faith in
the power of abstraction,
and abstraction as a kind of
anti-religious faith in itself….”
Related material:
The more industrious scholars
will derive considerable pleasure
from describing how the art-history
professors and journalists of the period
1945-75, along with so many students,
intellectuals, and art tourists of every
sort, actually struggled to see the
paintings directly, in the old
pre-World War II way,
like Plato’s cave dwellers
watching the shadows, without
knowing what had projected them,
which was the Word.”
— Tom Wolfe, The Painted Word
“Concept (scholastics’ verbum mentis)–
theological analogy of Son’s procession
as Verbum Patris, 111-12″
— Index to Joyce and Aquinas,
by William T. Noon, S.J.,
Yale University Press 1957,
second printing 1963, page 162
“So did God cause the big bang?
Overcome by metaphysical lassitude,
I finally reach over to my bookshelf
for The Devil’s Bible.
Turning to Genesis I read:
‘In the beginning
there was nothing.
And God said,
‘Let there be light!’
And there was still nothing,
but now you could see it.'”
— Jim Holt, Big-Bang Theology,
Slate‘s “High Concept” department
“Bang.”
“…Mondrian and Malevich
are not discussing canvas
or pigment or graphite or
any other form of matter.
They are talking about
Being or Mind or Spirit.
From their point of view,
the grid is a staircase
to the Universal….”
For properties of the
“nothing” represented
by the 3×3 grid, see
The Field of Reason.
For religious material related
to the above and to Epiphany,
a holy day observed by some,
see Plato, Pegasus, and the
Evening Star and Shining Forth.
Click on picture
for related symbolism.
“This is the garden of Apollo,
the field of Reason….”
John Outram, architect
I need a photo-opportunity
In memory of Joseph Barbera–
co-creator ot the Flintstones–
who died yesterday, a photo
from today’s Washington Post:
Playing the role of
recording angel —
Halle Berry as
Rosetta Stone:
Related material:
“Citizen Stone“
and
“Putting the X in Xmas.”
"Time and chance
happeneth to them all."
— Ecclesiastes
The number 048
may be interpreted
as referring to…
"Function defined form,
expressed in a pure geometry
that the eye could easily grasp
in its entirety."
— J. G. Ballard on Modernism
(The Guardian, March 20, 2006)
"The greatest obstacle to discovery
is not ignorance —
it is the illusion of knowledge."
— Daniel J. Boorstin,
Librarian of Congress,
quoted in Beyond Geometry
"Like all men of the Library,
I have traveled in my youth."
— Jorge Luis Borges,
The Library of Babel
"Papá me mandó un artículo
de J. G. Ballard en el que
se refiere a cómo el lugar
de la muerte es central en
nuestra cultura contemporánea."
— Sonya Walger,
interview dated September 14
(Feast of the Triumph of the Cross),
Anno Domini 2006
Sonya Walger,
said to have been
born on D-Day,
the sixth of June,
in 1974
Walger's father is, like Borges,
from Argentina.
She "studied English Literature
at Christ Church College, Oxford,
where she received
a First Class degree…. "
"… un artículo de J. G. Ballard…."–
A Handful of Dust, by J. G. Ballard
(The Guardian, March 20, 2006):
"… The Atlantic wall was only part of a huge system of German fortifications that included the Siegfried line, submarine pens and huge flak towers that threatened the surrounding land like lines of Teutonic knights. Almost all had survived the war and seemed to be waiting for the next one, left behind by a race of warrior scientists obsessed with geometry and death.
Death was what the Atlantic wall and Siegfried line were all about….
… modernism of the heroic period, from 1920 to 1939, is dead, and it died first in the blockhouses of Utah beach and the Siegfried line…
Modernism's attempt to build a better world with the aid of science and technology now seems almost heroic. Bertolt Brecht, no fan of modernism, remarked that the mud, blood and carnage of the first world war trenches left its survivors longing for a future that resembled a white-tiled bathroom. Architects were in the vanguard of the new movement, led by Le Corbusier and the Bauhaus design school. The old models were thrown out. Function defined form, expressed in a pure geometry that the eye could easily grasp in its entirety."
"This is the garden of Apollo,
the field of Reason…."
— John Outram, architect
(Click on picture for details.)
Related material:
Rosalind Krauss
in "Grids," 1979:
"If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World, for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.
Or, to take a more up-to-date example…."
"He was looking at
the nine engravings
and at the circle,
checking strange
correspondences
between them."
— The Club Dumas,1993
"And it's whispered that soon
if we all call the tune
Then the piper will lead us
to reason."
— Robert Plant,1971
The nine engravings of
The Club Dumas
(filmed as "The Ninth Gate")
are perhaps more an example
of the concrete than of the
universal.
An example of the universal*–
or, according to Krauss, a
"staircase" to the universal–
is the ninefold square:
"This is the garden of Apollo,
the field of Reason…."
— John Outram, architect
For more on the field
of reason, see
Log24, Oct. 9, 2006.
A reasonable set of
"strange correspondences"
in the garden of Apollo
has been provided by Ezra Brown
in a mathematical essay (pdf).
Unreason is, of course,
more popular.
* The ninefold square is perhaps a "concrete universal" in the sense of Hegel:
"Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
— Hegel, Lectures on the History of Philosophy, "Idea of a Concrete Universal Unity"
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon
“This is the garden of Apollo,
the field of Reason….”
John Outram, architect
To Apollo (10/09/02)
Art Wars: Apollo and Dionysus (10/09/02)
Balanchine’s Birthday (01/09/03)
Art Theory for Yom Kippur (10/05/03)
A Form (05/22/04)
Ineluctable (05/27/04)
A Form, continued (06/05/04)
Parallelisms (06/06/04)
Ado (06/25/04)
Deep Game (06/26/04)
Gameplayers of Zen (06/27/04)
And So To Bed (06/29/04)
Translation Plane for Rosh Hashanah (09/15/04)
Derrida Dead (10/09/04)
The Nine (11/09/04)
From Tate to Plato (11/19/04)
Art History (05/11/05)
A Miniature Rosetta Stone (08/06/05)
High Concept (8/23/05)
High Concept, Continued (8/24/05)
Analogical Train of Thought (8/25/05)
Today’s Sermon: Magical Thinking (10/09/05)
Balance (10/31/05)
Matrix (11/01/05)
Seven is Heaven, Eight is a Gate (11/12/05)
Nine is a Vine (11/12/05)
Apollo and Christ (12/02/05)
Hamilton’s Whirligig (01/05/06)
Cross (01/06/06)
On Beauty (01/26/06)
Sunday Morning (01/29/06)
Centre (01/29/06)
New Haven (01/29/06)
Washington Ballet (02/05/06)
Catholic Schools Sermon (02/05/06)
The Logic of Apollo (02/05/06)
Game Boy (08/06/06)
Art Wars Continued: The Krauss Cross (09/13/06)
Art Wars Continued: Pandora’s Box (09/16/06)
The Pope in Plato’s Cave (09/16/06)
Today’s Birthdays (09/26/06)
Symbology 101 (09/26/06)
In the punctual centre of all circles white
Stands truly….
… and Bloom with his vast accumulation
Stands and regards and repeats the primitive lines.
— Wallace Stevens,
“From the Packet of Anacharsis”
Related material:
Balanchine’s Birthday.
On Beauty
“Something beautiful fills the mind yet invites the search for something beyond itself, something larger or something of the same scale with which it needs to be brought into relation. Beauty, according to its critics, causes us to gape and suspend all thought. This complaint is manifestly true: Odysseus does stand marveling before the palm; Odysseus is similarly incapacitated in front of Nausicaa; and Odysseus will soon, in Book 7, stand ‘gazing,’ in much the same way, at the season-immune orchards of King Alcinous, the pears, apples, and figs that bud on one branch while ripening on another, so that never during the cycling year do they cease to be in flower and in fruit. But simultaneously what is beautiful prompts the mind to move chronologically back in the search for precedents and parallels, to move forward into new acts of creation, to move conceptually over, to bring things into relation, and does all this with a kind of urgency as though one’s life depended on it.”
The above symbol of Apollo suggests, in accordance with Scarry’s remarks, larger structures. Two obvious structures are the affine 4-space over GF(3), with 81 points, and the affine plane over GF(32), also with 81 points. Less obvious are some related projective structures. Joseph Malkevitch has discussed the standard method of constructing GF(32) and the affine plane over that field, with 81 points, then constructing the related Desarguesian projective plane of order 9, with
Miniquaternion geometry: An introduction to the study of projective planes, by T. G. Room and P. B. Kirkpatrick. Cambridge Tracts in Mathematics and Mathematical Physics, No. 60. Cambridge University Press, London, 1971. viii+176 pp.
For “miniquaternions” of a different sort, see my entry on Visible Mathematics for Hamilton’s birthday last year:
A Miniature
Rosetta Stone
John Baez discussed (Sept. 6, 2003) the analogies of Weil, and he himself furnished another such Rosetta stone on a much smaller scale:
“… a 24-element group called the ‘binary tetrahedral group,’ a 24-element group called ‘SL(2,Z/3),’ and the vertices of a regular polytope in 4 dimensions called the ’24-cell.’ The most important fact is that these are all the same thing!”
For further details, see Wikipedia on the 24-cell, on special linear groups, and on Hurwitz quaternions,
The group SL(2,Z/3), also known as “SL(2,3),” is of course derived from the general linear group GL(2,3). For the relationship of this group to the quaternions, see the Log24 entry for August 4 (the birthdate of the discoverer of quaternions, Sir William Rowan Hamilton).
The 3×3 square shown above may, as my August 4 entry indicates, be used to picture the quaternions and, more generally, the 48-element group GL(2,3). It may therefore be regarded as the structure underlying the miniature Rosetta stone described by Baez.
“The typical example of a finite group is GL(n,q), the general linear group of n dimensions over the field with q elements. The student who is introduced to the subject with other examples is being completely misled.”
— J. L. Alperin, book review,
Bulletin (New Series) of the American
Mathematical Society 10 (1984), 121
From Tate to Plato
In honor of Allen Tate's birthday (today)
and of the MoMA re-opening (tomorrow)
"For Allen Tate the concept of tension was the most useful formal tool at the critic’s disposal, as irony and paradox were for Brooks. The principle of tension sustains the whole structure of meaning, and, as Tate declares in Tension in Poetry (1938), he derives it from lopping the prefixes off the logical terms extension and intension (which define the abstract and denotative aspect of the poetic language and, respectively, the concrete and connotative one). The meaning of the poem is 'the full organized body of all the extension and intension that we can find in it.' There is an infinite line between extreme extension and extreme intension and the readers select the meaning at the point they wish along that line, according to their personal drives, interests or approaches. Thus the Platonist will tend to stay near the extension end, for he is more interested in deriving an abstraction of the object into a universal…."
— from Form, Structure, and Structurality,
by Radu Surdulescu
"Eliot, in a conception comparable to Wallace Stevens' 'Anecdote of the Jar,' has suggested how art conquers time:
Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness."
— F. O. Matthiessen
in The Achievement of T.S. Eliot,
Oxford University Press, 1958
From Writing Chinese Characters:
"It is practical to think of a character centered within an imaginary square grid…. The grid can… be… subdivided, usually to 9 or 16 squares…."
These "Chinese jars"
(as opposed to their contents)
are as follows:
Various previous Log24.net entries have
dealt with the 3×3 "form" or "pattern"
(to use the terms of T. S. Eliot).
For the 4×4 form, see Poetry's Bones
and Geometry of the 4×4 Square.
From Lullus to Cognitive Semantics:
The Evolution of a Theory of Semantic Fields
by Wolfgang Wildgen and in
Another Page in the Foundation of Semiotics:
A Book Review of On the Composition of Images, Signs & Ideas, by Giordano Bruno…
by Mihai Nadin
“We have had a gutful of fast art and fast food. What we need more of is slow art: art that holds time as a vase holds water: art that grows out of modes of perception and whose skill and doggedness make you think and feel; art that isn’t merely sensational, that doesn’t get its message across in 10 seconds, that isn’t falsely iconic, that hooks onto something deep-running in our natures. In a word, art that is the very opposite of mass media. For no spiritually authentic art can beat mass media at their own game.”
— Robert Hughes, speech of June 2, 2004
Whether the 3×3 square grid is fast art or slow art, truly or falsely iconic, perhaps depends upon the eye of the beholder.
For a meditation on the related 4×4 square grid as “art that holds time,” see Time Fold.
ART WARS:
Graphical Password
From a summary of “The Design and Analysis of Graphical Passwords“:
“Results from cognitive science show that people can remember pictures much better than words….
The 5×5 grid creates a good balance between security and memorability.”
— Ian Jermyn, New York University; Alain Mayer, Fabian Monrose, Michael K. Reiter, Bell Labs, Lucent Technologies; Aviel Rubin, AT&T Labs — Research
Illustration — Warren Beatty as
a graphical password:
“Town & Country,”
released April 27, 2001
Those who prefer the simplicity of a 3×3 grid are referred to my entry of Jan. 9, 2003, Balanchine’s Birthday. For material related to the “Town & Country” theme and to Balanchine, see Leadbelly Under the Volcano (Jan. 27, 2003). (“Sometimes I live in the country, sometimes I live in town…” – Huddie Ledbetter). Those with more sophisticated tastes may prefer the work of Stephen Ledbetter on Gershwin’s piano preludes or, in view of Warren Beatty’s architectural work in “Town & Country,” the work of Stephen R. Ledbetter on window architecture.
As noted in Balanchine’s Birthday, Apollo (of the Balanchine ballet) has been associated by an architect with the 3×3, or “ninefold” grid. The reader who wishes a deeper meditation on the number nine, related to the “Town & Country” theme and more suited to the fact that April is Poetry Month, is referred to my note of April 27 two years ago, Nine Gates to the Temple of Poetry.
Intermediate between the simplicity of the 3×3 square and the (apparent) complexity of the 5×5 square, the 4×4 square offers an introduction to geometrical concepts that appears deceptively simple, but is in reality fiendishly complex. See Geometry for Jews. The moral of this megilla?
32 + 42 = 52.
Balanchine's Birthday
Today seems an appropriate day to celebrate Apollo and the nine Muses.
From a website on Balanchine's and Stravinsky's ballet, "Apollon Musagete":
In his Poetics of Music (1942) Stravinsky says: "Summing up: What is important for the lucid ordering of the work– for its crystallization– is that all the Dionysian elements which set the imagination of the artist in motion and make the life-sap rise must be properly subjugated before they intoxicate us, and must finally be made to submit to the law: Apollo demands it." Stravinsky conceived Apollo as a ballet blanc– a "white ballet" with classical choreography and monochromatic attire. Envisioning the work in his mind's eye, he found that "the absence of many-colored hues and of all superfluities produced a wonderful freshness." Upon first hearing Apollo, Diaghilev found it "music somehow not of this world, but from somewhere else above." The ballet closes with an Apotheosis in which Apollo leads the Muses towards Parnassus. Here, the gravely beautiful music with which the work began is truly recapitulated "on high"– ceaselessly recycled, frozen in time.
— Joseph Horowitz
Another website invoking Apollo:
The icon that I use… is the nine-fold square…. The nine-fold square has centre, periphery, axes and diagonals. But all are present only in their bare essentials. It is also a sequence of eight triads. Four pass through the centre and four do not. This is the garden of Apollo, the field of Reason….
In accordance with these remarks, here is the underlying structure for a ballet blanc:
This structure may seem too simple to support movements of interest, but consider the following (click to enlarge):
As Sir Arthur Quiller-Couch, paraphrasing Horace, remarks in his Whitsun, 1939, preface to the new edition of the Oxford Book of English Verse, "tamen usque recurret Apollo."
The alert reader will note that in the above diagrams, only eight of the positions move.
Which muse remains at the center?
Consider the remark of T. S. Eliot, "At the still point, there the dance is," and the fact that on the day Eliot turned 60, Olivia Newton-John was born. How, indeed, in the words of another "sixty-year-old smiling public man," can we know the dancer from the dance?
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.
Powered by WordPress
