From this evening's online New York Times , a death from yesterday—
Mr. Hardy’s song “St. Clare” was covered by Ms. Vega
and appears on her 2001 album “Songs in Red and Gray.”
[Lyrics here.]
See also "red and gray" and "The Eye" in this journal.
From this evening's online New York Times , a death from yesterday—
Mr. Hardy’s song “St. Clare” was covered by Ms. Vega
and appears on her 2001 album “Songs in Red and Gray.”
[Lyrics here.]
See also "red and gray" and "The Eye" in this journal.
Today's New York Lottery numbers:
Continuing the Serious Hardy Apology sequence,
here is a reference to volume number 231 in the
Springer Graduate Texts in Mathematics series—
For some less serious work, see posts on 4403 (4/4/03)
as well as posts numbered 550 and 764.
"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy, A Mathematician's Apology
"Beauty is the first test." — G. H. Hardy, A Mathematician's Apology
“Chess problems are the
hymn-tunes of mathematics.”
— G. H. Hardy,
A Mathematician’s Apology

|

|

“The key is the cocktail that begins the proceedings.”
– Brian Harley, Mate in Two Moves

"I named this script ocode and chmod 755'd it to make it executable…"
— Software forum post on the OCR program Tesseract
From the author of
The Pearly Gates of Cyberspace:
"Like so many other heroes
who have seen the light
of a higher order…."
Epigraph to The Dark Interval , by John Dominic Crossan —
“I am the pause between two notes that fall
into a real accordance scarce at all:
for Death’s note tends to dominate—
Both, though, are reconciled in the dark interval,
tremblingly.
And the song remains immaculate.”
—Rilke, The Book of Hours , I


"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy in A Mathematician's Apology
"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy,
A Mathematician's Apology
G. H. Hardy in A Mathematician's Apology —
|
What ‘purely aesthetic’ qualities can we distinguish in such theorems as Euclid’s or Pythagoras’s? I will not risk more than a few disjointed remarks. In both theorems (and in the theorems, of course, I include the proofs) there is a very high degree of unexpectedness, combined with inevitability and economy. The arguments take so odd and surprising a form; the weapons used seem so childishly simple when compared with the far-reaching results; but there is no escape from the conclusions. There are no complications of detail—one line of attack is enough in each case; and this is true too of the proofs of many much more difficult theorems, the full appreciation of which demands quite a high degree of technical proficiency. We do not want many ‘variations’ in the proof of a mathematical theorem: ‘enumeration of cases’, indeed, is one of the duller forms of mathematical argument. A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way. |
Related material:

Yesterday's online LA Times had an obituary for a
traveling salesman:
"Besides writing and teaching, Borg was a frequent speaker,
usually racking up 100,000 frequent flier miles a year.
He and Crossan, along with their wives, led annual tours
to Turkey to follow the path of the Apostle Paul and to give
a sense of his world. They also led tours to Ireland to
showcase a different brand of Christianity."
Borg and Crossan were members of the Jesus Seminar.
For Crossan, see remarks on "The Story Theory of Truth."
See also, from the date of Borg's death, a different salesman joke.
Some backstory —


"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy in A Mathematician's Apology
"The character and events depicted in this
motion picture are fictitious. Any similarity
to actual persons, living or dead, is purely
coincidental."
— Ending credits of the 2012 film
"Travelling Salesman"
From that film's introduction to the
main character:
"He is presently the Rouse Ball Professor
of Mathematics in the Department of
Pure Mathematics and Mathematical Statistics
at Cambridge University and a fellow at
Trinity College. In 2008… he was awarded
the greatest honor in our profession
when he was presented with the Fields Medal
….
Ladies and gentlemen, it is with great honor
that I now present to you Dr. Timothy Horton."
See also…
A line for a fellow of Trinity:
"What am I, the farmer's daughter?"
“I can hardly do better than go back to the Greeks.”
— G. H. Hardy in A Mathematician's Apology
See also Hardy and Brosnan in today's earlier post Stark and Bleak.
C. P. Snow on G. H. Hardy in the foreword to
A Mathematician's Apology :
"… he had another favourite entertainment.
'Mark that man we met last night,'
he said, and someone had to be marked
out of 100 in each of the categories
Hardy had long since invented and defined.
STARK, BLEAK ('a stark man is not necessarily
bleak: but all bleak men without exception
want to be considered stark')…."
Related material :
Tommy Lee Jones in The New York Times on Nov. 6th, 2014,
and Pierce Brosnan in the 2014 film "The November Man" :


“Geometry was very important to us in this movie.”
— The Missing ART (Log24, November 7th, 2014)
"… this notion of ‘depth’ is an elusive one
even for a mathematician who can recognize it…."
— G. H. Hardy, A Mathematician's Apology
Part I: An Inch Deep

Part II: An Inch Wide
See a search for "square inch space" in this journal.
See also recent posts with the tag depth.
"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy, A Mathematician's Apology
The diamond theorem group, published without acknowledgment
of its source by the Mathematical Association of America in 2011—
Inscribed hexagon (1984)
The well-known fact that a regular hexagon
may be inscribed in a cube was the basis
in 1984 for two ways of coloring the faces
of a cube that serve to illustrate some graphic
aspects of embodied Galois geometry—
Inscribed hexagon (2013)
A redefinition of the term "symmetry plane"
also uses the well-known inscription
of a regular hexagon in the cube—
Related material
"Here is another way to present the deep question 1984 raises…."
— "The Quest for Permanent Novelty," by Michael W. Clune,
The Chronicle of Higher Education , Feb. 11, 2013
“What we do may be small, but it has a certain character of permanence.”
— G. H. Hardy, A Mathematician’s Apology
C. P. Snow on G. H. Hardy, in Snow's foreword to A Mathematician's Apology—
"One morning early in 1913, he found, among the letters on his breakfast table, a large untidy envelope decorated with Indian stamps. When he opened it, he found sheets of paper by no means fresh, on which, in a non-English holograph, were line after line of symbols. Hardy glanced at them without enthusiasm. He was by this time, at the age of thirty-six, a world famous mathematician: and world famous mathematicians, he had already discovered, are unusually exposed to cranks. He was accustomed to receiving manuscripts from strangers, proving the prophetic wisdom of the Great Pyramid, the revelations of the Elders of Zion, or the cryptograms that Bacon has inserted in the plays of the so-called Shakespeare."
Some related material (click to enlarge)—
The author links to, but does not name, the source of the above
"line after line of symbols." It is "Visualizing GL(2,p)." See that webpage
for some less esoteric background.
See also the two Wikipedia articles Finite geometry and Hesse configuration
and an image they share—
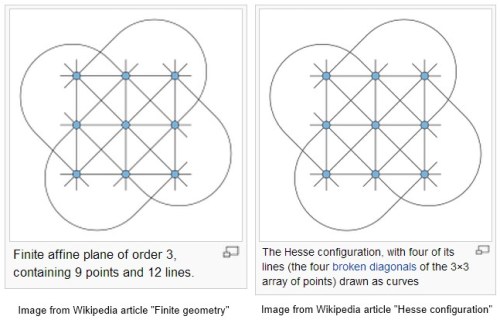
In memory of Sir Andrew Huxley, OM, who died on May 30, 2012
C. P. Snow on G. H. Hardy at Trinity College, Cambridge—
He played his games and indulged his eccentricities.
He was living in some of the best intellectual company
in the world— G. E. Moore, Whitehead, Bertrand Russell,
Trevelyan, the high Trinity society which was shortly to
find its artistic complement in Bloomsbury. (Hardy himself
had links with Bloomsbury, both of personal friendship
and of sympathy.)
See also "Max Black" + Trinity in this journal.
"… this notion of ‘depth’ is an elusive one
even for a mathematician who can recognize it…."
— G. H. Hardy, A Mathematician's Apology
In Geometry and the Imagination , Hilbert and Cohn-Vossen
describe the Brianchon-Pascal configuration of 9 points
and 9 lines, with 3 points on each line and 3 lines through
each point, as being "the most important configuration of all geometry."
The Brianchon-Pascal configuration is also known as the Pappus configuration—
"The Theorem of Pappus: A Bridge Between Algebra and Geometry"
Elena Anne Marchisotto
The American Mathematical Monthly
Vol. 109, No. 6 (Jun. – Jul., 2002), pp. 497-516
Wednesday's Marginal Remarks pictured Robert De Niro
and Sean Penn in "We're No Angels." De Niro appeared
again in a Saturday Night Live sketch linked to
in last night's 9:29 post.
Here are some remarks featuring Penn related to
Peter J. Cameron's description yesterday of Sudoku
as an example of mathematics.
(Recall that the symbol #, known as 'hash,"
can stand for checkmate.)
|
"Chess problems are the hymn-tunes of mathematics."
|
For a sample chess problem, see a post from Oct. 10, 2005,
the day that the Sudoku remark Cameron describes was
in the news.
From math16.com—
Quotations on Realism
|
The story of the diamond mine continues
(see Coordinated Steps and Organizing the Mine Workers)—
From The Search for Invariants (June 20, 2011):
The conclusion of Maja Lovrenov's
"The Role of Invariance in Cassirer’s Interpretation of the Theory of Relativity"—
"… physical theories prove to be theories of invariants
with regard to certain groups of transformations and
it is exactly the invariance that secures the objectivity
of a physical theory."
— SYNTHESIS PHILOSOPHICA 42 (2/2006), pp. 233–241

Related material from Sunday's New York Times travel section—
"Total grandeur of a total edifice,
Chosen by an inquisitor of structures
For himself. He stops upon this threshold,
As if the design of all his words takes form
And frame from thinking and is realized."
— Wallace Stevens, "To an Old Philosopher in Rome"
The following edifice may be lacking in grandeur,
and its properties as a configuration were known long
before I stumbled across a description of it… still…
"What we do may be small, but it has
a certain character of permanence…."
— G.H. Hardy, A Mathematician's Apology
The Kummer 166 Configuration
as seen by Kantor in 1969— (pdf, 2.5 MB)

For some background, see Configurations and Squares.
For some quite different geometry of the 4×4 square that is
original with me, see a page with that title. (The geometry's
importance depends in part on its connection with the
Miracle Octad Generator (MOG) of R.T. Curtis. I of course
had nothing to do with the MOG's discovery, but I do claim credit
for discovering some geometric properties of the 4×4 square
that constitutes two-thirds of the MOG as originally defined .)
Related material— The Schwartz Notes of June 1.
For the title, see Palm Sunday.
"There is a pleasantly discursive treatment of
Pontius Pilate's unanswered question 'What is truth?'" — H. S. M. Coxeter, 1987
From this date (April 22) last year—
 Richard J. Trudeau in The Non-Euclidean Revolution , chapter on "Geometry and the Diamond Theory of Truth"– "… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions: (1) Diamonds– informative, certain truths about the world– exist. Presumption (1) is what I referred to earlier as the 'Diamond Theory' of truth. It is far, far older than deductive geometry." Trudeau's book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called "Diamond Theory." Although non-Euclidean,* the theorems of the 1976 "Diamond Theory" are also, in Trudeau's terminology, diamonds. * "Non-Euclidean" here means merely "other than Euclidean." No violation of Euclid's parallel postulate is implied. |
Trudeau comes to reject what he calls the "Diamond Theory" of truth. The trouble with his argument is the phrase "about the world."
Geometry, a part of pure mathematics, is not about the world. See G. H. Hardy, A Mathematician's Apology .
Literary remarks for Maundy Thursday—

— C. P. Snow, foreword to G. H. Hardy's A Mathematician's Apology
Related material—
Emory University press release of January 20th, 2011:
"In 1937, Hans Rademacher found an exact formula for calculating partition values. While the method was a big improvement over Euler's exact formula, it required adding together infinitely many numbers that have infinitely many decimal places. 'These numbers are gruesome,' Ono says….
… The final eureka moment occurred near another Georgia landmark: Spaghetti Junction. Ono and Jan Bruinier were stuck in traffic near the notorious Atlanta interchange. While chatting in the car, they hit upon a way to overcome the infinite complexity of Rademacher's method. They went on to prove a formula that requires only finitely many simple numbers.
'We found a function, that we call P, that is like a magical oracle,' Ono says. 'I can take any number, plug it into P, and instantly calculate the partitions of that number….'"
See also this journal on April 15 and a Google Groups [sage-devel] thread, Ono-Bruinier partition formula. That thread started on April 15 and was last updated this morning.
Conclusion of “The Place of Pure Mathematics” —
“Dogmas and philosophies, it would seem, rise and fall. But gradually accumulating throughout the ages, from the earliest dawn of history, there is a body of doctrine, a reasoned insight into the relations of exact ideas, painfully won and often tested. And this remains the main heritage of man; his little beacon of light amidst the solitudes and darknesses of infinite space; or, if you prefer, like the shout of children at play together in the cultivated valleys, which continues from generation to generation.
Yes, and continues for ever! A universe which has the potentiality of becoming thus conscious of itself is not without something of which that which we call memory is but an image. Somewhere, somehow, in ways we dream not of, when you and I have merged again into the illimitable whole, when all that is material has ceased, the faculty in which we now have some share, shall surely endure; the conceptions we now dimly struggle to grasp, the joy we have in the effort, these are but part of a greater whole. Some may fear, and some may hope, that they and theirs shall not endure for ever. But he must have studied Nature in vain who does not see that our spiritual activities are inherent in the mighty process of which we are part; who can doubt of their persistence.
And, on the intellectual side, of all that is best ascertained, and surest, and most definite, of these; of all that is oldest and most universal; of all that is most fundamental and far-reaching, of these activities, Pure Mathematics is the symbol and the sum.”
— From a 1913 address by geometry saint Henry Frederick Baker, who died on this date in 1956
The feast of another saint, Patrick, also falls on 3/17. The date itself is related, if only by chance, to the following remark—
“317 is a prime, not because we think so,
or because our minds are shaped in one way
rather than another, but because it is so,
because mathematical reality is built that way.”
— From a 1940 book by the somewhat less saintly number theorist G. H. Hardy
Part 3 of 5 (See also Part 1 and Part 2) begins as follows…
"Incommensurable. It is a strange word. I wondered, why did Kuhn choose it? What was the attraction?
Here’s one clue. At the very end of 'The Road Since Structure,' a compendium of essays on Kuhn’s work, there is an interview with three Greek philosophers of science, Aristides Baltas, Kostas Gavroglu and Vassiliki Kindi. Kuhn provides a brief account of the historical origins of his idea. Here is the relevant segment of the interview.
T. KUHN: Look, 'incommensurability' is easy.
V. KINDI: You mean in mathematics?
T. KUHN: …When I was a bright high school mathematician and beginning to learn Calculus, somebody gave me—or maybe I asked for it because I’d heard about it—there was sort of a big two-volume Calculus book by, I can’t remember whom. And then I never really read it. I read the early parts of it. And early on it gives the proof of the irrationality of the square root of 2. And I thought it was beautiful. That was terribly exciting, and I learned what incommensurability was then and there. So, it was all ready for me, I mean, it was a metaphor but it got at nicely what I was after. So, that’s where I got it.
'It was all ready for me.' I thought, 'Wow.' The language was suggestive. I imagined √2 provocatively dressed, its lips rouged. But there was an unexpected surprise. The idea didn’t come from the physical sciences or philosophy or linguistics, but from mathematics ."
A footnote from Morris (no. 29)—
"Those who are familiar with the proof [of irrationality] certainly don’t want me to explain it here; likewise, those who are unfamiliar with it don’t want me to explain it here, either. There are many simple proofs in many histories of mathematics — E.T. Bell, Sir Thomas Heath, Morris Kline, etc., etc. Barry Mazur offers a proof in his book, 'Imagining Numbers (particularly the square root of minus fifteen),' New York, NY: Farrar, Straus and Giroux. 2003, 26ff. And there are two proofs in his essay, 'How Did Theaetetus Prove His Theorem?', available on Mazur’s Harvard Web site."
There may, actually, be a few who do want the proof. They may consult the sources Morris gives, or the excellent description by G.H. Hardy in A Mathematician's Apology , or, perhaps best of all for present purposes, the proof as described in a "sort of a big two-volume Calculus book" (perhaps the one Kuhn mentioned)… See page 6 and page 7 of Volume One of Richard Courant's classic Differential and Integral Calculus (second edition, 1937, reprinted many times through 1970, and again in a Wiley Classics Library Edition in 1988).
"I know no writing— except perhaps Henry James's introductory essays— which conveys so clearly and with such an absence of fuss the excitement of the creative artist."
— Graham Greene on A Mathematician's Apology , review in The Spectator , 20 December 1940
"The mere quality and play of an ironic consciousness in the designer left wholly alone, amid a chattering unperceiving world, with the thing he has most wanted to do, with the design more or less realised— some effectual glimpse of that might, by itself, for instance, reward one's experiment."
— Henry James, "Prefaces to the New York Edition," in The Figure in the Carpet and Other Stories, Penguin Books, 1986, with introduction and notes by Frank Kermode
"What? You've found a pattern?"
— Greg Egan, "Wang's Carpets"
See also Notes on Mathematics and Narrative, with its discussion of the tiles of the creative artist Patrick Blackburn in the recent (August 2010) Pythagorean novel The Thousand and the discussion of Wang tiles in Modal Logic, a book from November 2002 whose author also happens to be named Patrick Blackburn.
(Credit for the Greene bibliographic information is due to Janelle Robyn Humphreys, whose doctoral thesis, Shadows of Another Dimension, was published in 2009 by the University of Wollongong.)
"'A Disappearing Number'… is lucid, dynamic and continuously engaging."
"'All beautiful theorems require a very high degree of economy, unexpectedness and inevitability,' the string-theory* specialist Aninda tells us after elucidating one of Ramanujan’s formulas. That’s not a bad recipe for beautiful theater either…."
Related material:
Hardy is also the play's (apparently uncredited) source of "economy."
"… a very high degree of unexpectedness, combined with inevitability and economy."
— A Mathematician's Apology, §18, by G. H. Hardy, 1940
* For more on string theory and a deus, see Not Even Wrong, July 7, 2010.
Rigor
“317 is a prime, not because we think so,
or because our minds are shaped in one way
rather than another, but because it is so,
because mathematical reality is built that way.”
– G. H. Hardy,
A Mathematician’s Apology

The above photo is taken from
a post in this journal dated
March 10, 2010.
This was, as the Pope might say,
the dies natalis of a master gameplayer–
| New York Times, March 16, 2010–
Tim Holland, Backgammon Master, Tim Holland, who was widely considered the world’s greatest backgammon player during that ancient board game’s modern heyday, in the 1960s and ’70s, died on March 10 at his home in West Palm Beach, Fla. He was 79. <<more>> |
In Holland's honor, a post
from Columbus Day, 2004—
Tuesday October 12, 200411:11 PM Time and Chance
Today’s winning lottery numbers
|
A quote from Holland on backgammon–
"It’s the luck factor that seduces everyone
into believing that they are good,
that they can actually win,
but that’s just wishful thinking."
For those who are, like G.H. Hardy,
suspicious of wishful thinking,
here is a quote and a picture from
Holland's ordinary birthday, March 3—
"The die is cast." — Caesar
Today's previous entry quoted a review by Edward Rothstein of Jung's The Red Book. The entry you are now reading quotes a review by Jim Holt of a notable book by Rothstein:
The Golden Book—

Cover illustration— Arithmetic and Music,
Borgia Apartments, The Vatican
Jim Holt reviewing Edward Rothstein's Emblems of Mind: The Inner Life of Music and Mathematics in The New Yorker of June 5, 1995:
Advent
"The fugues of Bach, the symphonies of Haydn, the sonatas of Mozart: these were explorations of ideal form, unprofaned by extramusical associations. Such 'absolute music,' as it came to be called, had sloughed off its motley cultural trappings. It had got in touch with its essence. Which is why, as Walter Pater famously put it, 'all art constantly aspires towards the condition of music.'
The only art that can rival music for sheer etheriality is mathematics. A century or so after the advent of absolute music, mathematics also succeeded in detaching itself from the world. The decisive event was the invention of strange, non-Euclidean geometries, which put paid to the notion that the mathematician was exclusively, or even primarily, concerned with the scientific universe. 'Pure' mathematics came to be seen by those who practiced it as a free invention of the imagination, gloriously indifferent to practical affairs– a quest for beauty as well as truth."
Related material: Hardy's Apology, Non-Euclidean Blocks, and The Story Theory of Truth.
See also Holt on music and emotion:

"Music does model… our emotional life… although
the methods by which it does so are 'puzzling.'"
Also puzzling: 2010 AMS Notices.
Solomon's Cube
continued
"There is a book… called A Fellow of Trinity, one of series dealing with what is supposed to be Cambridge college life…. There are two heroes, a primary hero called Flowers, who is almost wholly good, and a secondary hero, a much weaker vessel, called Brown. Flowers and Brown find many dangers in university life, but the worst is a gambling saloon in Chesterton run by the Misses Bellenden, two fascinating but extremely wicked young ladies. Flowers survives all these troubles, is Second Wrangler and Senior Classic, and succeeds automatically to a Fellowship (as I suppose he would have done then). Brown succumbs, ruins his parents, takes to drink, is saved from delirium tremens during a thunderstorm only by the prayers of the Junior Dean, has much difficulty in obtaining even an Ordinary Degree, and ultimately becomes a missionary. The friendship is not shattered by these unhappy events, and Flowers's thoughts stray to Brown, with affectionate pity, as he drinks port and eats walnuts for the first time in Senior Combination Room."
— G. H. Hardy, A Mathematician's Apology
"The Solomon Key is the working title of an unreleased novel in progress by American author Dan Brown. The Solomon Key will be the third book involving the character of the Harvard professor Robert Langdon, of which the first two were Angels & Demons (2000) and The Da Vinci Code (2003)." — Wikipedia
"One has O+(6) ≅ S8, the symmetric group of order 8! …."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint.
"The complete projective group of collineations and dualities of the [projective] 3-space is shown to be of order [in modern notation] 8! …. To every transformation of the 3-space there corresponds a transformation of the [projective] 5-space. In the 5-space, there are determined 8 sets of 7 points each, 'heptads' …."
— George M. Conwell, "The 3-space PG(3, 2) and Its Group," The Annals of Mathematics, Second Series, Vol. 11, No. 2 (Jan., 1910), pp. 60-76
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference (July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
(continued from Thursday's
"Through the Looking Glass")
"From the grave, Albert Einstein poured gasoline on the culture wars between science and religion this week.
A letter the physicist wrote in 1954 to the philosopher Eric Gutkind, in which he described the Bible as 'pretty childish' and scoffed at the notion that the Jews could be a 'chosen people,' sold for $404,000 at an auction in London. That was 25 times the presale estimate."
Einstein did not, at least in the place alleged, call the Bible "childish." Proof:

The image of the letter is
from the Sept./Oct. 2008
Search Magazine.
By the way, today is
the birthday of G. H. Hardy.
Here is an excerpt from his
thoughts on childish things:

"Space: what you
damn well have to see."
— James Joyce, Ulysses

“Leonard Mlodinow has had, to speak informally, a pretty random career….
A far more sober instance of randomness, however, underpins his new book, The Drunkard’s Walk. And it’s not hard to see it as a sort of Rosebud, explaining why the author finds unpredictability so compelling.”
Another sort of Rosebud–
C. P. Snow on G. H. Hardy:
“… A Mathematician’s Apology is, if read with the textual attention it deserves, a book of haunting sadness. Yes, it is witty and sharp with intellectual high spirits: yes, the crystalline clarity and candour are still there: yes, it is the testament of a creative artist. But it is also, in an understated stoical fashion, a passionate lament for creative powers that used to be and that will never come again.”
Perhaps in the afterlife Hardy, an expert on the theory of numbers, does again enjoy such powers. If so, his comments on the following would be of interest:
2 + 3 + 5 + 7 + 11 +
13 + 17 + 19 + 23 +
29 + 31 + 37 + 41 +
43 + 47 + 53 + 59 +
61 + 67 = 568
See The On-Line Encyclopedia
of Integer Sequences,
A046731, Sum of primes < 10^n, as well as
A006880, Number of primes < 10^n.
According to an amateur* mathematician named Cino Hilliard, “a very important relationship exists” between the sum of primes less than x and the prime counting function Pi(x) which is the number of primes less than x—
Whether this apparent relationship is, in fact, “very important,” or merely a straightforward consequence of other number-theoretical facts, is not obvious (to those of us not expert in number theory) from Google searches. Perhaps Hardy can clear this question up for those who will, by luck or grace, meet him in the next world.
* For some background, see a profile and user group messages here and here and here.
C. P. Snow in A Mathematician's Apology :
FOREWORD
"It was a perfectly ordinary night at Christ's high table, except that Hardy was dining as a guest. He had just returned to Cambridge as Sadleirian professor, and I had heard something of him from young Cambridge mathematicians. They were delighted to have him back: he was a real mathematician, they said, not like those Diracs and Bohrs the physicists were always talking about: he was the purest of the pure. He was also unorthodox, eccentric, radical, ready to talk about anything. This was 1931, and the phrase was not yet in English use, but in later days they would have said that in some indefinable way he had star quality."
Perhaps now also at Christ's high table– Scarlett O'Hara's Younger Sister , Evelyn Keyes, who died on July 4, 2008:
"… the memory of Evelyn Keyes looking at herself on the screen, exclaiming: 'There's star quality! Look at those tits!'"

Evelyn Keyes in 99 River Street
"Religions are hardy."
— TIME magazine,
issue dated July 14
"I confess I do not believe in time."
— Vladimir Nabokov
"I can hardly do better than
go back to the Greeks."
— G. H. Hardy
Figure 1:
The Greeks

Figure 2:
The Irrational

(A Mathematician's Apology, Cambridge at the University Press, first edition, 1940)
Brian Harley on chess problems–
"It is quite true that variation play is, in ninety-nine cases out of a hundred, the soul of a problem, or (to put it more materially) the main course of the solver's banquet, but the Key is the cocktail that begins the proceedings, and if it fails in piquancy the following dinner is not so satisfactory as it should be."
(Mate in Two Moves, London, Bell & Sons, first edition, 1931)
Faustus is gone:
regard his hellish fall
— Marlowe
On Faust— today's noon entry and yesterday's "Nightmare Lessons."
On "Meta Physicists"– an entry of June 6, on Cullinane College, has a section titled "Meta Physics."
On Copenhagen— an entry of Bloomsday Eve, 2004 on a native of that city.
"Words, words, words."
— Hamlet
Another metaphysics:
"317 is a prime,
not because we think so,
or because our minds
are shaped in one way
rather than another,
but because it is so,
because mathematical
reality is built that way."
— G. H. Hardy,
A Mathematician's Apology
“I don’t think the ‘diamond theorem’ is anything serious, so I started with blitzing that.”
— Charles Matthews at Wikipedia, Oct. 2, 2006
“The ‘seriousness’ of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is ‘significant’ if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas.”
— G. H. Hardy, A Mathematician’s Apology

“When he was taken to church
he amused himself by factorizing
the numbers of the hymns.”
— C. P. Snow, foreword to
A Mathematician’s Apology,
by G. H. Hardy
An application of
lottery hermeneutics:
420 –> 4/20 –>
Hall of Shame,
Easter Sunday,
April 20, 2003;
145 –> 5*29 –> 5/29 –>
The Rev. Wright may also
be interested in the following
Related material:
“Shem was a sham….”
(FW I.7, 170 and Log24 Oct. 13),
and The Hebrew Word Shem:
This word occurs, notably, in Psalm (or “hymn”) 145.
See http://scripturetext.com/psalms/145-1.htm:
thy name
shem (shame)
an appellation, as a mark or memorial of individuality; by implication honor, authority, character — + base, (in-)fame(-ous), named(-d), renown, report.

Related material:
The Crimson Passion
Cleavage Term
Snow is mainly remembered as the author of The Two Cultures and the Scientific Revolution (1959).
According to Orrin Judd, we can now see “how profoundly wrong Snow was in everything except for his initial metaphor, of a divide between science and the rest of the culture.”
For more on that metaphor, see the previous entry, “The Line.”
I prefer a lesser-known work of Snow– his long biographical foreword to G. H. Hardy’s A Mathematician’s Apology. The foreword, like the book itself, is an example of what Robert M. Pirsig calls “Quality.” It begins with these words:
“It was a perfectly ordinary night at Christ’s high table, except that Hardy was dining as a guest.”
Wallace Stevens,
Serious
"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."
— Charles Matthews at Wikipedia, Oct. 2, 2006
"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."
— G. H. Hardy, A Mathematician's Apology
Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:
Affine group
Reflection group
Symmetry in mathematics
Incidence structure
Invariant (mathematics)
Symmetry
Finite geometry
Group action
History of geometry
This would appear to be a fairly large complex of mathematical ideas.
See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:
Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.
| Excerpts from Log 24, January 18, 2004: A Living Church "Plato has told you a truth; but Plato is dead. Shakespeare has startled you with an image; but Shakespeare will not startle you with any more. But imagine what it would be to live with such men still living. To know that Plato might break out with an original lecture to-morrow, or that at any moment Shakespeare might shatter everything with a single song. The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast. He is always expecting to see some truth that he has never seen before." — G. K. Chesterton, Orthodoxy C. P. Snow on G. H. Hardy in the foreword to A Mathematician's Apology: "… he had another favourite entertainment…." … If, as Chesterton might surmise, he… met Plato and Shakespeare in Heaven, the former might discuss with him the eternal Platonic form of the number 17*, while the latter might offer…. * Footnote of 9/18/06: For the Platonic form of 17, see Feast of the Triumph of the Cross (9/14/06) and Medal (9/15/06). |
A Living Church,
continued…
Related material:
|
ON 6-6-6 —
"Seamus Davey-Fitzpatrick stars in a scene from the R-rated movie 'The Omen.' An official of the Australian bishops conference took on the superstition surrounding the movie's release date of June 6, 2006, noting that 'I take evil far too seriously to think "The Omen" is telling me anything realistic or important.'" (CNS/20th Century Fox) |
and
The following symbol
has been associated
with the date
December 1:
Click on the symbol
for details.
That date is connected
to today’s date since
Dec. 1 is the feast—
i.e., the deathday– of
a saint of mathematics:
G. H. Hardy, author of
the classic
A Mathematician’s Apology
(online, pdf, 52 pp. ),
while today is the birthday
of three less saintly
mathematical figures:
Sir William Rowan Hamilton,
For these birthdays, here is
a more cheerful version of
the above symbol:

For the significance of
this version, see
Chinese Jar Revisited
(Log24, June 27, 2006),
a memorial to mathematician
Irving Kaplansky
(student of Mac Lane).
This version may be regarded
as a box containing the
cross of St. Andrew.
If we add a Greek cross
(equal-armed) to the box,
we obtain the “spider,”
or “double cross,” figure

of my favorite mythology:
Fritz Leiber’s Changewar.
Proof 101
From a course description:
“This module aims to introduce the student to rigorous university level mathematics….
Syllabus: The idea of and need for mathematical statements and proofs…. proof by contradiction… proof by induction…. the infinite number of primes….”
In the December Notices of the American Mathematical Society, Brian (E. B.) Davies, a professor of mathematics at King’s College London, questions the consistency of Peano Arithmetic (PA), which has the following axioms:
From BookRags.com—
Axiom 1. 0 is a number.
Axiom 2. The successor of any number is a number.
Axiom 3. If a and b are numbers and if their successors are equal, then a and b are equal.
Axiom 4. 0 is not the successor of any number.
Axiom 5. If S is a set of numbers containing 0 and if the successor of any number in S is also in S, then S contains all the numbers.
It should be noted that the word “number” as used in the Peano axioms means “non-negative integer.” The fifth axiom deserves special comment. It is the first formal statement of what we now call the “induction axiom” or “the principle of mathematical induction.”
Peano’s fifth axiom particularly troubles Davies, who writes elsewhere:
I contend that our understanding of number should be placed in an historical context, and that the number system is a human invention. Elementary arithmetic enables one to determine the number of primes less than twenty as certainly as anything we know. On the other hand Peano arithmetic is a formal system, and its internal consistency is not provable, except within set-theoretic contexts which essentially already assume it, in which case their consistency is also not provable. The proof that there exists an infinite number of primes does not depend upon counting, but upon the law of induction, which is an abstraction from our everyday experience….
… Geometry was a well developed mathematical discipline based upon explicit axioms over one and a half millennia before the law of induction was first formulated. Even today many university students who have been taught the principle of induction prefer to avoid its use, because they do not feel that it is as natural or as certain as a purely algebraic or geometric proof, if they can find one. The feelings of university students may not settle questions about what is truly fundamental, but they do give some insight into our native intuitions.— E. B. Davies in
“Counting in the real world,”
March 2003 (word format),
To appear in revised form in
Brit. J. Phil. Sci. as
“Some remarks on
the foundations
of quantum mechanics”
Exercise:
Discuss Davies’s claim that
The proof that there exists an infinite number of primes does not depend upon counting, but upon the law of induction.
Cite the following passage in your discussion.
It will be clear by now that, if we are to have any chance of making progress, I must produce examples of “real” mathematical theorems, theorems which every mathematician will admit to be first-rate.
… I can hardly do better than go back to the Greeks. I will state and prove two of the famous theorems of Greek mathematics. They are “simple” theorems, simple both in idea and in execution, but there is no doubt at all about their being theorems of the highest class. Each is as fresh and significant as when it was discovered– two thousand years have not written a wrinkle on either of them. Finally, both the statements and the proofs can be mastered in an hour by any intelligent reader, however slender his mathematical equipment.
I. The first is Euclid’s proof of the existence of an infinity of prime numbers.
The prime numbers or primes are the numbers
(A) 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, …
which cannot be resolved into smaller factors. Thus 37 and 317 are prime. The primes are the material out of which all numbers are built up by multiplication: thus
666 = 2 . 3 . 3 . 37.
Every number which is not prime itself is divisible by at least one prime (usually, of course, by several). We have to prove that there are infinitely many primes, i.e. that the series (A) never comes to an end.
Let us suppose that it does, and that
2, 3, 5, . . . , P
is the complete series (so that P is the largest prime); and let us, on this hypothesis, consider the numberQ = (2 . 3 . 5 . . . . . P) + 1.
It is plain that Q is not divisible by any of
2, 3, 5, …, P;
for it leaves the remainder 1 when divided by any one of these numbers. But, if not itself prime, it is divisible by some prime, and therefore there is a prime (which may be Q itself) greater than any of them. This contradicts our hypothesis, that there is no prime greater than P; and therefore this hypothesis is false.
The proof is by reductio ad absurdum, and reductio ad absurdum, which Euclid loved so much, is one of a mathematician’s finest weapons. It is a far finer gambit than any chess gambit: a chess player may offer the sacrifice of a pawn or even a piece, but a mathematician offers the game.
— G. H. Hardy,
A Mathematician’s Apology,
quoted in the online guide for
Clear and Simple as the Truth:
Writing Classic Prose, by
Francis-Noël Thomas
and Mark Turner,
Princeton University Press
In discussing Davies’s claim that the above proof is by induction, you may want to refer to Davies’s statement that
Geometry was a well developed mathematical discipline based upon explicit axioms over one and a half millennia before the law of induction was first formulated
and to Hardy’s statement that the above proof is due to Euclid.
Permanence
“What we do may be small, but it has a certain character of permanence.”
— G. H. Hardy, A Mathematician’s Apology
For further details, see
Geometry of the 4×4 Square.
“There is no permanent place in the world for ugly mathematics.”
— Hardy, op. cit.
For further details, see
Four-colour proof claim.
In memory of C. P. Snow,
whose birthday is today

“It was a perfectly ordinary night
at Christ’s high table, except that
Hardy was dining as a guest.”
— C. P. Snow**
“666=2.3.3.37, and there is
no other decomposition.”
— G. H. Hardy***
** Foreword to
A Mathematician’s Apology
Oct. 15, 2004, 7:11:37 PM
Introduction to Aesthetics
“Chess problems are the
hymn-tunes of mathematics.”
— G. H. Hardy,
A Mathematician’s Apology
 |
 |
“We do not want many ‘variations’ in the proof of a mathematical theorem: ‘enumeration of cases,’ indeed, is one of the duller forms of mathematical argument. A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way.
A chess problem also has unexpectedness, and a certain economy; it is essential that the moves should be surprising, and that every piece on the board should play its part. But the aesthetic effect is cumulative. It is essential also (unless the problem is too simple to be really amusing) that the key-move should be followed by a good many variations, each requiring its own individual answer. ‘If P-B5 then Kt-R6; if …. then …. ; if …. then ….’ — the effect would be spoilt if there were not a good many different replies. All this is quite genuine mathematics, and has its merits; but it just that ‘proof by enumeration of cases’ (and of cases which do not, at bottom, differ at all profoundly*) which a real mathematician tends to despise.
* I believe that is now regarded as a merit in a problem that there should be many variations of the same type.”
(Cambridge at the University Press. First edition, 1940.)

Brian Harley in
Mate in Two Moves:
“It is quite true that variation play is, in ninety-nine cases out of a hundred, the soul of a problem, or (to put it more materially) the main course of the solver’s banquet, but the Key is the cocktail that begins the proceedings, and if it fails in piquancy the following dinner is not so satisfactory as it should be.”
(London, Bell & Sons. First edition, 1931.)
Readings for
St. Patrick's Day
Books:
Finnegans Wake (1939)
Gravity's Rainbow (1978)
Masks of the Illuminati (1981)
Quotations:
"Nature does not know extinction;
all it knows is transformation.
Everything science has taught me,
and continues to teach me,
strengthens my belief in
the continuity of our
spiritual existence
after death."
— Wernher von Braun
"I faced myself that day
with the nonplused apprehension
of someone who has
come across a vampire
and has no crucifix in hand."
— Joan Didion, "On Self-Respect,"
in Slouching Towards Bethlehem
|
"For every kind of vampire, — Thomas Pynchon, Inscribed
In Latin, NORMA Multa renascentur quae iam cecidere, cadentque Many terms will be born again All, all must perish — but, surviving last, "Norma was the latin word for what we now call a carpenter's square. It was used to construct lines which were at right angles to another line, so the created line was said to be 'normal.' The norma was also used as a standard to compare if objects, like a wall, might be erect (perpendicular to the ground) and so those that met the standard were called 'normal' and this use extended to the 'typical' element of any type of set. Eventually normal came to mean anything that 'met the |
"317 is a prime,
not because we think so,
or because our minds are shaped
in one way rather than another,
but because it is so,
because mathematical reality
is built that way."
— G. H. Hardy,
A Mathematician's Apology
A Living Church
"Plato has told you a truth; but Plato is dead. Shakespeare has startled you with an image; but Shakespeare will not startle you with any more. But imagine what it would be to live with such men still living. To know that Plato might break out with an original lecture to-morrow, or that at any moment Shakespeare might shatter everything with a single song. The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast. He is always expecting to see some truth that he has never seen before."
— G. K. Chesterton, Orthodoxy
C. P. Snow on G. H. Hardy in the foreword to A Mathematician's Apology:
"… he had another favourite entertainment. 'Mark that man we met last night,' he said, and someone had to be marked out of 100 in each of the categories Hardy had long since invented and defined. STARK, BLEAK ('a stark man is not necessarily bleak: but all bleak men without exception want to be considered stark')…."
S. H. Cullinane on religion and Hollywood:
"If the incomparable Max Bialystock were to remake 'Up Close and Personal,' he might retitle it 'Distant and Impersonal.' A Google search on this phrase suggests
a plot outline for Mel Brooks & Co."
In memory of
producer Ray Stark,
an excerpt from that plot outline:
The Oxford University Press summary of
God:
Myths of the Male Divine,
by David Leeming and Jake Page
"They [Leeming and Page] describe the rise of a male sky God as 'the equal to, the true mate, of Goddess, who was still associated with Earth.' In the Iron Age, the sky God became more aggressive, separating from the Goddess and taking his place as the King God, as Zeus, Odin, and Horus. Ultimately he emerged as the creator, a more distant and impersonal force. Here Leeming and Page also illuminate an important trend–a sense that the divine is beyond gender, that it permeates all things (as seen in the Chinese Tao and En Sof of the Kabbalah). They see a movement in the biography of God toward a reunion with the Goddess."
As for the Goddess, see
(December 17, 2002).
Stark, a saint among Hollywood producers, died yesterday, January 17. If, as Chesterton might surmise, he then met Plato and Shakespeare in Heaven, the former might discuss with him the eternal Platonic form of the number 17, while the latter might offer the following links on Stark's new heavenly laptop:
This concludes the tribute to Stark. For a tribute to Bleak, click here.
Bibles
Today is the feast day of St. Hermann Hesse. A quotation from a work by Hesse that is to some a sort of Bible:
"You treat world history as a mathematician does mathematics, in which nothing but laws and formulae exist, no reality, no good and evil, no time, no yesterday, no tomorrow, nothing but an eternal, shallow, mathematical present."
— Father Jacobus, Benedictine priest, in The Glass Bead Game, ch. 4 (1943, translated 1960), by Hermann Hesse
A Benedictine Archbishop's Apology:
"Archbishop Weakland described his feelings 'at this moment' as 'remorse, contrition, shame and emptiness,' also noting that 'much self-pity and pride remain.' He contended he 'must leave that pride behind.' "
C.P. Snow in his introduction to A Mathematician's Apology (also a Bible, or at least a book of a Bible, to some) quotes G. H. Hardy on hearing the chimes of Vespers:
"It's rather unfortunate that some of the happiest hours of my life should have been spent within sound of a Roman Catholic church.''
A Bible for Benedictines:
The Encyclopedic Dictionary of Mathematics,
by the Mathematical Society of Japan,
is suitable reading for those Benedictines in Purgatory who have too lightly used words like "no reality" and "shallow" to describe mathematics.
For other remedial reading in the afterlife, see Midsummer Eve's Dream and Quine in Purgatory.
Before and After
From Understanding the (Net) Wake:
| 24 A.
Joyce shows an understanding of the problems that an intertextual book like the Wake poses for the notion of authorship. |
G. H. Hardy in A Mathematician’s Apology:
“We do not want many ‘variations’ in the proof of a mathematical theorem: ‘enumeration of cases,’ indeed, is one of the duller forms of mathematical argument. A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way.
A chess problem also has unexpectedness, and a certain economy; it is essential that the moves should be surprising, and that every piece on the board should play its part. But the aesthetic effect is cumulative. It is essential also (unless the problem is too simple to be really amusing) that the key-move should be followed by a good many variations, each requiring its own individual answer. ‘If P-B5 then Kt-R6; if …. then …. ; if …. then ….’ — the effect would be spoilt if there were not a good many different replies. All this is quite genuine mathematics, and has its merits; but it just that ‘proof by enumeration of cases’ (and of cases which do not, at bottom, differ at all profoundly*) which a real mathematician tends to despise.
* I believe that is now regarded as a merit in a problem that there should be many variations of the same type.”
(Cambridge at the University Press. First edition, 1940.)

Brian Harley in Mate in Two Moves:
“It is quite true that variation play is, in ninety-nine cases out of a hundred, the soul of a problem, or (to put it more materially) the main course of the solver’s banquet, but the Key is the cocktail that begins the proceedings, and if it fails in piquancy the following dinner is not so satisfactory as it should be.”
(London, Bell & Sons. First edition, 1931.)
Seek and Ye Shall Find:
On the Mystical Properties
of the Number 162
On this date in history:
May 22, 1942: Unabomber Theodore John Kaczynski is born in the Chicago suburb of Evergreen Park, Ill., to Wanda Kaczynski and her husband Theodore R. Kaczynski, a sausage maker. His mother brings him up reading Scientific American.
From the June 2003 Scientific American:
“Seek and ye shall find.” – Michael Shermer
|
From my note Mark of April 25, 2003: “Tell me of runes to grave — A. E. Housman, quoted by G. H. Hardy in A Mathematician’s Apology “Here, as examples, are one rune and one bastion…. (illustrations: the Dagaz rune and the Nike bastion of the Acropolis)…. Neither the rune nor the bastion discussed has any apparent connection with the number 162… But seek and ye shall find.” |
Here is a connection to runes:
Mayer, R.M., “Runenstudien,” Beiträge zur Geschichte der deutschen Sprache und Literatur 21 (1896): pp. 162 – 184.
Here is a connection to Athenian bastions from a UN article on Communist educational theorist Dimitri Glinos:
“Educational problems cannot be scientifically solved by theory and reason alone….” (D. Glinos (1882-1943), Dead but not Buried, Athens, Athina, 1925, p. 162)
“Schools are…. not the first but the last bastion to be taken by… reform….”
“…the University of Athens, a bastion of conservatism and counter-reform….”
I offer the above with tongue in cheek as a demonstration that mystical numerology may have a certain heuristic value overlooked by fanatics of the religion of Scientism such as Shermer.
For a more serious discussion of runes at the Acropolis, see the photo on page 16 of the May 15, 2003, New York Review of Books, illustrating the article “Athens in Wartime,” by Brady Kiesling.
Mark
Today is the feast of Saint Mark. It seems an appropriate day to thank Dr. Gerald McDaniel for his online cultural calendar, which is invaluable for suggesting blog topics.
Yesterday's entry "Cross-Referenced" referred to a bizarre meditation of mine titled "The Matthias Defense," which combines some thoughts of Nabokov on lunacy with some of my own thoughts on the Judeo-Christian tradition (i.e., also on lunacy). In this connection, the following is of interest:
From a site titled Meaning of the Twentieth Century —
"Freeman Dyson has expressed some thoughts on craziness. In a Scientific American article called 'Innovation in Physics,' he began by quoting Niels Bohr. Bohr had been in attendance at a lecture in which Wolfgang Pauli proposed a new theory of elementary particles. Pauli came under heavy criticism, which Bohr summed up for him: 'We are all agreed that your theory is crazy. The question which divides us is whether it is crazy enough to have a chance of being correct. My own feeling is that is not crazy enough.' To that Freeman added: 'When a great innovation appears, it will almost certainly be in a muddled, incomplete and confusing form. To the discoverer, himself, it will be only half understood; to everyone else, it will be a mystery. For any speculation which does not at first glance look crazy, there is no hope!' "
— Kenneth Brower, The Starship and the Canoe, 1979, pp. 146, 147
It is my hope that the speculation, implied in The Matthias Defense, that the number 162 has astonishing mystical properties (as a page number, article number, etc.) is sufficiently crazy to satisfy Pauli and his friend Jung as well as the more conventional thinkers Bohr and Dyson. It is no less crazy than Christianity, and has a certain mad simplicity that perhaps improves on some of that religion's lunatic doctrines.
Some fruits of the "162 theory" —
Searching on Google for muses 162, we find the following Orphic Hymn to Apollo and a footnote of interest:
27 Tis thine all Nature's music to inspire,
28 With various-sounding, harmonising lyre;
29 Now the last string thou tun'ft to sweet accord,
30 Divinely warbling now the highest chord….
"Page 162 Verse 29…. Now the last string…. Gesner well observes, in his notes to this Hymn, that the comparison and conjunction of the musical and astronomical elements are most ancient; being derived from Orpheus and Pythagoras, to Plato. Now, according to the Orphic and Pythagoric doctrine, the lyre of Apollo is an image of the celestial harmony…."
For the "highest chord" in a metaphorical sense, see selection 162 of the 1919 edition of The Oxford Book of English Verse (whose editor apparently had a strong religious belief in the Muses (led by Apollo)). This selection contains the phrase "an ever-fixèd mark" — appropriately enough for this saint's day. The word "mark," in turn, suggests a Google search for the phrase "runes to grave" Hardy, after a poem quoted in G. H. Hardy's A Mathematician's Apology.
Such a search yields a website that quotes Housman as the source of the "runes" phrase, and a further search yields what is apparently the entire poem:
Smooth Between Sea and Land
by A. E. Housman
Smooth between sea and land
Is laid the yellow sand,
And here through summer days
The seed of Adam plays.Here the child comes to found
His unremaining mound,
And the grown lad to score
Two names upon the shore.Here, on the level sand,
Between the sea and land,
What shall I build or write
Against the fall of night?Tell me of runes to grave
That hold the bursting wave,
Or bastions to design
For longer date than mine.Shall it be Troy or Rome
I fence against the foam
Or my own name, to stay
When I depart for aye?Nothing: too near at hand
Planing the figured sand,
Effacing clean and fast
Cities not built to last
And charms devised in vain,
Pours the confounding main.(Said to be from More Poems (Knopf, 1936), p. 64)
Housman asks the reader to tell him of runes to grave or bastions to design. Here, as examples, are one rune and one bastion.
|
Represents |
 Dagaz: (Pronounced thaw-gauze, but with the "th" voiced as in "the," not unvoiced as in "thick") (Day or dawn.)
Dagaz: (Pronounced thaw-gauze, but with the "th" voiced as in "the," not unvoiced as in "thick") (Day or dawn.)
From Rune Meanings:
Dagaz means "breakthrough, awakening, awareness. Daylight clarity as opposed to nighttime uncertainty. A time to plan or embark upon an enterprise. The power of change directed by your own will, transformation. Hope/happiness, the ideal. Security and certainty. Growth and release. Balance point, the place where opposites meet."
Also known as "the rune of transformation."
For the Dagaz rune in another context, see Geometry of the I Ching. The geometry discussed there does, in a sense, "hold the bursting wave," through its connection with Walsh functions, hence with harmonic analysis.
 Temple of Athena Nike on the Nike Bastion, the Acropolis, Athens. Here is a relevant passage from Paul Valéry's Eupalinos ou L'Architecte about another temple of four columns:
Temple of Athena Nike on the Nike Bastion, the Acropolis, Athens. Here is a relevant passage from Paul Valéry's Eupalinos ou L'Architecte about another temple of four columns:
Et puis… Écoute, Phèdre (me disait-il encore), ce petit temple que j'ai bâti pour Hermès, à quelques pas d'ici, si tu savais ce qu'il est pour moi ! — Où le passant ne voit qu'une élégante chapelle, — c'est peu de chose: quatre colonnes, un style très simple, — j'ai mis le souvenir d'un clair jour de ma vie. Ô douce métamorphose ! Ce temple délicat, nul ne le sait, est l'image mathématique d'une fille de Corinthe que j'ai heureusement aimée. Il en reproduit fidèlement les proportions particulières. Il vit pour moi !
Four columns, in a sense more suited to Hardy's interests, are also a recurrent theme in The Diamond 16 Puzzle and Diamond Theory.
Apart from the word "mark" in The Oxford Book of English Verse, as noted above, neither the rune nor the bastion discussed has any apparent connection with the number 162… but seek and ye shall find.
A Red Mass
For G. H. Hardy, who, although he kept a portrait of Lenin in his rooms, knew more of truth than most Christians ever know.
"317 is a prime, not because we think so, or because our minds are shaped in one way rather than another, but because it is so, because mathematical reality is built that way."
— G. H. Hardy, A Mathematician's Apology, 1940
Art Wars:
Mathematics and the
Emperor's New Art
From Maureen Dowd's New York Times column of June 9, 2002:
"The shape of the government is not as important as the policy of the government. If he makes the policy aggressive and pre-emptive, the president can conduct the war on terror from the National Gallery of Art."
|
NY Times, April 5, 2003: |
|
Meanwhile, at the Washington Post, another example of great determination and strength of character:
Donald Coxeter Dies: Leader in Geometry
By Martin Weil
Washington Post Staff Writer
Saturday, April 5, 2003
"Donald Coxeter, 96, a mathematician who was one of the 20th century's foremost specialists in geometry and a man of great determination and strength of character as well, died March 31 at his home in Toronto."
From another Coxeter obituary:
In the Second World War, Coxeter was asked by the American government to work in Washington as a code-breaker. He accepted, but then backed out, partly because of his pacifist views and partly for aesthetic reasons: "The work didn't really appeal to me," he explained; "it was a different sort of mathematics."
For a differing account of how geometry is related to code-breaking, see the "Singer 7-cycle" link in yesterday's entry, "The Eight," of 3:33 PM. This leads to a site titled
An Introduction to the
Applications of Geometry in Cryptography.
"Now I have precisely the right instrument, at precisely the right moment of history, in exactly the right place."
— "Patton,"
the film

Added Sunday, April 6, 2003, 3:17 PM:
The New York Times Magazine of April 6
continues this Art Wars theme.

(Cover typography revised)
The military nature of our Art Wars theme appears in the Times's choice of words for its cover headline: "The Greatest Generation." (This headline appears in the paper, but not the Internet, version.)
Some remarks in today's Times Magazine article seem especially relevant to my journal entry for Michelangelo's birthday, March 6.
"…Conceptualism — suddenly art could be nothing more than an idea….
LeWitt moved between his syntax of geometric sculptures and mental propositions for images: concepts he wrote on paper that could be realized by him or someone else or not at all. Physical things are perishable. Ideas need not be."
— Michael Kimmelman, chief art critic of the New York Times, April 6, 2003
Compare this with a mathematician's aesthetics:
"A mathematician, like a painter or a poet, is a maker of patterns. If his patterns are more permanent than theirs, it is because they are made with ideas."
— G. H. Hardy, A Mathematician's Apology (1940), reprinted 1969, Cambridge U. Press, p. 84
It seems clear from these two quotations that the real conceptual art is mathematics and that Kimmelman is peddling the emperor's new clothes.
versus
One year ago today, Lorenzo Music, the voice of Carlton the doorman on Rhoda, died. His eulogy from Valerie Harper:
"Valerie's heart is breaking, but Rhoda is certain that Carlton the doorman is giving St. Peter at the gate a run for his money."
Today's birthday: Logician John Venn.
Appearing for the story theory…
Flannery O'Connor:
"In the long run, a people is known, not by its statements or statistics, but by the stories it tells. Fiction is the most impure and the most modest and the most human of the arts."
Appearing for the diamond theory…
Mary McCarthy and G. H. Hardy:
From the Hollywood Investigator:
On October 18, 1979, Mary McCarthy said on PBS's Dick Cavett Show: "Every word she writes is a lie, including 'and' and 'the.'"
Don't forget "a," as in "a people is known" —
"Greek mathematics is permanent, more permanent even than Greek literature. Archimedes will be remembered when Aeschylus is forgotten, because languages die and mathematical ideas do not."
— G. H. Hardy in A Mathematician's Apology
And a closing rebuttal from the story theory…
Martin Heidegger and Dean Martin:
Words of wisdom from Martin Heidegger, Catholic Nazi:
"The nature of art is poetry. The nature of poetry, in turn, is the founding of truth…. In the work, truth is thrown toward… an historical group of men."
— Poetry, Language, Thought, page 75, translated by Albert Hofstadter, Harper & Row paperback, 1975
And from Dean Martin, avatar of anti-art :
– Artist: Dean Martin as sung on "Dean Martin's Greatest Hits"
– Capitol 4XL-9389
– peak Billboard position # 2 in 1953
– from the movie "the Caddy" starring Dean, Jerry Lewis, and Donna Reed
– Words and Music by Harry Warren and Jack Brooks(In Napoli where love is King, when boy meets girl, here's what they say)
When the moon hits your eye like a big-a pizza pie,
That's amore!
When the world seems to shine like you've had too much wine,
That's amore!
Powered by WordPress