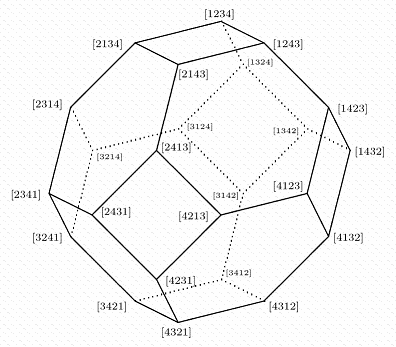
See also Clifford in this journal, in particular
The Matrix for Quantum Mystics
(Log24, St. Andrew's Day, 2017).
Sunday, January 13, 2019
The Clifford Narrative
Wednesday, December 20, 2017
A Snow Ball for Clifford Irving (1930-2017)
William Grimes in The New York Times this evening —
"Clifford Irving, who perpetrated one of the biggest literary hoaxes
of the 20th century in the early 1970s when he concocted a
supposedly authorized autobiography of the billionaire Howard Hughes
based on meetings and interviews that never took place, died on Tuesday
at a hospice facility near his home in Sarasota, Fla. He was 87."
A figure reproduced here on Tuesday —
A related figure —

See too the 1973 Orson Welles film "F for Fake."
Some background on the second figure above —
posts tagged April 8-11, 2016.
Some background on the first figure above —
today's previous post, January 2018 AMS Notices.
Tuesday, September 17, 2024
Six to Nine, My Dear Watson … Continues.
Continued from August 9, 2024.
Today 's previous post was on Ch. 6 of Selig's Geometric Fundamentals.
Ch. 9 is on Clifford Algebra.
Wednesday, July 3, 2024
Vedic Carnival: “Hey Rubik!” *
The Moolakaprithi Cube (as opposed to Rubik's Moola Cube ) —

"The key to these connections lies in a 3 x 3 x 3 cube, which
in Vedic Physics, forms the Moolaprakriti, a key component of
the Substratum, the invisible black hole form of matter."
— viXra.org, "Clifford Clock and the Moolakaprithi Cube"
* See Wikipedia.
Wednesday, December 6, 2023
Das Wiener Meisterstück
|
Introduction In the present article, the research work of many years is summarized in an interim report concerning the connection between logic, space, time and matter. The author always had in mind two things, namely 1. The discovery/construction of an interface between matter and mind, and 2. some entry points for the topos view that concern graphs, grade rotations and contravariant involutions in geometric Boolean lattices. In this part of the MiTopos theme I follow the historic approach to mathematical physics and remain with the Clifford algebra of the Minkowski space. It turns out that this interface is a basic morphogenetic structure inherent in both matter and thought. It resides in both oriented spaces and logic, and most surprisingly is closely linked to the symmetries of elementary particle physics.
— "MiTopos: Space Logic I," |
Sunday, August 1, 2021
The Savage Sixteens
"Savage ('wild,' 'undomesticated') modes of thought are primary
in human mentality. They are what we all have in common."
— "The Cerebral Savage: On the Work of Claude Lévi-Strauss,"
by Clifford Geertz (Encounter, vol. 28 no. 4, April 1967, pp. 25-32)
For more Geertz and some related art, see The Kaleidoscope Puzzle,
which lets you picture twin sixteens .
"Can you imagine the mathematical possibilities?"
— Line from "Annie Hall" (1977)
Wednesday, July 17, 2019
The Artsy Quantum Realm
|
arXiv.org > quant-ph > arXiv:1905.06914 Quantum Physics Placing Kirkman's Schoolgirls and Quantum Spin Pairs on the Fano Plane: A Rainbow of Four Primary Colors, A Harmony of Fifteen Tones J. P. Marceaux, A. R. P. Rau (Submitted on 14 May 2019) A recreational problem from nearly two centuries ago has featured prominently in recent times in the mathematics of designs, codes, and signal processing. The number 15 that is central to the problem coincidentally features in areas of physics, especially in today's field of quantum information, as the number of basic operators of two quantum spins ("qubits"). This affords a 1:1 correspondence that we exploit to use the well-known Pauli spin or Lie-Clifford algebra of those fifteen operators to provide specific constructions as posed in the recreational problem. An algorithm is set up that, working with four basic objects, generates alternative solutions or designs. The choice of four base colors or four basic chords can thus lead to color diagrams or acoustic patterns that correspond to realizations of each design. The Fano Plane of finite projective geometry involving seven points and lines and the tetrahedral three-dimensional simplex of 15 points are key objects that feature in this study. Comments:16 pages, 10 figures Subjects:Quantum Physics (quant-ph) Cite as:arXiv:1905.06914 [quant-ph] (or arXiv:1905.06914v1 [quant-ph] for this version) Submission history
From: A. R. P. Rau [view email] |
See also other posts tagged Tetrahedron vs. Square.
Tuesday, July 16, 2019
Schoolgirl Space for Quantum Mystics
Monday, March 11, 2019
Overarching Metanarratives
See also "Overarching + Tesseract" in this journal. From the results
of that search, some context for the "inscape" of the previous post —
Sunday, January 13, 2019
Into the Upside Down
Saturday, December 22, 2018
Cremona-Richmond
The following are some notes on the history of Clifford algebras
and finite geometry suggested by the "Clifford Modules" link in a
Log24 post of March 12, 2005 —
A more recent appearance of the configuration —
Saturday, December 23, 2017
The Right Stuff
A figure related to the general connecting theorem of Koen Thas —
See also posts tagged Dirac and Geometry in this journal.
Those who prefer narrative to mathematics may, if they so fancy, call
the above Thas connecting theorem a "quantum tesseract theorem ."
Sunday, December 10, 2017
Geometry
See also Symplectic in this journal.
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”

The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2). See also
related remarks on the notion of linear (or line ) complex
in the finite projective space PG(3,2) —
Algebra
Thursday, November 30, 2017
The Matrix for Quantum Mystics
Scholia on the title — See Quantum + Mystic in this journal.


"In Vol. I of Structural Anthropology , p. 209, I have shown that
this analysis alone can account for the double aspect of time
representation in all mythical systems: the narrative is both
'in time' (it consists of a succession of events) and 'beyond'
(its value is permanent)." — Claude Lévi-Strauss, 1976
I prefer the earlier, better-known, remarks on time by T. S. Eliot
in Four Quartets , and the following four quartets (from
The Matrix Meets the Grid) —
From a Log24 post of June 26-27, 2017:
A work of Eddington cited in 1974 by von Franz —

See also Dirac and Geometry and Kummer in this journal.
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
Friday, June 30, 2017
Hurriedly Put Together
The previous post quoted one theologian on a book
by another theologian, saying its tone "is patronizing
and its arguments are hurriedly put together."
For a more leisurely sort of argument, see a 1995* remark
by a mathematician, Ronald Shaw, quoted here on the morning
of Tuesday, June 27, in an update at the end of the previous day's
post "Upgrading to Six" —
". . . recall the notions of Eddington (1936) . . . ."
* In "Finite Geometry, Dirac Groups and the
Table of Real Clifford Algebras," pages 59-99 of
R. Ablamowicz and P. Lounesto (eds.),
Clifford Algebras and Spinor Structures ,
Kluwer Academic Publishers, 1995.
Monday, June 26, 2017
Upgrading to Six
This post was suggested by the previous post — Four Dots —
and by the phrase "smallest perfect" in this journal.
Related material (click to enlarge) —
Detail —

From the work of Eddington cited in 1974 by von Franz —

See also Dirac and Geometry and Kummer in this journal.
Updates from the morning of June 27 —
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
Tuesday, September 20, 2016
Savage Logic
From "The Cerebral Savage," by Clifford Geertz —
(Encounter, Vol. 28 No. 4 (April 1967), pp. 25-32.)

Sunday, July 24, 2016
Point Omega …
In this post, "Omega" denotes a generic 4-element set.
For instance … Cullinane's
or Schmeikal's
 .
.
The mathematics appropriate for describing
group actions on such a set is not Schmeikal's
Clifford algebra, but rather Galois's finite fields.
Saturday, July 23, 2016
But Seriously …
Those who want a serious approach to the mathematics
of Clifford algebras — via finite geometry, the natural setting
of the four-group of the previous post — should consult
"Finite Geometry, Dirac Groups and the Table of
Real Clifford Algebras," by Ron Shaw (1995).
Monday, November 23, 2015
Dirac and Line Geometry
Some background for my post of Nov. 20,
"Anticommuting Dirac Matrices as Skew Lines" —
His earlier paper that Bruins refers to, "Line Geometry
and Quantum Mechanics," is available in a free PDF.
For a biography of Bruins translated by Google, click here.
For some additional historical background going back to
Eddington, see Gary W. Gibbons, "The Kummer
Configuration and the Geometry of Majorana Spinors,"
pages 39-52 in Oziewicz et al., eds., Spinors, Twistors,
Clifford Algebras, and Quantum Deformations:
Proceedings of the Second Max Born Symposium held
near Wrocław, Poland, September 1992 . (Springer, 2012,
originally published by Kluwer in 1993.)
For more-recent remarks on quantum geometry, see a
paper by Saniga cited in today's update to my Nov. 20 post.
Friday, November 20, 2015
Anticommuting Dirac Matrices as Skew Lines
(Continued from November 13)
The work of Ron Shaw in this area, ca. 1994-1995, does not
display explicitly the correspondence between anticommutativity
in the set of Dirac matrices and skewness in a line complex of
PG(3,2), the projective 3-space over the 2-element Galois field.
Here is an explicit picture —

References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Shaw, Ron, "Finite Geometry, Dirac Groups, and the Table of
Real Clifford Algebras," undated article at ResearchGate.net
Update of November 23:
See my post of Nov. 23 on publications by E. M. Bruins
in 1949 and 1959 on Dirac matrices and line geometry,
and on another author who gives some historical background
going back to Eddington.
Some more-recent related material from the Slovak school of
finite geometry and quantum theory —
The matrices underlying the Saniga paper are those of Pauli, not
those of Dirac, but these two sorts of matrices are closely related.
Thursday, November 19, 2015
Highlights of the Dirac-Mathieu Connection
For the connection of the title, see the post of Friday, November 13th, 2015.
For the essentials of this connection, see the following two documents —
Sunday, November 15, 2015
The Diamond and the Cube
Anyone who clicked on the Dirac search at the end of
the previous post, "Dirac's Diamond," may wonder why the
"Solomon's Cube" post of 11 AM Sunday, March 1, 2009,
appeared in the Dirac search results, since there is no
apparent mention of Dirac in that Sunday post.
<!– See also "a linear transformation of V6… which preserves
the Klein quadric; in this way we arrive at the isomorphism of
Sym(8) withthe full orthogonal group O+(6; 2)." in "The
Classification of Flats in PG(9,2) which are External to the
Grassmannian G1,4,2 Authors: Shaw, Ron;
 Maks, Johannes; Gordon, Neil; Source: Designs,
Codes and Cryptography, Volume 34, Numbers 2-3, February
2005 , pp. 203-227; Publisher: Springer.  For more details,
see "Finite Geometry, Dirac Groups and the Table of Real
Clifford Algebras," by R. Shaw (U. of Hull), pp. 59-99 in
Clifford Algebras and Spinor Structures, by By Albert
Crumeyrolle, Rafał Abłamowicz, Pertti Lounesto,
published by Springer, 1995. –>
Friday, November 13, 2015
A Connection between the 16 Dirac Matrices and the Large Mathieu Group


Note that the six anticommuting sets of Dirac matrices listed by Arfken
correspond exactly to the six spreads in the above complex of 15 projective
lines of PG(3,2) fixed under a symplectic polarity (the diamond theorem
correlation ). As I noted in 1986, this correlation underlies the Miracle
Octad Generator of R. T. Curtis, hence also the large Mathieu group.
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Related material:
The 6-set in my 1986 note above also appears in a 1996 paper on
the sixteen Dirac matrices by David M. Goodmanson —

Background reading:
Ron Shaw on finite geometry, Clifford algebras, and Dirac groups
(undated compilation of publications from roughly 1994-1995)—
Thursday, November 5, 2015
Lyrics
“And I slept in last night’s clothes and tomorrow’s dreams
But they’re not quite what they seem.”
— Lyrics to "Uma Thurman," by Fall Out Boy
(Sung at CMA Awards last night)
"Does an empty vessel stop making most noise
once filled only with hopes and dreams?"
— Comment at Not Even Wrong this morning
Saturday, August 29, 2015
Studio Time
(The title is a phrase from Oslo artist Josefine Lyche's Instagram page today.)
Note that 6 PM ET is midnight in Oslo.
An image from St. Ursula's Day, 2010 —
Related material:
"Is it a genuine demolition of the walls which seem
to separate mind from mind …. ?"
— Clifford Geertz, conclusion of “The Cerebral Savage:
On the Work of Claude Lévi-Strauss“
Sunday, June 8, 2014
Vide

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Tuesday, April 9, 2013
Four Quartets
For the cruelest month
Click for a much larger version of the photo below.
These four Kountry Korn quartets are from the Fox Valleyaires
Men's Barbershop Chorus of Appleton, Wisconsin.
See also the fine arts here on Saturday, April 6, 2013—
The New York Times Magazine cover story
a decade ago, on Sunday, April 6, 2003:
"The artists demanded space
in tune with their aesthetic."
— "The Dia Generation,"
by Michael Kimmelman
Related material:
See Wikipedia for the difference between binary numbers
and binary coordinates from the finite Galois field GF(2).
For some background, see the relativity problem.
See also the chapter on vector spaces in Korn & Korn
(originally published by McGraw-Hill)—
 .
.
Monday, April 8, 2013
Magic for Jews
A commenter on Saturday's "Seize the Dia" has
suggested a look at the work of one Mark Collins.
Here is such a look (click to enlarge):
I find attempts to associate pure mathematics with the words
"magic" or "mystic" rather nauseating. (H. F. Baker's work
on Pascal's mystic hexagram is no exception; Baker was
stuck with Pascal's obnoxious adjective, but had no truck
with any mystic aspects of the hexagram.)
The remarks above by Clifford Pickover on Collins, Dürer, and
binary representations may interest some non-mathematicians,
who should not be encouraged to waste their time on this topic.
For the mathematics underlying the binary representation of
Dürer's square, see, for instance, my 1984 article "Binary
Coordinate Systems."
Those without the background to understand that article
may enjoy, instead of Pickover's abortive attempts above at
mathematical vulgarization, his impressively awful 2009 novel
Jews in Hyperspace .
Pickover's 2002 book on magic squares was, unfortunately,
published by the formerly reputable Princeton University Press.
Related material from today's Daily Princetonian :
See also Nash + Princeton in this journal.
Friday, November 9, 2012
Bali High Chair
The phrase "deep play" in the previous post
was a borrowing from Clifford Geertz.
From another weblog's post on Geertz and
deep play—
When family is involved, the Balinese
are much more engaged.
See also the Balinese empty chair
and Amy Adams in this journal.
Tuesday, July 3, 2012
Calling
—Rhodes, I want you to get to know people like that.
I'd like to sort of take you under my wing and educate you.
—Shucks, General, I'm just a country boy.
Saturday, August 20, 2011
Castles in the Air
"… the Jews have discovered a way to access a fourth spatial dimension."
— Clifford Pickover, description of his novel Jews in Hyperspace
"If you have built castles in the air, your work need not be lost;
that is where they should be. Now put the foundations under them.”
— Henry David Thoreau
"King Solomon's Mines," 1937—
The image above is an illustration from "Romancing the Hyperspace," May 4, 2010.
Happy birthday to the late Salomon Bochner.
Tuesday, December 21, 2010
Savage Solstice
In memory of kaleidoscope enthusiast Cozy Baker, who died at 86, according to Saturday's Washington Post , on October 19th.
This journal on that date — Savage Logic and Savage Logic continued.
See this journal on All Saints' Day 2006 for some background to those posts—
“Savage logic works like a kaleidoscope whose chips can fall into a variety of patterns while remaining unchanged in quantity, form, or color. The number of patterns producible in this way may be large if the chips are numerous and varied enough, but it is not infinite. The patterns consist in the disposition of the chips vis-a-vis one another (that is, they are a function of the relationships among the chips rather than their individual properties considered separately). And their range of possible transformations is strictly determined by the construction of the kaleidoscope, the inner law which governs its operation. And so it is too with savage thought. Both anecdotal and geometric, it builds coherent structures out of ‘the odds and ends left over from psychological or historical process.’
These odds and ends, the chips of the kaleidoscope, are images drawn from myth, ritual, magic, and empirical lore. (How, precisely, they have come into being in the first place is one of the points on which Levi-Strauss is not too explicit, referring to them vaguely as the ‘residue of events… fossil remains of the history of an individual or a society.’) Such images are inevitably embodied in larger structures– in myths, ceremonies, folk taxonomies, and so on– for, as in a kaleidoscope, one always sees the chips distributed in some pattern, however ill-formed or irregular. But, as in a kaleidoscope, they are detachable from these structures and arrangeable into different ones of a similar sort. Quoting Franz Boas that ‘it would seem that mythological worlds have been built up, only to be shattered again, and that new worlds were built from the fragments,’ Levi-Strauss generalizes this permutational view of thinking to savage thought in general.”
– Clifford Geertz, “The Cerebral Savage: the Structural Anthropology of Claude Levi-Strauss,” in Encounter, Vol. 28 No. 4 (April 1967), pp. 25-32.
Related material —
See also "Levi-Strauss" in this journal and "At Play in the Field."
Thursday, October 21, 2010
St. Ursula’s Day
Mathematics and Narrative continued
A search for Ursula in this journal yields a story…
“The main character is a slave woman who discovers new patterns in the mosaics.”
Other such stories: Plato’s Meno and Changing Woman —
|
“Kaleidoscope turning…
Shifting pattern within — Roger Zelazny, Eye of Cat |
Philosophical postscript—
“That Lévi-Strauss should have been able to transmute the romantic passion of Tristes Tropiques into the hypermodern intellectualism of La Pensée Sauvage is surely a startling achievement. But there remain the questions one cannot help but ask. Is this transmutation science or alchemy? Is the ‘very simple transformation’ which produced a general theory out of a personal disappointment real or a sleight of hand? Is it a genuine demolition of the walls which seem to separate mind from mind by showing that the walls are surface structures only, or is it an elaborately disguised evasion necessitated by a failure to breach them when they were directly encountered? Is Lévi-Strauss writing, as he seems to be claiming in the confident pages of La Pensée Sauvage, a prolegomenon to all future anthropology? Or is he, like some uprooted neolithic intelligence cast away on a reservation, shuffling the debris of old traditions in a vain attempt to revivify a primitive faith whose moral beauty is still apparent but from which both relevance and credibility have long since departed?”
— Clifford Geertz, conclusion of “The Cerebral Savage: On the Work of Claude Lévi-Strauss“
Tuesday, October 19, 2010
Savage Logic…
and the New York Lottery

A search in this journal for yesterday's evening number in the New York Lottery, 359, leads to…
The Cerebral Savage:
On the Work of Claude Lévi-Strauss
by Clifford Geertz
Shown below is 359, the final page of Chapter 13 in
The Interpretation of Cultures: Selected Essays by Clifford Geertz,
New York, 1973: Basic Books, pp. 345-359 —
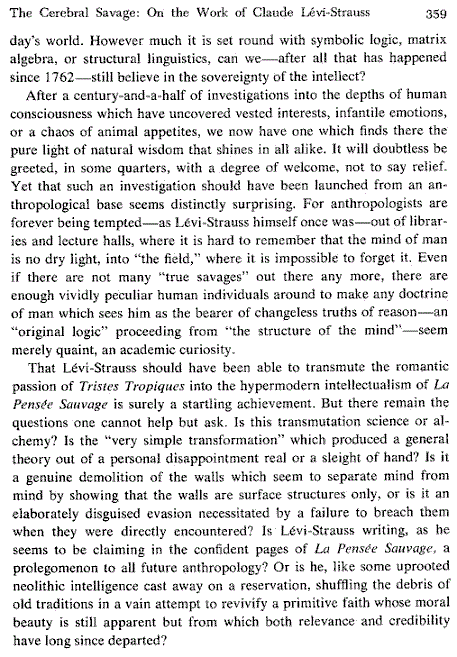
This page number 359 also appears in this journal in an excerpt from Dan Brown's novel Angels & Demons—
See this journal's entries for March 1-15, 2009, especially…
| Sunday, March 15, 2009 5:24 PM
Philosophy and Poetry: The Origin of Change A note on the figure "Two things of opposite natures seem to depend On one another, as a man depends On a woman, day on night, the imagined On the real. This is the origin of change. Winter and spring, cold copulars, embrace And forth the particulars of rapture come." -- Wallace Stevens, "Notes Toward a Supreme Fiction," Canto IV of "It Must Change" Sunday, March 15, 2009 11:00 AM Ides of March Sermon: Angels, Demons,
"Symbology" "On Monday morning, 9 March, after visiting the Mayor of Rome and the Municipal Council on the Capitoline Hill, the Holy Father spoke to the Romans who gathered in the square outside the Senatorial Palace…
'… a verse by Ovid, the great Latin poet, springs to mind. In one of his elegies he encouraged the Romans of his time with these words: "Perfer et obdura: multo graviora tulisti." "Hold out and persist: (Tristia, Liber V, Elegia XI, verse 7).'" This journal
on 9 March: Note the color-interchange Related material: |
The symmetry of the yin-yang symbol, of the diamond-theorem symbol, and of Brown's Illuminati Diamond is also apparent in yesterday's midday New York lottery number (see above).
"Savage logic works like a kaleidoscope…." — Clifford Geertz on Lévi-Strauss
Thursday, October 14, 2010
Diamond Theory and Magic Squares
"A world of made
is not a world of born— pity poor flesh
and trees, poor stars and stones, but never this
fine specimen of hypermagical
ultraomnipotence."
— e. e. cummings, 1944
For one such specimen, see The Matrix of Abraham—
a 5×5 square that is hypermagical… indeed, diabolical.
Related material on the algebra and geometry underlying some smaller structures
that have also, unfortunately, become associated with the word "magic"—
- Finite Geometry of the Square and Cube
- Clifford Pickover on a 4×4 square
-
Christopher J. Henrich on the geometry of 4×4 magic squares
(without any mention of [1] above or related work dating back to 1976)
" … listen: there's a hell
of a good universe next door; let's go"
— e. e. cummings
Happy birthday, e. e.
Monday, January 25, 2010
Key to All Mythologies
Recent Log24 entries on Hamlet suggest a look at Giorgio de Santillana's Hamlet's Mill on the Web.
There is a useful transcription by Clifford Stetner. See also an excellent review of Hamlet's Mill by Cecilia Payne-Gaposchkin.
The work of Giorgio de Santillana (like that of Stetner) suggests in turn the following recently quoted advice–
"…you should read Middlemarch every five years or so. Every time… it's a different book, and an even more powerful one."
— Robert Weisbuch, quoted at Critical Mass on Jan. 23.
Related material: Mr. Casaubon and the Key to All Mythologies.
For a simpler key, see On Linguistic Creation.
Thursday, November 19, 2009
Walden for Jews
"Orthodox Jews are disappearing from Jerusalem. One moment they are praying at the Western Wall, and in the blink of an eye, they seem to evaporate…. In order to build the Third Temple while being respectful of the Islamic structures on the Temple Mount, the Jews have discovered a way to access a fourth spatial dimension. They will build the Third Temple invisibly 'above' the Temple Mount and 'above' the Mosque in the direction of the fourth dimension."
— Clifford Pickover, description of his novel Jews in Hyperspace
"If you have built castles in the air, your work need not be lost; that is where they should be. Now put the foundations under them."
— Henry David Thoreau, conclusion of Walden
Related material: Log24 entries, morning and evening of June 11, 2009, "Text" (June 22, 2009), and Salomon Bochner's remarks on space in "Eight is a Gate" (Feb. 26, 2008).
Monday, November 16, 2009
A Wrinkle in Dimensions
Clifford Pickover now seems to be trying to catch up with Christian fantasists Madeleine L’Engle and Charles Williams. Click on the images below for further details.
Thursday, November 5, 2009
Universal Culture Machines
University of California anthropologist Alan Dundes:
"One could well argue that binary opposition is a universal. Presumably all human societies, past and present, made some kind of distinction between 'Male and Female,' 'Life and Death,' 'Day and Night' (or Light and Dark), etc." –"Binary Opposition in Myth: The Propp/Levi-Strauss Debate in Retrospect," Western Folklore, Winter 1997
To Levi-Strauss, I prefer Clifford Geertz —
"…what Levi-Strauss has made for himself is an infernal culture machine." –"The Cerebral Savage"
— and Heinrich Zimmer —
"…all opposition, as well as identity, stems from Maya. Great Maya is wisdom and increase, stability and readiness to assist, compassion and serenity. Queen of the World, she is alive in every nuance of feeling and perception; feelings and perceptions are her gestures. And her nature can be sensed only by one who has comprehended that she is the unity of opposites." —The King and the Corpse
And then there are more up-to-date culture machines.
Levi-Strauss, obtuse and boring, is an opposite, of sorts, to the smart and funny Dundes. The latter, in the binary opposition posed in yesterday's Log24 title "Sinner or Saint?," is definitely on the side of the saints. (See selected Log24 entries for the date of his death– Warren Beatty's birthday.)
Today's happy birthdays — Elke Sommer —
and Sesame Street —
Google logo today, Nov. 5, 2009
Click images for historical background.
Sunday, March 1, 2009
Sunday March 1, 2009
Solomon's Cube
continued
"There is a book… called A Fellow of Trinity, one of series dealing with what is supposed to be Cambridge college life…. There are two heroes, a primary hero called Flowers, who is almost wholly good, and a secondary hero, a much weaker vessel, called Brown. Flowers and Brown find many dangers in university life, but the worst is a gambling saloon in Chesterton run by the Misses Bellenden, two fascinating but extremely wicked young ladies. Flowers survives all these troubles, is Second Wrangler and Senior Classic, and succeeds automatically to a Fellowship (as I suppose he would have done then). Brown succumbs, ruins his parents, takes to drink, is saved from delirium tremens during a thunderstorm only by the prayers of the Junior Dean, has much difficulty in obtaining even an Ordinary Degree, and ultimately becomes a missionary. The friendship is not shattered by these unhappy events, and Flowers's thoughts stray to Brown, with affectionate pity, as he drinks port and eats walnuts for the first time in Senior Combination Room."
— G. H. Hardy, A Mathematician's Apology
"The Solomon Key is the working title of an unreleased novel in progress by American author Dan Brown. The Solomon Key will be the third book involving the character of the Harvard professor Robert Langdon, of which the first two were Angels & Demons (2000) and The Da Vinci Code (2003)." — Wikipedia
"One has O+(6) ≅ S8, the symmetric group of order 8! …."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint.
"The complete projective group of collineations and dualities of the [projective] 3-space is shown to be of order [in modern notation] 8! …. To every transformation of the 3-space there corresponds a transformation of the [projective] 5-space. In the 5-space, there are determined 8 sets of 7 points each, 'heptads' …."
— George M. Conwell, "The 3-space PG(3, 2) and Its Group," The Annals of Mathematics, Second Series, Vol. 11, No. 2 (Jan., 1910), pp. 60-76
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference (July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Friday, June 6, 2008
Friday June 6, 2008
"Harvard seniors have
every right to demand a
Harvard-calibre speaker."
— Adam Goldenberg in
The Harvard Crimson
"Look down now, Cotton Mather"
— Wallace Stevens,
Harvard College
Class of 1901
For Thursday, June 5, 2008,
commencement day for Harvard's
Class of 2008, here are the
Pennsylvania Lottery numbers:
Mid-day 025
Evening 761
Thanks to the late
Harvard professor
Willard Van Orman Quine,
the mid-day number 025
suggests the name
"Isaac Newton."
(For the logic of this suggestion,
see On Linguistic Creation
and Raiders of the Lost Matrix.)
Thanks to Google search, the
name of Newton, combined with
Thursday's evening number 761,
suggests the following essay:
PHILOSOPHY OF SCIENCE:
|
What can a non-scientist add?
Perhaps the Log24 entries for
the date of Koshland's death:
The Philosopher's Stone
and The Rock.
Or perhaps the following
observations:
On the figure of 25 parts
discussed in
"On Linguistic Creation"–
"The Moslems thought of the
central 1 as being symbolic
of the unity of Allah. "
— Clifford Pickover
"At the still point,
there the dance is."
— T. S. Eliot,
Harvard College
Class of 1910
Saturday, March 24, 2007
Saturday March 24, 2007
In face of which desire no longer moved,
Nor made of itself that which it could not find…
Three times the concentred self takes hold, three times
The thrice concentred self, having possessed
The object, grips it in savage scrutiny,
Once to make captive, once to subjugate
Or yield to subjugation, once to proclaim
The meaning of the capture, this hard prize,
Fully made, fully apparent, fully found.”
— “Credences of Summer,” VII,
by Wallace Stevens, from
Transport to Summer (1947)
Clifford Geertz on Levi-Strauss, from The Cerebral Savage:
“Savage logic works like a kaleidoscope whose chips can fall into a variety of patterns…. “
Related material:
The kaleidoscope puzzle and “Claude Levi-Strauss and the Aesthetic Object,” a videotaped interview with Dr. Boris Wiseman.
Wednesday, November 1, 2006
Wednesday November 1, 2006

Professor Emeritus,
Institute for Advanced Study
Savage Logic
"Savage logic works like a kaleidoscope whose chips can fall into a variety of patterns while remaining unchanged in quantity, form, or color. The number of patterns producible in this way may be large if the chips are numerous and varied enough, but it is not infinite. The patterns consist in the disposition of the chips vis-a-vis one another (that is, they are a function of the relationships among the chips rather than their individual properties considered separately). And their range of possible transformations is strictly determined by the construction of the kaleidoscope, the inner law which governs its operation. And so it is too with savage thought. Both anecdotal and geometric, it builds coherent structures out of 'the odds and ends left over from psychological or historical process.'
These odds and ends, the chips of the kaleidoscope, are images drawn from myth, ritual, magic, and empirical lore. (How, precisely, they have come into being in the first place is one of the points on which Levi-Strauss is not too explicit, referring to them vaguely as the 'residue of events… fossil remains of the history of an individual or a society.') Such images are inevitably embodied in larger structures– in myths, ceremonies, folk taxonomies, and so on– for, as in a kaleidoscope, one always sees the chips distributed in some pattern, however ill-formed or irregular. But, as in a kaleidoscope, they are detachable from these structures and arrangeable into different ones of a similar sort. Quoting Franz Boas that 'it would seem that mythological worlds have been built up, only to be shattered again, and that new worlds were built from the fragments,' Levi-Strauss generalizes this permutational view of thinking to savage thought in general."
— Clifford Geertz, "The Cerebral Savage: the Structural Anthropology of Claude Levi-Strauss," in Encounter, Vol. 28 No. 4 (April 1967), pp. 25-32.
Today's New York Times
reports that
Geertz died on Monday,
October 30, 2006.
Related material:
and Up the River:
|
While it's a story that's never been written, a suggested title– Indiana Jones Sails Up The River Of Death–
shows how readily we as individuals or we as a culture can automatically visualize a basic story motif. We may each see the particular elements of the story differently, but almost instantaneously we catch its drift. The hero sails up the river of death to discover what lies within his own heart: i.e., how much moral and physical strength he has. Indiana Jones sails up the River of Death. We are following Indiana Jones up the River of Death. We're going to visit with Colonel Kurtz. (You may not want to get off the boat.) No, I am not mixing up metaphors. These are the Story. |
Amen.
Thursday, August 11, 2005
Saturday, March 12, 2005
Wednesday, March 3, 2004
Wednesday March 3, 2004
Deep Play
In the previous entry, there was a reference to Carl Kaysen, former director of the Institute for Advanced Study at Princeton and father of Susanna Kaysen, author of Girl, Interrupted.
A search for further information on Carl Kaysen led to
Mark Turner, Cognitive Dimensions of Social Science: The Way We Think About Politics, Economics, Law, and Society, Oxford University Press, 2001. For a draft of this work, click here.
Turner's book describes thought and culture in terms of what he calls "blends." It includes a meditation on
Clifford Geertz, "Deep Play: Notes on the Balinese Cockfight," in Dædalus, Journal of the American Academy of Arts and Sciences, issue entitled, "Myth, Symbol, and Culture," Winter 1972, volume 101, number 1
That Turner bases weighty ruminations of what he is pleased to call "social science" on the properties of cockfights suggests that the academic world is, in some respects, even more bizarre than the mental hospital described by Kaysen's daughter.
Still, Turner's concept of "blends" is not without interest.
Here is a blend based on a diagram of the fields in which Turner and Kaysen père labor:
"politics, economics,
law, and society" (Turner)
and "economics, sociology,
politics and law" (Kaysen).
In the previous entry we abstracted from the nature of these academic pursuits, representing them simply as sets in a Venn diagram. This led to the following religious icon, an example of a Turner "blend" —

The Jewel
in Venn's Lotus.
Here is another "blend," related both to the religious material in the previous entry and to Geertz's influential essay.
From my entry for
St. Patrick's Day, 2003:
Summa Theologica
How can you tell there's an Irishman
present at a cockfight?
He enters a duck.
How can you tell a Pole is present?
He bets on the duck.
How can you tell an Italian is present?
The duck wins.
(Source: Blanche Knott,
Truly Tasteless Jokes)
Illustration for the entries
of Oct. 27, 2003:
El Pato-lógico and a

"dream of heaven."
Wednesday, September 3, 2003
Wednesday September 3, 2003
Reciprocity
From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity….
… E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies."
— William Boyd, review of Himmelfarb, New York Times Book Review, October 30, 1994
Last year's entry on this date:
|
Today's birthday:
"Mathematics is the music of reason."
Sylvester, a nineteenth-century mathematician, coined the phrase "synthematic totals" to describe some structures based on 6-element sets that R. T. Curtis has called "rather unwieldy objects." See Curtis's abstract, Symmetric Generation of Finite Groups, John Baez's essay, Some Thoughts on the Number 6, and my website, Diamond Theory. |
The picture above is of the complete graph
Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24.
If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See
Reciprocity and Reversal in Lao Tzu.
For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in
Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the
Click on the design for details.
Those who prefer a Jewish approach to physics can find the star of David, in the form of
A Graphical Representation
of the Dirac Algebra.
The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See
Tuesday, July 1, 2003
Tuesday July 1, 2003
Jew’s on First
This entry is dedicated to those worshippers of Allah who have at one time or another cried
“Itbah al-Yahud!” … Kill the Jew!
(See June 29 entries).
|
Dead at 78 Comedian Buddy Hackett died on Tuesday, July First, 2003, according to the New York Times. According to Bloomberg.com, he died Sunday or Monday. |

Associated Press
Buddy Hackett, |
| Whatever. We may imagine he has now walked, leading a parade of many other stand-up saints, into a bar. |
|
|
MIDRASH From my May 25 entry, Matrix of the Death God: R. M. Abraham’s Diversions and Pastimes, published by Constable and Company, London, in 1933, has the following magic square:
The Matrix of Abraham A summary of the religious import of the above from Princeton University Press: “Moslems of the Middle Ages were fascinated by pandiagonal squares with 1 in the center…. The Moslems thought of the central 1 as being symbolic of the unity of Allah. Indeed, they were so awed by that symbol that they often left blank the central cell on which the 1 should be positioned.” — Clifford A. Pickover, The Zen of Magic Squares, Circles, and Stars, Princeton U. Press, 2002, pp. 71-72 Other appearances of this religious icon on the Web include:
|
In the Picasso’s Birthday version, 22 of the 25 magic square cells are correlated with pictures on the “Class of ’91” cover of Rolling Stone magazine. Number 7 is Rod† Stewart. In accordance with the theological rhyme “Seven is heaven, eight is a gate,” our site music for today is “Forever Young,” a tune made famous by Stewart.
† Roderick, actually — the name of the hero in “Madwoman of Chaillot”
Sunday, May 25, 2003
Sunday May 25, 2003
— ART WARS —
Mental Health Month, Day 25:
Matrix of the Death God
Having dealt yesterday with the Death Goddess Sarah, we turn today to the Death God Abraham. (See Jacques Derrida, The Gift of Death, University of Chicago Press, 1996.) For a lengthy list of pictures of this damned homicidal lunatic about to murder his son, see The Text This Week.

See, too, The Matrix of Abraham, illustrated below. This is taken from a book by R. M. Abraham, Diversions and Pastimes, published by Constable and Company, London, in 1933.

The Matrix of Abraham
A summary of the religious import of the above from Princeton University Press:
“Moslems of the Middle Ages were fascinated by pandiagonal squares with 1 in the center…. The Moslems thought of the central 1 as being symbolic of the unity of Allah. Indeed, they were so awed by that symbol that they often left blank the central cell on which the 1 should be positioned.”
— Clifford A. Pickover, The Zen of Magic Squares, Circles, and Stars, Princeton U. Press, 2002, pp. 71-72
Other appearances of this religious icon on the Web:
A less religious approach to the icon may be found on page 393 of R. D. Carmichael’s Introduction to the Theory of Groups of Finite Order (Ginn, Boston, 1937, reprinted by Dover, 1956).
This matrix did not originate with Abraham but, unlike Neo, I have not yet found its Architect.
Thursday, December 5, 2002
Thursday December 5, 2002
Sacerdotal Jargon
From the website
Abstracts and Preprints in Clifford Algebra [1996, Oct 8]:
Paper: clf-alg/good9601
From: David M. Goodmanson
Address: 2725 68th Avenue S.E., Mercer Island, Washington 98040
Title: A graphical representation of the Dirac Algebra
Abstract: The elements of the Dirac algebra are represented by sixteen 4×4 gamma matrices, each pair of which either commute or anticommute. This paper demonstrates a correspondence between the gamma matrices and the complete graph on six points, a correspondence that provides a visual picture of the structure of the Dirac algebra. The graph shows all commutation and anticommutation relations, and can be used to illustrate the structure of subalgebras and equivalence classes and the effect of similarity transformations….
Published: Am. J. Phys. 64, 870-880 (1996)
The following is a picture of K6, the complete graph on six points. It may be used to illustrate various concepts in finite geometry as well as the properties of Dirac matrices described above.

From
"The Relations between Poetry and Painting,"
by Wallace Stevens:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color. . . . The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space—which he calls the mind or heart of creation— determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."