For Red Riding Hood — Bandini in Vienna.
"Meanwhile, back in 1937 . . ." — Weyl in Vienna.
For Red Riding Hood — Bandini in Vienna.
"Meanwhile, back in 1937 . . ." — Weyl in Vienna.
The conclusion of a Hungarian political figure's obituary in
tonight's online New York Times, written by Clay Risen —
"A quietly religious man, he spent his last years translating
works dealing with Roman Catholic canon law."
This journal on the Hungarian's date of death, October 8,
a Sunday, dealt in part with the submission to Wikipedia of
the following brief article . . . and its prompt rejection.
|
The Cullinane diamond theorem is a theorem
The theorem also explains symmetry properties of the Reference
1. Cullinane diamond theorem at |
Some quotations I prefer to Catholic canon law —
|
Ludwig Wittgenstein,
97. Thought is surrounded by a halo. * See the post Wittgenstein's Diamond. Related language in Łukasiewicz (1937)— |
See as well Diamond Theory in 1937.
Poet Wallace Stevens was born 140 years ago today.
For another 140, see Diamond Theory in 1937.
For some notes related to a Stevens poem from 1937,
see "arrowy, still strings" in this journal.
From a Log24 post of March 4, 2008 —
|
SINGER, ISAAC:
"Sets forth his own aims in writing for children and laments
— An Annotated Listing of Criticism
"She returned the smile, then looked across the room to
— A Swiftly Tilting Planet,
For "the dimension of time," see A Fold in Time, Time Fold,
A Swiftly Tilting Planet is a fantasy for children |
Ibid. —
The pen's point:

John Trever, Albuquerque Journal, 2/29/08
Note the figure on the cover of National Review above —

A related figure from Pentagram Design —
See, more generally, Isaac Singer in this journal.
The title is that of a presentation by Arnold Emch
at the 1928 International Congress of Mathematicians:
See also yesterday's "Emch as a Forerunner of S(5, 8, 24)."
Related material: Diamond Theory in 1937.
Further remarks: Christmas 2013 and the fact that
759 × 322,560 = the order of the large Mathieu group M24 .

Nan Tucker McEvoy, last of founding family
to run Chronicle, dies
By Sam Whiting at SFGate.com, Friday, March 27, 2015
From the story —
"After graduating from Dominican Convent Upper School
in San Rafael in 1937, she was discouraged from attending college
by family members who wanted her to be a socialite."
Related material —
"There is such a thing as a tesseract." — Madeleine L'Engle
An approach via the Omega Matrix:
See, too, Rosenhain and Göpel as The Shadow Guests .
"I need a photo opportunity, I want a shot at redemption.
Don't want to end up a cartoon in a cartoon graveyard."
– Paul Simon

|
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less.
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951) |
For background on the planes illustrated above,
see Diamond theory in 1937.
“… her mind rotated the facts….”
Related material— hypercube rotation,* in the context
of rotational symmetries of the Platonic solids:

“I’ve heard of affairs that are strictly Platonic”
* Footnote added on Dec. 26, 2013 —
See Arnold Emch, “Triple and Multiple Systems, Their Geometric
Configurations and Groups,” Trans. Amer. Math. Soc. 31 (1929),
No. 1, 25–42.
On page 42, Emch describes the above method of rotating a
hypercube’s 8 facets (i.e., three-dimensional cubes) to count
rotational symmetries —
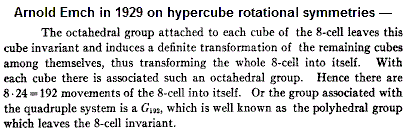
See also Diamond Theory in 1937.
Also on p. 42, Emch mentions work of Carmichael on a
Steiner system with the Mathieu group M11 as automorphism
group, and poses the problem of finding such systems and
groups that are larger. This may have inspired the 1931
discovery by Carmichael of the Steiner system S(5, 8, 24),
which has as automorphisms the Mathieu group M24 .
Mathematics:
A review of posts from earlier this month —
Wednesday, September 4, 2013
|
Narrative:

Aooo.
Happy birthday to Stephen King.
"How do you get young people excited
about space? How do you get them interested
not just in watching movies about space,
or in playing video games set in space …
but in space itself?"
— Megan Garber in The Atlantic , Aug. 16, 2012
One approach:
"There is such a thing as a tesseract" and
Diamond Theory in 1937.
See, too, Baez in this journal.
The finite (i.e., Galois) field GF(16),
according to J. J. Seidel in 1974—
The same field according to Steven H. Cullinane in 1986,
in its guise as the affine 4-space over GF(2)—

The same field, again disguised as an affine 4-space,
according to John H. Conway and N.J.A. Sloane in
Sphere Packings, Lattices, and Groups , first published in 1988—

The above figure by Conway and Sloane summarizes, using
a 4×4 array, the additive vector-space structure of the finite
field GF(16).
This structure embodies what in Euclidean space is called
the parallelogram rule for vector addition—

(Thanks to June Lester for the 3D (uvw) part of the above figure.)
For the transition from this colored Euclidean hypercube
(used above to illustrate the parallelogram rule) to the
4×4 Galois space (illustrated by Cullinane in 1979 and
Conway and Sloane in 1988— or later… I do not have
their book’s first edition), see Diamond Theory in 1937,
Vertex Adjacency in a Tesseract and in a 4×4 Array,
Spaces as Hypercubes, and The Galois Tesseract.
For some related narrative, see tesseract in this journal.
(This post has been added to finitegeometry.org.)
Update of August 9, 2013—
Coordinates for hypercube vertices derived from the
parallelogram rule in four dimensions were better
illustrated by Jürgen Köller in a web page archived in 2002.
Update of August 13, 2013—
The four basis vectors in the 2002 Köller hypercube figure
are also visible at the bottom of the hypercube figure on
page 7 of “Diamond Theory,” excerpts from a 1976 preprint
in Computer Graphics and Art , Vol. 2, No. 1, February 1977.
A predecessor: Coxeter’s 1950 hypercube figure from
“Self-Dual Configurations and Regular Graphs.”
(An episode of Mathematics and Narrative )
A report on the August 9th opening of Sondheim's Into the Woods—
Amy Adams… explained why she decided to take on the role of the Baker’s Wife.
“It’s the ‘Be careful what you wish’ part,” she said. “Since having a child, I’m really aware that we’re all under a social responsibility to understand the consequences of our actions.” —Amanda Gordon at businessweek.com
Related material—
Amy Adams in Sunshine Cleaning "quickly learns the rules and ropes of her unlikely new market. (For instance, there are products out there specially formulated for cleaning up a 'decomp.')" —David Savage at Cinema Retro
Compare and contrast…
1. The following item from Walpurgisnacht 2012—
2. The six partitions of a tesseract's 16 vertices
into four parallel faces in Diamond Theory in 1937—

Literary remarks for Maundy Thursday—

— C. P. Snow, foreword to G. H. Hardy's A Mathematician's Apology
Related material—
Emory University press release of January 20th, 2011:
"In 1937, Hans Rademacher found an exact formula for calculating partition values. While the method was a big improvement over Euler's exact formula, it required adding together infinitely many numbers that have infinitely many decimal places. 'These numbers are gruesome,' Ono says….
… The final eureka moment occurred near another Georgia landmark: Spaghetti Junction. Ono and Jan Bruinier were stuck in traffic near the notorious Atlanta interchange. While chatting in the car, they hit upon a way to overcome the infinite complexity of Rademacher's method. They went on to prove a formula that requires only finitely many simple numbers.
'We found a function, that we call P, that is like a magical oracle,' Ono says. 'I can take any number, plug it into P, and instantly calculate the partitions of that number….'"
See also this journal on April 15 and a Google Groups [sage-devel] thread, Ono-Bruinier partition formula. That thread started on April 15 and was last updated this morning.
The late mathematician V.I. Arnold was born on this date in 1937.
"By groping toward the light we are made to realize
how deep the darkness is around us."
— Arthur Koestler, The Call Girls: A Tragi-Comedy
Light
Choosing light rather than darkness, we observe Arnold's birthday with a quotation from his 1997 Paris talk 'On Teaching Mathematics.'
"The Jacobi identity (which forces the heights of a triangle to cross at one point) is an experimental fact…."
The "experimental fact" part, perhaps offered with tongue in cheek, is of less interest than the assertion that the Jacobi identity forces the altitude-intersection theorem.
Albert Einstein on that theorem in the "holy geometry book" he read at the age of 12—
"Here were assertions, as for example the intersection of the three altitudes of a triangle in one point, which– though by no means evident– could nevertheless be proved with such certainty that any doubt appeared to be out of the question. This lucidity and certainty made an indescribable impression upon me.”
Arnold's much less evident assertion about altitudes and the Jacobi identity is discussed in "Arnol'd, Jacobi identity, and orthocenters" (pdf) by Nikolai V. Ivanov.
Ivanov says, without giving a source, that the altitudes theorem "was known to Euclid." Alexander Bogomolny, on the other hand, says it is "a matter of real wonderment that the fact of the concurrency of altitudes is not mentioned in either Euclid's Elements or subsequent writings of the Greek scholars. The timing of the first proof is still an open question."
For other remarks on geometry, search this journal for the year of Arnold's birth.
This journal’s Christmas Day entry, Brightness at Noon, was in response to the Orwellian headline “Arthur Koestler, Man of Darkness,” at the top of the online New York Times front page on Christmas morning.
The entry offered, as an example of brightness, some thoughts of Leibniz on his discovery of binary arithmetic.
Related material:
“To make all things from nothing, unity suffices.” So it is written on a medal entitled Imago Creationis and designed by Leibniz to “exhibit to posterity in silver” his discovery of the binary system. Baron Gottfried Wilhelm von Leibniz (also Leibnitz) 1646-1716. Philosopher and mathematician. Invented calculus independently of Newton. Proposed the metaphysical theory that we live in “the best of all possible worlds.” He also discovered binary number system and believed in its profound metaphysical significance. He noticed similarity with the ancient Chinese divination system “I Ching.” We chose him for our patron, for Krawtchuk polynomials can be understood as a sophistication of the simple counting of 0 and 1… — Philip Feinsilver and Jerzy Kocik, 17 July 2001 |
From Mikhail Krawtchouk: Short Biography—
Anyone knowing even a little Soviet history of the thirties can conclude that Krawtchouk could not avoid the Great Terror. During the Orwellian “hours of hatred” in 1937 he was denounced as a “Polish spy,” “bourgeois nationalist,” etc. In 1938, he was arrested and sentenced to 20 years of confinement and 5 years of exile.
Academician Krawtchouk, the author of results which became part of the world’s mathematical knowledge, outstanding lecturer, member of the French, German, and other mathematical societies, died on March 9, 1942, in Kolyma branch of the GULAG (North-Eastern Siberia) more than 6 months short of his 50th birthday.
Incidentally, happy birthday
to John von Neumann.
From the September 1953 Bulletin of the American Mathematical Society—
Emil Artin, in a review of Éléments de mathématique, by N. Bourbaki, Book II, Algebra, Chaps. I-VII–
"We all believe that mathematics is an art. The author of a book, the lecturer in a classroom tries to convey the structural beauty of mathematics to his readers, to his listeners. In this attempt he must always fail. Mathematics is logical to be sure; each conclusion is drawn from previously derived statements. Yet the whole of it, the real piece of art, is not linear; worse than that its perception should be instantaneous. We all have experienced on some rare occasions the feeling of elation in realizing that we have enabled our listeners to see at a moment's glance the whole architecture and all its ramifications. How can this be achieved? Clinging stubbornly to the logical sequence inhibits the visualization of the whole, and yet this logical structure must predominate or chaos would result."
Art Versus Chaos

From an exhibit,
"Reimagining Space"
The above tesseract (4-D hypercube)
sculpted in 1967 by Peter Forakis
provides an example of what Artin
called "the visualization of the whole."
For related mathematical details see
Diamond Theory in 1937.
"'The test?' I faltered, staring at the thing.
'Yes, to determine whether you can live
in the fourth dimension or only die in it.'"
— Fritz Leiber, 1959
See also the Log24 entry for
Nov. 26, 2009, the date that
Forakis died.
"There is such a thing
as a tesseract."
— Madeleine L'Engle, 1962
From Braque's birthday, 2006:
"The senses deform, the mind forms. Work to perfect the mind. There is no certitude but in what the mind conceives."
— Georges Braque,
Reflections on Painting, 1917
Those who wish to follow Braque's advice may try the following exercise from a book first published in 1937:

For a different view
of the square and cube
see yesterday's entry
Abstraction and Faith.
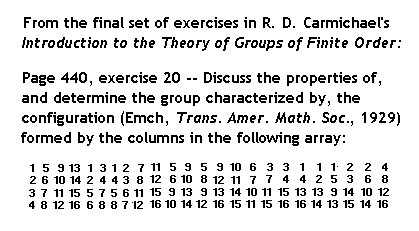
On April 16, the Pope’s birthday, the evening lottery number in Pennsylvania was 441. The Log24 entries of April 17 and April 18 supplied commentaries based on 441’s incarnation as a page number in an edition of Heidegger’s writings. Here is a related commentary on a different incarnation of 441. (For a context that includes both today’s commentary and those of April 17 and 18, see Gian-Carlo Rota– a Heidegger scholar as well as a mathematician– on mathematical Lichtung.)
From R. D. Carmichael, Introduction to the Theory of Groups of Finite Order (Boston, Ginn and Co., 1937)– an exercise from the final page, 441, of the final chapter, “Tactical Configurations”–
“23. Let G be a multiply transitive group of degree n whose degree of transitivity is k; and let G have the property that a set S of m elements exists in G such that when k of the elements S are changed by a permutation of G into k of these elements, then all these m elements are permuted among themselves; moreover, let G have the property P, namely, that the identity is the only element in G which leaves fixed the n – m elements not in S. Then show that G permutes the m elements S into
m(m – 1) … (m – k + 1)
This exercise concerns an important mathematical structure said to have been discovered independently by the American Carmichael and by the German Ernst Witt.
For some perhaps more comprehensible material from the preceding page in Carmichael– 440– see Diamond Theory in 1937.

John Trever, Albuquerque Journal, 2/29/08
The pen's point:
|
Log24, Dec. 11, 2006
SINGER, ISAAC:
"Sets forth his own aims in writing for children and laments 'slice of life' and chaos in children's literature. Maintains that children like good plots, logic, and clarity, and that they have a concern for 'so-called eternal questions.'"
— An Annotated Listing
"She returned the smile, then looked across the room to her youngest brother, Charles Wallace, and to their father, who were deep in concentration, bent over the model they were building of a tesseract: the square squared, and squared again: a construction of the dimension of time."
— A Swiftly Tilting Planet,
A Swiftly Tilting Planet is a fantasy for children set partly in Vespugia, a fictional country bordered by Chile and Argentina.
|
The title of the previous entry, "Where Entertainment is God," comes (via Log24, Nov. 26, 2004) from Frank Rich.
The previous entry dealt, in part, with a dead Jesuit whose obituary appears in today's Los Angeles Times. The online obituaries page places the Jesuit, without a photo, beneath a picture of a dead sitcom writer and to the left of a picture of a dead guru.
"Walter John Burghardt was born July 10, 1914, in New York, the son of immigrants from what is now Poland. He entered a Jesuit seminary in Poughkeepsie, N.Y., at 16, and in 1937 received a master's degree from Woodstock College in Maryland. He was ordained in 1941." He died, by the way, on Saturday, Feb. 16, 2008.
The reference to Woodstock College brings to mind a fellow Jesuit, Joseph T. Clark, who wrote a book on logic published by that college.
From a review of the book:
"In order to show that Aristotelian logicians were at least vaguely aware of a kind of analogy or possible isomorphism between logical relations and mathematical relations, Father Clark seizes at one place (p. 8) upon the fact that Aristotle uses the word, 'figure' (schema), in describing the syllogism and concludes from this that 'it is obvious that the schema of the syllogism is to serve the logician precisely as the figure serves the geometer.' On the face of it, this strikes one as a bit far fetched…."
— Henry Veatch in Speculum, Vol. 29, No. 2, Part 1 (Apr., 1954), pp. 266-268 (review of Conventional Logic and Modern Logic: A Prelude to Transition (1952), by Joseph T. Clark, Society of Jesus)
J. G. Ballard on “the architecture of death“:
“… a huge system of German fortifications that included the Siegfried line, submarine pens and huge flak towers that threatened the surrounding land like lines of Teutonic knights. Almost all had survived the war and seemed to be waiting for the next one, left behind by a race of warrior scientists obsessed with geometry and death.”
— The Guardian, March 20, 2006
“For him, writing is a struggle both with geometry and death.”
— “The Duende,” American Poetry Review, July/August 1999
— Harper’s Magazine review
quoted on back cover of
Cubism and Twentieth-Century Art,
by Robert Rosenblum
(Abrams paperback, 2001)
— An Annotated Listing of Criticism
by Linnea Hendrickson
“She returned the smile, then looked
across the room to her youngest brother,
Charles Wallace, and to their father,
who were deep in concentration, bent
over the model they were building
of a tesseract: the square squared,
and squared again: a construction
of the dimension of time.”
— A Swiftly Tilting Planet,
by Madeleine L’Engle

For “the dimension of time,”
see A Fold in Time,
Time Fold, and
Diamond Theory in 1937.
For a more adult audience —
In memory of General Augusto Pinochet, who died yesterday in Santiago, Chile, a quotation from Federico Garcia Lorca‘s lecture on “the Duende” (Buenos Aires, Argentina, 1933):
Casino Royale
and
Time in the Rock

In today’s cognitive blend,
the role of Casino Royale
is played by the
Pennsylvania Lottery,
which points to 7/26,
Venus at St. Anne’s
(title of the closing chapter
of That Hideous Strength).
The role of
Time in the Rock
is played by a
Log24 entry of 3/29,
Diamond Theory in 1937.
“There is such a thing
as a tesseract.“
From 7/07, an art review from The New York Times:
Endgame Art?
It's Borrow, Sample and Multiply
in an Exhibition at Bard College
"The show has an endgame, end-time mood….
I would call all these strategies fear of form…. the dismissal of originality is perhaps the oldest ploy in the postmodern playbook. To call yourself an artist at all is by definition to announce a faith, however unacknowledged, in some form of originality, first for yourself, second, perhaps, for the rest of us.
Fear of form above all means fear of compression– of an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible."
— Roberta Smith
It nevertheless does
"announce a faith."

"First for yourself"
Today's mid-day
Pennsylvania number:
707
See Log24 on 7/07
and the above review.
"Second, perhaps,
for the rest of us"
Today's evening
Pennsylvania number:
384
This number is an
example of what the
reviewer calls "compression"–
"an artistic focus that condenses
experiences, ideas and feelings
into something
whole, committed
and visually comprehensible."
"Experiences"
See (for instance)
Joan Didion's writings
(1160 pages, 2.35 pounds)
on "the shifting phantasmagoria
which is our actual experience."
"Ideas"
"Feelings"
See A Wrinkle in Time.
"Whole"
The automorphisms
of the tesseract
form a group
of order 384.
"Committed"
See the discussions of
groups of degree 16 in
R. D. Carmichael's classic
Introduction to the Theory
of Groups of Finite Order.
"Visually comprehensible"
See "Diamond Theory in 1937,"
an excerpt from which
is shown below.

The "faith" announced by
the above lottery numbers
on All Hallows' Eve is
perhaps that of the artist
Madeleine L'Engle:
ART WARS continued…
|
|
 |
“The senses deform, the mind forms. Work to perfect the mind. There is no certitude but in what the mind conceives.”
— Georges Braque, Reflections on Painting, 1917
Those who wish to follow Braque’s advice may try the following exercise from a book first published in 1937:

Hint: See the above picture of
Braque and the construction of
a tesseract.
— Cynthia Zarin on Madeleine L’Engle,
“The Storyteller,” in The New Yorker,
issue dated April 12, 2004
"Does the word 'tesseract'
mean anything to you?"
— Robert A. Heinlein in
The Number of the Beast
(1980)
My reply–
Part I:

A Wrinkle in Time, by
Madeleine L'Engle
(first published in 1962)
Part II:

Diamond Theory in 1937
and
Geometry of the 4×4 Square
Part III:
"Wells and trees were dedicated to saints. But the offerings at many wells and trees were to something other than the saint; had it not been so they would not have been, as we find they often were, forbidden. Within this double and intertwined life existed those other capacities, of which we know more now, but of which we still know little– clairvoyance, clairaudience, foresight, telepathy."
— Charles Williams, Witchcraft, Faber and Faber, London, 1941
A New Yorker profile of Madeleine L'Engle from April 2004, which I found tonight online for the first time. For a related reflection on truth, stories, and values, see Saint's Day. For a wider context, see the Log24 entries of February 1-15, 2003 and February 1-15, 2006.
2:23 PM
Sequel
to the previous two entries
"This world is not conclusion;
A sequel stands beyond…."
— Emily Dickinson
Today's birthday: dancer/actress Ann Miller.
"In 1937, she was discovered by Lucille Ball…."

Lucille Ball, Desi Arnaz,
and Ann Miller, cast photo
from Too Many Girls (1940)
"Just goes to show star quality shines through…."
— Website on Too Many Girls"It'll shine when it shines."
— Folk saying, epigraph to The Shining"Shine on, you crazy diamond."
— Pink Floyd"Well we all shine on…"
— John Lennon, "Instant Karma"
On This Date
|
|
In 1937, composer Our site music for today For “Bolero” purposes, some may prefer Kylie Minogue’s rendition of “Locomotion.” |
Zen meditation: “Kylie Eleison!”
(For evidence that this is a valid Japanese religious exclamation, click here.)
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.
Powered by WordPress