For Jung’s 7/26 Birthday:
A Logocentric Meditation
Leftist academics are trying to pull a fast one again. An essay in the most prominent American mathematical publication tries to disguise a leftist attack on Christian theology as harmless philosophical woolgathering.
In a review of Vladimir Tasic’s Mathematics and the Roots of Postmodern Thought, the reviewer, Michael Harris, is being less than candid when he discusses Derrida’s use of “logocentrism”:
“Derrida uses the term ‘logocentrism’… as ‘the metaphysics of phonetic writing’….”
— Notices of the American Mathematical Society, August 2003, page 792
We find a rather different version of logocentrism in Tasic’s own Sept. 24, 2001, lecture “Poststructuralism and Deconstruction: A Mathematical History,” which is “an abridged version of some arguments” in Tasic’s book on mathematics and postmodernism:
“Derrida apparently also employs certain ideas of formalist mathematics in his critique of idealist metaphysics: for example, he is on record saying that ‘the effective progress of mathematical notation goes along with the deconstruction of metaphysics.’
Derrida’s position is rather subtle. I think it can be interpreted as a valiant sublation of two completely opposed schools in mathematical philosophy. For this reason it is not possible to reduce it to a readily available philosophy of mathematics. One could perhaps say that Derrida continues and critically reworks Heidegger’s attempt to ‘deconstruct’ traditional metaphysics, and that his method is more ‘mathematical’ than Heidegger’s because he has at his disposal the entire pseudo-mathematical tradition of structuralist thought. He has himself implied in an interview given to Julia Kristeva that mathematics could be used to challenge ‘logocentric theology,’ and hence it does not seem unreasonable to try looking for the mathematical roots of his philosophy.”
The unsuspecting reader would not know from Harris’s review that Derrida’s main concern is not mathematics, but theology. His ‘deconstruction of metaphysics’ is actually an attack on Christian theology.
From “Derrida and Deconstruction,” by David Arneson, a University of Manitoba professor and writer on literary theory:
“Logocentrism: ‘In the beginning was the word.’ Logocentrism is the belief that knowledge is rooted in a primeval language (now lost) given by God to humans. God (or some other transcendental signifier: the Idea, the Great Spirit, the Self, etc.) acts a foundation for all our thought, language and action. He is the truth whose manifestation is the world.”
Some further background, putting my July 23 entry on Lévi-Strauss and structuralism in the proper context:
Part I. The Roots of Structuralism
“Literary science had to have a firm theoretical basis…”
Part II. Structuralism/Poststructuralism
“Most [structuralists] insist, as Levi-Strauss does, that structures are universal, therefore timeless.”
Part III. Structuralism and
Jung’s Archetypes
Jung’s “theories, like those of Cassirer and Lévi-Strauss, command for myth a central cultural position, unassailable by reductive intellectual methods or procedures.”
And so we are back to logocentrism, with the Logos — God in the form of story, myth, or archetype — in the “central cultural position.”
What does all this have to do with mathematics? See
Plato’s Diamond,
Rosalind Krauss on Art –
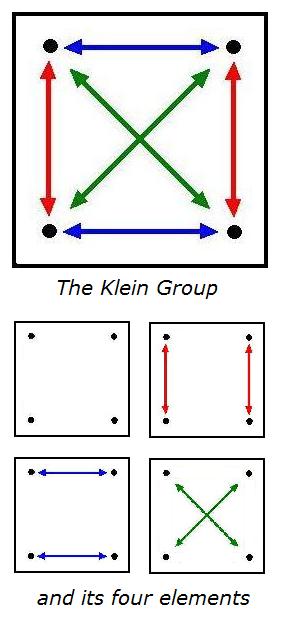
“the Klein group (much beloved of Structuralists)”
Another Michael Harris Essay, Note 47 –
“From Krauss’s article I learned that the Klein group is also called the Piaget group.”
and Jung on Quaternity:
Beyond the Fringe –
“…there is no denying the fact that [analytical] psychology, like an illegitimate child of the spirit, leads an esoteric, special existence beyond the fringe of what is generally acknowledged to be the academic world.”
leads an esoteric, special existence beyond the fringe of what is generally acknowledged to be the academic world.”
What attitude should mathematicians have towards all this?
Towards postmodern French
atheist literary/art theorists –
Mathematicians should adopt the attitude toward “the demimonde of chic academic theorizing” expressed in Roger Kimball’s essay, Feeling Sorry for Rosalind Krauss.
Towards logocentric German
Christian literary/art theorists –
Mathematicians should, of course, adopt a posture of humble respect, tugging their forelocks and admitting their ignorance of Christian theology. They should then, if sincere in their desire to honestly learn something about logocentric philosophy, begin by consulting the website
The Quest for the Fiction of an Absolute.
 For a better known, if similarly disrespected, “illegitimate child of the spirit,” see my July 22 entry.
For a better known, if similarly disrespected, “illegitimate child of the spirit,” see my July 22 entry.


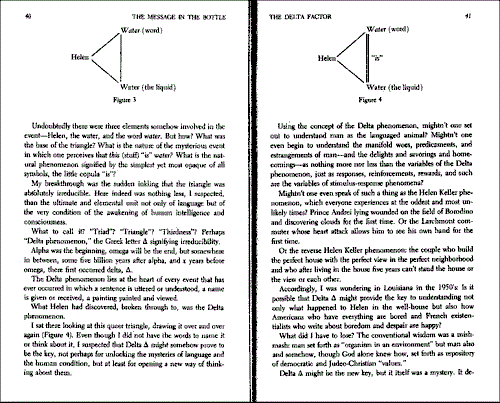






































 The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…."
The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…." leads an esoteric, special existence beyond the fringe of what is generally acknowledged to be the academic world.”
leads an esoteric, special existence beyond the fringe of what is generally acknowledged to be the academic world.”