The previous post discussed the phrase "plot structure."
A different approach —
Textbook art from 1974 —
See as well a more interesting book I enjoyed reading in 1974.
Monday, November 14, 2022
Monday, February 22, 2021
Design Theory
Comments Off on Design Theory
Friday, December 25, 2020
Design Theory
| Mathematics
The Fano plane block design |
Magic
The Deathly Hallows symbol— |
Another name for the Fano plane design — The Ghostly Hallows.
From a search in this journal for Ghostly —
Comments Off on Design Theory
Friday, January 17, 2020
Design Theory
On a recently deceased professor emeritus of architecture
at Princeton —
“… Maxwell ‘established the school as a principal
center of design research, history and theory.’ ”
“This is not the Maxwell you’re looking for.”
Comments Off on Design Theory
Sunday, August 25, 2019
Design Theory
"Mein Führer… Steiner…"
See Hitler Plans and Quadruple System.
"There is such a thing as a quadruple system."
— Saying adapted from a 1962 young-adult novel
Comments Off on Design Theory
Friday, August 9, 2019
Friday, January 25, 2019
Design Theory
Last night's post "Night at the Social Media" suggests . . .
A 404 for Katherine Neville (born on 4/04) —
Comments Off on Design Theory
Tuesday, March 29, 2011
Design Theory
See the new note Configurations and Squares at finitegeometry.org/sc/.
Comments Off on Design Theory
Wednesday, July 10, 2024
Hexagram 52: Ken
Today's description of Dartmouth College as a "gin-soaked gutter"
by Margaret Soltan (i.e., University Diaries) suggests a review:
Monday, November 14, 2022
|
See also "KenKen" and today's previous post.
Comments Off on Hexagram 52: Ken
Saturday, June 29, 2024
Desperately Seeking Sustainable Design
See Design Theory and Sustainable in this journal.
Comments Off on Desperately Seeking Sustainable Design
Monday, December 19, 2022
Mathematics and Narrative, Continued . . .
“Apart from that, Mrs. Lincoln . . .”
Midrash from Philip Pullman . . .
"The 1929 Einstein-Carmichael Expedition"
I prefer the 1929 Emch-Carmichael expedition —
This is from . . .
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer
(Ch. 14 (pp. 693-746) of Handbook of Combinatorics,
Vol. I, MIT Press, 1995, edited by Ronald L. Graham,
Martin Grötschel, and László Lovász, Section 16 (p. 716))
Comments Off on Mathematics and Narrative, Continued . . .
“Apart from that, Mrs. Lincoln . . .”
“Apart from that, Mrs. Lincoln . . .”
Wednesday, July 15, 2020
A Four-Color Diamond
Browsing related to the graphic design theory described in the previous post
yielded a four-color diamond illustrating design at Microsoft —
For some related mathematics see . . .
The Four-Color Diamond’s 2007 Source —
See also Log24 posts from August 2007 now tagged The Four-Color Ring.
Comments Off on A Four-Color Diamond
Category Theory
A related quotation:
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer
(Ch. 14 (pp. 693-746) of Handbook of Combinatorics,
Vol. I, MIT Press, 1995, edited by Ronald L. Graham,
Martin Grötschel, and László Lovász, Section 16 (p. 716))
See also the webpage Block Designs in Art and Mathematics
and Log24 posts tagged Plastic Elements.
Comments Off on Category Theory
Friday, January 17, 2020
September Morn
Epigraph from Ch. 4 of Design Theory , Vol. I:
"Es is eine alte Geschichte,
doch bleibt sie immer neu "
—Heine (Lyrisches Intermezzo XXXIX)
This epigraph was quoted here earlier on
the morning of September 1, 2011.
Comments Off on September Morn
Thursday, August 15, 2019
Schoolgirl Space — Tetrahedron or Square?
The exercise in the previous post was suggested by a passage
purporting to "use standard block design theory" that was written
by some anonymous author at Wikipedia on March 1, 2019:
Here "rm OR" apparently means "remove original research."
Before the March 1 revision . . .
The "original research" objected to and removed was the paragraph
beginning "To explain this further." That paragraph was put into the
article earlier on Feb. 28 by yet another anonymous author (not by me).
An account of my own (1976 and later) original research on this subject
is pictured below, in a note from Feb. 20, 1986 —
Comments Off on Schoolgirl Space — Tetrahedron or Square?
Tuesday, September 12, 2017
Think Different
The New York Times online this evening —
"Mr. Jobs, who died in 2011, loomed over Tuesday’s
nostalgic presentation. The Apple C.E.O., Tim Cook,
paid tribute, his voice cracking with emotion, Mr. Jobs’s
steeple-fingered image looming as big onstage as
Big Brother’s face in the classic Macintosh '1984' commercial."
Review —
|
Thursday, September 1, 2011
How It Works
|
See also 1984 Bricks in this journal.
Comments Off on Think Different
Saturday, September 9, 2017
How It Works
Del Toro and the History of Mathematics ,
Or: Applied Bullshit Continues
For del Toro —
For the history of mathematics —
|
Thursday, September 1, 2011
How It Works
|
Comments Off on How It Works
Thursday, December 26, 2013
How It Works
“Design is how it works.” — Steve Jobs
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer (Ch. 14 (pp. 693-746),
Section 16 (p. 716) of Handbook of Combinatorics, Vol. I ,
MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel,
and László Lovász)
For some background on that Steiner system, see the footnote to
yesterday’s Christmas post.
Comments Off on How It Works
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—
Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Comments Off on Vril Chick
Thursday, September 1, 2011
How It Works
“Design is how it works.” — Steven Jobs (See Symmetry and Design.)
“By far the most important structure in design theory is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer
The name Carmichael is not to be found in Booher’s thesis. A book he does cite for the history of S(5,8,24) gives the date of Carmichael’s construction of this design as 1937. It should be dated 1931, as the following quotation shows—
From Log24 on Feb. 20, 2010—
“The linear fractional group modulo 23 of order 24•23•11 is often represented as a doubly transitive group of degree 24 on the symbols ∞, 0, 1, 2,…, 22. This transitive group contains a subgroup of order 8 each element of which transforms into itself the set ∞, 0, 1, 3, 12, 15, 21, 22 of eight elements, while the whole group transforms this set into 3•23•11 sets of eight each. This configuration of octuples has the remarkable property that any given set of five of the 24 symbols occurs in one and just one of these octuples. The largest permutation group Γ on the 24 symbols, each element of which leaves this configuration invariant, is a five-fold transitive group of degree 24 and order 24•23•22•21•20•48. This is the Mathieu group of degree 24.”
– R. D. Carmichael, “Tactical Configurations of Rank Two,” in American Journal of Mathematics, Vol. 53, No. 1 (Jan., 1931), pp. 217-240
Epigraph from Ch. 4 of Design Theory , Vol. I:
“Es is eine alte Geschichte,
doch bleibt sie immer neu ”
—Heine (Lyrisches Intermezzo XXXIX)
See also “Do you like apples?“
Comments Off on How It Works
Wednesday, August 24, 2011
Symmetry
An article from cnet.com tonight —
For Jobs, design is about more than aesthetics
By: Jay Greene
… The look of the iPhone, defined by its seamless pane of glass, its chrome border, its perfect symmetry, sparked an avalanche of copycat devices that tried to mimic its aesthetic.
Virtually all of them failed. And the reason is that Jobs understood that design wasn't merely about what a product looks like. In a 2003 interview with the New York Times' Rob Walker detailing the genesis of the iPod, Jobs laid out his vision for product design.
''Most people make the mistake of thinking design is what it looks like,'' Jobs told Walker. "People think it's this veneer— that the designers are handed this box and told, 'Make it look good!' That's not what we think design is. It's not just what it looks like and feels like. Design is how it works.''
Related material: Open, Sesame Street (Aug. 19) continues… Brought to you by the number 24—
"By far the most important structure in design theory is the Steiner system
— "Block Designs," by Andries E. Brouwer (Ch. 14 (pp. 693-746) of Handbook of Combinatorics , Vol. I, MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel, and László Lovász, Section 16 (p. 716))
Comments Off on Symmetry
Saturday, June 26, 2010
Plato’s Logos
"The present study is closely connected with a lecture* given by Prof. Ernst Cassirer at the Warburg Library whose subject was 'The Idea of the Beautiful in Plato's Dialogues'…. My investigation traces the historical destiny of the same concept…."
* See Cassirer's Eidos und Eidolon : Das Problem des Schönen und der Kunst in Platons Dialogen, in Vorträge der Bibliothek Warburg II, 1922/23 (pp. 1–27). Berlin and Leipzig, B.G. Teubner, 1924.
— Erwin Panofsky, Idea: A Concept in Art Theory, foreword to the first German edition, Hamburg, March 1924
On a figure from Plato's Meno—
The above figures illustrate Husserl's phrase "eidetic variation"—
a phrase based on Plato's use of eidos, a word
closely related to the word "idea" in Panofsky's title.
For remarks by Cassirer on the theory of groups, a part of
mathematics underlying the above diamond variations, see
his "The Concept of Group and the Theory of Perception."
Sketch of some further remarks—
The Waterfield question in the sketch above
is from his edition of Plato's Theaetetus
(Penguin Classics, 1987).
The "design theory" referred to in the sketch
is that of graphic design, which includes the design
of commercial logos. The Greek word logos
has more to do with mathematics and theology.
"If there is one thread of warning that runs
through this dialogue, from beginning to end,
it is that verbal formulations as such are
shot through with ambiguity."
— Rosemary Desjardins, The Rational Enterprise:
Logos in Plato's Theaetetus, SUNY Press, 1990
Related material—
Comments Off on Plato’s Logos
Monday, August 17, 2009
Monday August 17, 2009
Design Theory,
continued
continued
“… Kirkman has established an incontestable claim to be regarded as the founding father of the theory of designs.”
— “T.P. Kirkman, Mathematician,” by N.L. Biggs, Bulletin of the London Mathematical Society, Volume 13, Number 2 (March 1981), 97-120.
This paper is now available online for $12.
For more about this subject, see Design Theory, by Beth, Jungnickel, and Lenz, Cambridge U. Press, Volume I (2nd ed., 1999, 1120 pages) and Volume II (2nd ed., 2000, 513 pages).
For an apparently unrelated subject with the same name, see Graphic Design Theory: Readings from the Field, by Helen Armstrong (Princeton Architectural Press, 2009).
For what the two subjects have in common, see Block Designs in Art and Mathematics.
Comments Off on Monday August 17, 2009
Tuesday, May 19, 2009
Tuesday May 19, 2009
Exquisite Geometries
"By far the most important structure in design theory is the Steiner system
— "Block Designs," 1995, by Andries E. Brouwer
"The Steiner system S(5, 8, 24) is a set S of 759 eight-element subsets ('octads') of a twenty-four-element set T such that any five-element subset of T is contained in exactly one of the 759 octads. Its automorphism group is the large Mathieu group M24."
— The Miracle Octad Generator (MOG) of R.T. Curtis (webpage)
"… in 1861 Mathieu… discovered five multiply transitive permutation groups…. In a little-known 1931 paper of Carmichael… they were first observed to be automorphism groups of exquisite finite geometries."
The 1931 paper of Carmichael is now available online from the publisher for $10.
Comments Off on Tuesday May 19, 2009
Sunday, May 17, 2009
Sunday May 17, 2009
Design Theory
Laura A. Smit, Calvin College, "Towards an Aesthetic Teleology: Romantic Love, Imagination and the Beautiful in the Thought of Simone Weil and Charles Williams"–
"My work is motivated by a hope that there may be a way to recapture the ancient and medieval vision of both Beauty and purpose in a way which is relevant to our own century. I even dare to hope that the two ideas may be related, that Beauty is actually part of the meaning and purpose of life."
Hans Ludwig de Vries, "On Orthogonal Resolutions of the Classical Steiner Quadruple System SQS(16)," Designs, Codes and Cryptography Vol. 48, No. 3 (Sept. 2008) 287-292 (DOI 10.1007/s10623-008-9207-5)–
"The Reverend T. P. Kirkman knew in 1862 that there exists a group of degree 16 and order 322560 with a normal, elementary abelian, subgroup of order 16 [1, p. 108]. Frobenius identified this group in 1904 as a subgroup of the Mathieu group M24 [4, p. 570]…."
1. Biggs N.L., "T. P. Kirkman, Mathematician," Bulletin of the London Mathematical Society 13, 97–120 (1981).
4. Frobenius G., "Über die Charaktere der mehrfach transitiven Gruppen," Sitzungsber. Königl. Preuss. Akad. Wiss. zu Berlin, 558–571 (1904). Reprinted in Frobenius, Gesammelte Abhandlungen III (J.-P. Serre, editor), pp. 335–348. Springer, Berlin (1968).
Olli Pottonen, "Classification of Steiner Quadruple Systems" (Master's thesis, Helsinki, 2005)–
"The concept of group actions is very useful in the study of isomorphisms of combinatorial structures."
"Simplify, simplify."
— Thoreau
"Beauty is bound up
with symmetry."
— Weyl
Pottonen's thesis is
dated Nov. 16, 2005.
For some remarks on
images and theology,
see Log24 on that date.
Click on the above image
for some further details.
Comments Off on Sunday May 17, 2009
Monday, February 23, 2009
Monday February 23, 2009
Another Manic Monday—
McGee and Smee
McGee and Smee
| Project MUSE — … and interpretations, “any of the Zingari shoolerim [gypsy schoolchildren] may pick a peck of kindlings yet from the sack of auld hensyne” (FW 112.4-8). … — Patrick McGee, “Reading Authority: |
“The ulterior motive behind this essay [“Reading Authority,” above], the purpose for which I seize this occasion, concerns the question or problem of authority. I stress at the outset my understanding of authority as the constructed repository of value or foundation of a system of values, the final effect of fetishism– in this case, literary fetishism. [Cf. Marx, Das Kapital] Reading– as in the phrase ‘reading authority’– should be grasped as the institutionally determined act of constructing authority….”
“[In Peter Pan] Smee is Captain Hook’s right-hand man… Barrie describes him as ‘Irish’ and ‘a man who stabbed without offence‘….”
Background: In yesterday’s morning entry, James Joyce as Jesuit, with “Dagger Definitions.”
A different Smee appears as an art critic in yesterday’s afternoon entry “Design Theory.”–
“Brock, who has a brisk mind, is a man on a mission. He read mathematical economics and political philosophy at Princeton (he has five degrees in all) and is the founder and president of Strategic Economic Decisions Inc., a think tank specializing in applying the economics of uncertainty to forecasting and risk assessment.
But phooey to all that; Brock has deeper things to think about. He believes he has cracked the secret of beautiful design. He even has equations and graphs to prove it.”
A Jesuit in Portrait of the Artist as a Young Man:
“When may we expect to have something from you on the esthetic question?”
“Our entanglement in the wilderness of Finnegans Wake is exemplified by the neologism ‘Bethicket.’ This word condenses a range of possible meanings and reinforces a diversity of possible syntactic interpretations. Joyce seems to allude to Beckett, creating a portmanteau word that melds ‘Beckett’ with ‘thicket’ (continuing the undergrowth metaphor), ‘thick’ (adding mental density to floral density)…. As a single word ‘Bethicket’ contains the confusion that its context suggests. On the one hand, ‘Bethicket me for a stump of a beech’ has the sound of a proverbial expletive that might mean something like ‘I’ll be damned’ or ‘Well, I’ll be a son of a gun.’….”
At the Oscars, 2009
Related material:Frame Tales and Dickung
Comments Off on Monday February 23, 2009
Thursday, March 6, 2008
Thursday March 6, 2008
This note is prompted by the March 4 death of Richard D. Anderson, writer on geometry, President (1981-82) of the Mathematical Association of America (MAA), and member of the MAA's Icosahedron Society.
Royal Road
"The historical road
from the Platonic solids
to the finite simple groups
is well known."
— Steven H. Cullinane,
November 2000,
Symmetry from Plato to
the Four-Color Conjecture
Euclid is said to have remarked that "there is no royal road to geometry." The road to the end of the four-color conjecture may, however, be viewed as a royal road from geometry to the wasteland of mathematical recreations.* (See, for instance, Ch. VIII, "Map-Colouring Problems," in Mathematical Recreations and Essays, by W. W. Rouse Ball and H. S. M. Coxeter.) That road ended in 1976 at the AMS-MAA summer meeting in Toronto– home of H. S. M. Coxeter, a.k.a. "the king of geometry."
A different road– from Plato to the finite simple groups– is, as I noted in November 2000, well known. But new roadside attractions continue to appear. One such attraction is the role played by a Platonic solid– the icosahedron– in design theory, coding theory, and the construction of the sporadic simple group M24.
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," by Andries E. Brouwer (Ch. 14 (pp. 693-746) of Handbook of Combinatorics, Vol. I, MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel, and László Lovász, Section 16 (p. 716))
This Steiner system is closely connected to M24 and to the extended binary Golay code. Brouwer gives an elegant construction of that code (and therefore of M24):
"Let N be the adjacency matrix of the icosahedron (points: 12 vertices, adjacent: joined by an edge). Then the rows of the 12×24 matrix
— Op. cit., p. 719
Related material:
* For a road out of this wasteland, back to geometry, see The Kaleidoscope Puzzle and Reflection Groups in Finite Geometry.
Finite Geometry of
the Square and Cube
and
Jewel in the Crown
"There is a pleasantly discursive
treatment of Pontius Pilate's
unanswered question
'What is truth?'"
— H. S. M. Coxeter, 1987,
introduction to Trudeau's
"story theory" of truth
Those who prefer stories to truth
may consult the Log24 entries
of March 1, 2, 3, 4, and 5.
They may also consult
the poet Rubén Darío:
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
* For a road out of this wasteland, back to geometry, see The Kaleidoscope Puzzle and Reflection Groups in Finite Geometry.
Comments Off on Thursday March 6, 2008
Tuesday, October 3, 2006
Tuesday October 3, 2006
Serious
"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."
— Charles Matthews at Wikipedia, Oct. 2, 2006
"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."
— G. H. Hardy, A Mathematician's Apology
Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:
Affine group
Reflection group
Symmetry in mathematics
Incidence structure
Invariant (mathematics)
Symmetry
Finite geometry
Group action
History of geometry
This would appear to be a fairly large complex of mathematical ideas.
See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:
Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.
Comments Off on Tuesday October 3, 2006
Monday, January 9, 2006
Monday January 9, 2006
Cornerstone
“In 1782, the Swiss mathematician Leonhard Euler posed a problem whose mathematical content at the time seemed about as much as that of a parlor puzzle. 178 years passed before a complete solution was found; not only did it inspire a wealth of mathematics, it is now a cornerstone of modern design theory.”
— Dean G. Hoffman, Auburn U.,
July 2001 Rutgers talk
Diagrams from Dieter Betten’s 1983 proof
of the nonexistence of two orthogonal
6×6 Latin squares (i.e., a proof
of Tarry’s 1900 theorem solving
Euler’s 1782 problem of the 36 officers):
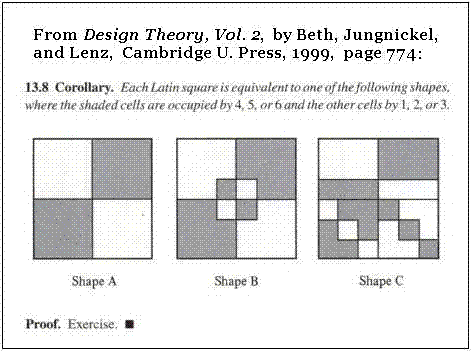
Compare with the partitions into
two 8-sets of the 4×4 Latin squares
discussed in my 1978 note (pdf).
Comments Off on Monday January 9, 2006
Saturday, August 31, 2002
Saturday August 31, 2002
Today’s birthday: Dr. Maria Montessori
THE MONTESSORI METHOD: CHAPTER VI
HOW LESSONS SHOULD BE GIVEN
“Let all thy words be counted.”
Dante, Inf., canto X.
CONCISENESS, SIMPLICITY, OBJECTIVITY.
…Dante gives excellent advice to teachers when he says, “Let thy words be counted.” The more carefully we cut away useless words, the more perfect will become the lesson….
Another characteristic quality of the lesson… is its simplicity. It must be stripped of all that is not absolute truth…. The carefully chosen words must be the most simple it is possible to find, and must refer to the truth.
The third quality of the lesson is its objectivity. The lesson must be presented in such a way that the personality of the teacher shall disappear. There shall remain in evidence only the object to which she wishes to call the attention of the child….
 |
 |
Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale “block design” subtest.
Mathematicians mean something different by the phrase “block design.”
A University of London site on mathematical design theory includes a link to my diamond theory site, which discusses the mathematics of the sorts of visual designs that Professor Pope is demonstrating. For an introduction to the subject that is, I hope, concise, simple, and objective, see my diamond 16 puzzle.
Comments Off on Saturday August 31, 2002