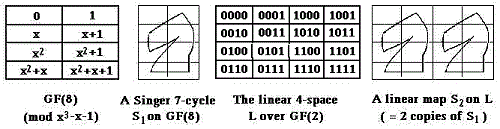
The Eight

Other knight figures:
Click on the SpringerLink
knight for a free copy
(pdf, 1.2 mb) of
the following paper
dealing with the geometry
underlying the R.T. Curtis
knight figures above:

Context:
Literature and Chess and
Sporadic Group References
Details:
| Adapted (for HTML) from the opening paragraphs of the above paper, W. Jonsson's 1970 "On the Mathieu Groups M22, M23, M24…"–
"[A]… uniqueness proof is offered here based upon a detailed knowledge of the geometric aspects of the elementary abelian group of order 16 together with a knowledge of the geometries associated with certain subgroups of its automorphism group. This construction was motivated by a question posed by D.R. Hughes and by the discussion Edge [5] (see also Conwell [4]) gives of certain isomorphisms between classical groups, namely
where A8 is the alternating group on eight symbols, S6 the symmetric group on six symbols, Sp(4,2) and PSp(4,2) the symplectic and projective symplectic groups in four variables over the field GF(2) of two elements, [and] PGL, PSL and SL are the projective linear, projective special linear and special linear groups (see for example [7], Kapitel II). The symplectic group PSp(4,2) is the group of collineations of the three dimensional projective space PG(3,2) over GF(2) which commute with a fixed null polarity tau…." References 4. Conwell, George M.: The three space PG(3,2) and its group. Ann. of Math. (2) 11, 60-76 (1910). 5. Edge, W.L.: The geometry of the linear fractional group LF(4,2). Proc. London Math. Soc. (3) 4, 317-342 (1954). 7. Huppert, B.: Endliche Gruppen I. Berlin-Heidelberg-New York: Springer 1967. |