Symmetry and Change
in the Dreamtime
Notes from the Journal
of Steven H. Cullinane
Summary:
Symmetry and Change, Part 1…
Early Evening,
Shining Star
7:31:01 PM ET
Hexagram 01
The Creative:

Heaven
Heaven
The movement of heaven
is full of power.

Click on picture
for details.
The Clare Lawler Prize
for Literature goes to…
Wednesday, September 1, 2004
Symmetry and Change, Part 2…
Words and Images
9:00:35 AM ET
Hexagram 35
Progress:

The Image
Fire
Earth
The sun rises over the earth.
|
From Aug. 18, 2004:
“Oh, my Lolita. I have only words
to play with!” (Nabokov, Lolita)
“This is the best toy train set
a boy ever had!”
(Orson Welles, after first touring
RKO Studios, quoted in Halliwell)
“As the quotes above by Nabokov and Welles suggest, we need to be able to account for the specific functions available to narrative in each medium, for the specific elements that empirical creators will ‘play with’ in crafting their narratives.”
— Donald F. Larsson
|
For
James Whale
and
William French Anderson —
Words
In the Spirit of
Dave Barry’s Book of Bad Songs:

Stay for just a while…
Stay, and let me look at you.
It’s been so long, I hardly knew you.
Standing in the door…
Stay with me a while.
I only want to talk to you.
We’ve traveled halfway ’round the world
To find ourselves again.
September morn…
We danced until the night
became a brand new day,
Two lovers playing scenes
from some romantic play.
September morning still can
make me feel this way.
Look at what you’ve done…
Why, you’ve become a grown-up girl…
— Neil Diamond
Images
In the Spirit of
September Morn:

The Last Day of Summer:
Photographs by Jock Sturges
“In 1990, the FBI entered Sturges’s studio and seized his work, claiming violation of child pornography laws.”
Related material:
Bill’s Diamond Theory

and

Log24 entries of
Aug. 15, 2004.
Those interested in the political implications of Diamond’s songs may enjoy Neil Performs at Kerry Fundraiser.
I personally enjoyed this site’s description of Billy Crystal’s remarks, which included “a joke about former President Clinton’s forthcoming children’s book — ‘It’s called The Little Engine That Could Because It Could.'”
“Puff, puff, woo, woo, off we go!”
Symmetry and Change, Part 3…
Whale Rider
12:07:28 PM
Hexagram 28
Preponderance of
the Great:

The Image
Lake
Wind
The lake rises
above the trees.

Cullinane College News:
“Congratulations to Clare Lawler, who participated very successfully in the recently held Secondary Schools Judo Championships in Wellington.”
For an explanation of this entry’s title, see the previous two entries and
Oxford Word
(Log24, July 10, 2004)
Thursday, September 2, 2004
Symmetry and Change, Part 4…
Heaven and Earth
11:11:42 AM ET
Hexagram 42
Increase:

The Image
Wind
Thunder
Wind and thunder:
the image of Increase.
“This time resembles that of
the marriage of heaven and earth”
|

Kylie
|

Finney
|
Well if you want to ride
you gotta ride it like you find it.
Get your ticket at the station
of the Rock Island Line. — Lonnie Donegan (d. Nov. 3) and others  The Rock Island Line’s namesake depot
The Rock Island Line’s namesake depot
in Rock Island, Illinois
“What it all boiled down to really was everybody giving everybody else a hard time for no good reason whatever… You just couldn’t march to your own music. Nowadays, you couldn’t even hear it… It was lost, the music which each person had inside himself, and which put him in step with things as they should be.”
— The Grifters, Ch. 10, 1963, by
James Myers Thompson

“The Old Man’s still an artist
with a Thompson.”
— Terry in “Miller’s Crossing”
For some of “the music which
each person had inside,”
click on the picture
with the Thompson.
It may be that Kylie is,
in her own way, an artist…
with a 357:

(Hits counter at
The Quality of Diamond
as of 11:05 AM Sept. 2, 2004)
For more on
“the marriage of heaven and earth,”
see
Plato, Pegasus, and the Evening Star.
Thursday, September 2, 2004
Symmetry and Change, Part 5…
Whale Road
7:00:23 PM
Hexagram 23
Splitting Apart:

The Image
Mountain
Earth
The mountain rests
on the earth.
“… the plot is different but the monsters, names, and manner of speaking will ring a bell.”
— Frank Pinto, Jr., review of Seamus Heaney’s translation of Beowulf
Other recommended reading, found during a search for the implications of today’s previous entry, “Hexagram 42”:
Water Wings.
This excellent meditation
on symmetry and change
comes from a site whose
home page
has the following image:

Friday, September 3, 2004
Symmetry and Change, Part 6…
Cinderella’s Slipper
12:00:54 AM ET
Hexagram 54
The Marrying Maiden:

The Image
Thunder
Lake
See
Friday, September 3, 2004
Symmetry and Change, Part 7…
Another September Morn
10:01:56 AM ET
Hexagram 56:
The Wanderer

The Image
Fire
Mountain
Fire on the mountain,
Run boys run…
Devil’s in the House of
The Rising Sun!
Friday, September 3, 2004
Symmetry and Change, Part 8…
Noon
12:00:25 PM ET
Hexagram 25
Innocence:

The Image
Heaven
Thunder
Under heaven
thunder rolls.
Friday, September 3, 2004
Symmetry and Change, Part 9…
De Nada

Helen Lane
1:13:49 PM ET
Hexagram 49
Revolution:

The Image
Lake
Fire Fire in the lake:
the image of Revolution.
“I sit now in a little room off the bar at four-thirty in the morning drinking ochas and then mescal and writing this on some Bella Vista notepaper I filched the other night…. But this is worst of all, to feel your soul dying. I wonder if it is because to-night my soul has really died that I feel at the moment something like peace. Or is it because right through hell there is a path, as Blake well knew, and though I may not take it, sometimes lately in dreams I have been able to see it? …And this is how I sometimes think of myself, as a great explorer who has discovered some extraordinary land from which he can never return to give his knowledge to the world: but the name of this land is hell. It is not Mexico of course but in the heart.”
— Malcolm Lowry, Under the Volcano
Friday, September 3, 2004
Symmetry and Change, conclusion…
Ite, Missa Est
3:17:13 PM ET
Hexagram 13
Fellowship With Men:

The Image
Heaven
Fire
Heaven together with fire.
“A pretty girl —
is like a melody —- !”
For details, see
A Mass for Lucero.















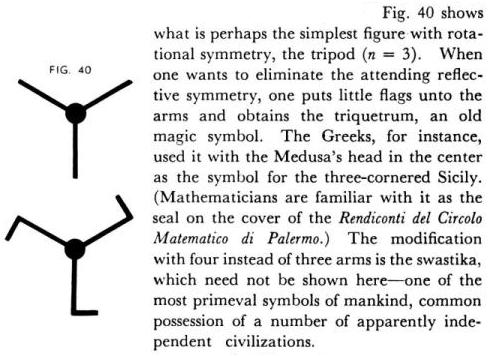





 .
. .
. .
.
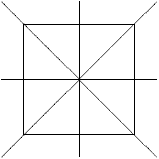













 The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…."
The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…."













































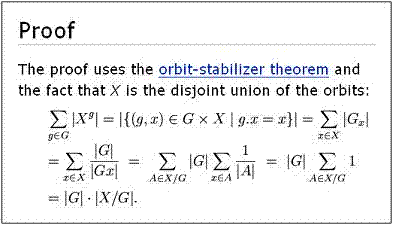
 “Imaginary time is a relatively simple concept that is rather difficult to visualize or conceptualize. In essence, it is another direction of time moving at right angles to ordinary time. In the image at right, the light gray lines represent ordinary time flowing from left to right – past to future. The dark gray lines depict imaginary time, moving at right angles to ordinary time.”
“Imaginary time is a relatively simple concept that is rather difficult to visualize or conceptualize. In essence, it is another direction of time moving at right angles to ordinary time. In the image at right, the light gray lines represent ordinary time flowing from left to right – past to future. The dark gray lines depict imaginary time, moving at right angles to ordinary time.”



























