See as well Buranyi in the previous post and Pergamon in this journal.
Wednesday, June 29, 2022
Sunday, August 18, 2019
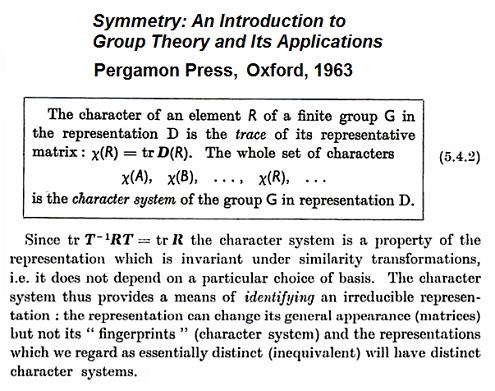
Structure at Pergamon
Some background for The Epstein Chronicles —
|
“What modern painters — James J. Gibson, Leonardo, |
See also Robert Maxwell,
Frank Oppenheimer,
and the history of Leonardo .
Click the above Pergamon Press image
for Pergamon-related material.
Tuesday, September 7, 2021
Raiders of the Lost Symbol … Continues*
A Log24 search for "Watercourse" leads to . . .
("Watercourse" is in the Customer review link.)
The "five years ago" link leads to . . .
|
"What modern painters are trying to do,
— James J. Gibson in Leonardo An example of invariant structure:
The three line diagrams above result from the three partitions, into pairs of 2-element sets, of the 4-element set from which the entries of the bottom colored figure are drawn. Taken as a set, these three line diagrams describe the structure of the bottom colored figure. After coordinatizing the figure in a suitable manner, we find that this set of three line diagrams is invariant under the group of 16 binary translations acting on the colored figure. A more remarkable invariance — that of symmetry itself — is observed if we arbitrarily and repeatedly permute rows and/or columns and/or 2×2 quadrants of the colored figure above. Each resulting figure has some ordinary or color-interchange symmetry. This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms." |
* See that title in this journal.
Friday, September 27, 2019
The Black List
"… Max Black, the Cornell philosopher, and others have pointed out
how 'perhaps every science must start with metaphor and end with
algebra, and perhaps without the metaphor there would never have
been any algebra' …."
— Max Black, Models and Metaphors, Cornell U. Press, 1962,
page 242, as quoted in Dramas, Fields, and Metaphors, by
Victor Witter Turner, Cornell U. Press, paperback, 1975, page 25
Metaphor —
Algebra —
|
The 16 Dirac matrices form six anticommuting sets of five matrices each (Arfken 1985, p. 214):
1.
2.
3.
4.
5.
6. SEE ALSO: Pauli Matrices REFERENCES: Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 211-217, 1985. Berestetskii, V. B.; Lifshitz, E. M.; and Pitaevskii, L. P. "Algebra of Dirac Matrices." §22 in Quantum Electrodynamics, 2nd ed. Oxford, England: Pergamon Press, pp. 80-84, 1982. Bethe, H. A. and Salpeter, E. Quantum Mechanics of One- and Two-Electron Atoms. New York: Plenum, pp. 47-48, 1977. Bjorken, J. D. and Drell, S. D. Relativistic Quantum Mechanics. New York: McGraw-Hill, 1964. Dirac, P. A. M. Principles of Quantum Mechanics, 4th ed. Oxford, England: Oxford University Press, 1982. Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, p. 580, 1980. Good, R. H. Jr. "Properties of Dirac Matrices." Rev. Mod. Phys. 27, 187-211, 1955. Referenced on Wolfram|Alpha: Dirac Matrices CITE THIS AS: Weisstein, Eric W. "Dirac Matrices."
From MathWorld— A Wolfram Web Resource. |
Desiring the exhilarations of changes:
The motive for metaphor, shrinking from
The weight of primary noon,
The A B C of being,
The ruddy temper, the hammer
Of red and blue, the hard sound—
Steel against intimation—the sharp flash,
The vital, arrogant, fatal, dominant X.
Monday, August 19, 2019
Gods and Giants
Wednesday, March 8, 2017
Inscapes
"The particulars of attention,
whether subjective or objective,
are unshackled through form,
and offered as a relational matrix …."
— Kent Johnson in a 1993 essay
|
The 16 Dirac matrices form six anticommuting sets of five matrices each (Arfken 1985, p. 214):
1.
2.
3.
4.
5.
6. SEE ALSO: Pauli Matrices REFERENCES: Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 211-217, 1985. Berestetskii, V. B.; Lifshitz, E. M.; and Pitaevskii, L. P. "Algebra of Dirac Matrices." §22 in Quantum Electrodynamics, 2nd ed. Oxford, England: Pergamon Press, pp. 80-84, 1982. Bethe, H. A. and Salpeter, E. Quantum Mechanics of One- and Two-Electron Atoms. New York: Plenum, pp. 47-48, 1977. Bjorken, J. D. and Drell, S. D. Relativistic Quantum Mechanics. New York: McGraw-Hill, 1964. Dirac, P. A. M. Principles of Quantum Mechanics, 4th ed. Oxford, England: Oxford University Press, 1982. Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, p. 580, 1980. Good, R. H. Jr. "Properties of Dirac Matrices." Rev. Mod. Phys. 27, 187-211, 1955. Referenced on Wolfram|Alpha: Dirac Matrices CITE THIS AS: Weisstein, Eric W. "Dirac Matrices."
From MathWorld— A Wolfram Web Resource. |
Thursday, July 22, 2010
By Chance
PA Lottery 7/21— Midday 312, Evening 357.
Related material:
and a .357—

Related philosophy—
"Character is fate." — Heraclitus
"Pray for the grace of accuracy." — Robert Lowell
Oh, and a belated happy 7/21 birthday to Ernest Hemingway and Robin Williams.
Friday, March 12, 2010
Group Characters
“… this gentle little movie… is, after all, a character study– and in an alcoholic country singer named Bad Blake, we’ve got one hell of a character.”
And then there’s Baaad Blake–
Related material:
This journal on the president of
London’s Blake Society and
Wikipedia on the founder of
Pergamon Press
Thursday, August 12, 2004
Thursday August 12, 2004
Battle of Gods and Giants,
Part III:
The Invisible Made Visible
"Leon Golub, an American painter of expressionistic, heroic-scale figures that reflect dire modern political conditions, died on Sunday in Manhattan. He was 82….
In the 1960's he produced a series, called 'Gigantomachies,' of battling, wrestling figures. They were based on classical models, including the Hellenistic Altar of Pergamon. But there was nothing idealized about them."
The Hellenistic Altar of Pergamon,
from Battle of Gods and Giants:
Golub's New York Times obituary concludes with a quote from a 1991 interview:
"Asked about his continuing and future goal he said, 'To head into real!'"
From Tuesday's Battle of Gods and Giants:
This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms."
Perhaps, if Golub is fortunate enough to escape from the afterlife version of Plato's Cave, he will also be fortunate enough to enter Purgatory, where there awaits a course in reality, in the form of…
Tuesday, August 10, 2004
Tuesday August 10, 2004
Battle of Gods and Giants
In checking the quotations from Dante in the previous entry, I came across the intriguing site Gigantomachia:
"A gigantomachia or primordial battle between the gods has been retold in myth, cult, art and theory for thousands of years, from the Egyptians to Heidegger. This site will present the history of the theme. But it will do so in an attempt to raise the question of the contemporary relevance of it. Does the gigantomachia take place today? Where? When? In what relation to you and me?"
Perhaps atop the Empire State Building?
(See An Affair to Remember and Empire State Building to Honor Fay Wray.)
Perhaps in relation to what the late poet Donald Justice called "the wood within"?
Perhaps in relation to T. S. Eliot's "The Waste Land" and the Feast of the Metamorphosis?
Or perhaps not.
Perhaps at Pergamon:
Perhaps at Pergamon Press:
"What modern painters are trying to do,
if they only knew it, is paint invariants."
— James J. Gibson in Leonardo
(Vol. 11, pp. 227-235.
Pergamon Press Ltd., 1978)
An example of invariant structure:

The three line diagrams above result from the three partitions, into pairs of 2-element sets, of the 4-element set from which the entries of the bottom colored figure are drawn. Taken as a set, these three line diagrams describe the structure of the bottom colored figure. After coordinatizing the figure in a suitable manner, we find that this set of three line diagrams is invariant under the group of 16 binary translations acting on the colored figure.
A more remarkable invariance — that of symmetry
This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms."
For further details, see a section on Plato in the Gigantomachia site.
Monday, April 5, 2004
Monday April 5, 2004
Ideas and Art

— Motto of
Plato's Academy
|
From Minimalist Fantasies,
All I want anyone to get out of my paintings, and all I ever get out of them, is the fact that you can see the whole idea without any confusion. … What you see is what you see.
Minimal Art remains too much a feat of ideation, and not enough anything else. Its idea remains an idea, something deduced instead of felt and discovered.
The artists even questioned whether art needed to be a tangible object. Minimalism … Conceptualism — suddenly art could be nothing more than an idea, a thought on a piece of paper….
There was a period, a decade or two ago, when you could hardly open an art journal without encountering the quotation from Frank Stella I used as an epigraph. The bit about “what you see is what you see” was reproduced ad nauseam. It was thought by some to be very deep. In fact, Stella’s remarks—from a joint interview with him and Donald Judd—serve chiefly to underscore the artistic emptiness of the whole project of minimalism. No one can argue with the proposition that “what you see is what you see,” but there’s a lot to argue with in what he calls “the fact that you can see the whole idea without any confusion.” We do not, of course, see ideas. Stella’s assertion to the contrary might be an instance of verbal carelessness, but it is not merely verbal carelessness. At the center of minimalism, as Clement Greenberg noted, is the triumph of ideation over feeling and perception, over aesthetics. |
|
From How Not Much Is a Whole World, Decades on, it's curious how much Minimalism, the last great high modern movement, still troubles people who just can't see why … a plain white canvas with a line painted across it
should be considered art. That line might as well be in the sand: on this side is art, it implies. Go ahead. Cross it. …. The tug of an art that unapologetically sees itself as on a par with science and religion is not to be underestimated, either. Philosophical ambition and formal modesty still constitute Minimalism's bottom line. If what results can sometimes be more fodder for the brain than exciting to look at, it can also have a serene and exalted eloquence…. That line in the sand doesn't separate good art from bad, or art from nonart, but a wide world from an even wider one. |
I maintain that of course
we can see ideas.
Example: the idea of
invariant structure.

"What modern painters
are trying to do,
if they only knew it,
is paint invariants."
— James J. Gibson, Leonardo,
Vol. 11, pp. 227-235.
Pergamon Press Ltd., 1978
For a discussion
of how this works, see
Block Designs,
4×4 Geometry, and
Diamond Theory.
Incidentally, structures like the one shown above are invariant under an important subgroup of the affine group AGL(4,2)… That is to say, they are not lost in translation. (See previous entry.)
Sunday, February 22, 2004
Sunday February 22, 2004
"What modern painters are trying to do,
if they only knew it, is paint invariants."
— James J. Gibson in Leonardo

(Vol. 11, pp. 227-235.
Pergamon Press Ltd., 1978)
Those who have clicked
on the title above
may find the following of interest.
|
Sean Socha
Imagination/Reality: I see modern art's usefulness for Stevens in its reconfiguration of the relationship between imagination and reality…. Stevens will incorporate a device from painting to illustrate his poetic idea. For instance, "Metaphors of a Magnifico" (Harmonium) illustrates an idea about the fragmentation and/or subjectivity of reality and the importance of perspective by incorporating the Cubist technique of multiple perspectives. |
Also perhaps relevant:
|
Einstein wanted to know what was invariant (the same) for all observers. The original title for his theory was (translated from German) "Theory of Invariants." — Wikipedia |