From posts tagged Schoolgirl Space —
Thursday, May 16, 2024
Para Los Muertos: Thoroughly Modern Schoolgirl Space
Comments Off on Para Los Muertos: Thoroughly Modern Schoolgirl Space
Thursday, January 23, 2020
Exploring Schoolgirl Space…
"Old men ought to be explorers." — T. S. Eliot.
Rose the Hat in her younger days.
See as well Barsotti in this journal.
Comments Off on Exploring Schoolgirl Space…
Saturday, September 21, 2019
Exploring Egypt: The Sequel to Exploring Schoolgirl Space*
Comments Off on Exploring Egypt: The Sequel to Exploring Schoolgirl Space*
Friday, September 13, 2019
Schoolgirl Space…
Comments Off on Schoolgirl Space…
Thursday, August 15, 2019
Schoolgirl Space — Tetrahedron or Square?
The exercise in the previous post was suggested by a passage
purporting to "use standard block design theory" that was written
by some anonymous author at Wikipedia on March 1, 2019:
Here "rm OR" apparently means "remove original research."
Before the March 1 revision . . .
The "original research" objected to and removed was the paragraph
beginning "To explain this further." That paragraph was put into the
article earlier on Feb. 28 by yet another anonymous author (not by me).
An account of my own (1976 and later) original research on this subject
is pictured below, in a note from Feb. 20, 1986 —
Comments Off on Schoolgirl Space — Tetrahedron or Square?
Saturday, August 10, 2019
Schoolgirl Space* Revisited:
The Square "Inscape" Model of
the Generalized Quadrangle W(2)
Click image to enlarge.
-embedding-in-PG(3,2)-Planat-Saniga-1000w.jpg)
* The title refers to the role of PG (3,2) in Kirkman's schoolgirl problem.
For some backstory, see my post Anticommuting Dirac Matrices as Skew Lines
and, more generally, posts tagged Dirac and Geometry.
Comments Off on Schoolgirl Space* Revisited:
Tuesday, July 16, 2019
Schoolgirl Space for Quantum Mystics
Comments Off on Schoolgirl Space for Quantum Mystics
Monday, July 15, 2019
Arche’s Schoolgirl Space
From Dan Brown's novel Angels & Demons (2000)—
Comments Off on Arche’s Schoolgirl Space
Tuesday, July 9, 2019
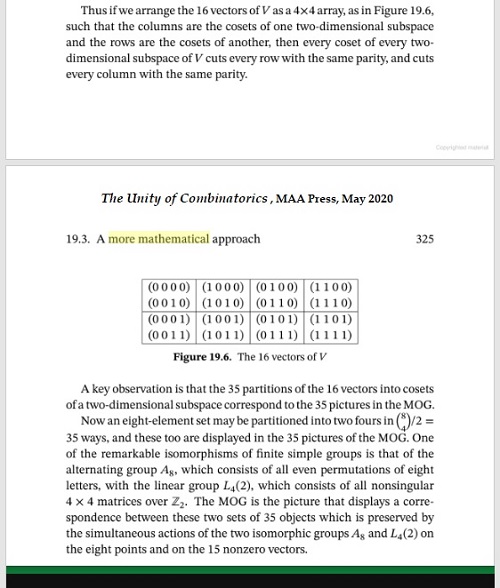
Schoolgirl Space: 1984 Revisited
Cube Bricks 1984 —
From "Tomorrowland" (2015) —
From John Baez (2018) —
See also this morning's post Perception of Space
and yesterday's Exploring Schoolgirl Space.
Comments Off on Schoolgirl Space: 1984 Revisited
Monday, July 8, 2019
Exploring Schoolgirl Space
See also "Quantum Tesseract Theorem" and "The Crosswicks Curse."
Comments Off on Exploring Schoolgirl Space
Thursday, May 19, 2022
Saturday, February 17, 2024
Sixteen Finite-Space Topologies
From Wikipedia, sixteen T0 topologies on a four-set —
{∅, {a}, {a, b}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {a, b}, {a, c}, {a, b, c}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {a, c}, {a, b, c}, {a, b, c, d}} (T0)
{∅, {a}, {a, b}, {a, b, c}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {a, b, c}, {a, b, c, d}} (T0)
{∅, {a}, {a, b}, {c}, {a, c}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {a, b}, {a, c}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {a, c}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {b, c}, {a, b, c}, {a, d}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {a, b}, {a, c}, {a, b, c}, {a, d}, {a, b, d}, {a, c, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {a, c}, {a, b, c}, {a, d}, {a, b, d}, {a, c, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {c}, {a, c}, {b, c}, {a, b, c}, {a, b, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {c}, {a, c}, {b, c}, {a, b, c}, {a, d}, {a, b, d}, {a, c, d}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {c}, {a, c}, {b, c}, {a, b, c}, {a, b, c, d}} (T0)
{∅, {a}, {b}, {a, b}, {c}, {a, c}, {b, c}, {a, b, c}, {d}, {a, d}, {b, d}, {a, b, d}, {c, d}, {a, c, d}, {b, c, d}, {a, b, c, d}} (T0)
Compare and contrast with the finite geometry of the power set of a four-set.
Comments Off on Sixteen Finite-Space Topologies
Friday, February 11, 2022
For Space Groupies
A followup to Wednesday's post Deep Space —
Related material from this journal on July 9, 2019 —
Cube Bricks 1984 —
From "Tomorrowland" (2015) —
From other posts tagged 1984 Cubes —
Comments Off on For Space Groupies
Friday, September 27, 2019
Algebra for Schoolgirls
The 15 points of the finite projective 3-space PG(3,2)
arranged in tetrahedral form:
The letter labels, but not the tetrahedral form,
are from The Axioms of Projective Geometry , by
Alfred North Whitehead (Cambridge U. Press, 1906).
The above space PG(3,2), because of its close association with
Kirkman's schoolgirl problem, might be called "schoolgirl space."
Screen Rant on July 31, 2019:
A Google Search sidebar this morning:
Apocalypse Soon! —
Comments Off on Algebra for Schoolgirls
Wednesday, June 29, 2022
Mathematical Plays*
A song whose melody was used in
Westworld, Season 4, Episode 1 —
"Singin' in the old bars
Swingin' with the old stars
Livin' for the fame
Kissin' in the blue dark
Playin' pool and wild darts
Video games"
In memory of a video game executive
who reportedly died on June 22, 2022 —
* Adapted from a book title.
Comments Off on Mathematical Plays*
Tuesday, May 5, 2020
Monday, March 16, 2020
Mathematics and Narrative* Continues:
Mathematics: See Tetrahedron vs. Square in this journal
(Notes on two different models of schoolgirl space ).
Narrative: Replacing the square from the above posts by
a related cube …
… yields a merchandising inspiration —
Dueling Holocrons:
Jedi Cube vs. Sith Tetrahedron —
.* See also earlier posts on Mathematics and Narrative.
Comments Off on Mathematics and Narrative* Continues:
Wednesday, February 26, 2020
“Perfect”
Usage example —
(Click to enlarge.)
See also the previous post as well as PG(3,2),
Schoolgirl Space, and Tetrahedron vs. Square.
Comments Off on “Perfect”
Friday, November 22, 2019
The Virgin Field:
Representing Schoolgirl Space
From a book reviewed in the April 1923
Bulletin of the American Mathematical Society —
From a later book —
"Her wall is filled with pictures" — Chuck Berry
Comments Off on The Virgin Field:
Wednesday, September 18, 2019
Powers of X
Screen Rant on July 31, 2019 —
The above space PG(3,2), because of its close association with
Kirkman's schoolgirl problem, might be called "schoolgirl space."
See as well a Log24 post from the above Screen Rant date —
Comments Off on Powers of X
Monday, August 19, 2019
A Couple of Tots
The title is from the post "Child's Play" of May 21, 2012 . . .
"It seems that only one course is open to the philosopher
who values knowledge and truth above all else. He must
refuse to accept from the champions of the forms the
doctrine that all reality is changeless [and exclusively
immaterial], and he must turn a deaf ear to the other party
who represent reality as everywhere changing [and as only
material]. Like a child begging for 'both', he must declare
that reality or the sum of things is both at once [το όν τε και
το παν συναμφότερα] (Sophist 246a-249d)."
Related material —
"Schoolgirl Space: 1984 Revisited" (July 9, 2019) and
posts tagged Tetrahedron vs. Square.
Comments Off on A Couple of Tots
Friday, August 16, 2019
Nocciolo
A revision of the above diagram showing
the Galois-addition-table structure —
Related tables from August 10 —
See "Schoolgirl Space Revisited."
Comments Off on Nocciolo
Sunday, August 11, 2019
The Culture Desk
American Songwriters (the Petrusich versions)
The New Yorker Culture Desk online yesterday —
The New Yorker Culture Desk onine July 16 —
Related aesthetic meditation from this journal on July 16 —
(From the post "Schoolgirl Space for Quantum Mystics")
Comments Off on The Culture Desk
Wednesday, July 31, 2019
The Epstein Chronicles, or: Z is for Zorro
The geometry of the 15 point-pairs in the previous post suggests a review:
From "Exploring Schoolgirl Space," July 8 —
The date in the previous post — Oct. 9, 2018 — also suggests a review
of posts from that date now tagged Gen-Z:
Comments Off on The Epstein Chronicles, or: Z is for Zorro
Wednesday, July 10, 2019
Artifice* of Eternity …
… and Schoolgirl Space
"This poem contrasts the prosaic and sensual world of the here and now
with the transcendent and timeless world of beauty in art, and the first line,
'That is no country for old men,' refers to an artless world of impermanence
and sensual pleasure."
— "Yeats' 'Sailing to Byzantium' and McCarthy's No Country for Old Men :
Art and Artifice in the New Novel,"
Steven Frye in The Cormac McCarthy Journal ,
Vol. 5, No. 1 (Spring 2005), pp. 14-20.
See also Schoolgirl Space in this journal.
* See, for instance, Lewis Hyde on the word "artifice" and . . .
Comments Off on Artifice* of Eternity …
Sunday, February 18, 2024
A Scene from “Terminator Zero: Rise of the Chatbots” —
Deepfake Tango in Paris
Deepfake Image from the Internet:
Not Emily in Paris.
Related Deep Learning and Deepfake Learning:
The study of finite topological spaces may be regarded as a
deepfake approach to finite geometry. See the previous post.
Comments Off on A Scene from “Terminator Zero: Rise of the Chatbots” —
Deepfake Tango in Paris
Deepfake Tango in Paris
Monday, July 4, 2022
♫ I’ll be seeing you . . . ♫
Comments Off on ♫ I’ll be seeing you . . . ♫
Friday, May 27, 2022
Wednesday, August 26, 2020
Thursday, July 2, 2020
The Maxwell* Enticement
[Update of Sunday morning, July 12, 2020 —
This July 2 post was suggested in part by the July 1 post Magic Child
and in part by the Sept. 15, 1984, date in the image below. For more
details about that date, possibly the death date of author Richard
Brautigan, see "The Life and Death of Richard Brautigan," by
Lawrence Wright, in Rolling Stone on April 11, 1985.
From that article:
Marcia called him the next night [Sept. 15, 1984]
in Bolinas. He asked if she liked his mind. "I said,
‘Yes, Richard, I like your mind. You have the ability
to jump in and out of spaces. It’s not linear thinking;
it’s exciting, catalytic, random thinking.’ "
Such thinking, though interesting, is not recommended for the
general public. Sept. 15, 1984, was perhaps Brautigan's last day alive.]
* See Maxwell in posts tagged Gods and Giants.
Comments Off on The Maxwell* Enticement
Thursday, April 23, 2020
Octads and Geometry
See the web pages octad.group and octad.us.
Related geometry (not the 759 octads, but closely related to them) —
#/media/File:150614-PG-3-2-schoolgirls-arrangement.png)
The 4×6 rectangle of R. T. Curtis
illustrates the geometry of octads —
Curtis splits the 4×6 rectangle into three 4×2 "bricks" —
.
"In fact the construction enables us to describe the octads
in a very revealing manner. It shows that each octad,
other than Λ1, Λ2, Λ3, intersects at least one of these ' bricks' —
the 'heavy brick' – in just four points." . . . .
— R. T. Curtis (1976). "A new combinatorial approach to M24,"
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42.
Comments Off on Octads and Geometry
Sunday, March 1, 2020
Saturday, August 10, 2019
Wednesday, August 7, 2019
Arts: Boom-Booms Meet Pom-Poms —
Exploring Schoolgirl Space (July 8) continues.
"Eh Fatty Boom-Boom
Hit me with the Ching-Ching"
— Song lyric
Comments Off on Arts: Boom-Booms Meet Pom-Poms —
Wednesday, July 17, 2019
Life in Palermo
See also other posts tagged Tetrahedron vs. Square, and a related
Log24 search for "Schoolgirl + Space."
Comments Off on Life in Palermo
Wednesday, July 5, 2017
Imaginarium of a Different Kind
The title refers to that of the previous post, "The Imaginarium."
In memory of a translator who reportedly died on May 22, 2017,
a passage quoted here on that date —
Related material — A paragraph added on March 15, 2017,
to the Wikipedia article on Galois geometry —
|
George Conwell gave an early demonstration of Galois geometry in 1910 when he characterized a solution of Kirkman's schoolgirl problem as a partition of sets of skew lines in PG(3,2), the three-dimensional projective geometry over the Galois field GF(2).[3] Similar to methods of line geometry in space over a field of characteristic 0, Conwell used Plücker coordinates in PG(5,2) and identified the points representing lines in PG(3,2) as those on the Klein quadric. — User Rgdboer |
Comments Off on Imaginarium of a Different Kind
Friday, February 17, 2017
Heptads and Heptapods
In the recent science fiction film "Arrival," Amy Adams portrays
a linguist, Louise Banks, who must learn to translate the language of
aliens ("Heptapods") who have just arrived in their spaceships.
The point of this tale seems to have something to do with Banks
learning, along with the aliens' language, their skill of seeing into
the future.
Louise Banks wannabes might enjoy the works of one
Metod Saniga, who thinks that finite geometry might have
something to do with perceptions of time.
See Metod Saniga, “Algebraic Geometry: A Tool for Resolving
the Enigma of Time?”, in R. Buccheri, V. Di Gesù and M. Saniga (eds.),
Studies on the Structure of Time: From Physics to Psycho(patho)logy,
Kluwer Academic / Plenum Publishers, New York, 2000, pp. 137–166.
Available online at www.ta3.sk/~msaniga/pub/ftp/mathpsych.pdf .
Although I share an interest in finite geometry with Saniga —
see, for instance, his remarks on Conwell heptads in the previous post
and my own remarks in yesterday's post "Schoolgirls and Heptads" —
I do not endorse his temporal speculations.
Comments Off on Heptads and Heptapods
Wednesday, February 15, 2017
Warp and Woof
Space —
Space structure —
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Space shuttle —
Related ethnic remarks —
… As opposed to Michael Larsen —
Funny, you don't look Danish.
Comments Off on Warp and Woof
Monday, May 30, 2016
Perfect Universe
(A sequel to the previous post, Perfect Number)
Since antiquity, six has been known as
"the smallest perfect number." The word "perfect"
here means that a number is the sum of its
proper divisors — in the case of six: 1, 2, and 3.
The properties of a six-element set (a "6-set")
divided into three 2-sets and divided into two 3-sets
are those of what Burkard Polster, using the same
adjective in a different sense, has called
"the smallest perfect universe" — PG(3,2), the projective
3-dimensional space over the 2-element Galois field.
A Google search for the phrase "smallest perfect universe"
suggests a turnaround in meaning , if not in finance,
that might please Yahoo CEO Marissa Mayer on her birthday —
The semantic turnaround here in the meaning of "perfect"
is accompanied by a model turnaround in the picture of PG(3,2) as
Polster's tetrahedral model is replaced by Cullinane's square model.
Further background from the previous post —
See also Kirkman's Schoolgirl Problem.
Comments Off on Perfect Universe
Monday, December 21, 2015
The Eppstein Edit
A Wikipedia edit today by David Eppstein, a professor
at the University of California, Irvine:
See the Fano-plane page before and after the Eppstein edit.
Eppstein deleted my Dec. 6 Fano 3-space image as well as
today's Fano-plane image. He apparently failed to read the
explanatory notes for both the 3-space model and the
2-space model. The research he refers to was original
(in 1979) but has been published for some time now in the
online Encyclopedia of Mathematics, as he could have
discovered by following a link in the notes for the 3-space
model.
For a related recent display of ignorance, see Hint of Reality.
Happy darkest night.
Comments Off on The Eppstein Edit
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—
Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Comments Off on Vril Chick
Wednesday, March 11, 2009
Wednesday March 11, 2009
Sein Feld
in Translation
(continued from
May 15, 1998)
in Translation
(continued from
May 15, 1998)
The New York Times March 10–
"Paris | A Show About Nothing"–
The Times describes one of the empty rooms on exhibit as…
"… Yves Klein’s 'La spécialisation de la sensibilité à l’état matière première en sensibilité picturale stabilisée, Le Vide' ('The Specialization of Sensibility in the Raw Material State Into Stabilized Pictorial Sensibility, the Void')"
This is a mistranslation. See "An Aesthetics of Matter" (pdf), by Kiyohiko Kitamura and Tomoyuki Kitamura, pp. 85-101 in International Yearbook of Aesthetics, Volume 6, 2002—
"The exhibition «La spécialisation de la sensibilité à l’état matière-première en sensibilité picturale stabilisée», better known as «Le Vide» (The Void) was held at the Gallery Iris Clert in Paris from April 28th till May 5th, 1955." –p. 94
"… «Sensibility in the state of prime matter»… filled the emptiness." –p. 95
Kitamura and Kitamura translate matière première correctly as "prime matter" (the prima materia of the scholastic philosophers) rather than "raw material." (The phrase in French can mean either.)
Related material:
The Diamond Archetype and
The Illuminati Diamond.
The Diamond Archetype and
The Illuminati Diamond.
The link above to
prima materia
is to an 1876 review
by Cardinal Manning of
a work on philosophy
by T. P. Kirkman, whose
"schoolgirl problem" is
closely related to the
finite space of the
diamond theorem.
Comments Off on Wednesday March 11, 2009
Tuesday, October 3, 2006
Tuesday October 3, 2006
Serious
"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."
— Charles Matthews at Wikipedia, Oct. 2, 2006
"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."
— G. H. Hardy, A Mathematician's Apology
Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:
Affine group
Reflection group
Symmetry in mathematics
Incidence structure
Invariant (mathematics)
Symmetry
Finite geometry
Group action
History of geometry
This would appear to be a fairly large complex of mathematical ideas.
See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:
Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.
Comments Off on Tuesday October 3, 2006