"The four base units commute and satisfy
the multiplication table of the Klein 4 group."
— Bernd Schmeikal, article accepted
for publication on 11 April 2015
See also Log24 on 11 April 2015 (Orthodox Holy Saturday).
"The four base units commute and satisfy
the multiplication table of the Klein 4 group."
— Bernd Schmeikal, article accepted
for publication on 11 April 2015
See also Log24 on 11 April 2015 (Orthodox Holy Saturday).

For some background, see "four-group" in this journal.
The exercise posted here on Sept. 11, 2022, suggests a
more precisely stated problem . . .
The 24 coordinate-positions of the 4096 length-24 words of the
extended binary Golay code G24 can be arranged in a 4×6 array
in, of course, 24! ways.
Some of these ways are more geometrically natural than others.
See, for instance, the Miracle Octad Generator of R. T. Curtis.
What is the size of the largest subcode C of G24 that can be
arranged in a 4×6 array in such a way that the set of words of C
is invariant under the symmetry group of the rectangle itself, i.e. the
four-group of the identity along with horizontal and vertical reflections
and 180-degree rotation.
Recent Log24 posts tagged Bitspace describe the structure of
an 8-dimensional (256-word) code in a 4×6 array that has such
symmetry, but it is not yet clear whether that "cube-motif" code
is a Golay subcode. (Its octads are Golay, but possibly not all its
dodecads; the octads do not quite generate the entire code.)
Magma may have an answer, but I have had little experience in
its use.
* Footnote of 30 September 2022. The 4×6 problem is a
special case of a more general symmetric embedding problem.
Given a linear code C and a mapping of C to parts of a geometric
object A with symmetry group G, what is the largest subcode of C
invariant under G? What is the largest such subcode under all
such mappings from C to A?
Prominent in the oeuvre of art theorist Rosalind Krauss, the Klein group
is a four-element group named for Felix Christian Klein.
It is commonly known as the four-group.
Mathematicians sometimes call this group
"V," for its German name, Vierergruppe .
For those who prefer narrative to mathematics —

Those who want a serious approach to the mathematics
of Clifford algebras — via finite geometry, the natural setting
of the four-group of the previous post — should consult
"Finite Geometry, Dirac Groups and the Table of
Real Clifford Algebras," by Ron Shaw (1995).
From the Harvard Graduate School of Design's introduction
to a lecture on All Souls' Day 2015 —
"Calvin Klein is an award-winning fashion icon.
He is recognized globally as a master of minimalism
and has spent his career distilling things to
their very essence. His name ranks among the
best-known brands in the world, with Calvin Klein, Inc.
reaching over seven billion dollars in global retail sales."
A Klein icon I prefer —
Click the above image for some backstory.
Remarks by an ignorant professor quoted here
yesterday suggest a Log24 search for "Lost in Translation."
That search yields instances of the following figure:

See also the post Red October (Oct. 2, 2012).
The above is the result of a (fruitless) image search today for a current version of Giovanni Sambin's "Basic Picture: A Structure for Topology."
That search was suggested by the title of today's New York Times op-ed essay "Found in Translation" and an occurrence of that phrase in this journal on January 5, 2007.
Further information on one of the images above—
A search in this journal on the publication date of Giaquinto's Visual Thinking in Mathematics yields the following—
|
In defense of Plato’s realism (vs. sophists’ nominalism– see recent entries.) Plato cited geometry, notably in the Meno , in defense of his realism. |
For the Meno 's diamond figure in Giaquinto, see a review—

— Review by Jeremy Avigad (preprint)
Finite geometry supplies a rather different context for Plato's "basic picture."
In that context, the Klein four-group often cited by art theorist Rosalind Krauss appears as a group of translations in the mathematical sense. (See Kernel of Eternity and Sacerdotal Jargon at Harvard.)
The Times op-ed essay today notes that linguistic translation "… is not merely a job assigned to a translator expert in a foreign language, but a long, complex and even profound series of transformations that involve the writer and reader as well."
The list of four-group transformations in the mathematical sense is neither long nor complex, but is apparently profound enough to enjoy the close attention of thinkers like Krauss.
This 1495 image is found in
The Janus Faces of Genius:
The Role of Alchemy
in Newton's Thought,
by B. J. T. Dobbs,
Cambridge U. Press,
2002, p. 85
From
Kernel of Eternity:

From
Sacerdotal Jargon
at Harvard:

From "The Fifth Element"
(1997, Milla Jovovich
and Bruce Willis) —
The crossing of the beams:
Happy birthday, Bruce Willis.

The Square of Oppositon
at Stanford Encylopedia of Philosophy

The Square of Opposition
in its original form
"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."
— Edward Buckner at The Logic Museum
From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:

The Semiotic Square
"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."
* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter
Another version of the semiotic square:

Here is a more explicit figure representing the Klein group:
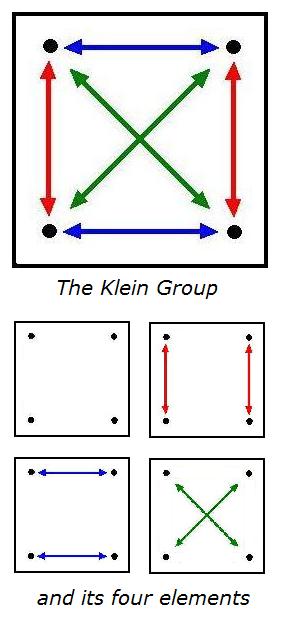
There is also the logical
diamond of opposition —

A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
a Marxist literary theorist:
|
"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–
The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.) Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1): 
As Jameson explains in the Foreword to Greimas' On Meaning, "-s1 and -s2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.
* Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.
|
— The Gameplayers of Zan, by M.A. Foster
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
Gravity's Rainbow
Crosses used by semioticians
to baffle their opponents
are illustrated above.
Some other kinds of crosses,
and another kind of opponent:
|
Monday, July 11, 2005
Logos
for St. Benedict's Day Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org. Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth. Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion. I personally prefer the "diamond theory" of truth, represented by the logo below.
See also the previous entry Sunday, July 10, 2005
Mathematics
and Narrative Click on the title for a narrative about
Nikolaos K. Artemiadis,

"First of all, I'd like to
— Remark attributed to Plato
|
||||||||

Related material:
Aspects of Symmetry,
from the day that
Scarlett Johansson
turned 23, and…
|
"…A foyer of the spirit in a landscape
In which we read the critique of paradise — "Crude Foyer," by Wallace Stevens |

In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
For radicals of another sort, see A Logocentric Meditation, A Mass for Lucero, and Steven Erlanger in The New York Times— "France Still Divided Over Lessons of 1968 Unrest."
The Klein Group as Kernel
of a Map from S4 to S3:
For those who prefer Galois's
politics to his mathematics,
there is
MAY 68: STREET POSTERS
FROM THE PARIS REBELLION
at London's Southbank Centre
(May 1 – June 1, 2008).

Thomas Wolfe
(Harvard M.A., 1922)
versus

Rosalind Krauss
(Harvard M.A., 1964,
Ph.D., 1969)
on
"No culture has a pact with eternity."
— George Steiner, interview in
The Guardian of
"At that instant he saw,
in one blaze of light, an image
of unutterable conviction….
the core of life, the essential
pattern whence all other things
proceed, the kernel of eternity."
— Thomas Wolfe, Of Time
and the River, quoted in
Log24 on June 9, 2005
From today's online Harvard Crimson:
"… under the leadership of Faust,
Harvard students should look forward
to an ever-growing opportunity for
international experience
and artistic endeavor."

Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen
From a recent book
on Wolfgang Pauli,
The Innermost Kernel:

A belated happy birthday
to the late
Felix Christian Klein
(born on April 25) —

Another Harvard figure quoted here on Dec. 5, 2002:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951)
From a review of Rosalind Krauss's The Optical Unconscious (MIT Press hardcover, 1993):
Krauss is concerned to present Modernism less in terms of its history than its structure, which she seeks to represent by means of a kind of diagram: "It is more interesting to think of modernism as a graph or table than a history." The "table" is a square with diagonally connected corners, of the kind most likely to be familiar to readers as the Square of Opposition, found in elementary logic texts since the mid-19th century. The square, as Krauss sees it, defines a kind of idealized space "within which to work out unbearable contradictions produced within the real field of history." This she calls, using the inevitable gallicism, "the site of Jameson's Political Unconscious" and then, in art, the optical unconscious, which consists of what Utopian Modernism had to kick downstairs, to repress, to "evacuate… from its field."
— Arthur C. Danto in ArtForum, Summer 1993
Rosalind Krauss in The Optical Unconscious (MIT Press paperback, 1994):
For a presentation of the Klein Group, see Marc Barbut, "On the Meaning of the Word 'Structure' in Mathematics," in Introduction to Structuralism, ed. Michael Lane (New York: Basic Books, 1970). Claude Lévi-Strauss uses the Klein group in his analysis of the relation between Kwakiutl and Salish masks in The Way of the Masks, trans. Sylvia Modelski (Seattle: University of Washington Press, 1982), p. 125; and in relation to the Oedipus myth in "The Structural Analysis of Myth," Structural Anthropology, trans. Claire Jackobson [sic] and Brooke Grundfest Schoepf (New York: Basic Books, 1963). In a transformation of the Klein Group, A. J. Greimas has developed the semiotic square, which he describes as giving "a slightly different formulation to the same structure," in "The Interaction of Semiotic Constraints," On Meaning (Minneapolis: University of Minnesota Press, 1987), p. 50. Jameson uses the semiotic square in The Political Unconscious (see pp. 167, 254, 256, 277) [Fredric Jameson, The Political Unconscious: Narrative as a Socially Symbolic Act (Ithaca: Cornell University Press, 1981)], as does Louis Marin in "Disneyland: A Degenerate Utopia," Glyph, no. 1 (1977), p. 64.
Wikipedia on the Klein group (denoted V, for Vierergruppe):
In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
For material related to Klee's phrase mentioned above by Stevens, "the organic center of all movement in time and space," see the following Google search:
Kernel
Mathematical Reviews citation:
MR2163497 (2006g:81002) 81-03 (81P05)
Gieser, Suzanne The innermost kernel. Depth psychology and quantum physics. Wolfgang Pauli's dialogue with C. G. Jung. Springer-Verlag, Berlin, 2005. xiv+378 pp. ISBN: 3-540-20856-9
A quote from MR at Amazon.com:
"This revised translation of a Swedish Ph. D. thesis in philosophy offers far more than a discussion of Wolfgang Pauli's encounters with the psychoanalyst Carl Gustav Jung…. Here the book explains very well how Pauli attempted to extend his understanding beyond superficial esotericism and spiritism…. To understand Pauli one needs books like this one, which… seems to open a path to a fuller understanding of Pauli, who was seeking to solve a quest even deeper than quantum physics." (Arne Schirrmacher, Mathematical Reviews, Issue 2006g)

The four-group is also known as the Vierergruppe or Klein group. It appears, notably, as the translation subgroup of A, the group of 24 automorphisms of the affine plane over the 2-element field, and therefore as the kernel of the homomorphism taking A to the group of 6 automorphisms of the projective line over the 2-element field. (See Finite Geometry of the Square and Cube.)
The "chessboard" of
Nov. 7, 2006
(as revised Nov. 7, 2012)–
(Faust, Part Two, as
quoted by Jung in
Memories, Dreams, Reflections)
Final page of The New York Times Book Review, issue dated January 7, 2007:
On using speech-recognition software to dictate a book:
"Writing is the act of accepting the huge shortfall between the story in the mind and what hits the page. 'From your lips to God's ears,' goes the old Yiddish wish. The writer, by contrast, tries to read God's lips and pass along the words…. And for that, an interface will never be clean or invisible enough for us to get the passage right….
Everthing we write– through any medium– is lost in translation. But something new is always found again, in their eager years. In Derrida's fears. Make that: in the reader's ears."
— Richard Powers (author of The Gold Bug Variations)
continued
"At that instant he saw,
in one blaze of light,
an image of unutterable conviction….
the core of life, the essential pattern
whence all other things proceed,
the kernel of eternity."
— Thomas Wolfe,
Of Time and the River
From "The Relations between
Poetry and Painting," by Wallace Stevens:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety…. It was from the point of view of… [such a] subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space—which he calls the mind or heart of creation— determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
Diagrams of this group may have influenced Giovanni Sambin, professor of mathematical logic at the University of Padua; the following impressive-looking diagram is from Sambin's

Sambin argues that this diagram reflects some of the basic structures of thought itself… making it perhaps one way to describe what Klee called the "mind or heart of creation."
But this verges on what Stevens called the sacerdotal. It seems that a simple picture of the "kernel of eternity" as the four-group, a picture without reference to logic or philosophy, and without distracting letters and labels, is required. The following is my attempt to supply such a picture:

This is a picture of the four-group
as a permutation group on four points.
Pairs of colored arrows indicate the three
transformations other than the identity,
which may be regarded either as
invisible or as rendered by
the four black points themselves.
Update of 7:45 PM Thursday:
Review of the above (see comments)
by a typical Xanga reader:
"Ur a FUCKIN' LOSER!!!!! LMFAO!!!!"
For more merriment, see
The Optical Unconscious
and
The Painted Word.
A recent Xangan movie review:
So a big ol' fuck you to George Lucas. Fuck you, George!"
Both Xangans seem to be fluent in what Tom Wolfe has called the "fuck patois."
A related suggestion from Google:
These remarks from Xangans and Google
suggest the following photo gift,
based on a 2003 journal entry:

"Professor Krauss even uses many of the same decorations with which she festooned earlier volumes. Bataille’s photograph of a big toe, for example, which I like to think of as her mascot, reappears. As does her favorite doodle, a little graph known as a 'Klein Group' or 'L Schema' whose sides and diagonals sport arrows pointing to corners labeled with various opposing pairs: e.g., 'ground' and 'not ground,' 'figure' and 'not figure.' Professor Krauss seems to believe that this device, lifted from the pages of structuralist theory, illuminates any number of deep mysteries: the nature of modernism, to begin with, but also the essence of gender relations, self-consciousness, perception, vision, castration anxiety, and other pressing conundrums that, as it happens, she has trouble distinguishing from the nature of modernism. Altogether, the doodle is a handy thing to have around. One is not surprised that Professor Krauss reproduces it many times in her new book."

 A Jungian on this six-line figure: "They are the same six lines |
"People have believed in the fundamental character of binary oppositions since at least classical times. For instance, in his Metaphysics Aristotle advanced as primary oppositions: form/matter, natural/unnatural, active/passive, whole/part, unity/variety, before/after and being/not-being.* But it is not in isolation that the rhetorical power of such oppositions resides, but in their articulation in relation to other oppositions. In Aristotle's Physics the four elements of earth, air, fire and water were said to be opposed in pairs. For more than two thousand years oppositional patterns based on these four elements were widely accepted as the fundamental structure underlying surface reality….
 The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…."
The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully…."
— Daniel Chandler, Semiotics for Beginners.
* Compare Chandler's list of Aristotle's primary oppositions with Aristotle's list (also in the Metaphysics) of Pythagorean oppositions (see Midrash Jazz Quartet).
Powered by WordPress