Symplectic Correlation « Search Results

Sunday, August 24, 2014

Symplectic Structure…

Filed under: General,Geometry — Tags: Diamond Theorem Correlation, Octad, Octad Generator, Picture Show, Rosenhain and Göpel, Six-Set — m759 @ 12:00 pm

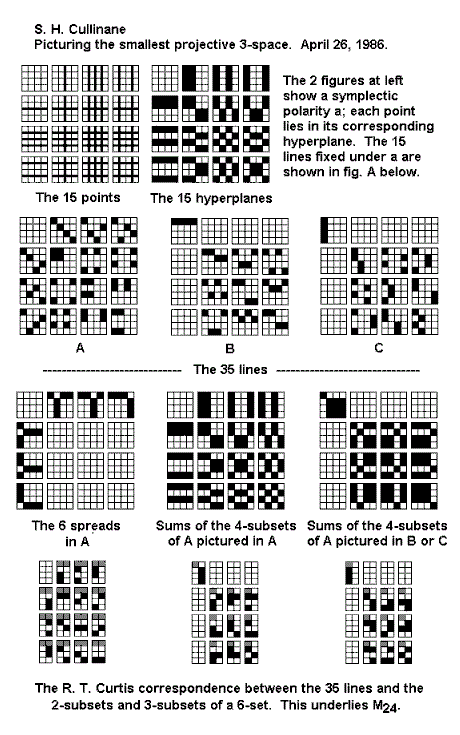
In the Miracle Octad Generator (MOG):

The above details from a one-page note of April 26, 1986, refer to the
Miracle Octad Generator of R. T. Curtis, as it was published in 1976:

From R. T. Curtis (1976). A new combinatorial approach to M₂₄,
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42. doi:10.1017/S0305004100052075.

The 1986 note assumed that the reader would be able to supply, from the
MOG itself, the missing top row of each heavy brick.

Note that the interchange of the two squares in the top row of each
heavy brick induces the diamond-theorem correlation.

Note also that the 20 pictured 3-subsets of a 6-set in the 1986 note
occur as paired complements in two pictures, each showing 10 of the
3-subsets.

This pair of pictures corresponds to the 20 Rosenhain tetrads among
the 35 lines of PG(3,2), while the picture showing the 2-subsets
corresponds to the 15 Göpel tetrads among the 35 lines.

See Rosenhain and Göpel tetrads in PG(3,2). Some further background:

Comments Off

Monday, August 11, 2014

Syntactic/Symplectic

Filed under: General,Geometry — Tags: Articulation, Diamond Theorem Correlation, Langer Key, Rosenhain and Göpel, Springer — m759 @ 4:00 pm

(Continued from August 9, 2014.)

Syntactic:

Symplectic:

"Visual forms— lines, colors, proportions, etc.— are just as capable of
articulation , i.e. of complex combination, as words. But the laws that govern
this sort of articulation are altogether different from the laws of syntax that
govern language. The most radical difference is that visual forms are not
discursive . They do not present their constituents successively, but
simultaneously, so the relations determining a visual structure are grasped
in one act of vision."

– Susanne K. Langer, Philosophy in a New Key

For examples, see The Diamond-Theorem Correlation
in Rosenhain and Göpel Tetrads in PG(3,2).

This is a symplectic correlation,* constructed using the following
visual structure:

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven) .

* Defined in (for instance) Paul B. Yale, Geometry and Symmetry ,
Holden-Day, 1968, sections 6.9 and 6.10.

Comments Off

Wednesday, August 6, 2014

Symplectic Structure*

Filed under: General,Geometry — Tags: Diamond Theorem Correlation — m759 @ 1:00 pm

From Gotay and Isenberg, "The Symplectization of Science,"
Gazette des Mathématiciens 54, 59-79 (1992):

"… what is the origin of the unusual name 'symplectic'? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure 'line complex group' the 'symplectic group.'
… the adjective 'symplectic' means 'plaited together' or 'woven.'
This is wonderfully apt…."

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven)

The above symplectic structure** now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).

Some related passages from the literature:

* The title is a deliberate abuse of language .
For the real definition of "symplectic structure," see (for instance)
"Symplectic Geometry," by Ana Cannas da Silva (article written for
Handbook of Differential Geometry, vol 2.) To establish that the
above figure is indeed symplectic , see the post Zero System of
July 31, 2014.

** See Steven H. Cullinane, Inscapes III, 1986

Comments Off

Friday, August 1, 2014

The Diamond-Theorem Correlation

Filed under: General,Geometry — Tags: Diamond Theorem Correlation, Rosenhain and Göpel — m759 @ 2:00 am

Click image for a larger, clearer version.

Comments Off

Friday, August 26, 2016

Wolfe vs. Chomsky

Filed under: General — Tags: Clash of the Titans, Crooked House — m759 @ 12:00 am

1. Tom Wolfe has a new book on Chomsky, "The Kingdom of Speech."

2. This suggests a review of a post of Aug. 11, 2014, Syntactic/Symplectic.

To paraphrase Wittgenstein, sentence 1 above is about "correlating in real life"
(cf. Crooked House and Wolfe's From Bauhaus to Our House ), and may be
compared to sentence 2 above, which links to a sort of "correlating in
mathematics" that is a particular example of the more general sort of
mathematical correlating mentioned by Wittgenstein in 1939.

Comments Off

Thursday, July 31, 2014

Zero System

Filed under: General,Geometry — Tags: Diamond Theorem Correlation, Geometry, The Rosenhain Symmetry — m759 @ 6:11 pm

The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and

"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."

See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.

An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):

IMAGE- The natural symplectic polarity in PG(3,2), illustrating a symplectic structure

See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.

Comments Off

Sunday, December 10, 2017

Geometry

Filed under: G-Notes,General,Geometry — Tags: Dirac and Geometry, Interlacing Derrida, Quantum Tesseract Theorem — m759 @ 8:45 pm

See also Symplectic in this journal.

From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):

“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven)

The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2). See also
related remarks on the notion of linear (or line ) complex
in the finite projective space PG(3,2) —

Comments Off

Wednesday, February 15, 2017

Warp and Woof

Filed under: General,Geometry — m759 @ 3:00 pm

Space —

Space structure —

From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven)

The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).

Space shuttle —

Related ethnic remarks —

… As opposed to Michael Larsen —

Funny, you don't look Danish.

Comments Off

Wednesday, November 23, 2016

Yogiism

Filed under: General,Geometry — Tags: Diamond Theorem Correlation, Inner-Outer — m759 @ 12:31 pm

From the American Mathematical Society (AMS) webpage today —

From the current AMS Notices —

Related material from a post of Aug. 6, 2014 —

(Here "five point sets" should be "five-point sets.")

From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven)

The above symplectic structure* now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).

* The phrase as used here is a deliberate
abuse of language . For the real definition of
“symplectic structure,” see (for instance)
“Symplectic Geometry,” by Ana Cannas da Silva
(article written for Handbook of Differential
Geometry , Vol 2.) To establish that the above
figure is indeed symplectic , see the post
Zero System of July 31, 2014.

Comments Off

Friday, November 13, 2015

A Connection between the 16 Dirac Matrices and the Large Mathieu Group

Filed under: General,Geometry — Tags: Cubehenge, Diamond Theorem Correlation, Dirac and Geometry, Octad, Octad Generator, Quantum Tesseract Theorem, Six-Set — m759 @ 2:45 am

Note that the six anticommuting sets of Dirac matrices listed by Arfken
correspond exactly to the six spreads in the above complex of 15 projective
lines of PG(3,2) fixed under a symplectic polarity (the diamond theorem
correlation ). As I noted in 1986, this correlation underlies the Miracle
Octad Generator of R. T. Curtis, hence also the large Mathieu group.

References:

Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214

Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986

Related material:

The 6-set in my 1986 note above also appears in a 1996 paper on
the sixteen Dirac matrices by David M. Goodmanson —

Background reading:

Ron Shaw on finite geometry, Clifford algebras, and Dirac groups
(undated compilation of publications from roughly 1994-1995)—

Comments Off

Thursday, October 15, 2015

Contrapuntal Interweaving

Filed under: General,Geometry — Tags: Diamond Theorem Correlation — m759 @ 2:01 am

The title is a phrase from R. D. Laing's book The Politics of Experience .
(Published in the psychedelic year 1967. The later "contrapuntal interweaving"
below is of a less psychedelic nature.)

An illustration of the "interweaving' part of the title —
The "deep structure" of the diamond theorem:

IMAGE- A symplectic structure -- i.e. a structure that is symplectic (meaning plaited or woven) .

The word "symplectic" from the end of last Sunday's (Oct. 11) sermon
describes the "interwoven" nature of the above illustration.

An illustration of the "contrapuntal" part of the title (click to enlarge):

Comments Off

Saturday, July 4, 2015

Context

Filed under: General,Geometry — Tags: Diamond Theorem Correlation, Priority — m759 @ 10:00 am

Some context for yesterday's post on a symplectic polarity —

This 1986 note may or may not have inspired some remarks
of Wolf Barth in his foreword to the 1990 reissue of Hudson's
1905 Kummer's Quartic Surface .

See also the diamond-theorem correlation.

Comments Off

Wednesday, February 25, 2015

Words and Images

Filed under: General,Geometry — Tags: Diamond Theorem Correlation — m759 @ 5:30 pm

The words: "symplectic polarity"—

The images:

The Natural Symplectic Polarity in PG(3,2)

Symmetry Invariance in a Diamond Ring

The Diamond-Theorem Correlation

Picturing the Smallest Projective 3-Space

Quilt Block Designs

Comments Off

Saturday, February 21, 2015

High and Low Concepts

Filed under: General,Geometry — Tags: Diamond Theorem Correlation — m759 @ 4:30 pm

Steven Pressfield on April 25, 2012:

What exactly is High Concept?

Let’s start with its opposite, low concept.
Low concept stories are personal,
idiosyncratic, ambiguous, often European.
“Well, it’s a sensitive fable about a Swedish
sardine fisherman whose wife and daughter
find themselves conflicted over … ”

ZZZZZZZZ.

Fans of Oslo artist Josefine Lyche know she has
valiantly struggled to find a high-concept approach
to the diamond theorem. Any such approach must,
unfortunately, reckon with the following low
(i.e., not easily summarized) concept —

The Diamond Theorem Correlation:

From left to right …

http://www.log24.com/log/pix14B/140824-Diamond-Theorem-Correlation-1202w.jpg

http://www.log24.com/log/pix14B/140731-Diamond-Theorem-Correlation-747w.jpg

http://www.log24.com/log/pix14B/140824-Picturing_the_Smallest-1986.gif

http://www.log24.com/log/pix14B/140806-ProjPoints.gif

For some backstory, see ProjPoints.gif and "Symplectic Polarity" in this journal.

Comments Off

Tuesday, July 9, 2013

Vril Chick

Filed under: General,Geometry — Tags: Fields, Octad, Octad Generator — m759 @ 4:30 am

Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—

Profile picture for "Jo Lyxe" (Josefine Lyche) at Vimeo

Compare to an image of Vril muse Maria Orsitsch.

From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —

Josefine Lyche
Born in 1973 in Bergen, Norway.
Lives and works in Oslo and Berlin.

Keywords (to help place my artwork in the
proper context): Aliens, affine geometry, affine
planes, affine spaces, automorphisms, binary
codes, block designs, classical groups, codes,
coding theory, collineations, combinatorial,
combinatorics, conjugacy classes, the Conwell
correspondence, correlations, Cullinane,
R. T. Curtis, design theory, the diamond theorem,
diamond theory, duads, duality, error correcting
codes, esoteric, exceptional groups,
extraterrestrials, finite fields, finite geometry, finite
groups, finite rings, Galois fields, generalized
quadrangles, generators, geometry, GF(2),
GF(4), the (24,12) Golay code, group actions,
group theory, Hadamard matrices, hypercube,
hyperplanes, hyperspace, incidence structures,
invariance, Karnaugh maps, Kirkman’s schoolgirls
problem, Latin squares, Leech lattice, linear
groups, linear spaces, linear transformations,
Magick, Mathieu groups, matrix theory, Meno,
Miracle Octad Generator, MOG, multiply transitive
groups, occultism, octahedron, the octahedral
group, Orsic, orthogonal arrays, outer automorphisms,
parallelisms, partial geometries,
permutation groups, PG(3,2), Plato, Platonic
solids, polarities, Polya-Burnside theorem, projective
geometry, projective planes, projective
spaces, projectivities, Pythagoras, reincarnation,
Reed-Muller codes, the relativity problem,
reverse engineering, sacred geometry, Singer
cycle, skew lines, Socrates, sporadic simple
groups, Steiner systems, Sylvester, symmetric,
symmetry, symplectic, synthemes, synthematic,
Theosophical Society tesseract, Tessla, transvections,
Venn diagrams, Vril society, Walsh
functions, Witt designs.

(See also the original catalog page.)

Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.

For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .

Comments Off

Tuesday, October 3, 2006

Tuesday October 3, 2006

Filed under: General,Geometry — Tags: Fields, Octad, Octad Generator, Sundown Ending — m759 @ 9:26 am

Serious

"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."

— Charles Matthews at Wikipedia, Oct. 2, 2006

"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."

— G. H. Hardy, A Mathematician's Apology

Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:

Affine group‎
Reflection group‎
Symmetry in mathematics‎
Incidence structure‎
Invariant (mathematics)‎
Symmetry‎
Finite geometry‎
Group action‎
History of geometry‎

This would appear to be a fairly large complex of mathematical ideas.

See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:

Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.

Comments Off