Friday, November 17, 2023
Schoolgirl Problem: Not Queen of the Desert
Comments Off on Schoolgirl Problem: Not Queen of the Desert
Friday, April 16, 2021
Schoolgirl Problem (Kirkman’s, not Epstein’s)
As for Epstein’s problem . . .
Comments Off on Schoolgirl Problem (Kirkman’s, not Epstein’s)
Thursday, November 19, 2020
Set Design and the Schoolgirl Problem
Underlying Structure of the Design —
Schoolgirl Problem —
Comments Off on Set Design and the Schoolgirl Problem
Sunday, July 7, 2019
Schoolgirl Problem
Anonymous remarks on the schoolgirl problem at Wikipedia —
"This solution has a geometric interpretation in connection with
Galois geometry and PG(3,2). Take a tetrahedron and label its
vertices as 0001, 0010, 0100 and 1000. Label its six edge centers
as the XOR of the vertices of that edge. Label the four face centers
as the XOR of the three vertices of that face, and the body center
gets the label 1111. Then the 35 triads of the XOR solution correspond
exactly to the 35 lines of PG(3,2). Each day corresponds to a spread
and each week to a packing."
See also Polster + Tetrahedron in this journal.
There is a different "geometric interpretation in connection with
Galois geometry and PG(3,2)" that uses a square model rather
than a tetrahedral model. The square model of PG(3,2) last
appeared in the schoolgirl-problem article on Feb. 11, 2017, just
before a revision that removed it.
Comments Off on Schoolgirl Problem
Saturday, August 25, 2018
Schoolgirl Problem
Comments Off on Schoolgirl Problem
Sunday, July 15, 2018
Schoolgirl Problems
A Warren, Pennsylvania, newspaper article from May 12, 2018,
“A terrorist among them,” quotes Ann Creal of Warren on
schooldays of the late 1950’s and on a German exchange student,
Gudrun Ensslin, who later became famous for her violent political
activities:
“She said Ensslin dated while here (the man
she identified as Ensslin’s date told the Times Observer
he had no recollection of her).”
I am the man that was identified as Ensslin’s date, and I still
have no recollection of her.
Ann Creal is the former Ann Fuellhart, who was a college freshman
in the fall of 1959, when I was a high school senior —
Ann Creal apparently confused me with Scott Mohr, who
graduated from Warren High School in 1958. See the Log24
posts Crux and Doppelgänger.
Comments Off on Schoolgirl Problems
Tuesday, November 29, 2016
Thursday, August 4, 2016
Schoolgirl Problems
Compare and contrast the recent films
"The Diary of a Teenage Girl" and "Strangerland."
(This post was suggested by yesterday's
"How Deep the Rabbit Hole Goes.")
Comments Off on Schoolgirl Problems
Monday, February 22, 2016
Schoolgirl Problems…
and versions of "Both Sides Now"
See a New York Times version of "Both Sides Now."
I prefer a version by Umberto Eco.
Related material for storytellers and the Church of Synchronology —
This journal on the date of the above shooting script, 03/19/15.
Comments Off on Schoolgirl Problems…
Tuesday, September 15, 2015
Schoolgirl Problem
Or: Ten Years and a Day
In memory of film director Robert Wise,
who died ten years ago yesterday.
A search in this journal for "Schoolgirl" ends with a post
from Sept. 10, 2002, The Sound of Hanging Rock.
See as well a Log24 search for "Strangerland"
(a 2015 film about a search for a schoolgirl) and
a Log24 search for "Weaving."
Related mathematics: Symplectic.
Some related images (click to enlarge) —
Comments Off on Schoolgirl Problem
Friday, August 14, 2015
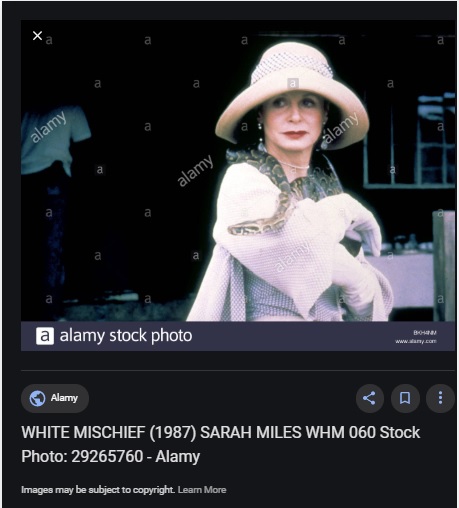
Schoolgirl Problem
But first, a word from our sponsa* …
Sir Laurence Olivier in "Term of Trial" (1962),
a film starring Sarah Miles as a schoolgirl —
* Bride in Latin. See also "bride's chair,"
a phrase from mathematical pedagogy.
Comments Off on Schoolgirl Problem
Friday, June 19, 2015
Schoolgirl Problem
The star of the new film "Chappie" is on tour.
Comments Off on Schoolgirl Problem
Friday, September 27, 2019
Algebra for Schoolgirls
The 15 points of the finite projective 3-space PG(3,2)
arranged in tetrahedral form:
The letter labels, but not the tetrahedral form,
are from The Axioms of Projective Geometry , by
Alfred North Whitehead (Cambridge U. Press, 1906).
The above space PG(3,2), because of its close association with
Kirkman's schoolgirl problem, might be called "schoolgirl space."
Screen Rant on July 31, 2019:
A Google Search sidebar this morning:
Apocalypse Soon! —
Comments Off on Algebra for Schoolgirls
Thursday, August 15, 2019
Schoolgirl Space — Tetrahedron or Square?
The exercise in the previous post was suggested by a passage
purporting to "use standard block design theory" that was written
by some anonymous author at Wikipedia on March 1, 2019:
Here "rm OR" apparently means "remove original research."
Before the March 1 revision . . .
The "original research" objected to and removed was the paragraph
beginning "To explain this further." That paragraph was put into the
article earlier on Feb. 28 by yet another anonymous author (not by me).
An account of my own (1976 and later) original research on this subject
is pictured below, in a note from Feb. 20, 1986 —
Comments Off on Schoolgirl Space — Tetrahedron or Square?
Saturday, August 10, 2019
Schoolgirl Space* Revisited:
The Square "Inscape" Model of
the Generalized Quadrangle W(2)
Click image to enlarge.
-embedding-in-PG(3,2)-Planat-Saniga-1000w.jpg)
* The title refers to the role of PG (3,2) in Kirkman's schoolgirl problem.
For some backstory, see my post Anticommuting Dirac Matrices as Skew Lines
and, more generally, posts tagged Dirac and Geometry.
Comments Off on Schoolgirl Space* Revisited:
Tuesday, July 16, 2019
Sunday, February 17, 2019
For the Schoolgirls of 1959
The dies natalis of St. Buddy Holly was Feb. 3, 1959.
This year on Feb. 3, a geometric illustration of the well-known
schoolgirl problem was added to a brand-new Wikipedia article
on the finite geometry PG(3,2).
Comments Off on For the Schoolgirls of 1959
Thursday, February 16, 2017
Schoolgirls and Heptads
A Feb. 12 note in the "talk" section of the Wikipedia article
"Kirkman's schoolgirl problem" —
The illustration above was replaced by a new section in the article,
titled "Galois geometry."
The new section improves the article by giving it greater depth.
For related material, see Conwell Heptads in this journal
(or, more generally, Conwell) and a 1985 note citing Conwell's work.
Comments Off on Schoolgirls and Heptads
Monday, November 18, 2024
Wednesday, September 18, 2019
Powers of X
Screen Rant on July 31, 2019 —
The above space PG(3,2), because of its close association with
Kirkman's schoolgirl problem, might be called "schoolgirl space."
See as well a Log24 post from the above Screen Rant date —
Comments Off on Powers of X
Tuesday, April 30, 2019
Heraldry for Walpurgisnacht
Comments Off on Heraldry for Walpurgisnacht
Tuesday, January 2, 2018
Debs and Redhead
The above images were suggested in part by the birthdays
on Sept. 21, 2011, of Bill Murray and Stephen King.
More seriously, also in this journal on that date, from a post
titled Symmetric Generation —
Comments Off on Debs and Redhead
Sunday, November 12, 2017
Review
|
Sunday, October 29, 2017
File System… Unlocked
|
See as well Chloë Grace Moretz portraying a schoolgirl problem.
Comments Off on Review
Thursday, November 2, 2017
History of Mathematics for All Souls’ Day
Schoolgirl Problem
"Buy this image" . . . Or not.
Related material from the date of the above photo —
For related drama, see "Child's Play" in this journal.
Comments Off on History of Mathematics for All Souls’ Day
Thursday, October 12, 2017
Gifted Continues
Related material — See Gifted in this journal.
See as well Tulips.
Yesterday was the International Day of the Girl Child . . .
A related archived Wikipedia article on Kirkman's schoolgirl problem :
See also the previous post— "IPFS Version"— and https://ipfs.io/.
Comments Off on Gifted Continues
Wednesday, July 5, 2017
Imaginarium of a Different Kind
The title refers to that of the previous post, "The Imaginarium."
In memory of a translator who reportedly died on May 22, 2017,
a passage quoted here on that date —
Related material — A paragraph added on March 15, 2017,
to the Wikipedia article on Galois geometry —
|
George Conwell gave an early demonstration of Galois geometry in 1910 when he characterized a solution of Kirkman's schoolgirl problem as a partition of sets of skew lines in PG(3,2), the three-dimensional projective geometry over the Galois field GF(2).[3] Similar to methods of line geometry in space over a field of characteristic 0, Conwell used Plücker coordinates in PG(5,2) and identified the points representing lines in PG(3,2) as those on the Klein quadric. — User Rgdboer |
Comments Off on Imaginarium of a Different Kind
Wednesday, June 1, 2016
Mathematics and Narrative
Principles before Personalities — AA Saying .
Principles —
See Schoolgirl Problem in Wikipedia.
Personalities —
See Alexandra Alter in the May 26 online New York Times :
"With the proliferation of 'girl' titles,
there are signs that the trend may have peaked;
it already seems ripe for parody."
Update of 12:40 PM ET on Wednesday, June 1, 2016 —
A note for the Church of Synchronology …
See a post from this journal on the date of the Alter piece, May 26:
(Click image for the rest of the post .)
Comments Off on Mathematics and Narrative
Monday, May 30, 2016
Perfect Universe
(A sequel to the previous post, Perfect Number)
Since antiquity, six has been known as
"the smallest perfect number." The word "perfect"
here means that a number is the sum of its
proper divisors — in the case of six: 1, 2, and 3.
The properties of a six-element set (a "6-set")
divided into three 2-sets and divided into two 3-sets
are those of what Burkard Polster, using the same
adjective in a different sense, has called
"the smallest perfect universe" — PG(3,2), the projective
3-dimensional space over the 2-element Galois field.
A Google search for the phrase "smallest perfect universe"
suggests a turnaround in meaning , if not in finance,
that might please Yahoo CEO Marissa Mayer on her birthday —
The semantic turnaround here in the meaning of "perfect"
is accompanied by a model turnaround in the picture of PG(3,2) as
Polster's tetrahedral model is replaced by Cullinane's square model.
Further background from the previous post —
See also Kirkman's Schoolgirl Problem.
Comments Off on Perfect Universe
Wednesday, July 17, 2019
The Artsy Quantum Realm
|
arXiv.org > quant-ph > arXiv:1905.06914 Quantum Physics Placing Kirkman's Schoolgirls and Quantum Spin Pairs on the Fano Plane: A Rainbow of Four Primary Colors, A Harmony of Fifteen Tones J. P. Marceaux, A. R. P. Rau (Submitted on 14 May 2019) A recreational problem from nearly two centuries ago has featured prominently in recent times in the mathematics of designs, codes, and signal processing. The number 15 that is central to the problem coincidentally features in areas of physics, especially in today's field of quantum information, as the number of basic operators of two quantum spins ("qubits"). This affords a 1:1 correspondence that we exploit to use the well-known Pauli spin or Lie-Clifford algebra of those fifteen operators to provide specific constructions as posed in the recreational problem. An algorithm is set up that, working with four basic objects, generates alternative solutions or designs. The choice of four base colors or four basic chords can thus lead to color diagrams or acoustic patterns that correspond to realizations of each design. The Fano Plane of finite projective geometry involving seven points and lines and the tetrahedral three-dimensional simplex of 15 points are key objects that feature in this study. Comments:16 pages, 10 figures Subjects:Quantum Physics (quant-ph) Cite as:arXiv:1905.06914 [quant-ph] (or arXiv:1905.06914v1 [quant-ph] for this version) Submission history
From: A. R. P. Rau [view email] |
See also other posts tagged Tetrahedron vs. Square.
Comments Off on The Artsy Quantum Realm
Friday, February 22, 2019
Desperately Seeking Comedy
"I need a photo opportunity . . ." — Paul Simon
Comments Off on Desperately Seeking Comedy
Sunday, October 29, 2017
File System… Unlocked
Logo from the above webpage —
See also the similar structure of the eightfold cube, and …
Related dialogue from the new film "Unlocked" —
1057
01:31:59,926 –> 01:32:01,301
Nice to have you back, Alice.
1058
01:32:04,009 –> 01:32:05,467
Don't be a stranger.
Comments Off on File System… Unlocked
Wednesday, October 11, 2017
Saturday, May 20, 2017
van Lint and Wilson Meet the Galois Tesseract*
Click image to enlarge.
The above 35 projective lines, within a 4×4 array —
The above 15 projective planes, within a 4×4 array (in white) —
* See Galois Tesseract in this journal.
Comments Off on van Lint and Wilson Meet the Galois Tesseract*
Wednesday, February 15, 2017
Warp and Woof
Space —
Space structure —
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Space shuttle —
Related ethnic remarks —
… As opposed to Michael Larsen —
Funny, you don't look Danish.
Comments Off on Warp and Woof
Sunday, June 14, 2015
New Image of PG(3,2)
… was added to the Wikipedia article Finite geometry.
(Shown above is a slightly newer image, changed to reflect
the Wikipedia article's remarks on the schoolgirl problem.)
Comments Off on New Image of PG(3,2)
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—
Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Comments Off on Vril Chick
Wednesday, March 11, 2009
Wednesday March 11, 2009
Sein Feld
in Translation
(continued from
May 15, 1998)
in Translation
(continued from
May 15, 1998)
The New York Times March 10–
"Paris | A Show About Nothing"–
The Times describes one of the empty rooms on exhibit as…
"… Yves Klein’s 'La spécialisation de la sensibilité à l’état matière première en sensibilité picturale stabilisée, Le Vide' ('The Specialization of Sensibility in the Raw Material State Into Stabilized Pictorial Sensibility, the Void')"
This is a mistranslation. See "An Aesthetics of Matter" (pdf), by Kiyohiko Kitamura and Tomoyuki Kitamura, pp. 85-101 in International Yearbook of Aesthetics, Volume 6, 2002—
"The exhibition «La spécialisation de la sensibilité à l’état matière-première en sensibilité picturale stabilisée», better known as «Le Vide» (The Void) was held at the Gallery Iris Clert in Paris from April 28th till May 5th, 1955." –p. 94
"… «Sensibility in the state of prime matter»… filled the emptiness." –p. 95
Kitamura and Kitamura translate matière première correctly as "prime matter" (the prima materia of the scholastic philosophers) rather than "raw material." (The phrase in French can mean either.)
Related material:
The Diamond Archetype and
The Illuminati Diamond.
The Diamond Archetype and
The Illuminati Diamond.
The link above to
prima materia
is to an 1876 review
by Cardinal Manning of
a work on philosophy
by T. P. Kirkman, whose
"schoolgirl problem" is
closely related to the
finite space of the
diamond theorem.
Comments Off on Wednesday March 11, 2009
Tuesday, October 3, 2006
Tuesday October 3, 2006
Serious
"I don't think the 'diamond theorem' is anything serious, so I started with blitzing that."
— Charles Matthews at Wikipedia, Oct. 2, 2006
"The 'seriousness' of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects. We may say, roughly, that a mathematical idea is 'significant' if it can be connected, in a natural and illuminating way, with a large complex of other mathematical ideas."
— G. H. Hardy, A Mathematician's Apology
Matthews yesterday deleted references to the diamond theorem and related material in the following Wikipedia articles:
Affine group
Reflection group
Symmetry in mathematics
Incidence structure
Invariant (mathematics)
Symmetry
Finite geometry
Group action
History of geometry
This would appear to be a fairly large complex of mathematical ideas.
See also the following "large complex" cited, following the above words of Hardy, in Diamond Theory:
Affine geometry, affine planes, affine spaces, automorphisms, binary codes, block designs, classical groups, codes, coding theory, collineations, combinatorial, combinatorics, conjugacy classes, the Conwell correspondence, correlations, design theory, duads, duality, error correcting codes, exceptional groups, finite fields, finite geometry, finite groups, finite rings, Galois fields, generalized quadrangles, generators, geometry, GF(2), GF(4), the (24,12) Golay code, group actions, group theory, Hadamard matrices, hypercube, hyperplanes, hyperspace, incidence structures, invariance, Karnaugh maps, Kirkman's schoolgirl problem, Latin squares, Leech lattice, linear groups, linear spaces, linear transformations, Mathieu groups, matrix theory, Meno, Miracle Octad Generator, MOG, multiply transitive groups, octads, the octahedral group, orthogonal arrays, outer automorphisms, parallelisms, partial geometries, permutation groups, PG(3,2), polarities, Polya-Burnside theorem, projective geometry, projective planes, projective spaces, projectivities, Reed-Muller codes, the relativity problem, Singer cycle, skew lines, sporadic simple groups, Steiner systems, symmetric, symmetry, symplectic, synthemes, synthematic, tesseract, transvections, Walsh functions, Witt designs.
Comments Off on Tuesday October 3, 2006