Matthew Hunter on May 22, 2024 —
And then there are large language models . . .
See machine learning on the Web and "emergent" here.
Matthew Hunter on May 22, 2024 —
And then there are large language models . . .
See machine learning on the Web and "emergent" here.
Analogy . . . When A/B = C/D . . .
Illustration from a Log24 post tagged Quale —
— and related material from the date of the above Quale post …
… as well as, from July 31, a version for the institutionalized —
From the previous post, "The Large Language Model,"
a passage from Wikipedia —
"… sometimes large models undergo a 'discontinuous phase shift'
where the model suddenly acquires substantial abilities not seen
in smaller models. These are known as 'emergent abilities,' and
have been the subject of substantial study." — Wikipedia
Compare and contrast
this with the change undergone by a "small space model,"
that of the finite affine 4-space A with 16 points (a Galois tesseract ),
when it is augmented by an eight-point "octad." The 30 eight-point
hyperplanes of A then have a natural extension within the new
24-point set to 759 eight-point octads, and the 322,560 affine
automorphisms of the space expand to the 244,823,040 Mathieu
automorphisms of the 759-octad set — a (5, 8, 24) Steiner system.
For a visual analogue of the enlarged 24-point space and some remarks
on analogy by Simone Weil's brother, a mathematician, see this journal
on September 8 and 9, 2022.
"… sometimes large models undergo a 'discontinuous phase shift'
where the model suddenly acquires substantial abilities not seen
in smaller models. These are known as 'emergent abilities,' and
have been the subject of substantial study." — Wikipedia
See also the first five episodes of "Mrs. Davis."
Welcome to Westview continues.
My Windows lockscreen this morning features a badger
emerging from his den. Microsoft’s commentary —

Related commentary from Bellevue —
“History, Stephen said, is a nightmare
from which I am trying to awake.”
— James Joyce, Ulysses
Story, Structure, and the Galois Tesseract
Recent Log24 posts have referred to the
"Penrose diamond" and Minkowski space.
The Penrose diamond has nothing whatever
to do with my 1976 monograph "Diamond Theory,"
except for the diamond shape and the connection
of the Penrose diamond to the Klein quadric—

The Klein quadric occurs in the five-dimensional projective space
over a field. If the field is the two-element Galois field GF(2), the
quadric helps explain certain remarkable symmetry properties
of the R. T. Curtis Miracle Octad Generator (MOG), hence of
the large Mathieu group M24. These properties are also
relevant to the 1976 "Diamond Theory" monograph.
For some background on the quadric, see (for instance)…

See also The Klein Correspondence,
Penrose Space-Time, and a Finite Model.
Related material:
|
"… one might crudely distinguish between philosophical – J. M. E. Hyland. "Proof Theory in the Abstract." (pdf) |
Those who prefer story to structure may consult
From "The Stone" (a philosophy series) in today's New York Times —
Louis Sullivan, in his Kindergarten Chats , on the pier and lintel as basic elements of architecture:
… [Sullivan says that the pier] embodies “the simplest physical beginnings"….
Add the lintel and “presto!” ….
But he has a question: what is this sudden coming-into-being of a way of being?
“We have no true name for it in the language. But if you fix the phenomenon well in your thought,
the absence of an exact word for it need not matter much.”
The source —
A related passage —
"Crucial here is the concept of emergence , the sudden coming into being of something that is greater than its component parts (like water is greater than its component parts, hydrogen and oxygen; therefore, water is an emergent property)."
— Article on Bakhtin in The Cervantes Encyclopedia , by Howard Mancing (Greenwood Publishing Group, 2004)
See also "strong emergence" in this journal.
The Dreidel Is Cast
The Nietzschean phrase "ruling and Caesarian spirits" occurred in yesterday morning's post "Novel Ending."
That post was followed yesterday morning by a post marking, instead, a beginning— that of Hanukkah 2010. That Jewish holiday, whose name means "dedication," commemorates the (re)dedication of the Temple in Jerusalem in 165 BC.
The holiday is celebrated with, among other things, the Jewish version of a die— the dreidel . Note the similarity of the dreidel to an illustration of The Stone* on the cover of the 2001 Eerdmans edition of Charles Williams's 1931 novel Many Dimensions—

For mathematics related to the dreidel , see Ivars Peterson's column on this date fourteen years ago.
For mathematics related (if only poetically) to The Stone , see "Solomon's Cube" in this journal.
Here is the opening of Many Dimensions—

For a fanciful linkage of the dreidel 's concept of chance to The Stone 's concept of invariant law, note that the New York Lottery yesterday evening (the beginning of Hanukkah) was 840. See also the number 840 in the final post (July 20, 2002) of the "Solomon's Cube" search.
Some further holiday meditations on a beginning—
Today, on the first full day of Hanukkah, we may or may not choose to mark another beginning— that of George Frederick James Temple, who was born in London on this date in 1901. Temple, a mathematician, was President of the London Mathematical Society in 1951-1953. From his MacTutor biography—
"In 1981 (at the age of 80) he published a book on the history of mathematics. This book 100 years of mathematics (1981) took him ten years to write and deals with, in his own words:-
those branches of mathematics in which I had been personally involved.
He declared that it was his last mathematics book, and entered the Benedictine Order as a monk. He was ordained in 1983 and entered Quarr Abbey on the Isle of Wight. However he could not stop doing mathematics and when he died he left a manuscript on the foundations of mathematics. He claims:-
The purpose of this investigation is to carry out the primary part of Hilbert's programme, i.e. to establish the consistency of set theory, abstract arithmetic and propositional logic and the method used is to construct a new and fundamental theory from which these theories can be deduced."
For a brief review of Temple's last work, see the note by Martin Hyland in "Fundamental Mathematical Theories," by George Temple, Philosophical Transactions of the Royal Society, A, Vol. 354, No. 1714 (Aug. 15, 1996), pp. 1941-1967.
The following remarks by Hyland are of more general interest—
"… one might crudely distinguish between philosophical and mathematical motivation. In the first case one tries to convince with a telling conceptual story; in the second one relies more on the elegance of some emergent mathematical structure. If there is a tradition in logic it favours the former, but I have a sneaking affection for the latter. Of course the distinction is not so clear cut. Elegant mathematics will of itself tell a tale, and one with the merit of simplicity. This may carry philosophical weight. But that cannot be guaranteed: in the end one cannot escape the need to form a judgement of significance."
— J. M. E. Hyland. "Proof Theory in the Abstract." (pdf)
Annals of Pure and Applied Logic 114, 2002, 43-78.
Here Hyland appears to be discussing semantic ("philosophical," or conceptual) and syntactic ("mathematical," or structural) approaches to proof theory. Some other remarks along these lines, from the late Gian-Carlo Rota—
See also "Galois Connections" at alpheccar.org and "The Galois Connection Between Syntax and Semantics" at logicmatters.net.
* Williams's novel says the letters of The Stone are those of the Tetragrammaton— i.e., Yod, He, Vau, He (cf. p. 26 of the 2001 Eerdmans edition). But the letters on the 2001 edition's cover Stone include the three-pronged letter Shin , also found on the dreidel . What esoteric religious meaning is implied by this, I do not know.
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
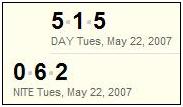
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):

This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:

Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
— Don McLean
— Michael Silberstein, “Reduction, Emergence and Explanation” (pdf), Chapter Five in The Blackwell Guide to the Philosophy of Science
“The whole is greater than the sum of its parts, no matter what you name it.”
— Alfred Bester, Chapter Eight, “The Search,” in The Deceivers
Powered by WordPress