CHANGE
FEW CAN BELIEVE IN |
What I Loved, a novel by Siri Hustvedt (New York, Macmillan, 2003), contains a paragraph on the marriage of a fictional artist named Wechsler–
Page 67 —
“… Bill and Violet were married. The wedding was held in the Bowery loft on June 16th, the same day Joyce’s Jewish Ulysses had wandered around Dublin. A few minutes before the exchange of vows, I noted that Violet’s last name, Blom, was only an o away from Bloom, and that meaningless link led me to reflect on Bill’s name, Wechsler, which carries the German root for change, changing, and making change. Blooming and changing, I thought.”
For Hustvedt’s discussion of Wechsler’s art– sculptured cubes, which she calls “tightly orchestrated semantic bombs” (p. 169)– see Log24, May 25, 2008.
Related material:
Wechsler cubes
(after David Wechsler,
1896-1981, chief
psychologist at Bellevue)
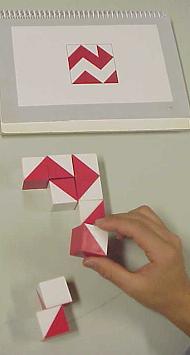
These cubes are used to
make 3×3 patterns for
psychological testing.
Related 3×3 patterns appear
in “nine-patch” quilt blocks
and in the following–
Don Park at docuverse.com, Jan. 19, 2007:
“How to draw an Identicon

A 9-block is a small quilt using only 3 types of patches, out of 16 available, in 9 positions. Using the identicon code, 3 patches are selected: one for center position, one for 4 sides, and one for 4 corners.
Positions and Rotations
For center position, only a symmetric patch is selected (patch 1, 5, 9, and 16). For corner and side positions, patch is rotated by 90 degree moving clock-wise starting from top-left position and top position respectively.” |
| Jared Tarbell at levitated.net, May 15, 2002:
“The nine block is a common design pattern among quilters. Its construction methods and primitive building shapes are simple, yet produce millions of interesting variations.
Figure A. Four 9 block patterns, arbitrarily assembled, show the
grid composition of the block.
Each block is composed of 9 squares, arranged in a 3 x 3 grid. Each square is composed of one of 16 primitive shapes. Shapes are arranged such that the block is radially symmetric. Color is modified and assigned arbitrarily to each new block.
The basic building blocks of the nine block are limited to 16 unique geometric shapes. Each shape is allowed to rotate in 90 degree increments. Only 4 shapes are allowed in the center position to maintain radial symmetry.
Figure B. The 16 possible shapes allowed
for each grid space. The 4 shapes allowed
in the center have bold numbers.”
|
Such designs become of mathematical interest when their size is increased slightly, from square arrays of nine blocks to square arrays of sixteen. See Block Designs in Art and Mathematics.
(This entry was suggested by examples of 4×4 Identicons in use at Secret Blogging Seminar.)















