Some illustrations:
Chess Knight
(in German, Springer)
See also…
More technically (click image for details):
Sunday, August 10, 2014
Saturday, November 4, 2017
Seven-Cycles in an Octad
Figures from a search in this journal for Springer Knight
and from the All Souls' Day post The Trojan Pony —
For those who prefer pure abstraction to the quasi-figurative
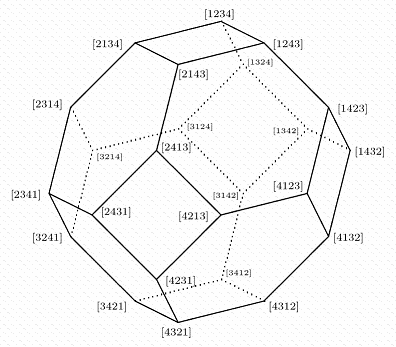
1985 seven-cycle above, a different 7-cycle for M24 , from 1998 —
Compare and contrast with my own "knight" labeling
of a 4-row 2-column array (an M24 octad, in the system
of R. T. Curtis) by the 8 points of the projective line
over GF(7), from 2008 —
Comments Off on Seven-Cycles in an Octad
Thursday, November 2, 2017
The Trojan Pony
From a search in this journal for Springer Knight —
Related material from Academia —
See also Log24 posts from the above "magic" date,
December 4, 2014, now tagged The Pony Argument.
Comments Off on The Trojan Pony
Sunday, August 23, 2020
Sprechen Sie Neutsch?
Comments Off on Sprechen Sie Neutsch?
Friday, April 5, 2019
April 1 Omega
From posts tagged Number Art —
From the novel Point Omega —
Related material for
Mathematics Awareness Month —
Also on 07/18/2015 —
Comments Off on April 1 Omega
Tuesday, December 25, 2018
Simply
|
"So to obtain the isomorphism from L2(7) onto L3(2) we simply
— Sphere Packings, Lattices and Groups , |
Compare and contrast —
.gif)
This post was suggested by a New York Times headline today —
Comments Off on Simply
Tuesday, October 3, 2017
Personalized Book Search
Click to enlarge —
The quote from Hermann Weyl on which the above search is based
is from a search within this journal for Springer + Knight.
Comments Off on Personalized Book Search
Wednesday, October 26, 2011
Erlanger and Galois
Peter J. Cameron yesterday on Galois—
"He was killed in a duel at the age of 20…. His work languished for another 14 years until Liouville published it in his Journal; soon it was recognised as the foundation stone of modern algebra, a position it has never lost."
Here Cameron is discussing Galois theory, a part of algebra. Galois is known also as the founder* of group theory, a more general subject.
Group theory is an essential part of modern geometry as well as of modern algebra—
"In der Galois'schen Theorie, wie hier, concentrirt sich das Interesse auf Gruppen von Änderungen. Die Objecte, auf welche sich die Änderungen beziehen, sind allerdings verschieden; man hat es dort mit einer endlichen Zahl discreter Elemente, hier mit der unendlichen Zahl von Elementen einer stetigen Mannigfaltigkeit zu thun."
— Felix Christian Klein, Erlanger Programm , 1872
("In the Galois theory, as in ours, the interest centres on groups of transformations. The objects to which the transformations are applied are indeed different; there we have to do with a finite number of discrete elements, here with the infinite number of elements in a continuous manifoldness." (Translated by M.W. Haskell, published in Bull. New York Math. Soc. 2, (1892-1893), 215-249))
Related material from Hermann Weyl, Symmetry , Princeton University Press, 1952 (paperback reprint of 1982, pp. 143-144)—
"A field is perhaps the simplest algebraic structure we can invent. Its elements are numbers…. Space is another example of an entity endowed with a structure. Here the elements are points…. What we learn from our whole discussion and what has indeed become a guiding principle in modern mathematics is this lesson: Whenever you have to do with a structure-endowed entity Σ try to determine is group of automorphisms , the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of Σ in this way."
For a simple example of a group acting on a field (of 8 elements) that is also a space (of 8 points), see Generating the Octad Generator and Knight Moves.
* Joseph J. Rotman, An Introduction to the Theory of Groups , 4th ed., Springer, 1994, page 2
Comments Off on Erlanger and Galois
Tuesday, February 16, 2010
Mysteries of Faith
From today's NY Times—
Obituaries for mystery authors
Ralph McInerny and Dick Francis
From the date (Jan. 29) of McInerny's death–
"…although a work of art 'is formed around something missing,' this 'void is its vanishing point, not its essence.'"
– Harvard University Press on Persons and Things (Walpurgisnacht, 2008), by Barbara Johnson
From the date (Feb. 14) of Francis's death–
The EIghtfold Cube
The "something missing" in the above figure is an eighth cube, hidden behind the others pictured.
This eighth cube is not, as Johnson would have it, a void and "vanishing point," but is instead the "still point" of T.S. Eliot. (See the epigraph to the chapter on automorphism groups in Parallelisms of Complete Designs, by Peter J. Cameron. See also related material in this journal.) The automorphism group here is of course the order-168 simple group of Felix Christian Klein.
For a connection to horses, see
a March 31, 2004, post
commemorating the birth of Descartes
and the death of Coxeter–
Putting Descartes Before Dehors
For a more Protestant meditation,
see The Cross of Descartes—
"I've been the front end of a horse
and the rear end. The front end is better."
— Old vaudeville joke
For further details, click on
the image below–
Notre Dame Philosophical Reviews
Comments Off on Mysteries of Faith
Saturday, April 4, 2009
Saturday April 4, 2009
Annual Tribute to
The Eight
The Eight
Other knight figures:
Click on the SpringerLink
knight for a free copy
(pdf, 1.2 mb) of
the following paper
dealing with the geometry
underlying the R.T. Curtis
knight figures above:
Context:
Literature and Chess and
Sporadic Group References
Details:
| Adapted (for HTML) from the opening paragraphs of the above paper, W. Jonsson's 1970 "On the Mathieu Groups M22, M23, M24…"–
"[A]… uniqueness proof is offered here based upon a detailed knowledge of the geometric aspects of the elementary abelian group of order 16 together with a knowledge of the geometries associated with certain subgroups of its automorphism group. This construction was motivated by a question posed by D.R. Hughes and by the discussion Edge [5] (see also Conwell [4]) gives of certain isomorphisms between classical groups, namely
where A8 is the alternating group on eight symbols, S6 the symmetric group on six symbols, Sp(4,2) and PSp(4,2) the symplectic and projective symplectic groups in four variables over the field GF(2) of two elements, [and] PGL, PSL and SL are the projective linear, projective special linear and special linear groups (see for example [7], Kapitel II). The symplectic group PSp(4,2) is the group of collineations of the three dimensional projective space PG(3,2) over GF(2) which commute with a fixed null polarity tau…." References 4. Conwell, George M.: The three space PG(3,2) and its group. Ann. of Math. (2) 11, 60-76 (1910). 5. Edge, W.L.: The geometry of the linear fractional group LF(4,2). Proc. London Math. Soc. (3) 4, 317-342 (1954). 7. Huppert, B.: Endliche Gruppen I. Berlin-Heidelberg-New York: Springer 1967. |
Comments Off on Saturday April 4, 2009
Sunday, June 1, 2008
Sunday June 1, 2008
Yet Another
Cartoon Graveyard
Cartoon Graveyard
The conclusion of yesterday’s commentary on the May 30-31 Pennsylvania Lottery numbers:
Thomas Pynchon, Gravity’s Rainbow:
“The fear balloons again inside his brain. It will not be kept down with a simple Fuck You…. A smell, a forbidden room, at the bottom edge of his memory. He can’t see it, can’t make it out. Doesn’t want to. It is allied with the Worst Thing.
He knows what the smell has to be: though according to these papers it would have been too early for it, though he has never come across any of the stuff among the daytime coordinates of his life, still, down here, back here in the warm dark, among early shapes where the clocks and calendars don’t mean too much, he knows that’s what haunting him now will prove to be the smell of Imipolex G.
Then there’s this recent dream he is afraid of having again. He was in his old room, back home. A summer afternoon of lilacs and bees and
286”
What are we to make of this enigmatic 286? (No fair peeking at page 287.)
One possible meaning, given The Archivist‘s claim that “existence is infinitely cross-referenced”–
Page 286 of Ernest G. Schachtel, Metamorphosis: On the Conflict of Human Development and the Psychology of Creativity (first published in 1959), Hillsdale NJ and London, The Analytic Press, 2001 (chapter– “On Memory and Childhood Amnesia”):
“Both Freud and Proust speak of the autobiographical [my italics] memory, and it is only with regard to this memory that the striking phenomenon of childhood amnesia and the less obvious difficulty of recovering any past experience may be observed.”
The concluding “summer afternoon of lilacs and bees” suggests that 286 may also be a chance allusion to the golden afternoon of Disney’s Alice in Wonderland. (Cf. St. Sarah’s Day, 2008)
Some may find the Disney afternoon charming; others may see it as yet another of Paul Simon’s dreaded cartoon graveyards.
More tastefully, there is poem 286 in the 1919 Oxford Book of English Verse– “Love.”
For a midrash on this poem, see Simone Weil, who became acquainted with the poem by chance:
“I always prefer saying chance rather than Providence.”
— Simone Weil, letter of about May 15, 1942
Weil’s brother André might prefer Providence (source of the Bulletin of the American Mathematical Society.)
(Photo from Providence)
Related material:
Log24, December 20, 2003–
White, Geometric, and Eternal—
White, Geometric, and Eternal—
A description in Gravity’s Rainbow of prewar Berlin as “white and geometric” suggested, in combination with a reference elsewhere to “the eternal,” a citation of the following illustration of the concept “white, geometric, and eternal”–
For more on the mathematical significance of this figure, see (for instance) Happy Birthday, Hassler Whitney, and Combinatorics of Coxeter Groups, by Anders Björner and Francesco Brenti, Graduate Texts in Mathematics, vol. 231, Springer, New York, 2005.
This book is reviewed in the current issue (July 2008) of the above-mentioned Providence Bulletin.
The review in the Bulletin discusses reflection groups in continuous spaces.
For a more elementary approach, see Reflection Groups in Finite Geometry and Knight Moves: The Relativity Theory of Kindergarten Blocks.
See also a commentary on
the phrase “as a little child.”
the phrase “as a little child.”
Comments Off on Sunday June 1, 2008
Saturday, February 23, 2008
Saturday February 23, 2008
Jumpers
"An acute study of the links
between word and fact"
— Nina daVinci Nichols
Thanks to a Virginia reader for a reminder:
| Virginia | /391062427/item.html? | 2/22/2008 7:37 PM |
The link is to a Log24 entry
that begins as follows…
that begins as follows…
Johnny Cash:
"And behold,
a white horse."
Chess Knight
(in German, Springer)
This, along with the "jumper" theme in the previous two entries, suggests a search on springer jumper.That search yields a German sports phrase, "Springer kommt!" A search on that phrase yields the following:
"Liebe Frau vBayern,
mich würde interessieren wie man
mit diesem Hintergrund
(vonbayern.de/german/anna.html)
zu Springer kommt?"
Background of "Frau vBayern" from thePeerage.com:
Anna-Natascha Prinzessin zu Sayn-Wittgenstein-Berleburg
F, #64640, b. 15 March 1978Last Edited=20 Oct 2005
Anna-Natascha Prinzessin zu Sayn-Wittgenstein-Berleburg was born on 15 March 1978. She is the daughter of Ludwig Ferdinand Prinz zu Sayn-Wittgenstein-Berleburg and Countess Yvonne Wachtmeister af Johannishus. She married Manuel Maria Alexander Leopold Jerg Prinz von Bayern, son of Leopold Prinz von Bayern and Ursula Mohlenkamp, on 6 August 2005 at Nykøping, Södermanland, Sweden.
The date of the above "Liebe Frau vBayern" inquiry, Feb. 1, 2007, suggests the following:
From Log24 on
St. Bridget's Day, 2007:
The quotation
"Science is a Faustian bargain"
and the following figure–
Change
From a short story by
the above Princess:
"'I don't even think she would have wanted to change you. But she for sure did not want to change herself. And her values were simply a part of her.' It was true, too. I would even go so far as to say that they were her basis, if you think about her as a geometrical body. That's what they couldn't understand, because in this age of the full understanding for stretches of values in favor of self-realization of any kind, it was a completely foreign concept."
To make this excellent metaphor mathematically correct,
change "geometrical body" to "space"… as in
"For Princeton's Class of 2007"—
Review of a 2004 production of a 1972 Tom Stoppard play, "Jumpers"–
Related material:
Knight Moves (Log24, Jan. 16),
Kindergarten Theology (St. Bridget's Day, 2008),
and
(Click on image for details.)
Comments Off on Saturday February 23, 2008
Sunday, November 20, 2005
Sunday November 20, 2005
“There is a pleasantly discursive treatment of Pontius Pilate’s unanswered question ‘What is truth?'”
— H. S. M. Coxeter, 1987, introduction to Richard J. Trudeau’s remarks on the “Story Theory” of truth as opposed to the “Diamond Theory” of truth in The Non-Euclidean Revolution
“A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes*….”
— Richard J. Trudeau in
The Non-Euclidean Revolution
“‘Deniers’ of truth… insist that each of us is trapped in his own point of view; we make up stories about the world and, in an exercise of power, try to impose them on others.”
— Jim Holt in The New Yorker.
(Click on the box below.)
Exercise of Power:
Show that a white horse–
a figure not unlike the
symbol of the mathematics
publisher Springer–
is traced, within a naturally
arranged rectangular array of
polynomials, by the powers of x
modulo a polynomial
irreducible over a Galois field.
This horse, or chess knight–
“Springer,” in German–
plays a role in “Diamond Theory”
(a phrase used in finite geometry
in 1976, some years before its use
by Trudeau in the above book).
Related material
On this date:
In 1490, The White Knight
(Tirant lo Blanc 
)–
a major influence on Cervantes–
was published, and in 1910
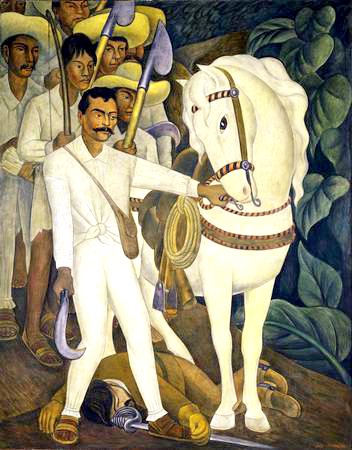
the Mexican Revolution began.
Illustration:
Zapata by Diego Rivera,
Museum of Modern Art,
New York
“First published in the Catalan language in Valencia in 1490…. Reviewing the first modern Spanish translation in 1969 (Franco had ruthlessly suppressed the Catalan language and literature), Mario Vargas Llosa hailed the epic’s author as ‘the first of that lineage of God-supplanters– Fielding, Balzac, Dickens, Flaubert, Tolstoy, Joyce, Faulkner– who try to create in their novels an all-encompassing reality.'”
Comments Off on Sunday November 20, 2005
Sunday, September 7, 2003
Sunday September 7, 2003
Horse Sense
Mathematicians are familiar with the emblem of Springer Verlag, the principal publisher of higher mathematics.
Ferdinand Springer, son of Julius Springer, founder of Springer Verlag, “was a passionate chess player and published a number of books on the subject. In 1881 this personal hobby and the name Springer led the company to adopt the knight in chess (in German, Springer) as its colophon.”
Hermann Hesse on a certain sort of serenity:
“I would like to say something more to you about cheerful serenity, the serenity of the stars and of the mind…. neither frivolity nor complacency; it is supreme insight and love, affirmation of all reality, alertness on the brink of all depths and abysses; it is a virtue of saints and of knights; it is indestructible and only increases with age and nearness to death. It is the secret of beauty and the real substance of all art.”
A saint and a knight, Jeanne d’Arc, was memorably portrayed by Milla Jovovich in The Messenger.
(Jovovich seems fated to play more-than-human characters in religious epics; see The Fifth Element.)
Another Springer, related to horses and to the accusation of witchcraft faced by Jeanne d’Arc, is Nancy Springer, the author of
Springer has written a number of books about horses, as well as other topics.
All of the above…. especially the parts having to do with mathematics and horses… was prompted by my redrawing today of a horse-shape within mathematics. See my entry The Eight of April 4, 2003, and the horse-figure redrawn at right below.
|
|
|
|
Believers in the story theory of truth may wish to relate the gifts of Jeanne d’Arc and of the girl in The Hex Witch of Seldom to the legend of Pegasus. See, for instance,
Plato, Pegasus, and the Evening Star.
For another connection between mathematics and horses, see Sangaku.
Comments Off on Sunday September 7, 2003