In the spirit of the Linz website in the previous post,
the title refers to New Yorker writer Adam Gopnik:
|
Tuesday, November 7, 2017
Polarities and Correlation
|
See also a search in this journal for Polarity + Correlation.
Friday, September 20, 2019
Garbage-Pail Kid
Comments Off on Garbage-Pail Kid
Saturday, November 21, 2015
The Zero System
For the title phrase, see Encyclopedia of Mathematics .
The zero system illustrated in the previous post*
should not be confused with the cinematic Zero Theorem .
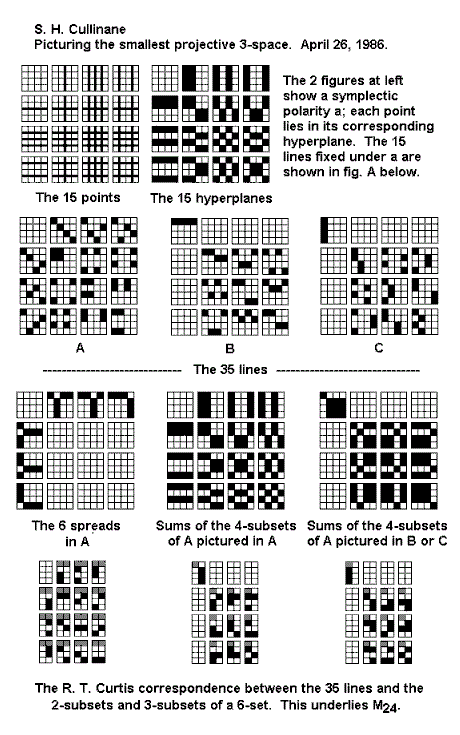
* More precisely, in the part showing the 15 lines fixed under
a zero-system polarity in PG(3,2). For the zero system
itself, see diamond-theorem correlation.
Comments Off on The Zero System
Friday, November 13, 2015
A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Note that the six anticommuting sets of Dirac matrices listed by Arfken
correspond exactly to the six spreads in the above complex of 15 projective
lines of PG(3,2) fixed under a symplectic polarity (the diamond theorem
correlation ). As I noted in 1986, this correlation underlies the Miracle
Octad Generator of R. T. Curtis, hence also the large Mathieu group.
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Related material:
The 6-set in my 1986 note above also appears in a 1996 paper on
the sixteen Dirac matrices by David M. Goodmanson —
Background reading:
Ron Shaw on finite geometry, Clifford algebras, and Dirac groups
(undated compilation of publications from roughly 1994-1995)—
Comments Off on A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Saturday, July 4, 2015
Context
Some context for yesterday's post on a symplectic polarity —
This 1986 note may or may not have inspired some remarks
of Wolf Barth in his foreword to the 1990 reissue of Hudson's
1905 Kummer's Quartic Surface .
See also the diamond-theorem correlation.
Comments Off on Context
Wednesday, February 25, 2015
Words and Images
The words: "symplectic polarity"—
The images:
The Natural Symplectic Polarity in PG(3,2)
Symmetry Invariance in a Diamond Ring
The Diamond-Theorem Correlation
Comments Off on Words and Images
Saturday, February 21, 2015
High and Low Concepts
Steven Pressfield on April 25, 2012:
What exactly is High Concept?
Let’s start with its opposite, low concept.
Low concept stories are personal,
idiosyncratic, ambiguous, often European.
“Well, it’s a sensitive fable about a Swedish
sardine fisherman whose wife and daughter
find themselves conflicted over … ”
ZZZZZZZZ.
Fans of Oslo artist Josefine Lyche know she has
valiantly struggled to find a high-concept approach
to the diamond theorem. Any such approach must,
unfortunately, reckon with the following low
(i.e., not easily summarized) concept —
The Diamond Theorem Correlation:
From left to right …
http://www.log24.com/log/pix14B/140824-Diamond-Theorem-Correlation-1202w.jpg
http://www.log24.com/log/pix14B/140731-Diamond-Theorem-Correlation-747w.jpg
http://www.log24.com/log/pix14B/140824-Picturing_the_Smallest-1986.gif
http://www.log24.com/log/pix14B/140806-ProjPoints.gif
For some backstory, see ProjPoints.gif and "Symplectic Polarity" in this journal.
Comments Off on High and Low Concepts
Thursday, July 31, 2014
Zero System
The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and
"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."
See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.
An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):
See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.
Comments Off on Zero System