
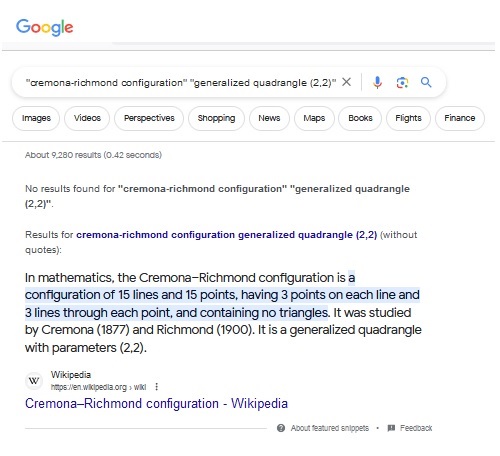
My own term "inscape" names a square incarnation of what is also
known as the "Cremona-Richmond configuration," the "generalized
quadrangle of order (2, 2)," and the "doily." —

My own term "inscape" names a square incarnation of what is also
known as the "Cremona-Richmond configuration," the "generalized
quadrangle of order (2, 2)," and the "doily." —

The "quantum kernel" of Koen Thas is a version of the incidence
structure — the Cremona-Richmond configuration — discussed
in the previous post, Doily vs. Inscape .
That post's inscape is, as noted there, an incarnation of the
abstract incidence structure. More generally, see incarnation
in this journal . . . In particular, from Michaelmas last year,
Annals of Mathematical Theology.
A somewhat more sophisticated "incarnation" example
related to the "inscape" concept —
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets

See also Numberland in this journal.
Wikipedia on what has been called “the doily” —
“The smallest non-trivial generalized quadrangle
is GQ(2,2), whose representation* has been dubbed
‘the doily’ by Stan Payne in 1973.”
A later publication relates the doily to grids.
From Finite Generalized Quadrangles , by Stanley E. Payne
and J. A. Thas, December 1983, at researchgate.net, pp. 81-82—
“Then the lines … define a 3×3 grid G (i.e. a grid
consisting of 9 points and 6 lines).”
. . . .
“So we have shown that the grid G can completed [sic ]
in a unique way to a grid with 8 lines and 16 points.”
. . . .
“A 4×4 grid defines a linear subspace
of the 2−(64,4,1) design, i.e. a 4×4 grid
together with the affine lines on it is AG(2,4).”
A more graphic approach from this journal —
Click the image for further details.
* This wording implies that GQ(2,2) has a unique
visual representation. It does not. See inscape .
The Ball-Weiner date above, 5 September 2011,
suggests a review of this journal on that date —
"Think of a DO NOT ENTER pictogram,
a circle with a diagonal slash, a type of ideogram.
It tells you what to do or not do, but not why.
The why is part of a larger context, a bigger picture."
— Customer review at Amazon.com
This passage was quoted here on August 10, 2009.
Also from that date:
The Sept. 5, 2011, Ball-Weiner paper illustrates the
"doily" view of the mathematical structure W(3,2), also
known as GQ(2,2), the Sp(4,2) generalized quadrangle.
(See Fig. 3.1 on page 33, exercise 13 on page 38, and
the answer to that exercise on page 55, illustrated by
Fig. 5.1 on page 56.)
For "another view, hidden yet true," of GQ(2,2),
see Inscape and Symplectic Polarity in this journal.
Powered by WordPress