Log24

The finite (i.e., Galois) field GF(16),
according to J. J. Seidel in 1974—

The same field according to Steven H. Cullinane in 1986,
in its guise as the affine 4-space over GF(2)—

The same field, again disguised as an affine 4-space,
according to John H. Conway and N.J.A. Sloane in
Sphere Packings, Lattices, and Groups , first published in 1988—

The above figure by Conway and Sloane summarizes, using
a 4×4 array, the additive vector-space structure of the finite
field GF(16).

This structure embodies what in Euclidean space is called
the parallelogram rule for vector addition—

(Thanks to June Lester for the 3D (uvw) part of the above figure.)

For the transition from this colored Euclidean hypercube
(used above to illustrate the parallelogram rule) to the
4×4 Galois space (illustrated by Cullinane in 1979 and
Conway and Sloane in 1988— or later… I do not have
their book’s first edition), see Diamond Theory in 1937,
Vertex Adjacency in a Tesseract and in a 4×4 Array,
Spaces as Hypercubes, and The Galois Tesseract.

For some related narrative, see tesseract  in this journal.

(This post has been added to finitegeometry.org.)

Update of August 9, 2013—

Coordinates for hypercube vertices derived from the
parallelogram rule in four dimensions were better
illustrated by Jürgen Köller in a web page archived in 2002.

Update of August 13, 2013—

The four basis vectors in the 2002 Köller hypercube figure
are also visible at the bottom of the hypercube figure on
page 7 of “Diamond Theory,” excerpts from a 1976 preprint
in Computer Graphics and Art , Vol. 2, No. 1, February 1977.
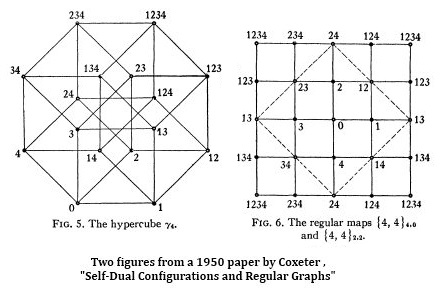
A predecessor:  Coxeter’s 1950 hypercube figure from
“Self-Dual Configurations and Regular Graphs.”