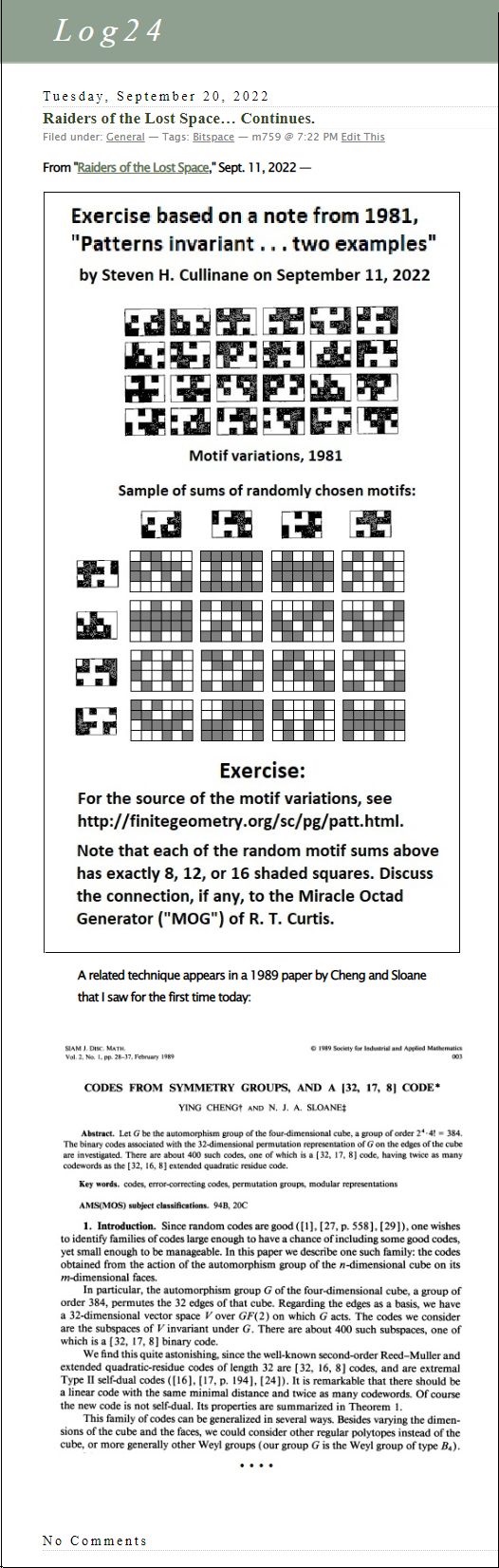
The above figures are from a 2016 post
on local and global symmetries.
Wednesday, March 8, 2023
Opening the Local/Global Seal
Wednesday, February 8, 2023
Local-Global lnduced Actions
See "Two Approaches to Local-Global Symmetry"
(this journal, Jan. 19, 2023), which discusses
local group actions on plane and solid graphic
patterns that induce global group actions.
See also local and global group actions of a different sort in
the July 11, 1986, note "Inner and Outer Group Actions."
This post was suggested by some remarks of Barry Mazur,
quoted in the previous post, on " Wittgenstein's 'language game,' "
Grothendieck, global views, local views and "locales."

Further reading on "locales" — Wikipedia, Pointless topology.
The word "locale" in mathematics was apparently* introduced by Isbell —
ISBELL, JOHN R. “ATOMLESS PARTS OF SPACES.”
Mathematica Scandinavica, vol. 31, no. 1, 1972, pp. 5–32.
JSTOR, http://www.jstor.org/stable/24490585.
* According to page 841 of . . .
Johnstone, P. (2001). "Elements of the History of Locale Theory."
Pp. 835–851 in: Aull, C.E., Lowen, R. (eds) Handbook of the
History of General Topology, Vol 3. Springer, Dordrecht.
Thursday, January 19, 2023
Two Approaches to Local-Global Symmetry
Last revised: January 20, 2023 @ 11:39:05
The First Approach — Via Substructure Isomorphisms —
From "Symmetry in Mathematics and Mathematics of Symmetry"
by Peter J. Cameron, a Jan. 16, 2007, talk at the International
Symmetry Conference, Edinburgh, Jan. 14-17, 2007 —
|
Local or global? "Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous * if every isomorphism between finite substructures of M can be extended to an automorphism of M ; in other words, 'any local symmetry is global.' " |
A related discussion of the same approach —
|
"The aim of this thesis is to classify certain structures
— Alice Devillers, |
The Wikipedia article Homogeneous graph discusses the local-global approach
used by Cameron and by Devillers.
For some historical background on this approach
via substructure isomorphisms, see a former student of Cameron:
Dugald Macpherson, "A survey of homogeneous structures,"
Discrete Mathematics , Volume 311, Issue 15, 2011,
Pages 1599-1634.
Related material:
Cherlin, G. (2000). "Sporadic Homogeneous Structures."
In: Gelfand, I.M., Retakh, V.S. (eds)
The Gelfand Mathematical Seminars, 1996–1999.
Gelfand Mathematical Seminars. Birkhäuser, Boston, MA.
https://doi.org/10.1007/978-1-4612-1340-6_2
and, more recently,
Gill et al., "Cherlin's conjecture on finite primitive binary
permutation groups," https://arxiv.org/abs/2106.05154v2
(Submitted on 9 Jun 2021, last revised 9 Jul 2021)
This approach seems to be a rather deep rabbit hole.
The Second Approach — Via Induced Group Actions —
My own interest in local-global symmetry is of a quite different sort.
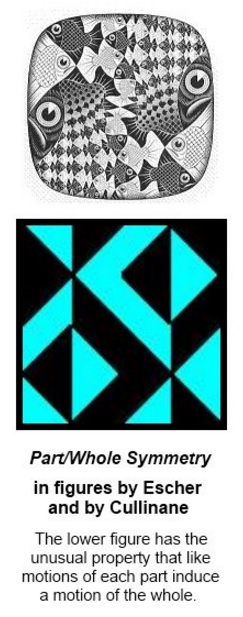
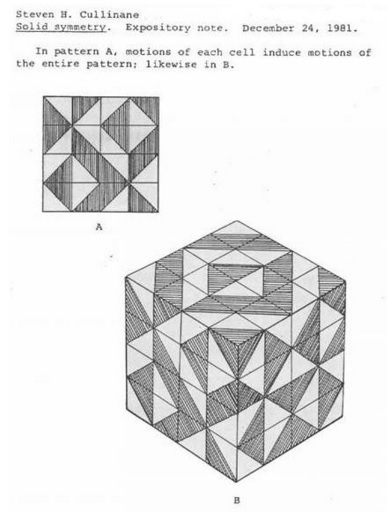
See properties of the two patterns illustrated in a note of 24 December 1981 —
Pattern A above actually has as few symmetries as possible
(under the actions described in the diamond theorem ), but it
does enjoy, as does patttern B, the local-global property that
a group acting in the same way locally on each part induces
a global group action on the whole .
* For some historical background on the term "homogeneous,"
see the Wikipedia article Homogeneous space.
Wednesday, April 27, 2016
Local and Global
Three notes on local symmetries
that induce global symmetries
From July 1, 2011 —
From November 5, 1981 —
From December 24, 1981 —
Monday, February 15, 2016
Global and Local
- "Dirty Laundry" (Henley, Danny Kortchmar) – 5:36
- "The Boys of Summer" (Mike Campbell, Henley) – 4:45
- "All She Wants to Do Is Dance" (Kortchmar) – 4:28
- "Not Enough Love in the World" (Henley, Kortchmar, Benmont Tench) – 3:54
- "Sunset Grill" (Henley, Kortchmar, Tench) – 6:22
- "The End of the Innocence" (Henley, Bruce Hornsby) – 5:14
- "The Last Worthless Evening" (John Corey, Henley, Stan Lynch) – 6:05
- "New York Minute" (Henley, Kortchmar, Jai Winding) – 6:34
- "I Will Not Go Quietly" (Henley, Kortchmar) – 5:41
- "The Heart of the Matter" (Campbell, Henley, J.D. Souther) – 5:21
- "The Garden of Allah" (Corey, Paul Gurian, Henley, Lynch) – 7:02
- "You Don't Know Me at All" (Corey, Henley, Lynch) – 5:36
- "Everybody Knows" (Leonard Cohen, Sharon Robinson) – 6:10
Sunday, February 14, 2016
Saturday, January 16, 2016
Global and Local
Two approaches to philosophy —
For a global perspective, see Cullinane College in this journal.
For a local perspective, see last night's post on a Pennsylvania philosopher.
Friday, January 15, 2016
Local and Global
The American Mathematical Society today —
|
George E. Weaver (1942-2015) Weaver was a philosophy professor at Bryn Mawr College before retiring in 2008. He had an interest in mathematics, among other fields, and taught discrete mathematics and mathematical logic at Bryn Mawr. A colleague said that Weaver "taught with passion and rigor, and cared deeply about his courses and the students. Students who studied with him had a deep respect and admiration for him." Weaver was an AMS member since 1972. Read more about his life in an obituary published in the Philadelphia Inquirer. |
Weaver reportedly died on December 4, 2015.

Related material in this journal on the date of Weaver's death —
|
Friday, December 4, 2015
Symbology for the Vatican Gift Shop
|
Saturday, September 26, 2015
Symbols, Local and Global
Local:

Global:
Photo by Brendan Smialowski today
Msgr. Mark Miles, the Pope's translator, at
Independence Hall in Philadelphia today.
What, if anything, the Church means by the symbol
he holds is not clear, but presumably its meaning,
if there is one, is more global than local.
Saturday, February 27, 2016
Thursday, January 11, 2024
Friday, September 15, 2023
For Fritz Leiber

See also this journal on the above April 27, 2016,
art date: "Local and Global."
Wednesday, April 27, 2016
Kubrick’s Rube
In memory of a culture jammer *—
* "Mr. Lyons … made a living partly by buying,
reconditioning and selling used cars." —
— Ben Ratliff in The New York Times this evening.
See also the previous post and, from Feb. 14 in
this journal, the phrase "more global than local."
Saturday, April 9, 2016
Thursday, February 4, 2016
Excellent Adventure
(Continued from Jan. 29)
For a literary tigress —
The image reproduced below was
revisited here on January 27, 2016.
That date was the reported date of death
for another literary tigress.
Wednesday, January 27, 2016
For the Sundance Kid
Sunday, December 6, 2015
American Hustlers
A Log24 Hanukkah from five years ago (Dec. 5, 2010) —
Related material: Oslo artist Josefine Lyche's
private Instagram photo of her adolescence with
a ZX Spectrum, and Symbols, Local and Global.
Friday, December 4, 2015
Symbology for the Vatican Gift Shop
(Title adapted from a 1996 Stone Temple Pilots album)
See Symbols, Local and Global (September 26, 2015),
Change Descends (April 11, 2014), and Altar (August 27, 2014).
Thursday, September 11, 2014
A Class by Itself
The American Mathematical Society yesterday:
Harvey Cohn (1923-2014)
Wednesday September 10th 2014
Cohn, an AMS Fellow and a Putnam Fellow (1942), died May 16 at the age of 90. He served in the Navy in World War II and following the war received his PhD from Harvard University in 1948 under the direction of Lars Ahlfors. He was a member of the faculty at Wayne State University, Stanford University, Washington University in St. Louis, the University of Arizona, and at City College of New York, where he was a distinguished professor. After retiring from teaching, he also worked for the NSA. Cohn was an AMS member since 1942.
Paid death notice from The New York Times , July 27, 2014:
COHN–Harvey. Fellow of the American Mathematical Society and member of the Society since 1942, died on May 16 at the age of 90. He was a brilliant Mathematician, an adoring husband, father and grandfather, and faithful friend and mentor to his colleagues and students. Born in New York City in 1923, Cohn received his B.S. degree (Mathematics and Physics) from CCNY in 1942. He received his M.S. degree from NYU (1943), and his Ph.D. from Harvard (1948) after service in the Navy (Electronic Technicians Mate, 1944-46). He was a member of Phi Beta Kappa (Sigma Chi), won the William Lowell Putnam Prize in 1942, and was awarded the Townsend Harris Medal in 1972. A pioneer in the intensive use of computers in an innovative way in a large number of classical mathematical problems, Harvey Cohn held faculty positions at Wayne State University, Stanford, Washington University Saint Louis (first Director of the Computing Center 1956-58), University of Arizona (Chairman 1958-1967), University of Copenhagen, and CCNY (Distinguished Professor of Mathematics). After his retirement from teaching, he worked in a variety of capacities for the National Security Agency and its research arm, IDA Center for Computing Sciences. He is survived by his wife of 63 years, Bernice, of Laguna Woods, California and Ft. Lauderdale, FL, his son Anthony, daughter Susan Cohn Boros, three grandchildren and one great-granddaughter.
— Published in The New York Times on July 27, 2014
See also an autobiographical essay found on the web.
None of the above sources mention the following book, which is apparently by this same Harvey Cohn. (It is dedicated to "Tony and Susan.")

Advanced Number Theory, by Harvey Cohn
Courier Dover Publications, 1980 – 276 pages
(First published by Wiley in 1962 as A Second Course in Number Theory )
Publisher's description:
" 'A very stimulating book … in a class by itself.'— American Mathematical Monthly
Advanced students, mathematicians and number theorists will welcome this stimulating treatment of advanced number theory, which approaches the complex topic of algebraic number theory from a historical standpoint, taking pains to show the reader how concepts, definitions and theories have evolved during the last two centuries. Moreover, the book abounds with numerical examples and more concrete, specific theorems than are found in most contemporary treatments of the subject.
The book is divided into three parts. Part I is concerned with background material — a synopsis of elementary number theory (including quadratic congruences and the Jacobi symbol), characters of residue class groups via the structure theorem for finite abelian groups, first notions of integral domains, modules and lattices, and such basis theorems as Kronecker's Basis Theorem for Abelian Groups.
Part II discusses ideal theory in quadratic fields, with chapters on unique factorization and units, unique factorization into ideals, norms and ideal classes (in particular, Minkowski's theorem), and class structure in quadratic fields. Applications of this material are made in Part III to class number formulas and primes in arithmetic progression, quadratic reciprocity in the rational domain and the relationship between quadratic forms and ideals, including the theory of composition, orders and genera. In a final concluding survey of more recent developments, Dr. Cohn takes up Cyclotomic Fields and Gaussian Sums, Class Fields and Global and Local Viewpoints.
In addition to numerous helpful diagrams and tables throughout the text, appendices, and an annotated bibliography, Advanced Number Theory also includes over 200 problems specially designed to stimulate the spirit of experimentation which has traditionally ruled number theory."
User Review –
"In a nutshell, the book serves as an introduction to Gauss' theory of quadratic forms and their composition laws (the cornerstone of his Disquisitiones Arithmeticae) from the modern point of view (ideals in quadratic number fields). I strongly recommend it as a gentle introduction to algebraic number theory (with exclusive emphasis on quadratic number fields and binary quadratic forms). As a bonus, the book includes material on Dirichlet L-functions as well as proofs of Dirichlet's class number formula and Dirichlet's theorem in primes in arithmetic progressions (of course this material requires the reader to have the background of a one-semester course in real analysis; on the other hand, this material is largely independent of the subsequent algebraic developments).
Better titles for this book would be 'A Second Course in Number Theory' or 'Introduction to quadratic forms and quadratic fields'. It is not a very advanced book in the sense that required background is only a one-semester course in number theory. It does not assume prior familiarity with abstract algebra. While exercises are included, they are not particularly interesting or challenging (if probably adequate to keep the reader engaged).
While the exposition is *slightly* dated, it feels fresh enough and is particularly suitable for self-study (I'd be less likely to recommend the book as a formal textbook). Students with a background in abstract algebra might find the pace a bit slow, with a bit too much time spent on algebraic preliminaries (the entire Part I—about 90 pages); however, these preliminaries are essential to paving the road towards Parts II (ideal theory in quadratic fields) and III (applications of ideal theory).
It is almost inevitable to compare this book to Borevich-Shafarevich 'Number Theory'. The latter is a fantastic book which covers a large superset of the material in Cohn's book. Borevich-Shafarevich is, however, a much more demanding read and it is out of print. For gentle self-study (and perhaps as a preparation to later read Borevich-Shafarevich), Cohn's book is a fine read."
Thursday, December 5, 2013
Friday, February 17, 2012
Pregeometry and Finite Geometry
Today's previous post, on the Feb. 2012 Scientific American
article "Is Space Digital?", suggested a review of a notion
that the theoretical physicist John Archibald Wheeler called
pregeometry .
From a paper on that topic—
"… the idea that geometry should constitute
'the magic building material of the universe'
had to collapse on behalf of what Wheeler
has called pregeometry (see Misner et al. 1973,
pp. 1203-1212; Wheeler 1980), a somewhat
indefinite term which expresses “a combination
of hope and need, of philosophy and physics
and mathematics and logic” (Misner et al. 1973,
p. 1203)."
— Jacques Demaret, Michael Heller, and
Dominique Lambert, "Local and Global Properties
of the World," preprint of paper published in
Foundations of Science 2 (1): 137-176
Misner, C. W., Thorne, K. S. and Wheeler, J. A.
1973, Gravitation , W.H. Freeman and Company:
San Francisco.
Wheeler, J.A. 1980, "Pregeometry: Motivations
and Prospects," in: Quantum Theory and Gravitation ,
ed. A.R. Marlow, Academic Press: New York, pp. 1-11.
Some related material from pure mathematics—
Click image for further details.
Tuesday, June 29, 2010
Kind of Bleu
Click for a Global Partners story.
Related material:
"We want to promote the vitality of mathematics
by playing an increasingly active role in political affairs."
— Princeton Class Notes, Jan. 27, 1999, remark by Felix E. Browder,
then president-elect of the American Mathematical Society (AMS).
See also web pages on Browder's brothers
William (also an AMS president, 1989-1990)
and Andrew and their father Earl.
Earl was General Secretary of
the Communist Party USA from 1930 to 1944.
Princeton Class Notes on the Browders— "The senior Browder 'discouraged me and my two brothers from taking an active part in politics, but strongly encouraged our intellectual interests.' That all three brothers became mathematicians– the others are Princeton professor William Browder '58 (a former president of the AMS) and Brown professor Andrew Browder– is an outcome for which Felix Browder 'can offer no rational explanation.'"
"As a trusted partner, we do more than consult and train.
We add a new dimension to our client’s thinking…."
Thursday, September 27, 2007
Thursday September 27, 2007
continues:
Classics 101 —

(See September 15. )
"The communication
of the dead is tongued with fire
beyond the language of the living."
— T. S. Eliot, Four Quartets
The Boston Globe,
Tuesday, Sept. 25, 2007-
Psychiatrist treated veterans
using Homer
"When Boston psychiatrist Jonathan Shay wanted to understand the psychological toll of the Vietnam War on the veterans he treated, he turned to the 'Iliad' and the 'Odyssey.'
The classical Greek epics perfectly encapsulate the mental damage of combat, said Shay, who works for the Department of Veterans Affairs in Boston….
Today, the John D. and Catherine T. MacArthur Foundation will announce that Shay, 65, has been selected as a 2007 MacArthur fellow 'for his work in using literary parallels from Homer's "Iliad" and "Odyssey" to treat combat trauma suffered by Vietnam veterans.'….
'I was hearing elements of the story of Achilles over and over again,' Shay said.
Achilles, the hero of the 'Iliad,' is mistreated by his commander, who takes a girl, a prize of war, from him. Achilles is also tormented by the loss of his best friend in the Trojan War. With his ethical universe upended, he goes berserk.
Soon, Shay began to work on his first book, 'Achilles in Vietnam: Combat Trauma and the Undoing of Character.'
In the book, he interspersed the story of Achilles with examples of his patients' losses and contentious relationships with their commanders in Vietnam to illustrate some of the causes of the troops' psychological wounds."
The first word of the 'Iliad,'
Menin, is written in Greek
on Professor Silk's blackboard
in the photo at top.
It means "wrath."
Related material:
The wrath of a Vietnam
veteran, portrayed by
Ed Harris, in the film
"The Human Stain,"
and a calmer Harris in
the illustration below,
from Log24, Oct. 8, 2005:
A History of Death

Adapted from
the film
"A History of Violence"
Thursday, June 21, 2007
Thursday June 21, 2007
Let No Man
Write My Epigraph
"His graceful accounts of the Bach Suites for Unaccompanied Cello illuminated the works’ structural logic as well as their inner spirituality."
—Allan Kozinn on Mstislav Rostropovich in The New York Times, quoted in Log24 on April 29, 2007
"At that instant he saw, in one blaze of light, an image of unutterable conviction…. the core of life, the essential pattern whence all other things proceed, the kernel of eternity."
— Thomas Wolfe, Of Time and the River, quoted in Log24 on June 9, 2005
"… the stabiliser of an octad preserves the affine space structure on its complement, and (from the construction) induces AGL(4,2) on it. (It induces A8 on the octad, the kernel of this action being the translation group of the affine space.)"
— Peter J. Cameron, "The Geometry of the Mathieu Groups" (pdf)
"… donc Dieu existe, réponse!"
what the mandala really is:
'Formation, Transformation,
Eternal Mind's eternal recreation'"
(Faust, Part Two, as
quoted by Jung in
Memories, Dreams, Reflections)

"Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen.
The drawing is one of
many by George Gamow
illustrating the script."
— Physics Today
'To meet someone' was his enigmatic answer. 'To search for the stone that the Great Architect rejected, the philosopher's stone, the basis of the philosophical work. The stone of power. The devil likes metamorphoses, Corso.'"
— The Club Dumas, basis for the Roman Polanski film "The Ninth Gate" (See 12/24/05.)
"Pauli linked this symbolism
with the concept of automorphism."
— The Innermost Kernel
(previous entry)
And from
"Symmetry in Mathematics
and Mathematics of Symmetry"
(pdf), by Peter J. Cameron,
a paper presented at the
International Symmetry Conference,
Edinburgh, Jan. 14-17, 2007,
we have
The Epigraph–

(Here "whatever" should
of course be "whenever.")
Also from the
Cameron paper:
|
Local or global?
Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous if every isomorphism between finite substructures of M can be extended to an automorphism of M; in other words, "any local symmetry is global." |
Some Log24 entries
related to the above politically
(women in mathematics)–
Global and Local:
One Small Step
and mathematically–
Structural Logic continued:
Structure and Logic (4/30/07):
This entry cites
Alice Devillers of Brussels–

"The aim of this thesis
is to classify certain structures
which are, from a certain
point of view, as homogeneous
as possible, that is which have
as many symmetries as possible."
"There is such a thing
as a tesseract."
Friday, May 19, 2006
Friday May 19, 2006
Meeting at Princeton
From May 15 through May 26, there is a women-only meeting on zeta functions at the Institute for Advanced Study in Princeton, New Jersey. Today’s activities:
| Breakfast (Dining Hall) | |
| T-shirt Sale, Harry’s Bar – Dining Hall | |
| Depart for Princeton University (talks, lunch, campus and art museum tour, and dinner) |
|
From Log24, July 27, 2003: “…my despair with words as instruments of communion is often near total.” — Charles Small, Harvard ’64 25th Anniversary Report, 1989 (See 11/21/02).
|
|
|
Lucero |
See also |
Women’s History Month–
Global and Local: One Small Step
Friday, March 3, 2006
Friday March 3, 2006
Women's History Month continues.
One Small Step
Audrey Terras, University of Maryland '64:
We cannot discuss the proof here as it requires some knowledge of zeta functions of curves over finite fields.
Charles Small, Harvard '64:
The moral is that the zeta function exhibits a subtle connection between the "global" (topological, characteristic 0) nature of the curve and its "local" (diophantine, characteristic p for all but finitely many "bad" primes p) behaviour. The full extent of this connection only becomes apparent in the context of varieties more general than curves….

"Some friends of mine
are in this band…."
— David Auburn, "Proof"
"Women and Mathematics
is a joint program of
the Institute for Advanced Study
and Princeton University."
— School of Mathematics,
1 Einstein Drive,
Princeton, New Jersey