triangle square mapping « Search Results

Thursday, February 13, 2020

Square-Triangle Mappings: The Continuous Case

Filed under: General — Tags: Figurate Geometry, Triangles — m759 @ 12:00 pm

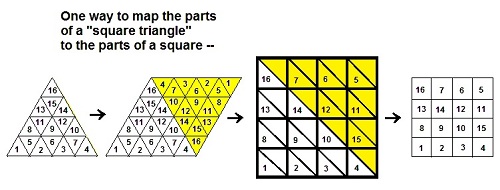
On Feb. 11, Christian Lawson-Perfect posed an interesting question
about mappings between square and triangular grids:

For the same question posed about non -continuous bijections,
see "Triangles are Square."

I posed the related non– continuous question in correspondence in
the 1980's, and later online in 2012. Naturally, I wondered in the
1980's about the continuous question and conformal mappings,
but didn't follow up that line of thought.

Perfect last appeared in this journal on May 20, 2014,
in the HTML title line for the link "offensive."

Comments Off

Monday, March 11, 2024

Fundamental Figurate Geometry: Triangle Subdivision

Filed under: General — Tags: Congruent Subarrays, Congruent Subsets, Pie — m759 @ 5:41 am

Click to enlarge.

See as well "Triangles are Square," at
http://finitegeometry.org/sc/16/trisquare.html.

(I happened to find the Basu-Owen paper tonight
via a Google image search for "congruent subsets" . . .
as opposed to the "congruent subarrays" of
the previous post.)

Update of 3:54 PM ET Monday, March 11, 2024 —

This Stanford version of my square-to-triangle mapping
is the first publication in a new Zenodo community —

Citation for the research note:
Cullinane, Steven H. (2024). Fundamental Figurate Geometry:
Triangle Subdivision (Version 2). Zenodo.
https://doi.org/10.5281/zenodo.10822848
(latest version as of March 15, 2024)

Comments Off

Thursday, July 7, 2022

Square-Triangle Geometry

Filed under: General — Tags: Triangle.graphics — m759 @ 3:29 pm

Each of the above mappings is, in some sense, "natural."

Is there any general order-n natural square-to-triangle mapping?

Comments Off

Sunday, June 12, 2022

Triangle.graphics, 2012-2022

Filed under: General — Tags: Figurate Geometry, Triangle.graphics, Trilateral — m759 @ 2:13 am

Comments Off

Saturday, January 18, 2014

The Triangle Relativity Problem

Filed under: General,Geometry — Tags: Coordinatization, Figurate Geometry, Triangle.graphics, Triangles — m759 @ 5:01 pm

A sequel to last night's post The 4×4 Relativity Problem —

In other words, how should the triangle corresponding to
the above square be coordinatized ?

See also a post of July 8, 2012 — "Not Quite Obvious."

Context — "Triangles Are Square," a webpage stemming
from an American Mathematical Monthly item published
in 1984.

Comments Off

Monday, July 16, 2012

Mapping Problem continued

Filed under: General,Geometry — Tags: Figurate Geometry, Springer — m759 @ 2:56 am

Another approach to the square-to-triangle
mapping problem (see also previous post)—

IMAGE- Triangular analogs of the hyperplanes in the square model of PG(3,2)

For the square model referred to in the above picture, see (for instance)

Coordinates for the 16 points in the triangular arrays
of the corresponding affine space may be deduced
from the patterns in the projective-hyperplanes array above.

This should solve the inverse problem of mapping,
in a natural way, the triangular array of 16 points
to the square array of 16 points.

Update of 9:35 AM ET July 16, 2012:

Note that the square model's 15 hyperplanes S
and the triangular model's 15 hyperplanes T —

— share the following vector-space structure —

0	c	d	c + d
a	a + c	a + d	a + c + d
b	b + c	b + d	b + c + d
a + b	a + b + c	a + b + d	a + b + c + d

(This vector-space a b c d diagram is from
Chapter 11 of Sphere Packings, Lattices
and Groups , by John Horton Conway and
N. J. A. Sloane, first published by Springer
in 1988.)

Comments Off

Sunday, July 15, 2012

Mapping Problem

Filed under: General,Geometry — Tags: Figurate Geometry — m759 @ 4:00 pm

A trial solution to the
square-to-triangle mapping problem—

IMAGE- Mapping of square array to triangular array based on gnomons

Problem: Is there any good definition of "natural"
square-to-triangle mappings according to which
the above mapping is natural (or, for that matter,
un-natural)?

Comments Off

Monday, January 16, 2012

Mapping Problem

Filed under: General,Geometry — Tags: Figurate Geometry, Finite Geometry, Triangles — m759 @ 5:10 pm

Thursday's post Triangles Are Square posed the problem of
finding "natural" maps from the 16 subsquares of a 4×4 square
to the 16 equilateral subtriangles of an edge-4 equilateral triangle.

Here is a trial solution of the inverse problem—

(Click for larger version.)

Exercise— Devise a test for "naturality" of
such mappings and apply it to the above.

Comments Off

Thursday, January 12, 2012

Triangles Are Square

Filed under: General,Geometry — Tags: Figurate Geometry, Finite Geometry, Triangles — m759 @ 11:30 am

Coming across John H. Conway's 1991*
pinwheel triangle decomposition this morning—

— suggested a review of a triangle decomposition result from 1984:

IMAGE- Triangle and square, each with 16 parts

Figure A

(Click the below image to enlarge.)

The above 1985 note immediately suggests a problem—

What mappings of a square with c ² congruent parts
to a triangle with c ² congruent parts are "natural"?**

(In Figure A above, whether the 322,560 natural transformations
of the 16-part square map in any natural way to transformations
of the 16-part triangle is not immediately apparent.)

* Communicated to Charles Radin in January 1991. The Conway
decomposition may, of course, have been discovered much earlier.

** Update of Jan. 18, 2012— For a trial solution to the inverse
problem, see the "Triangles are Square" page at finitegeometry.org.

Comments Off

Tuesday, September 19, 2023

Figurate Geometry

Filed under: General — Tags: Figurate Geometry — m759 @ 9:18 am

The above title for a new approach to finite geometry
was suggested by the old phrase "figurate numbers."

See other posts in this journal now tagged Figurate Geometry.

Update of 10 AM ET on Sept. 19, 2023 —

Related material from social media:

Update of 10:30 AM ET Sept. 19 —

A related topic from figurate geometry:

The square-to-triangle mapping problem.

Comments Off

Saturday, August 6, 2016

Mystic Correspondence:

Filed under: General,Geometry — Tags: The Finkelstein Talisman — m759 @ 12:00 pm

The Cube and the Hexagram

The above illustration, by the late Harvey D. Heinz,
shows a magic cube* and a corresponding magic
hexagram, or Star of David, with the six cube faces
mapped to the six hexagram lines and the twelve
cube edges mapped to the twelve hexagram points.
The eight cube vertices correspond to eight triangles
in the hexagram (six small and two large).

Exercise: Is this noteworthy mapping** of faces to lines,
edges to points, and vertices to triangles an isolated
phenomenon, or can it be viewed in a larger context?

* See the discussion at magic-squares.net of
"perimeter-magic cubes"

** Apparently derived from the Cube + Hexagon figure
discussed here in various earlier posts. See also
"Diamonds and Whirls," a note from 1984.

Comments Off

Sunday, July 8, 2012

Not Quite Obvious

Filed under: General,Geometry — Tags: Figurate Geometry, Triangle.graphics — m759 @ 11:00 am

"That n² points fall naturally into a triangular array
is a not-quite-obvious fact which may have applications…
and seems worth stating more formally."

— Steven H. Cullinane, letter in the
American Mathematical Monthly 1985 June-July issue

If the ancient Greeks had not been distracted by
investigations of triangular (as opposed to square )
numbers, they might have done something with this fact.

A search for occurrences of the phrase

"n2 [i.e., n² ] congruent triangles"

indicates only fairly recent (i.e., later than 1984) results.*

Some related material, updated this morning—

This suggests a problem—

What mappings of a square array of n ² points to
a triangular array of n ² points are "natural"?

In the figure above, whether
the 322,560 natural permutations
of the square's 16 points
map in any natural way to
permutations of the triangle's 16 points
is not immediately apparent.

* Update of July 15, 2012 (11:07 PM ET)—

Theorem on " rep-n ²" (Golomb's terminology)
triangles from a 1982 book—

IMAGE- Theorem (12.3) on Golomb and 'rep-k^2' triangles in book published in 1982-- 'Transformation Geometry,' by George Edward Martin

Comments Off

Friday, February 25, 2011

Diamond Theorem Exposition

Filed under: General,Geometry — m759 @ 11:00 pm

"THE DIAMOND THEOREM AND QUILT PATTERNS
Victoria Blumen, Mathematics, Junior, Benedictine University
Tim Comar, Benedictine University
Mathematics
Secondary Source Research

Let D be a 4 by 4 block quilt shape, where each of the 16 square blocks is consists of [sic ] two triangles, one of which is colored red and the other of which is colored blue. Let G: D -> D_g be a mapping of D that interchanges a pair of columns, rows, or quadrants of D. The diamond theorem states that G(D) = D_g has either ordinary or color-interchange symmetry. In this talk, we will prove the diamond theorem and explore symmetries of quilt patterns of the form G(D)."

Exercise— Correct the above statement of the theorem.

Background— This is from a Google search result at about 10:55 PM ET Feb. 25, 2011—

[DOC] THE DIAMOND THEOREM AND QUILT PATTERNS – acca.elmhurst.edu
File Format: Microsoft Word – 14 hours ago –
Let G: D -> D_g be a mapping of D that interchanges a pair of columns, rows, or quadrants of D. The diamond theorem states that G(D) = D_g has either …
acca.elmhurst.edu/…/victoria_blumen9607_
THE%20DIAMOND%20THEOREM%20AND%20QUILT%20PATTERNS…

The document is from a list of mathematics abstracts for the annual student symposium of the ACCA (Associated Colleges of the Chicago Area) held on April 10, 2010.

Update of Feb. 26— For a related remark quoted here on the date of the student symposium, see Geometry for Generations.

Comments Off