
For art more closely related to the title "Alpha and Omega,"
see a different view of the above Hoyersten exhibition.

For art more closely related to the title "Alpha and Omega,"
see a different view of the above Hoyersten exhibition.
Version from "The Avengers" (2012) —
Version from Josefine Lyche (2009) —
See also this journal on the date that the above Avengers video was uploaded.
Today's news from Oslo suggests a review—
The circular sculpture in the foreground
is called by the artist "The Omega Point."
This has been described as
"a portal that leads in or out of time and space."
Some related philosophical remarks—
And I'd like to thank the heroine of Finale —
* The title refers to a 2009 sculpture by Lyche —

For a related shape, see today's noon post.
The "compact key to universal wisdom" passage in the previous post seemed
too well written to be the work of an anonymous webforum author.
Here is a slightly expanded version—
Throughout history mystics and philosophers have sought
a compact key to universal wisdom, a finite formula or text
that would provide the answer to every question. The use of
the Bible, the Koran and the I Ching for divination and the
tradition of the secret books of Hermes Trismegistus and the
medieval Jewish Cabala exemplify this belief or hope. Such
sources of universal wisdom are traditionally protected from
casual use by being difficult to find as well as difficult to un-
derstand and dangerous to use, tending to answer more quest-
ions and deeper ones than the searcher wishes to ask. The
esoteric book is, like God, simple yet undescribable. It is om-
niscient, and it transforms all who know it. The use of clas-
sical texts to foretell mundane events is considered supersti-
tious nowadays, yet in another sense science is in quest of its
own Cabala, a concise set of natural laws that would explain
all phenomena. In mathematics, where no set of axioms can
hope to prove all true statements, the goal might be a concise
axiomatization of all "interesting" true statements.
Ω is in many senses a Cabalistic number. It can be known
of through human reason, but not known. To know it in detail
one must accept its uncomputable sequence of digits on faith,
like words of a sacred text.
This is Martin Gardner's* and Charles H. Bennett's
revised version of a passage from Bennett's paper
"On Random and Hard-to-Describe Numbers," 1979.
The original passage from Bennett's paper—
Throughout history mystics and philosophers have sought a compact key to
universal wisdom, a finite formula or text which, when known and understood,
would provide the answer to every question. The Bible, the Koran, the mythical
secret books of Hermes Trismegistus, and the medieval Jewish Cabala have
been so regarded. Sources of universal wisdom are traditionally protected from
casual use by being hard to find, hard to understand when found, and dangerous
to use, tending to answer more and deeper questions than the user wishes to
ask. Like God the esoteric book is simple yet undescribable, omniscient, and
transforms all who know It. The use of classical texts to fortell [sic] mundane events
is considered superstitious nowadays, yet, in another sense, science is in quest of
its own Cabala, a concise set of natural laws which would explain all phenomena.
In mathematics, where no set of axioms can hope to prove all true statements,
the goal might be a concise axiomatization of all "interesting" true statements.
Ω is in many senses a Cabalistic number. It can be known of, but not known,
through human reason. To know it in detail, one would have to accept its un-
computable digit sequence on faith, like words of a sacred text.
The Bennett paper deals with Gregory Chaitin's concept of an "Omega Number."
I prefer the Omega of Josefine Lyche—
See also All Hallows' Eve, 2002.
* Martin Gardner's Mathematical Games column
"The Random Number Omega Bids Fair to Hold the Mysteries of the Universe,"
Scientific American, November 1979, 241(5), pp. 20–34.
The column is reprinted as "Chaitin's Omega," Ch. 21, pp. 307-319 in the
collection of Gardner's columns titled Fractal Music, Hypercards and More,
W.H. Freeman & Co., 1991
The Oslo Version and The Lyche Omega
Those who prefer more traditional art
may consult The Portal Project.
An image from the opening of the Netflix series “Locke & Key” —

See also Omega in this journal.
“The key is the cocktail that begins the proceedings.”
– Brian Harley, Mate in Two Moves


“The message was clear: having a finite frame of reference
creates the illusion of a world, but even the reference frame itself
is an illusion. Observers create reality, but observers aren’t real.
There is nothing ontologically distinct about an observer, because
you can always find a frame in which that observer disappears:
the frame of the frame itself, the boundary of the boundary.”
— Amanda Gefter in 2014, quoted here on Mayday 2020.
See as well the previous post.
The title was suggested by the "Crystal Cult" installations
of Oslo artist Josefine Lyche and by a post of May 30 —
|
Thursday, May 30, 2019 Filed under: General — m759 @ 8:02 PM Edit This Jeff Nichols, director of Midnight Special (2016) —
"When asked about the film's similarities to See also Jung's four-diamond figure and the previous post. |
Writers of fiction are, of course, also dabblers in the collective unconscious.
For instance . . .
A 1971 British paperback edition of The Dreaming Jewels,
a story by Theodore Sturgeon (first version published in 1950):

The above book cover, together with the Death Valley location
Zabriskie Point, suggests . . .
Those less enchanted by the collective unconscious may prefer a
different weblog's remarks on the same date as the above Borax post . . .
See also "Romancing the Omega" —
Related mathematics — Guitart in this journal —
See also Weyl + Palermo in this journal —
In Like Flynn
From the Wall Street Journal site Friday evening—
| ESSAY September 21, 2012, 9:10 p.m. ET
Are We Really Getting Smarter? Americans’ IQ scores have risen steadily over the past century.
|
No, thank you. I prefer the ninth configuration as is—

Why? See Josefine Lyche’s art installation “Grids, you say?“
Her reference there to “High White Noon” is perhaps
related to the use of that phrase in this journal.
The phrase is from a 2010 novel by Don DeLillo.
See “Point Omega,” as well as Lyche’s “Omega Point,”
in this journal.
The Wall Street Journal author above, James R. Flynn (born in 1934),
“is famous for his discovery of the Flynn effect, the continued
year-after-year increase of IQ scores in all parts of the world.”
—Wikipedia
His son Eugene Victor Flynn is a mathematician, co-author
of the following chapter on the Kummer surface— 
For use of the Kummer surface in Buddhist metaphysics, see last night’s
post “Occupy Space (continued)” and the letters of Nanavira Thera from the
late 1950s at nanavira.blogspot.com.
These letters, together with Lyche’s use of the phrase “high white noon,”
suggest a further quotation—
You know that it would be untrue
You know that I would be a liar
If I was to say to you
Girl, we couldn’t get much higher
See also the Kummer surface at the web page Configurations and Squares.
we are just like a couple of tots…
— Sinatra
Born 1973 in Bergen. Lives and works in Oslo.
Education
2000 – 2004 National Academy of Fine Arts, Oslo
1998 – 2000 Strykejernet Art School, Oslo, NO
1995 – 1998 Philosophy, University of Bergen
University of Bergen—
|
It might therefore seem that the idea of digital and analogical systems as rival fundaments to human experience is a new suggestion and, like digital technology, very modern. In fact, however, the idea is as old as philosophy itself (and may be much older). In his Sophist, Plato sets out the following ‘battle’ over the question of ‘true reality’: What we shall see is something like a battle of gods and giants going on between them over their quarrel about reality [γιγαντομαχία περì της ουσίας] ….One party is trying to drag everything down to earth out of heaven and the unseen, literally grasping rocks and trees in their hands, for they lay hold upon every stock and stone and strenuously affirm that real existence belongs only to that which can be handled and offers resistance to the touch. They define reality as the same thing as body, and as soon as one of the opposite party asserts that anything without a body is real, they are utterly contemptuous and will not listen to another word. (…) Their adversaries are very wary in defending their position somewhere in the heights of the unseen, maintaining with all their force that true reality [την αληθινήν ουσίαν] consists in certain intelligible and bodiless forms. In the clash of argument they shatter and pulverize those bodies which their opponents wield, and what those others allege to be true reality they call, not real being, but a sort of moving process of becoming. On this issue an interminable battle is always going on between the two camps [εν μέσω δε περι ταυτα απλετος αμφοτέρων μάχη τις (…) αει συνέστηκεν]. (…) It seems that only one course is open to the philosopher who values knowledge and truth above all else. He must refuse to accept from the champions of the forms the doctrine that all reality is changeless [and exclusively immaterial], and he must turn a deaf ear to the other party who represent reality as everywhere changing [and as only material]. Like a child begging for 'both', he must declare that reality or the sum of things is both at once [το όν τε και το παν συναμφότερα] (Sophist 246a-249d). The gods and the giants in Plato’s battle present two varieties of the analog position. Each believes that ‘true reality’ is singular, that "real existence belongs only to" one side or other of competing possibilities. For them, difference and complexity are secondary and, as secondary, deficient in respect to truth, reality and being (την αληθινήν ουσίαν, το όν τε και το παν). Difference and complexity are therefore matters of "interminable battle" whose intended end for each is, and must be (given their shared analogical logic), only to eradicate the other. The philosophical child, by contrast, holds to ‘both’ and therefore represents the digital position where the differentiated two yet belong originally together. Here difference, complexity and systematicity are primary and exemplary. It is an unfailing mark of the greatest thinkers of the tradition, like Plato, that they recognize the digital possibility and therefore recognize the principal difference of it from analog possibilities.
— Cameron McEwen, "The Digital Wittgenstein," |
* See that phrase in this journal.
See Notes for a Haiku.
Related material—
A novel published on Groundhog Day, 2010—
— as well as Conceptual Art, Josefine Lyche's
"Grids, You Say?" and The Speed of Thought.

For Fischer's fellow database enthusiast Codd, see Cross-Referenced (April 24, 2003).
For Birtel's fellow pseudoscience enthusiast Frank Tipler, see the artist's comment linked to in Romancing the Omega (April 19, 2011)—
"Omega Point" is a term used by mathematical physicist Frank Tipler for what he maintains is the ultimate fate of the universe required by the laws of physics."
For Norway's Niels Henrik Abel (1802-1829)
on his birthday, August Fifth
(6 PM Aug. 4, Eastern Time, is 12 AM Aug. 5 in Oslo.)
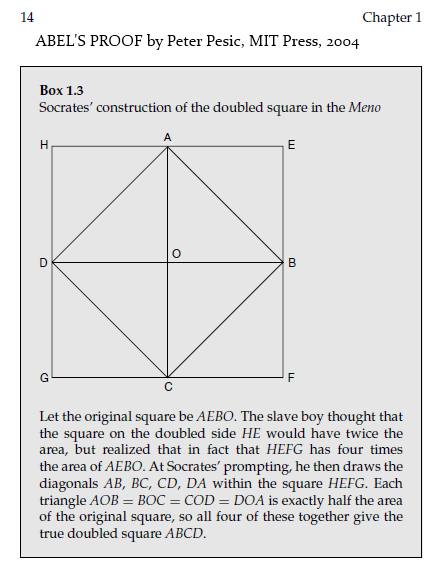
Plato's Diamond
The above version by Peter Pesic is from Chapter I of his book Abel's Proof , titled "The Scandal of the Irrational." Plato's diamond also occurs in a much later mathematical story that might be called "The Scandal of the Noncontinuous." The story—
Paradigms"These passages suggest that the Form is a character or set of characters common to a number of things, i.e. the feature in reality which corresponds to a general word. But Plato also uses language which suggests not only that the forms exist separately (χωριστά ) from all the particulars, but also that each form is a peculiarly accurate or good particular of its own kind, i.e. the standard particular of the kind in question or the model (παράδειγμα ) [i.e. paradigm ] to which other particulars approximate…. … Both in the Republic and in the Sophist there is a strong suggestion that correct thinking is following out the connexions between Forms. The model is mathematical thinking, e.g. the proof given in the Meno that the square on the diagonal is double the original square in area." – William and Martha Kneale, The Development of Logic , Oxford University Press paperback, 1985 Plato's paradigm in the Meno—
Changed paradigm in the diamond theorem (2×2 case) —
Aspects of the paradigm change— Monochrome figures to Areas to Continuous transformations to Euclidean geometry to Euclidean quantities to The 24 patterns resulting from the paradigm change—
Each pattern has some ordinary or color-interchange symmetry. This is the 2×2 case of a more general result. The patterns become more interesting in the 4×4 case. For their relationship to finite geometry and finite fields, see the diamond theorem. |
Related material: Plato's Diamond by Oslo artist Josefine Lyche.
“Plato’s Ghost evokes Yeats’s lament that any claim to worldly perfection inevitably is proven wrong by the philosopher’s ghost….”
— Princeton University Press on Plato’s Ghost: The Modernist Transformation of Mathematics (by Jeremy Gray, September 2008)
"Remember me to her."
— Closing words of the Algis Budrys novel Rogue Moon .
Background— Some posts in this journal related to Abel or to random thoughts from his birthday.
From Galleri MGM in Oslo —
A connection to today's earlier post, Sunday School— The Oslo Version, from Friday, May 21, 2010.
Lyche's "Omega Point" portal, together with her last name, suggested three posts from the following Saturday morning— which later proved to be the date of Martin Gardner's death—
Art Space, Through the Lyche Gate and The Lyche Gate Asterisk.
For some further religious remarks, see November 9th, 2010— A Theory of Pure Design.
"What exactly was Point Omega?"
This is Robert Wright in Nonzero: The Logic of Human Destiny.
Wright is discussing not the novel Point Omega by Don DeLillo,
but rather a (related) concept of the Jesuit philosopher Pierre Teilhard de Chardin.
My own idiosyncratic version of a personal "point omega"—
The circular sculpture in the foreground
is called by the artist "The Omega Point."
This has been described as
"a portal that leads in or out of time and space."
For some other sorts of points, see the drawings
on the wall and Geometry Simplified—

The two points of the trivial affine space are represented by squares,
and the one point of the trivial projective space is represented by
a line segment separating the affine-space squares.
For related darkness at noon, see Derrida on différance
as a version of Plato's khôra—
The above excerpts are from a work on and by Derrida
published in 1997 by Fordham University,
a Jesuit institution— Deconstruction in a Nutshell—
For an alternative to the Villanova view of Derrida,
see Angels in the Architecture.
Powered by WordPress