Today is the birthday of mathematician Jean-Pierre Serre.
Some remarks related to today's day number within the month, "15"—
The Wikipedia article on finite geometry has the following link—
Carnahan, Scott (2007-10-27), "Small finite sets", Secret Blogging Seminar, http://sbseminar.wordpress.com/2007/10/27/small-finite-sets/, notes on a talk by Jean-Pierre Serre on canonical geometric properties of small finite sets.
From Carnahan's notes (October 27, 2007)—
Serre has been giving a series of lectures at Harvard for the last month, on finite groups in number theory. It started off with some ideas revolving around Chebotarev density, and recently moved into fusion (meaning conjugacy classes, not monoidal categories) and mod p representations. In between, he gave a neat self-contained talk about small finite groups, which really meant canonical structures on small finite sets.
He started by writing the numbers 2,3,4,5,6,7,8, indicating the sizes of the sets to be discussed, and then he tackled them in order.
Related material on finite geometry and the indicated small numbers may, with one apparent exception, be found at my own Notes on Finite Geometry.
The apparent exception is "5." See, however, the role played in finite geometry by this number (and by "15") as sketched by Robert Steinberg at Yale in 1967—

See also …
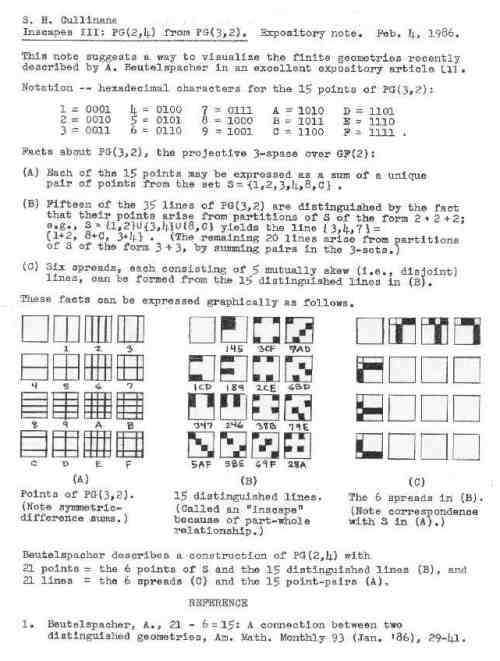
(Click to enlarge.)







