See also earlier posts mentioning Shrikhande in this journal.
He reportedly died on April 21, 2020.
Synchronologists may consult posts now tagged with that date.
Saturday, May 9, 2020
Shrikhande Continues.
Wednesday, April 22, 2020
Friday, November 30, 2018
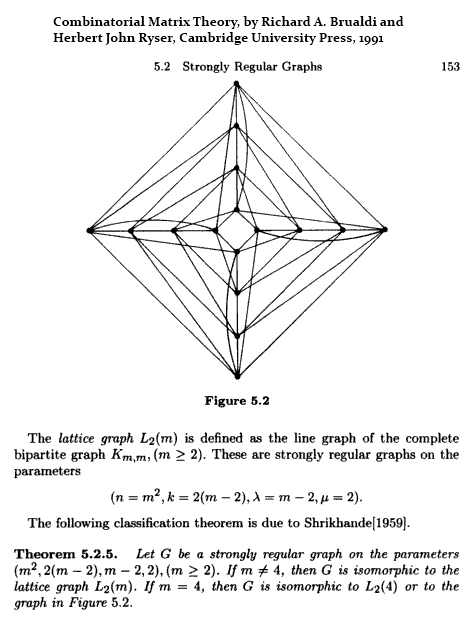
Latin-Square Structure
Continued from March 13, 2011 —
"…as we saw, there are two different Latin squares of order 4…."
— Peter J. Cameron, "The Shrikhande Graph," August 26, 2010
Cameron counts Latin squares as the same if they are isotopic .
Some further context for Cameron's remark—
A new website illustrates a different approach to Latin squares of order 4 —
Sunday, March 13, 2011
The Counter
"…as we saw, there are two different Latin squares of order 4…."
— Peter J. Cameron, "The Shrikhande Graph," August 26, 2010
Cameron counts Latin squares as the same if they are isotopic .
Some further context for Cameron's remark—
Cover Illustration Number 1 (1976):
Cover Illustration Number 2 (1991):
The Shrikhande Graph
______________________________________________________________________________
This post was prompted by two remarks…
1. In a different weblog, also on August 26, 2010—
The Accidental Mathematician— "The Girl Who Played with Fermat's Theorem."
"The worst thing about the series is the mathematical interludes in The Girl Who Played With Fire….
Salander is fascinated by a theorem on perfect numbers—
one can verify it for as many numbers as one wishes, and it never fails!—
and then advances through 'Archimedes, Newton, Martin Gardner,*
and a dozen other classical mathematicians,' all the way to Fermat’s last theorem."
2. "The fact that the pattern retains its symmetry when you permute the rows and columns
is very well known to combinatorial theorists who work with matrices."
[My italics; note resemblance to the Brualdi-Ryser title above.]
–Martin Gardner in 1976 on the diamond theorem
* Compare Eric Temple Bell (as quoted at the MacTutor history of mathematics site)—
"Archimedes, Newton, and Gauss, these three, are in a class by themselves
among the great mathematicians, and it is not for ordinary mortals
to attempt to range them in order of merit."
This is from the chapter on Gauss in Men of Mathematics .
Friday, March 11, 2011
Table Talk
The following was suggested by a link within this evening's earlier Kane site link.
Peter J. Cameron's weblog on August 26, 2010—
A Latin square of order n is a
|
|
Some related literary remarks—
Proginoskes and Latin Squares.
See also "It was a perfectly ordinary night at Christ's high table…."
Thursday, August 26, 2010
Home from Home continued
Or— Childhood's Rear End
This post was suggested by…
- Today's New York Times—
"For many artists Electric Lady has become a home away from home…. For Jimmy Page the personal imprimaturs of Hendrix and Mr. Kramer made all the difference when Led Zeppelin mixed parts of 'Houses of the Holy' there in 1972." - The album cover pictures for "Houses of the Holy"
- Boleskine House, home to Aleister Crowley and (occasionally) to Jimmy Page.
Related material:
The Zeppelin album cover, featuring rear views of nude children, was shot at the Giant's Causeway.
From a page at led-zeppelin.org—

See also Richard Rorty on Heidegger—
Safranski, the author of ''Schopenhauer and the Wild Years of Philosophy,'' never steps back and pronounces judgment on Heidegger, but something can be inferred from the German title of his book: ''Ein Meister aus Deutschland'' (''A Master From Germany''). Heidegger was, undeniably, a master, and was very German indeed. But Safranski's spine-chilling allusion is to Paul Celan's best-known poem, ''Death Fugue.'' In Michael Hamburger's translation, its last lines are:
death is a master from Germany his eyes are blue
he strikes you with leaden bullets his aim is true
a man lives in the house your golden hair Margarete
he sets his pack on us he grants us a grave in the air
he plays with the serpents and daydreams death is a master from Germany
your golden hair Margarete
your ashen hair Shulamith.
No one familiar with Heidegger's work can read Celan's poem without recalling Heidegger's famous dictum: ''Language is the house of Being. In its home man dwells.'' Nobody who makes this association can reread the poem without having the images of Hitler and Heidegger — two men who played with serpents and daydreamed — blend into each other. Heidegger's books will be read for centuries to come, but the smell of smoke from the crematories — the ''grave in the air'' — will linger on their pages.
Heidegger is the antithesis of the sort of philosopher (John Stuart Mill, William James, Isaiah Berlin) who assumes that nothing ultimately matters except human happiness. For him, human suffering is irrelevant: philosophy is far above such banalities. He saw the history of the West not in terms of increasing freedom or of decreasing misery, but as a poem. ''Being's poem,'' he once wrote, ''just begun, is man.''
For Heidegger, history is a sequence of ''words of Being'' — the words of the great philosophers who gave successive historical epochs their self-image, and thereby built successive ''houses of Being.'' The history of the West, which Heidegger also called the history of Being, is a narrative of the changes in human beings' image of themselves, their sense of what ultimately matters. The philosopher's task, he said, is to ''preserve the force of the most elementary words'' — to prevent the words of the great, houses-of-Being-building thinkers of the past from being banalized.
Related musical meditations—
Shine On (Saturday, April 21, 2007), Shine On, Part II, and Built (Sunday, April 22, 2007).
Related pictorial meditations—

The Giant's Causeway at Peter J. Cameron's weblog
and the cover illustration for Diamond Theory (1976)—

The connection between these two images is the following from Cameron's weblog today—
… as we saw, there are two different Latin squares of order 4;
one, but not the other, can be extended to a complete set
of 3 MOLS [mutually orthogonal Latin squares].
The underlying structures of the square pictures in the Diamond Theory cover are those of the two different Latin squares of order 4 mentioned by Cameron.
Connection with childhood—
The children's book A Wind in the Door, by Madeleine L'Engle. See math16.com. L'Engle's fantasies about children differ from those of Arthur C. Clarke and Led Zeppelin.