"To Phaedrus, this backlight from the conflict between
the Sophists and the Cosmologists adds an entirely
new dimension to the Dialogues of Plato." — Robert M. Pirsig
"It’s all in Plato, all in Plato;
bless me, what do they
teach them at these schools?”
— C. S. Lewis in
The Narnia Chronicles
Compare and Contrast — Plato's Diamond.
“The 2×2 matrix is commonly used in business strategy
as a representational tool to show conflicting concepts and
for decision making. This four-quadrant matrix diagram
is perfect to be used for business or marketing matrices
like BCG, SWOT, Ansoff, risk assessment…
Additionally, it will also be suitable to illustrate 4 ideas or
concepts.” [Link on “illustrate” added.]
See also a Log24 search for “Resplendent.”
From Ulysses , by James Joyce —
|
John Eglinton, frowning, said, waxing wroth: —Upon my word it makes my blood boil to hear anyone compare Aristotle with Plato. —Which of the two, Stephen asked, would have banished me from his commonwealth? |
Compare and contrast:
Fans of Plato might enjoy tales of Narnia, but fans of
James Joyce and Edgar Allan Poe might prefer
a tale by Michael Chabon from April 2001 about a
"doleful little corner of western Pennsylvania."
The title refers to the previous two posts.
Related literature —
Plato's Ghost: The Modernist Transformation of Mathematics
(Princeton University Press, 2008) and . . .
Plato's diamond-in-a-matrix:
(The title is from yesterday morning's Graphical Interfaces.)
For example, Plato's diamond as an object to be transformed —
Versions of the transformed object —
See also The 4×4 Relativity Problem in this journal.
Raiders of the Lost (Continued)
"Socrates: They say that the soul of man is immortal…."
From August 16, 2012—
In the geometry of Plato illustrated below,
"the figure of eight [square] feet" is not , at this point
in the dialogue, the diamond in Jowett's picture.
An 1892 figure by Jowett illustrating Plato's Meno—
A more correct version, from hermes-press.com —
|
Socrates: He only guesses that because the square is double, the line is double.Meno: True.
Socrates: Observe him while he recalls the steps in regular order. (To the Boy.) Tell me, boy, do you assert that a double space comes from a double line? Remember that I am not speaking of an oblong, but of a figure equal every way, and twice the size of this-that is to say of eight feet; and I want to know whether you still say that a double square comes from double line? [Boy] Yes. Socrates: But does not this line (AB) become doubled if we add another such line here (BJ is added)? [Boy] Certainly.
Socrates: And four such lines [AJ, JK, KL, LA] will make a space containing eight feet? [Boy] Yes. Socrates: Let us draw such a figure: (adding DL, LK, and JK). Would you not say that this is the figure of eight feet? [Boy] Yes. Socrates: And are there not these four squares in the figure, each of which is equal to the figure of four feet? (Socrates draws in CM and CN) [Boy] True. Socrates: And is not that four times four? [Boy] Certainly. Socrates: And four times is not double? [Boy] No, indeed. Socrates: But how much? [Boy] Four times as much. Socrates: Therefore the double line, boy, has given a space, not twice, but four times as much. [Boy] True. Socrates: Four times four are sixteen— are they not? [Boy] Yes. |
As noted in the 2012 post, the diagram of greater interest is
Jowett's incorrect version rather than the more correct version
shown above. This is because the 1892 version inadvertently
illustrates a tesseract:
A 4×4 square version, by Coxeter in 1950, of a tesseract—
This square version we may call the Galois tesseract.
The first two pages of a 1989 book by George Steiner—
See also yesterday's posts The Field of the Possible
and Abstraction.
Compare and contrast with Socrates in the Meno
quoting Pindar then discussing with a slave boy
the duplication of the square.
Was Socrates a great philosopher or, as the above
figure seems to indicate and as some say of Steiner,
too clever by half ?
Spidey Goes to Church
More realistically…
.
Today's previous post, "For Odin's Day," discussed
a mathematical object, the tesseract, from a strictly
narrative point of view.
In honor of George Balanchine, Odin might yield the
floor this evening to Apollo.
From a piece in today's online New York Times titled
"How a God Finds Art (the Abridged Version)"—
"… the newness at the heart of this story,
in which art is happening for the first time…."
Some related art—
and, more recently—
This more recent figure is from Ian Stewart's 1996 revision
of a 1941 classic, What Is Mathematics? , by Richard Courant
and Herbert Robbins.
Apollo might discuss with Socrates how the confused slave boy
of Plato's Meno would react to Stewart's remark that
"The number of copies required to double an
object's size depends on its dimension."
Apollo might also note an application of Socrates' Meno diagram
to the tesseract of this afternoon's Odin post—
.
(Continued from August 13. See also Coxeter Graveyard.)
Here the tombstone says
"GEOMETRY… 600 BC — 1900 AD… R.I.P."
In the geometry of Plato illustrated below,
"the figure of eight [square] feet" is not , at this point
in the dialogue, the diamond in Jowett's picture.
An 1892 figure by Jowett illustrating Plato's Meno—
Jowett's picture is nonetheless of interest for
its resemblance to a figure drawn some decades later
by the Toronto geometer H. S. M. Coxeter.
A similar 1950 figure by Coxeter illustrating a tesseract—
For a less scholarly, but equally confusing, view of the number 8,
see The Eight , a novel by Katherine Neville.
A passage from the Benjamin Jowett translation of Plato's Meno—
" 'For in the ninth year* Persephone sends the souls of those from whom she has received the penalty of ancient crime back again from beneath into the light of the sun above, and these are they who become noble kings and mighty men and great in wisdom and are called saintly heroes in after ages ⋄ .' The soul, then, as being immortal, and having been born again many times, and having seen all things that exist, whether in this world or in the world below, has knowledge of them all; and it is no wonder that she should be able to call to remembrance all that she ever knew about virtue, and about everything; for as all nature is akin, and the soul has learned all things; there is no difficulty in her eliciting or as men say learning, out of a single recollection all the rest, if a man is strenuous and does not faint; for all enquiry and all learning is but recollection."
* See this journal nine years ago, in August 2003.
⋄ Jowett's note— "Pindar, Frag. 98 (Boeckh)"
Wikipedia authors like Protious, an alleged resident of Egypt and
creator of The Socrates Swastika , may enjoy a less scholarly account:
From Babylon A. D. (a 2008 film)— Toorop with Egyptian Sacred Scarab tattoo—
— and Toorop with Aurora (who may be regarded as "the soul" in the Meno passage above)—
Toorop's neck tattoo in the second image above is from a fictional book
described in the writings of H. P. Lovecraft.
As swastika-like sacred symbols go, I prefer St. Bridget's Cross.
A Balliol Star
In memory of
mathematician
Graham Higman of
Balliol College and
Magdalen College,
Oxford,
Jan. 19, 1917 –
April 8, 2008
From a biography of an earlier Balliol student,
Gerard Manley Hopkins (1844-1889):
"In 1867 he won First-Class degrees in Classics
and 'Greats' (a rare 'double-first') and was
considered by Jowett to be the star of Balliol."
Hopkins, a poet who coined the term "inscape," was a member of the Society of Jesus.
According to a biography, Higman was the founder of Oxford's Invariant Society.
From a publication of that society, The Invariant, Issue 15– undated but (according to Issue 16, of 2005) from 1996 (pdf):
|
Taking the square root
of a function by Ian Collier "David Singmaster once gave a talk at the Invariants and afterwards asked this question: What is the square root of the exponential function? In other words, can you define a function f such that for all x, |
Another approach to the expression f(f(x)), by myself in 1982:
For further details,
see Inscapes.
For more about Higman, see an interview in the September 2001 newsletter of the European Mathematical Society (pdf).
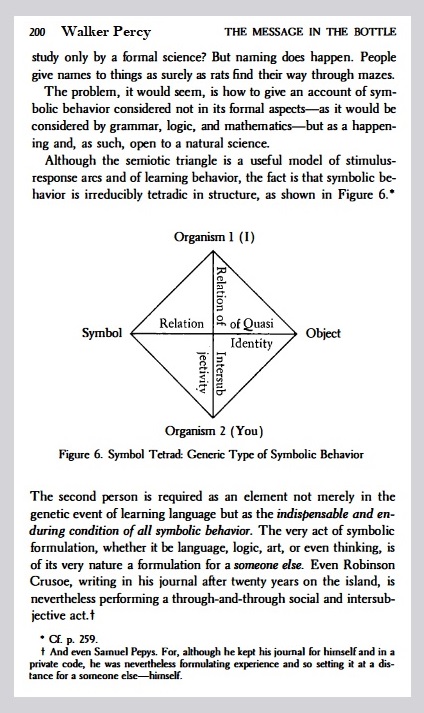
The Line
Robert M. Pirsig, Zen and the Art of Motorcycle Maintenance, Ch. 6 (italics are mine):
“A classical understanding sees the world primarily as underlying form itself. A romantic understanding sees it primarily in terms of immediate appearance.”
STRANGER – We are far from having exhausted the more exact thinkers who treat of being and not-being. But let us be content to leave them, and proceed to view those who speak less precisely; and we shall find as the result of all, that the nature of being is quite as difficult to comprehend as that of not-being.
THEAETETUS – Then now we will go to the others.
STRANGER – There appears to be a sort of war of Giants and Gods going on amongst them; they are fighting with one another about the nature of essence.
THEAETETUS – How is that?
STRANGER – Some of them are dragging down all things from heaven and from the unseen to earth, and they literally grasp in their hands rocks and oaks; of these they lay hold, and obstinately maintain, that the things only which can be touched or handled have being or essence, because they define being and body as one, and if any one else says that what is not a body exists they altogether despise him, and will hear of nothing but body.
THEAETETUS – I have often met with such men, and terrible fellows they are.
STRANGER – And that is the reason why their opponents cautiously defend themselves from above, out of an unseen world, mightily contending that true essence consists of certain intelligible and incorporeal ideas; the bodies of the materialists, which by them are maintained to be the very truth, they break up into little bits by their arguments, and affirm them to be, not essence, but generation and motion. Between the two armies, Theaetetus, there is always an endless conflict raging concerning these matters.
THEAETETUS – True.
— Translated by Benjamin Jowett
Robert M. Pirsig, Zen and the Art of Motorcycle Maintenance, Ch. 18:
“The wave of crystallization rolled ahead. He was seeing two worlds, simultaneously. On the intellectual side, the square side, he saw now that Quality was a cleavage term. What every intellectual analyst looks for. You take your analytic knife, put the point directly on the term Quality and just tap, not hard, gently, and the whole world splits, cleaves, right in two…
hip and square, classic and romantic, technological and humanistic…and the split is clean. There’s no mess. No slop. No little items that could be one way or the other. Not just a skilled break but a very lucky break. Sometimes the best analysts, working with the most obvious lines of cleavage, can tap and get nothing but a pile of trash. And yet here was Quality; a tiny, almost unnoticeable fault line; a line of illogic in our concept of the universe; and you tapped it, and the whole universe came apart, so neatly it was almost unbelievable. He wished Kant were alive. Kant would have appreciated it. That master diamond cutter. He would see. Hold Quality undefined. That was the secret.”
What Pirsig means by “quality” is close to what Yagoda means, in the previous entry, by “style.”
Powered by WordPress
