Wednesday, September 18, 2019
Battle Song
Friday, August 16, 2019
Nocciolo
A revision of the above diagram showing
the Galois-addition-table structure —

Related tables from August 10 —

See "Schoolgirl Space Revisited."
Thursday, March 21, 2019
Geometry of Interstices
Finite Galois geometry with the underlying field the simplest one possible —
namely, the two-element field GF(2) — is a geometry of interstices :
For some less precise remarks, see the tags Interstice and Interality.
The rationalist motto "sincerity, order, logic and clarity" was quoted
by Charles Jencks in the previous post.
This post was suggested by some remarks from Queensland that
seem to exemplify these qualities —

Monday, March 11, 2019
Ant-Man Meets Doctor Strange

The 4×4 square may also be called the Galois Tesseract .
By analogy, the 4x4x4 cube may be called the Galois Hexeract .
Thursday, February 28, 2019
Fooling

The two books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
Note: There is no Galois (i.e., finite) field with six elements, but
the theory of finite fields underlies applications of six-set geometry.
Sunday, February 10, 2019
Cold Open
The previous post, on the Bauhaus 100th anniversary, suggests a review . . .
"Congratulations to the leaders of both parties:
The past 20 years you’ve taken us far.
We’re entering Weimar, baby."
— Peggy Noonan in The Wall Street Journal
on August 13, 2015
Image from yesterday's Log24 search Bauhaus Space.
Thursday, January 10, 2019
Sunday, December 2, 2018
Symmetry at Hiroshima
A search this morning for articles mentioning the Miracle Octad Generator
of R. T. Curtis within the last year yielded an abstract for two talks given
at Hiroshima on March 8 and 9, 2018 —
|
http://www.math.sci.hiroshima-u.ac.jp/ branched/files/2018/abstract/Aitchison.txt
Iain AITCHISON Title: Construction of highly symmetric Riemann surfaces, related manifolds, and some exceptional objects, I, II Abstract: Since antiquity, some mathematical objects have played a special role, underpinning new mathematics as understanding deepened. Perhaps archetypal are the Platonic polyhedra, subsequently related to Platonic idealism, and the contentious notion of existence of mathematical reality independent of human consciousness. Exceptional or unique objects are often associated with symmetry – manifest or hidden. In topology and geometry, we have natural base points for the moduli spaces of closed genus 2 and 3 surfaces (arising from the 2-fold branched cover of the sphere over the 6 vertices of the octahedron, and Klein's quartic curve, respectively), and Bring's genus 4 curve arises in Klein's description of the solution of polynomial equations of degree greater than 4, as well as in the construction of the Horrocks-Mumford bundle. Poincare's homology 3-sphere, and Kummer's surface in real dimension 4 also play special roles. In other areas: we have the exceptional Lie algebras such as E8; the sporadic finite simple groups; the division algebras: Golay's binary and ternary codes; the Steiner triple systems S(5,6,12) and S(5,8,24); the Leech lattice; the outer automorphisms of the symmetric group S6; the triality map in dimension 8; and so on. We also note such as: the 27 lines on a cubic, the 28 bitangents of a quartic curve, the 120 tritangents of a sextic curve, and so on, related to Galois' exceptional finite groups PSL2(p) (for p= 5,7,11), and various other so-called `Arnol'd Trinities'. Motivated originally by the `Eightfold Way' sculpture at MSRI in Berkeley, we discuss inter-relationships between a selection of these objects, illustrating connections arising via highly symmetric Riemann surface patterns. These are constructed starting with a labeled polygon and an involution on its label set. Necessarily, in two lectures, we will neither delve deeply into, nor describe in full, contexts within which exceptional objects arise. We will, however, give sufficient definition and detail to illustrate essential inter-connectedness of those exceptional objects considered. Our starting point will be simplistic, arising from ancient Greek ideas underlying atomism, and Plato's concepts of space. There will be some overlap with a previous talk on this material, but we will illustrate with some different examples, and from a different philosophical perspective. Some new results arising from this work will also be given, such as an alternative graphic-illustrated MOG (Miracle Octad Generator) for the Steiner system S(5,8,24), and an alternative to Singerman – Jones' genus 70 Riemann surface previously proposed as a completion of an Arnol'd Trinity. Our alternative candidate also completes a Trinity whose two other elements are Thurston's highly symmetric 6- and 8-component links, the latter related by Thurston to Klein's quartic curve. |
See also yesterday morning's post, "Character."
Update: For a followup, see the next Log24 post.
Tuesday, November 20, 2018
Logos
Musical accompaniment from Sunday morning —
Update of Nov. 21 —
The reader may contrast the above Squarespace.com logo
(a rather serpentine version of the acronym SS) with a simpler logo
for a square space (the Galois window ):
Friday, September 14, 2018
Denkraum
Underlying the I Ching structure is the finite affine space
of six dimensions over the Galois field with two elements.
In this field, "1 + 1 = 0," as noted here Wednesday.
See also other posts now tagged Interstice.
Wednesday, June 27, 2018
Taken In
A passage that may or may not have influenced Madeleine L'Engle's
writings about the tesseract :
|
From Mere Christianity , by C. S. Lewis (1952) —
"Book IV – Beyond Personality: I warned you that Theology is practical. The whole purpose for which we exist is to be thus taken into the life of God. Wrong ideas about what that life is, will make it harder. And now, for a few minutes, I must ask you to follow rather carefully. You know that in space you can move in three ways—to left or right, backwards or forwards, up or down. Every direction is either one of these three or a compromise between them. They are called the three Dimensions. Now notice this. If you are using only one dimension, you could draw only a straight line. If you are using two, you could draw a figure: say, a square. And a square is made up of four straight lines. Now a step further. If you have three dimensions, you can then build what we call a solid body, say, a cube—a thing like a dice or a lump of sugar. And a cube is made up of six squares. Do you see the point? A world of one dimension would be a straight line. In a two-dimensional world, you still get straight lines, but many lines make one figure. In a three-dimensional world, you still get figures but many figures make one solid body. In other words, as you advance to more real and more complicated levels, you do not leave behind you the things you found on the simpler levels: you still have them, but combined in new ways—in ways you could not imagine if you knew only the simpler levels. Now the Christian account of God involves just the same principle. The human level is a simple and rather empty level. On the human level one person is one being, and any two persons are two separate beings—just as, in two dimensions (say on a flat sheet of paper) one square is one figure, and any two squares are two separate figures. On the Divine level you still find personalities; but up there you find them combined in new ways which we, who do not live on that level, cannot imagine. In God's dimension, so to speak, you find a being who is three Persons while remaining one Being, just as a cube is six squares while remaining one cube. Of course we cannot fully conceive a Being like that: just as, if we were so made that we perceived only two dimensions in space we could never properly imagine a cube. But we can get a sort of faint notion of it. And when we do, we are then, for the first time in our lives, getting some positive idea, however faint, of something super-personal—something more than a person. It is something we could never have guessed, and yet, once we have been told, one almost feels one ought to have been able to guess it because it fits in so well with all the things we know already. You may ask, "If we cannot imagine a three-personal Being, what is the good of talking about Him?" Well, there isn't any good talking about Him. The thing that matters is being actually drawn into that three-personal life, and that may begin any time —tonight, if you like. . . . . |
But beware of being drawn into the personal life of the Happy Family .
https://www.jstor.org/stable/24966339 —
"The colorful story of this undertaking begins with a bang."
And ends with …
"Galois was a thoroughly obnoxious nerd,
suffering from what today would be called
a 'personality disorder.' His anger was
paranoid and unremitting."
Friday, May 4, 2018
The Tuchman Radical*
Two excerpts from today's Art & Design section of
The New York Times —
For the deplorables of France —
For further remarks on l'ordre , see
other posts tagged Galois's Space
(… tag=galoiss-space).
* The radical of the title is Évariste Galois (1811-1832).
Sunday, April 29, 2018
Amusement
From the online New York Times this afternoon:
Disney now holds nine of the top 10
domestic openings of all time —
six of which are part of the Marvel
Cinematic Universe. “The result is
a reflection of 10 years of work:
of developing this universe, creating
stakes as big as they were, characters
that matter and stories and worlds that
people have come to love,” Dave Hollis,
Disney’s president of distribution, said
in a phone interview.
From this journal this morning:
"But she felt there must be more to this
than just the sensation of folding space
over on itself. Surely the Centaurs hadn't
spent ten years telling humanity how to
make a fancy amusement-park ride.
There had to be more—"
— Factoring Humanity , by Robert J. Sawyer,
Tom Doherty Associates, 2004 Orb edition,
page 168
"The sensation of folding space . . . ."
Or unfolding:
Click the above unfolded space for some background.
Friday, February 16, 2018
Two Kinds of Symmetry
The Institute for Advanced Study (IAS) at Princeton in its Fall 2015 Letter
revived "Beautiful Mathematics" as a title:
This ugly phrase was earlier used by Truman State University
professor Martin Erickson as a book title. See below.
In the same IAS Fall 2015 Letter appear the following remarks
by Freeman Dyson —
". . . a special case of a much deeper connection that Ian Macdonald
discovered between two kinds of symmetry which we call modular and affine.
The two kinds of symmetry were originally found in separate parts of science,
modular in pure mathematics and affine in physics. Modular symmetry is
displayed for everyone to see in the drawings of flying angels and devils
by the artist Maurits Escher. Escher understood the mathematics and got the
details right. Affine symmetry is displayed in the peculiar groupings of particles
created by physicists with high-energy accelerators. The mathematician
Robert Langlands was the first to conjecture a connection between these and
other kinds of symmetry. . . ." (Wikipedia link added.)
The adjective "modular" might aptly be applied to . . .

The adjective "affine" might aptly be applied to . . .
The geometry of the 4×4 square combines modular symmetry
(i.e., related to theta functions) with the affine symmetry above.
Hudson's 1905 discussion of modular symmetry (that of Rosenhain
tetrads and Göpel tetrads) in the 4×4 square used a parametrization
of that square by the digit 0 and the fifteen 2-subsets of a 6-set, but
did not discuss the 4×4 square as an affine space.
For the connection of the 15 Kummer modular 2-subsets with the 16-
element affine space over the two-element Galois field GF(2), see my note
of May 26, 1986, "The 2-subsets of a 6-set are the points of a PG(3,2)" —
— and the affine structure in the 1979 AMS abstract
"Symmetry invariance in a diamond ring" —
For some historical background on the symmetry investigations by
Dyson and Macdonald, see Dyson's 1972 article "MIssed Opportunities."
For Macdonald's own use of the words "modular" and "affine," see
Macdonald, I. G., "Affine Lie algebras and modular forms,"
Séminaire N. Bourbaki , Vol. 23 (1980-1981), Talk no. 577, pp. 258-276.
Tuesday, September 5, 2017
Annals of Critical Epistemology
"But unlike many who left the Communist Party, I turned left
rather than right, and returned—or rather turned for the first time—
to a critical examination of Marx's work. I found—and still find—
that his analysis of capitalism, which for me is the heart of his work,
provides the best starting point, the best critical tools, with which—
suitably developed—to understand contemporary capitalism.
I remind you that this year is also the sesquicentennial of the
Communist Manifesto , a document that still haunts the capitalist world."
— From "Autobiographical Reflections," a talk given on June 5, 1998, by
John Stachel at the Max Planck Institute for the History of Science in Berlin
on the occasion of a workshop honoring his 70th birthday,
"Space-Time, Quantum Entanglement and Critical Epistemology."
From a passage by Stachel quoted in the previous post —

From the source for Stachel's remarks on Weyl and coordinatization —

Note that Stachel distorted Weyl's text by replacing Weyl's word
"symbols" with the word "quantities." —

This replacement makes no sense if the coordinates in question
are drawn from a Galois field — a field not of quantities , but rather
of algebraic symbols .
"You've got to pick up every stitch… Must be the season of the witch."
— Donovan song at the end of Nicole Kidman's "To Die For"
Florence 2001
Or: Coordinatization for Physicists
This post was suggested by the link on the word "coordinatized"
in the previous post.


I regret that Weyl's term "coordinatization" perhaps has
too many syllables for the readers of recreational mathematics —
for example, of an article on 4×4 magic squares by Conway, Norton,
and Ryba to be published today by Princeton University Press.
Insight into the deeper properties of such squares unfortunately
requires both the ability to learn what a "Galois field" is and the
ability to comprehend seven-syllable words.
Sunday, August 27, 2017
Black Well
The “Black” of the title refers to the previous post.
For the “Well,” see Hexagram 48.
Related material —
The Galois Tesseract and, more generally, Binary Coordinate Systems.
Friday, August 11, 2017
Symmetry’s Lifeboat
A post suggested by the word tzimtzum (see Wednesday)
or tsimtsum (see this morning) —
Lifeboat from the Tsimtsum in Life of Pi —

Another sort of tsimtsum, contracting infinite space to a finite space —
Tuesday, July 11, 2017
A Date at the Death Cafe
The New York TImes reports this evening that
"Jon Underwood, Founder of Death Cafe Movement,"
died suddenly at 44 on June 27.
This journal on that date linked to a post titled "The Mystic Hexastigm."
A related remark on the complete 6-point from Sunday, April 28, 2013 —
(See, in Veblen and Young's 1910 Vol. I, exercise 11,
page 53: "A plane section of a 6-point in space can
be considered as 3 triangles perspective in pairs
from 3 collinear points with corresponding sides
meeting in 3 collinear points." This is the large
Desargues configuration. See Classical Geometry
in Light of Galois Geometry.)
This post was suggested, in part, by the philosophical ruminations
of Rosalind Krauss in her 2011 book Under Blue Cup . See
Sunday's post Perspective and Its Transections . (Any resemblance
to Freud's title Civilization and Its Discontents is purely coincidental.)
Sunday, July 9, 2017
Perspective and Its Transections
The title phrase is from Rosalind Krauss (Under Blue Cup , 2011) —
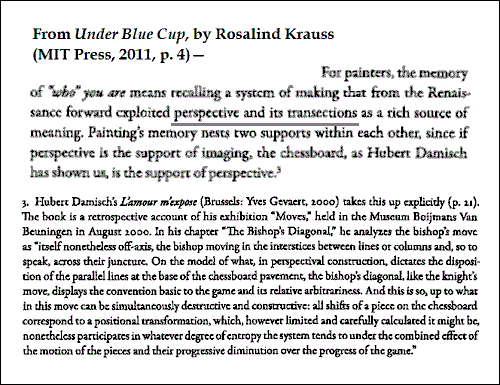
Another way of looking at the title phrase —
"A very important configuration is obtained by
taking the plane section of a complete space five-point."
(Veblen and Young, 1910, p. 39) —
For some context, see Desargues + Galois in this journal.
Wednesday, July 5, 2017
Imaginarium of a Different Kind
The title refers to that of the previous post, "The Imaginarium."
In memory of a translator who reportedly died on May 22, 2017,
a passage quoted here on that date —
Related material — A paragraph added on March 15, 2017,
to the Wikipedia article on Galois geometry —
|
George Conwell gave an early demonstration of Galois geometry in 1910 when he characterized a solution of Kirkman's schoolgirl problem as a partition of sets of skew lines in PG(3,2), the three-dimensional projective geometry over the Galois field GF(2).[3] Similar to methods of line geometry in space over a field of characteristic 0, Conwell used Plücker coordinates in PG(5,2) and identified the points representing lines in PG(3,2) as those on the Klein quadric. — User Rgdboer |
Monday, May 29, 2017
The American Sublime
Line from "Vide," a post of June 8, 2014 —
Vide Classical Geometry in Light of Galois Geometry.
Recall that vide means different things in Latin and in French.
See also Stevens + "Vacant Space" in this journal.
Friday, April 14, 2017
Hudson and Finite Geometry
The above four-element sets of black subsquares of a 4×4 square array
are 15 of the 60 Göpel tetrads , and 20 of the 80 Rosenhain tetrads , defined
by R. W. H. T. Hudson in his 1905 classic Kummer's Quartic Surface .
Hudson did not view these 35 tetrads as planes through the origin in a finite
affine 4-space (or, equivalently, as lines in the corresponding finite projective
3-space).
In order to view them in this way, one can view the tetrads as derived,
via the 15 two-element subsets of a six-element set, from the 16 elements
of the binary Galois affine space pictured above at top left.
This space is formed by taking symmetric-difference (Galois binary)
sums of the 15 two-element subsets, and identifying any resulting four-
element (or, summing three disjoint two-element subsets, six-element)
subsets with their complements. This process was described in my note
"The 2-subsets of a 6-set are the points of a PG(3,2)" of May 26, 1986.
The space was later described in the following —

Sunday, January 29, 2017
Lottery Hermeneutics
For some backstory, see Lottery in this journal,
esp. a post of June 28, 2007:
Real Numbers: An Object Lesson.
One such number, 8775, is suggested by
a Heinlein short story in a Jan. 25 post.
A search today for that number —
That Jan. 25 post, "For Your Consideration," also mentions logic.
Logic appears as well within a post from the above "8775" date,
August 16, 2016 —
|
Update of 10 am on August 16, 2016 —
See also Atiyah on the theology of |
Related: Remarks by Charles Altieri on Wittgenstein in
today's previous post.
For remarks by Wittgenstein related to geometry and logic, see
(for instance) "Logical space" in "A Wittgenstein Dictionary," by
Hans-Johann Glock (Wiley-Blackwell, 1996).
Saturday, December 31, 2016
Habeas
Thursday, September 15, 2016
The Smallest Perfect Number/Universe
The smallest perfect number,* six, meets
"the smallest perfect universe,"** PG(3,2).
* For the definition of "perfect number," see any introductory
number-theory text that deals with the history of the subject.
** The phrase "smallest perfect universe" as a name for PG(3,2),
the projective 3-space over the 2-element Galois field GF(2),
was coined by math writer Burkard Polster. Cullinane's square
model of PG(3,2) differs from the earlier tetrahedral model
discussed by Polster.
Tuesday, September 13, 2016
Parametrizing the 4×4 Array
The previous post discussed the parametrization of
the 4×4 array as a vector 4-space over the 2-element
Galois field GF(2).
The 4×4 array may also be parametrized by the symbol
0 along with the fifteen 2-subsets of a 6-set, as in Hudson's
1905 classic Kummer's Quartic Surface —
Hudson in 1905:
These two ways of parametrizing the 4×4 array — as a finite space
and as an array of 2-element sets — were related to one another
by Cullinane in 1986 in describing, in connection with the Curtis
"Miracle Octad Generator," what turned out to be 15 of Hudson's
1905 "Göpel tetrads":
A recap by Cullinane in 2013:
Click images for further details.
Wednesday, August 24, 2016
Core Statements
"That in which space itself is contained" — Wallace Stevens
An image by Steven H. Cullinane from April 1, 2013:
The large Desargues configuration of Euclidean 3-space can be
mapped canonically to the 4×4 square of Galois geometry —
On an Auckland University of Technology thesis by Kate Cullinane —

The thesis reportedly won an Art Directors Club award on April 5, 2013.
Monday, August 1, 2016
Cube
From this journal —
See (for instance) Sacred Order, July 18, 2006 —
From a novel published July 26, 2016, and reviewed
in yesterday's (print) New York Times Book Review —
|
The doors open slowly. I step into a hangar. From the rafters high above, lights blaze down, illuminating a twelve-foot cube the color of gunmetal. My pulse rate kicks up. I can’t believe what I’m looking at. Leighton must sense my awe, because he says, “Beautiful, isn’t it?” It is exquisitely beautiful. At first, I think the hum inside the hangar is coming from the lights, but it can’t be. It’s so deep I can feel it at the base of my spine, like the ultralow-frequency vibration of a massive engine. I drift toward the box, mesmerized.
— Crouch, Blake. Dark Matter: A Novel |
See also Log24 on the publication date of Dark Matter .
Thursday, July 28, 2016
Monday, May 30, 2016
Perfect Universe
(A sequel to the previous post, Perfect Number)
Since antiquity, six has been known as
"the smallest perfect number." The word "perfect"
here means that a number is the sum of its
proper divisors — in the case of six: 1, 2, and 3.
The properties of a six-element set (a "6-set")
divided into three 2-sets and divided into two 3-sets
are those of what Burkard Polster, using the same
adjective in a different sense, has called
"the smallest perfect universe" — PG(3,2), the projective
3-dimensional space over the 2-element Galois field.
A Google search for the phrase "smallest perfect universe"
suggests a turnaround in meaning , if not in finance,
that might please Yahoo CEO Marissa Mayer on her birthday —


The semantic turnaround here in the meaning of "perfect"
is accompanied by a model turnaround in the picture of PG(3,2) as
Polster's tetrahedral model is replaced by Cullinane's square model.
Further background from the previous post —
See also Kirkman's Schoolgirl Problem.
Tuesday, May 24, 2016
Rosenhain and Göpel Revisited
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above

Monday, April 25, 2016
Seven Seals
An old version of the Wikipedia article "Group theory"
(pictured in the previous post) —

"More poetically …"
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
The seven seals from the previous post, with some context —
These models of projective points are drawn from the underlying
structure described (in the 4×4 case) as part of the proof of the
Cullinane diamond theorem .
Thursday, January 21, 2016
Dividing the Indivisible
My statement yesterday morning that the 15 points
of the finite projective space PG(3,2) are indivisible
was wrong. I was misled by quoting the powerful
rhetoric of Lincoln Barnett (LIFE magazine, 1949).
Points of Euclidean space are of course indivisible:
"A point is that which has no parts" (in some translations).
And the 15 points of PG(3,2) may be pictured as 15
Euclidean points in a square array (with one point removed)
or tetrahedral array (with 11 points added).
The geometry of PG(3,2) becomes more interesting,
however, when the 15 points are each divided into
several parts. For one approach to such a division,
see Mere Geometry. For another approach, click on the
image below.
Wednesday, January 13, 2016
Geometry for Jews
(Continued from previous episodes)
Boole and Galois also figure in the mathematics of space —
i.e. , geometry. See Boole + Galois in this journal.
Related material, according to Jung’s notion of synchronicity —
- This journal on the date, August 6, 2007, of the
above paper, and the following day —
posts now tagged Metamorphosis 2007 - This journal on two of the dates of the 2003 Haifa workshop
that the paper mentions in a footnote —
posts now tagged Solomon’s Mental Health Month
Monday, December 28, 2015
ART WARS Continues
Combining two headlines from this morning’s
New York Times and Washington Post , we have…
Deceptively Simple Geometries
on a Bold Scale
Voilà —
Click image for details.
More generally, see
Boole vs. Galois.
Tuesday, December 1, 2015
Pascal’s Finite Geometry
See a search for "large Desargues configuration" in this journal.
The 6 Jan. 2015 preprint "Danzer's Configuration Revisited,"
by Boben, Gévay, and Pisanski, places this configuration,
which they call the Cayley-Salmon configuration , in the
interesting context of Pascal's Hexagrammum Mysticum .
They show how the Cayley-Salmon configuration is, in a sense,
dual to something they call the Steiner-Plücker configuration .
This duality appears implicitly in my note of April 26, 1986,
"Picturing the smallest projective 3-space." The six-sets at
the bottom of that note, together with Figures 3 and 4
of Boben et. al. , indicate how this works.

The duality was, as they note, previously described in 1898.


Related material on six-set geometry from the classical literature—
Baker, H. F., "Note II: On the Hexagrammum Mysticum of Pascal,"
in Principles of Geometry , Vol. II, Camb. U. Press, 1930, pp. 219-236
Richmond, H. W., "The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen (1900), Volume 53, Issue 1-2, pp 161-176
Richmond, H. W., "On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics , Vol. 31 (1900), pp. 125-160
Related material on six-set geometry from a more recent source —
Cullinane, Steven H., "Classical Geometry in Light of Galois Geometry," webpage
Friday, November 20, 2015
Anticommuting Dirac Matrices as Skew Lines
(Continued from November 13)
The work of Ron Shaw in this area, ca. 1994-1995, does not
display explicitly the correspondence between anticommutativity
in the set of Dirac matrices and skewness in a line complex of
PG(3,2), the projective 3-space over the 2-element Galois field.
Here is an explicit picture —

References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Shaw, Ron, "Finite Geometry, Dirac Groups, and the Table of
Real Clifford Algebras," undated article at ResearchGate.net
Update of November 23:
See my post of Nov. 23 on publications by E. M. Bruins
in 1949 and 1959 on Dirac matrices and line geometry,
and on another author who gives some historical background
going back to Eddington.
Some more-recent related material from the Slovak school of
finite geometry and quantum theory —
The matrices underlying the Saniga paper are those of Pauli, not
those of Dirac, but these two sorts of matrices are closely related.
Monday, November 2, 2015
The Devil’s Offer
This is a sequel to the previous post and to the Oct. 24 post
Two Views of Finite Space. From the latter —
” ‘All you need to do is give me your soul:
give up geometry and you will have this
marvellous machine.’ (Nowadays you
can think of it as a computer!) “
Saturday, October 31, 2015
Raiders of the Lost Crucible
Stanford Encyclopedia of Philosophy
on the date Friday, April 5, 2013 —
“First published Tue Sep 24, 1996;
substantive revision Fri Apr 5, 2013”
This journal on the date Friday, April 5, 2013 —
The object most closely resembling a “philosophers’ stone”
that I know of is the eightfold cube .
For some related philosophical remarks that may appeal
to a general Internet audience, see (for instance) a website
by I Ching enthusiast Andreas Schöter that displays a labeled
eightfold cube in the form of a lattice diagram —

Related material by Schöter —
A 20-page PDF, “Boolean Algebra and the Yi Jing.”
(First published in The Oracle: The Journal of Yijing Studies ,
Vol 2, No 7, Summer 1998, pp. 19–34.)
I differ with Schöter’s emphasis on Boolean algebra.
The appropriate mathematics for I Ching studies is,
I maintain, not Boolean algebra but rather Galois geometry.
See last Saturday’s post Two Views of Finite Space.
Unfortunately, that post is, unlike Schöter’s work, not
suitable for a general Internet audience.
Sunday, September 6, 2015
Elementally, My Dear Watson
Sarah Larson in the online New Yorker on Sept. 3, 2015,
discussed Google’s new parent company, “Alphabet”—
“… Alphabet takes our most elementally wonderful
general-use word—the name of the components of
language itself*—and reassigns it, like the words
tweet, twitter, vine, facebook, friend, and so on,
into a branded realm.”

Emma Watson in “The Bling Ring”
This journal, also on September 3 —
| Thursday, September 3, 2015
Filed under: Uncategorized — m759 @ 7:20 AM For the title, see posts from August 2007 Related theological remarks: Boolean spaces (old) vs. Galois spaces (new) in |
* Actually, Sarah, that would be “phonemes.”
Thursday, September 3, 2015
Rings of August
For the title, see posts from August 2007 tagged Gyges.
Related theological remarks:
Boolean spaces (old) vs. Galois spaces (new) in
“The Quality Without a Name”
(a post from August 26, 2015) and the…
Related literature: A search for Borogoves in this journal will yield
remarks on the 1943 tale underlying the above film.
Wednesday, August 26, 2015
“The Quality Without a Name”
The title phrase, paraphrased without quotes in
the previous post, is from Christopher Alexander's book
The Timeless Way of Building (Oxford University Press, 1979).
A quote from the publisher:
"Now, at last, there is a coherent theory
which describes in modern terms
an architecture as ancient as
human society itself."
Three paragraphs from the book (pp. xiii-xiv):
19. Within this process, every individual act
of building is a process in which space gets
differentiated. It is not a process of addition,
in which preformed parts are combined to
create a whole, but a process of unfolding,
like the evolution of an embryo, in which
the whole precedes the parts, and actualy
gives birth to then, by splitting.
20. The process of unfolding goes step by step,
one pattern at a time. Each step brings just one
pattern to life; and the intensity of the result
depends on the intensity of each one of these
individual steps.
21. From a sequence of these individual patterns,
whole buildings with the character of nature
will form themselves within your thoughts,
as easily as sentences.
Compare to, and contrast with, these illustrations of "Boolean space":

(See also similar illustrations from Berkeley and Purdue.)
Detail of the above image —

Note the "unfolding," as Christopher Alexander would have it.
These "Boolean" spaces of 1, 2, 4, 8, and 16 points
are also Galois spaces. See the diamond theorem —
Sunday, July 19, 2015
Lying Rhyme
|
Tom Stoppard, Jumpers —
“Heaven, how can I believe in Heaven?”
“To begin at the beginning: Is God?…” “Leave a space.” |
See as well a search for "Heaven.gif" in this journal.
For the more literate among us —
… and the modulation from algebra to space.
Wednesday, June 17, 2015
Slow Art, Continued
The title of the previous post, "Slow Art," is a phrase
of the late art critic Robert Hughes.
Example from mathematics:
-
Göpel tetrads as subsets of a 4×4 square in the classic
1905 book Kummer's Quartic Surface by R. W. H. T. Hudson.
These subsets were constructed as helpful schematic diagrams,
without any reference to the concept of finite geometry they
were later to embody.
-
Göpel tetrads (not named as such), again as subsets of
a 4×4 square, that form the 15 isotropic projective lines of the
finite projective 3-space PG(3,2) in a note on finite geometry
from 1986 —
-
Göpel tetrads as these figures of finite geometry in a 1990
foreword to the reissued 1905 book of Hudson:
Click the Barth passage to see it with its surrounding text.
Related material:
Monday, June 15, 2015
Omega Matrix
See that phrase in this journal.
See also last night's post.

The Greek letter Ω is customarily used to
denote a set that is acted upon by a group.
If the group is the affine group of 322,560
transformations of the four-dimensional
affine space over the two-element Galois
field, the appropriate Ω is the 4×4 grid above.
Thursday, June 11, 2015
Omega
Omega is a Greek letter, Ω , used in mathematics to denote
a set on which a group acts.
For instance, the affine group AGL(3,2) is a group of 1,344
actions on the eight elements of the vector 3-space over the
two-element Galois field GF(2), or, if you prefer, on the Galois
field Ω = GF(8).
Related fiction: The Eight , by Katherine Neville.
Related non-fiction: A remark by Werner Heisenberg
in this journal on Saturday, June 6, 2015, the eightfold cube ,
and the illustrations below —
|
Mathematics
The Fano plane block design |
Magic
The Deathly Hallows symbol— |
Tuesday, March 24, 2015
Hirzebruch
(Continued from July 16, 2014.)
Some background from Wikipedia:
"Friedrich Ernst Peter Hirzebruch ForMemRS[2]
(17 October 1927 – 27 May 2012)
was a German mathematician, working in the fields of topology,
complex manifolds and algebraic geometry, and a leading figure
in his generation. He has been described as 'the most important
mathematician in Germany of the postwar period.'
[3][4][5][6][7][8][9][10][11]"
A search for citations of the A. E. Brouwer paper in
the previous post yields a quotation from the preface
to the third ("2013") edition of Wolfgang Ebeling's
Lattices and Codes: A Course Partially Based
on Lectures by Friedrich Hirzebruch , a book
reportedly published on September 19, 2012 —
|
"Sadly, on May 27 this year, Friedrich Hirzebruch, Hannover, July 2012 Wolfgang Ebeling "
(Prof. Dr. Wolfgang Ebeling, Institute of Algebraic Geometry, |
Also sadly …
Friday, November 14, 2014
Another Opening, Another Show
“What happens when you mix the brilliant wit of Noel Coward
with the intricate plotting of Agatha Christie? Set during a
weekend in an English country manor in 1932, Death by Design
is a delightful and mysterious ‘mash-up’ of two of the greatest
English writers of all time. Edward Bennett, a playwright, and
his wife Sorel Bennett, an actress, flee London and head to
Cookham after a disastrous opening night. But various guests
arrive unexpectedly….”
— Samuel French (theatrical publisher) on a play that
opened in Houston on September 9, 2011.
Related material:
- This journal on May 28, 2014,
- on October 7, 2014,
- on November 13, 2014,
- and on the above opening night, September 9, 2011.
Friday, October 31, 2014
Structure
Introducing a group of 322,560 affine transformations of Dürer’s ‘Magic’ Square
The four vector-space substructures of digits in 1st, 2nd, 3rd, 4th place,
together with the diamond theorem, indicate that Dürer’s square “minus one”
can be transformed by permutations of rows, columns, and quadrants to a
square with (decimal) digits in the usual numerical order, increasing from
top left to bottom right. Such permutations form a group of order 322,560.
(Continued from Vector Addition in a Finite Field, Twelfth Night, 2013.)
Saturday, October 25, 2014
Foundation Square

In the above illustration of the 3-4-5 Pythagorean triangle,
the grids on each side may be regarded as figures of
Euclidean geometry or of Galois geometry.
In Euclidean geometry, these grids illustrate a property of
the inner triangle.
In elementary Galois geometry, ignoring the connection with
the inner triangle, the grids may be regarded instead as
illustrating vector spaces over finite (i.e., Galois) fields.
Previous posts in this journal have dealt with properties of
the 3×3 and 4×4 grids. This suggests a look at properties of
the next larger grid, the 5×5 array, viewed as a picture of the
two-dimensional vector space (or affine plane) over the finite
Galois field GF(5) (also known as ℤ5).
The 5×5 array may be coordinatized in a natural way, as illustrated
in (for instance) Matters Mathematical , by I.N. Herstein and
Irving Kaplansky, 2nd ed., Chelsea Publishing, 1978, p. 171:

See Herstein and Kaplansky for the elementary Galois geometry of
the 5×5 array.
For 5×5 geometry that is not so elementary, see…
-
"The Hoffman-Singleton Graph and its Automorphisms," by
Paul R. Hafner, Journal of Algebraic Combinatorics , 18 (2003), 7–12, and -
the Web pages "Hoffman-Singleton Graph" and "Higman-Sims Graph"
of A. E. Brouwer.
Hafner's abstract:
We describe the Hoffman-Singleton graph geometrically, showing that
it is closely related to the incidence graph of the affine plane over ℤ5.
This allows us to construct all automorphisms of the graph.
The remarks of Brouwer on graphs connect the 5×5-related geometry discussed
by Hafner with the 4×4 geometry related to the Steiner system S(5,8,24).
(See the Miracle Octad Generator of R. T. Curtis and the related coordinatization
by Cullinane of the 4×4 array as a four-dimensional vector space over GF(2).)
Sunday, September 21, 2014
Uncommon Noncore
This post was suggested by Greg Gutfeld’s Sept. 4 remarks on Common Core math.
Problem: What is 9 + 6 ?
Here are two approaches suggested by illustrations of Desargues’s theorem.
Solution 1:
9 + 6 = 10 + 5,
as in Common Core (or, more simply, as in common sense), and
10 + 5 = 5 + 10 = 15 as in Veblen and Young:

Solution 2:
In the figure below,
9 + 6 = no. of V’s + no. of A’s + no. of C’s =
no. of nonempty squares = 16 – 1 = 15.
(Illustration from Feb. 10, 2014.)

The silly educationists’ “partner, anchor, decompose” jargon
discussed by Gutfeld was their attempt to explain “9 + 6 = 10 + 5.”
As he said of the jargon, “That’s not math, that’s the plot from ‘Silence of the Lambs.'”
Or from Richard, Frank, and Marcus in last night’s “Intruders”
(BBC America, 10 PM).
Saturday, August 30, 2014
Physics and Theology
The titles of the previous three posts refer to
Hermann Weyl’s 1918 book Raum, Zeit, Materie
(Space, Time, Matter).
This suggests a look at a poetically parallel 1950 title —
The Lion, the Witch and the Wardrobe —
and at its underlying philosophy:

I am among “those who do not know that this great myth became Fact.”
I do, however, note that some other odd things have become fact.
Those who wish more on this topic may consult:
- The Metaphysics of the Incarnation , from
Oxford University Press on Epiphany 2011. - Epiphany Riddle, from that same date.
- Incarnation in this journal.
Thursday, July 31, 2014
Zero System
The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and
"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."
See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.
An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):

See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Sunday, July 6, 2014
Sticks and Stones
The title is from this morning's previous post.
From a theater review in that post—
… "all flying edges and angles, a perpetually moving and hungry soul"
… "a formidably centered presence, the still counterpoint"
A more abstract perspective:
See also Desargues via Galois (August 6, 2013).
Sunday, June 8, 2014
Vide

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Tuesday, June 3, 2014
Robert Steinberg, 1922-2014
Galois matrices, the subject of the previous post,
are of course not new. See, for instance, Steinberg in 1951:

The American Mathematical Society reports that Steinberg died
on May 25, 2014.
As the above 1951 paper indicates, Steinberg was well acquainted with
what Weyl called "the devil of abstract algebra." In this journal, however,
Steinberg himself appears rather as an angel of geometry.
Wednesday, May 21, 2014
The Tetrahedral Model of PG(3,2)
The page of Whitehead linked to this morning
suggests a review of Polster's tetrahedral model
of the finite projective 3-space PG(3,2) over the
two-element Galois field GF(2).


|
The above passage from Whitehead's 1906 book suggests
that the tetrahedral model may be older than Polster thinks.
Shown at right below is a correspondence between Whitehead's
version of the tetrahedral model and my own square model,
based on the 4×4 array I call the Galois tesseract (at left below).
(Click to enlarge.)
Tuesday, May 20, 2014
Play
From a recreational-mathematics weblog yesterday:
"This appears to be the arts section of the post,
so I’ll leave Martin Probert’s page on
The Survival, Origin and Mathematics of String Figures
here. I’ll be back to pick it up at the end. Maybe it’d like
to play with Steven H. Cullinane’s pages on the
Finite Geometry of the Square and Cube."
I doubt they would play well together.
Perhaps the offensive linking of the purely recreational topic
of string figures to my own work was suggested by the
string figures' resemblance to figures of projective geometry.
A pairing I prefer: Desargues and Galois —
For further details, see posts on Desargues and Galois.
Sunday, April 27, 2014
Sunday School
Galois and Abel vs. Rubik
“Abel was done to death by poverty, Galois by stupidity.
In all the history of science there is no completer example
of the triumph of crass stupidity….”
— Eric Temple Bell, Men of Mathematics
Gray Space (Continued)
… For The Church of Plan 9.
Tuesday, March 11, 2014
Depth
"… this notion of ‘depth’ is an elusive one
even for a mathematician who can recognize it…."
— G. H. Hardy, A Mathematician's Apology
Part I: An Inch Deep

Part II: An Inch Wide
See a search for "square inch space" in this journal.
See also recent posts with the tag depth.
Sunday, March 2, 2014
Sermon
Raiders of the Lost (Continued)
"Socrates: They say that the soul of man is immortal…."
From August 16, 2012—
In the geometry of Plato illustrated below,
"the figure of eight [square] feet" is not , at this point
in the dialogue, the diamond in Jowett's picture.
An 1892 figure by Jowett illustrating Plato's Meno—
A more correct version, from hermes-press.com —
|
Socrates: He only guesses that because the square is double, the line is double.Meno: True.
Socrates: Observe him while he recalls the steps in regular order. (To the Boy.) Tell me, boy, do you assert that a double space comes from a double line? Remember that I am not speaking of an oblong, but of a figure equal every way, and twice the size of this-that is to say of eight feet; and I want to know whether you still say that a double square comes from double line? [Boy] Yes. Socrates: But does not this line (AB) become doubled if we add another such line here (BJ is added)? [Boy] Certainly.
Socrates: And four such lines [AJ, JK, KL, LA] will make a space containing eight feet? [Boy] Yes. Socrates: Let us draw such a figure: (adding DL, LK, and JK). Would you not say that this is the figure of eight feet? [Boy] Yes. Socrates: And are there not these four squares in the figure, each of which is equal to the figure of four feet? (Socrates draws in CM and CN) [Boy] True. Socrates: And is not that four times four? [Boy] Certainly. Socrates: And four times is not double? [Boy] No, indeed. Socrates: But how much? [Boy] Four times as much. Socrates: Therefore the double line, boy, has given a space, not twice, but four times as much. [Boy] True. Socrates: Four times four are sixteen— are they not? [Boy] Yes. |
As noted in the 2012 post, the diagram of greater interest is
Jowett's incorrect version rather than the more correct version
shown above. This is because the 1892 version inadvertently
illustrates a tesseract:
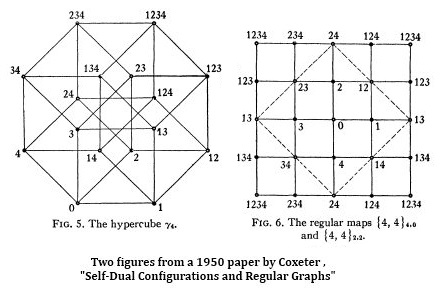
A 4×4 square version, by Coxeter in 1950, of a tesseract—
This square version we may call the Galois tesseract.
Saturday, February 15, 2014
Rosenhain and Göpel
See The Oslo Version in this journal and the New Year’s Day 2014 post.
The pictures of the 56 spreads in that post (shown below) are based on
the 20 Rosenhain and 15 Göpel tetrads that make up the 35 lines of
PG(3,2), the finite projective 3-space over the 2-element Galois field.
Click for a larger image.
Friday, January 17, 2014
The 4×4 Relativity Problem
The sixteen-dot square array in yesterday’s noon post suggests
the following remarks.
“This is the relativity problem: to fix objectively a class of
equivalent coordinatizations and to ascertain the group of
transformations S mediating between them.”
— Hermann Weyl, The Classical Groups ,
Princeton University Press, 1946, p. 16
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977—
The 1977 matrix Q is echoed in the following from 2002—

A different representation of Cullinane’s 1977 square model of the
16-point affine geometry over the two-element Galois field GF(2)
is supplied by Conway and Sloane in Sphere Packings, Lattices and Groups
(first published in 1988) :
Here a, b, c, d are basis vectors in the vector 4-space over GF(2).
(For a 1979 version of this vector space, see AMS Abstract 79T-A37.)
See also a 2011 publication of the Mathematical Association of America —
Sunday, December 15, 2013
Sermon
Odin's Jewel
Jim Holt, the author of remarks in yesterday's
Saturday evening post—
"It turns out that the Kyoto school of Buddhism
makes Heidegger seem like Rush Limbaugh—
it’s so rarified, I’ve never been able to
understand it at all. I’ve been knocking my head
against it for years."
— Vanity Fair Daily , July 16, 2012

Backstory: Odin + Jewel in this journal.
See also Odin on the Kyoto school —
For another version of Odin's jewel, see Log24
on the date— July 16, 2012— that Holt's Vanity Fair
remarks were published. Scroll to the bottom of the
"Mapping Problem continued" post for an instance of
the Galois tesseract —
Thursday, December 12, 2013
Outsider Art
"… Galois was a mathematical outsider…."
— Tony Mann, "head of the department of mathematical sciences,
University of Greenwich, and president, British Society for the
History of Mathematics," in a May 6, 2010, review of Duel at Dawn
in Times Higher Education.
Related art:
(Click for a larger image.)
For a less outside version of the central image
above, see Kunstkritikk on Oct. 15, 2013.
Thursday, December 5, 2013
Saturday, September 21, 2013
Mathematics and Narrative (continued)
Mathematics:
A review of posts from earlier this month —
Wednesday, September 4, 2013
|
Narrative:

Aooo.
Happy birthday to Stephen King.
Thursday, September 5, 2013
Wednesday, September 4, 2013
Friday, August 16, 2013
Six-Set Geometry
From April 23, 2013, in
"Classical Geometry in Light of Galois Geometry"—
Click above image for some background from 1986.
Related material on six-set geometry from the classical literature—
Baker, H. F., "Note II: On the Hexagrammum Mysticum of Pascal,"
in Principles of Geometry , Vol. II, Camb. U. Press, 1930, pp. 219-236
Richmond, H. W., "The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen (1900), Volume 53, Issue 1-2, pp 161-176
Richmond, H. W., "On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics , Vol. 31 (1900), pp. 125-160
Monday, August 12, 2013
Form
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977—
The Galois tesseract is the basis for a representation of the smallest
projective 3-space, PG(3,2), that differs from the representation at
Wolfram Demonstrations Project. For the latter, see yesterday’s post.
The tesseract representation underlies the diamond theorem, illustrated
below in its earliest form, also from the above February 1977 article—
As noted in a more recent version, the group described by
the diamond theorem is also the group of the 35 square
patterns within the 1976 Miracle Octad Generator (MOG) of
R. T. Curtis.
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—

Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Tuesday, May 28, 2013
Codes
The hypercube model of the 4-space over the 2-element Galois field GF(2):

The phrase Galois tesseract may be used to denote a different model
of the above 4-space: the 4×4 square.
MacWilliams and Sloane discussed the Miracle Octad Generator
(MOG) of R. T. Curtis further on in their book (see below), but did not
seem to realize in 1977 that the 4×4 structures within the MOG are
based on the Galois-tesseract model of the 4-space over GF(2).

The thirty-five 4×4 structures within the MOG:

Curtis himself first described these 35 square MOG patterns
combinatorially, (as his title indicated) rather than
algebraically or geometrically:

A later book co-authored by Sloane, first published in 1988,
did recognize the 4×4 MOG patterns as based on the 4×4
Galois-tesseract model.
Between the 1977 and 1988 Sloane books came the diamond theorem.
Update of May 29, 2013:
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977
(the year the above MacWilliams-Sloane book was first published):
Sunday, May 19, 2013
Priority Claim
From an arXiv preprint submitted July 18, 2011,
and last revised on March 11, 2013 (version 4):
"By our construction, this vector space is the dual
of our hypercube F24 built on I \ O9. The vector space
structure of the latter, to our knowledge, is first
mentioned by Curtis in [Cur89]. Hence altogether
our proposition 2.3.4 gives a novel geometric
meaning in terms of Kummer geometry to the known
vector space structure on I \ O9."
[Cur89] reference:
R. T. Curtis, "Further elementary techniques using
the miracle octad generator," Proc. Edinburgh
Math. Soc. 32 (1989), 345-353 (received on
July 20, 1987).
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ,"
arXiv.org > hep-th > arXiv:1107.3834
"First mentioned by Curtis…."
No. I claim that to the best of my knowledge, the
vector space structure was first mentioned by me,
Steven H. Cullinane, in an AMS abstract submitted
in October 1978, some nine years before the
Curtis article.
|
Update of the above paragraph on July 6, 2013—
No. The vector space structure was described by
The vector space structure as it occurs in a 4×4 array |
See Notes on Finite Geometry for some background.
See in particular The Galois Tesseract.
For the relationship of the 1978 abstract to Kummer
geometry, see Rosenhain and Göpel Tetrads in PG(3,2).
Sunday, April 28, 2013
The Octad Generator
… And the history of geometry —
Desargues, Pascal, Brianchon and Galois
in the light of complete n-points in space.
(Rewritten for clarity at about 10 AM ET April 29, with quote from Dowling added.
Updated with a reference to a Veblen and Young exercise (on p. 53) on April 30.)


Veblen and Young, Projective Geometry, Vol. I ,
Ginn and Company, 1910, page 39:
"The Desargues configuration. A very important configuration
is obtained by taking the plane section of a complete space five-point."

Each of figures 14 and 15 above has 15 points and 20 lines.
The Desargues configuration within each figure is denoted by
10 white points and 10 solid lines, with 3 points on each line and
3 lines on each point. Black points and dashed lines indicate the
complete space five-point and lines connecting it to the plane section
containing the Desargues configuration.
In a 1915 University of Chicago doctoral thesis, Archibald Henderson
used a complete space six -point to construct a configuration of
15 points and 20 lines in the context not of Desargues ' theorem, but
rather of Brianchon 's theorem and of the Pascal hexagram.
Henderson's 1915 configuration is, it turns out, isomorphic to that of
the 15 points and 20 lines in the configuration constructed via a
complete space five -point five years earlier by Veblen and Young.
(See, in Veblen and Young's 1910 Vol. I, exercise 11, page 53:
"A plane section of a 6-point in space can be considered as
3 triangles perspective in pairs from 3 collinear points with
corresponding sides meeting in 3 collinear points." This is the
large Desargues configuration. See Classical Geometry in Light of
Galois Geometry.)
For this large Desargues configuration see April 19.
For Henderson's complete six –point, see The Six-Set (April 23).
That post ends with figures relating the large Desargues configuration
to the Galois geometry PG(3,2) that underlies the Curtis
Miracle Octad Generator and the large Mathieu group M24 —
See also Note on the MOG Correspondence from April 25, 2013.
That correspondence was also discussed in a note 28 years ago, on this date in 1985.
Tuesday, April 9, 2013
Four Quartets
For the cruelest month
Click for a much larger version of the photo below.
These four Kountry Korn quartets are from the Fox Valleyaires
Men's Barbershop Chorus of Appleton, Wisconsin.
See also the fine arts here on Saturday, April 6, 2013—
The New York Times Magazine cover story
a decade ago, on Sunday, April 6, 2003:
"The artists demanded space
in tune with their aesthetic."
— "The Dia Generation,"
by Michael Kimmelman
Related material:
See Wikipedia for the difference between binary numbers
and binary coordinates from the finite Galois field GF(2).
For some background, see the relativity problem.
See also the chapter on vector spaces in Korn & Korn
(originally published by McGraw-Hill)—
 .
.
Monday, April 1, 2013
Desargues via Rosenhain
Background: Rosenhain and Göpel Tetrads in PG(3,2)
|
Introduction: The Large Desargues Configuration Added by Steven H. Cullinane on Friday, April 19, 2013 Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point The converse, from the same book:
"If two triangles are perspective from a line
Desargues' theorem according to Wikipedia
"Two triangles are in perspective axially [i.e., from a line]
A figure often used to illustrate the theorem,
A discussion of the "if and only if" version of the theorem
This large Desargues configuration involves a third triangle,
Point-line incidence in this larger configuration is,
The third triangle, within the larger configuration,
|

A connection discovered today (April 1, 2013)—
(Click to enlarge the image below.)
Update of April 18, 2013
Note that Baker's Desargues-theorem figure has three triangles,
ABC, A'B'C', A"B"C", instead of the two triangles that occur in
the statement of the theorem. The third triangle appears in the
course of proving, not just stating, the theorem (or, more precisely,
its converse). See, for instance, a note on a standard textbook for
further details.
(End of April 18, 2013 update.)
Update of April 14, 2013
See Baker's Proof (Edited for the Web) for a detailed explanation
of the above picture of Baker's Desargues-theorem frontispiece.
(End of April 14, 2013 update.)
Update of April 12, 2013
A different figure, from a site at National Tsing Hua University,
shows the three triangles of Baker's figure more clearly:
(End of update of April 12, 2013)
Update of April 13, 2013
Another in a series of figures illustrating
Desargues's theorem in light of Galois geometry:

See also the original Veblen-Young figure in context.
(End of update of April 13, 2013)

Rota's remarks, while perhaps not completely accurate, provide some context
for the above Desargues-Rosenhain connection. For some other context,
see the interplay in this journal between classical and finite geometry, i.e.
between Euclid and Galois.
For the recent context of the above finite-geometry version of Baker's Vol. I
frontispiece, see Sunday evening's finite-geometry version of Baker's Vol. IV
frontispiece, featuring the Göpel, rather than the Rosenhain, tetrads.
For a 1986 illustration of Göpel and Rosenhain tetrads (though not under
those names), see Picturing the Smallest Projective 3-Space.
In summary… the following classical-geometry figures
are closely related to the Galois geometry PG(3,2):
|
Volume I of Baker's Principles has a cover closely related to the Rosenhain tetrads in PG(3,2) |
Volume IV of Baker's Principles has a cover closely related to the Göpel tetrads in PG(3,2) |
|
Foundations (click to enlarge)
|
Higher Geometry (click to enlarge)
|
Sunday, March 31, 2013
For Baker
Baker, Principles of Geometry, Vol. IV (1925), Title:

Baker, Principles of Geometry, Vol. IV (1925), Frontispiece:

Baker's Vol. IV frontispiece shows "The Figure of fifteen lines
and fifteen points, in space of four dimensions."
Another such figure in a vector space of four dimensions
over the two-element Galois field GF(2):

(Some background grid parts were blanked by an image resizing process.)
Here the "lines" are actually planes in the vector 4-space over GF(2),
but as planes through the origin in that space, they are projective lines .
For some background, see today's previous post and Inscapes.
Update of 9:15 PM March 31—
The following figure relates the above finite-geometry
inscape incidences to those in Baker's frontispiece. Both the inscape
version and that of Baker depict a Cremona-Richmond configuration.

Tuesday, March 12, 2013
Smoke and Mirrors
Sistine Chapel Smoke
Tromso Kunsthall Mirrors
Background for the smoke image:
A remark by Michelangelo in a 2007 post, High Concept.
Background for the mirrors image:
Note the publication date— Mar. 10, 2013.
Monday, February 18, 2013
Permanence
Inscribed hexagon (1984)
The well-known fact that a regular hexagon
may be inscribed in a cube was the basis
in 1984 for two ways of coloring the faces
of a cube that serve to illustrate some graphic
aspects of embodied Galois geometry—
Inscribed hexagon (2013)
A redefinition of the term "symmetry plane"
also uses the well-known inscription
of a regular hexagon in the cube—
Related material
"Here is another way to present the deep question 1984 raises…."
— "The Quest for Permanent Novelty," by Michael W. Clune,
The Chronicle of Higher Education , Feb. 11, 2013
“What we do may be small, but it has a certain character of permanence.”
— G. H. Hardy, A Mathematician’s Apology
Wednesday, February 13, 2013
Form:
Story, Structure, and the Galois Tesseract
Recent Log24 posts have referred to the
"Penrose diamond" and Minkowski space.
The Penrose diamond has nothing whatever
to do with my 1976 monograph "Diamond Theory,"
except for the diamond shape and the connection
of the Penrose diamond to the Klein quadric—

The Klein quadric occurs in the five-dimensional projective space
over a field. If the field is the two-element Galois field GF(2), the
quadric helps explain certain remarkable symmetry properties
of the R. T. Curtis Miracle Octad Generator (MOG), hence of
the large Mathieu group M24. These properties are also
relevant to the 1976 "Diamond Theory" monograph.
For some background on the quadric, see (for instance)…

See also The Klein Correspondence,
Penrose Space-Time, and a Finite Model.
Related material:
|
"… one might crudely distinguish between philosophical – J. M. E. Hyland. "Proof Theory in the Abstract." (pdf) |
Those who prefer story to structure may consult
- today's previous post on the Penrose diamond
- the remarks of Scott Aaronson on August 17, 2012
- the remarks in this journal on that same date
- the geometry of the 4×4 array in the context of M24.
Midnight in the Garden
(Continued, to mark Tuesday's birthdays of Lincoln and Darwin.)
A British reporter who died at 97 on Tuesday is said to have
"covered the space race in its entirety." In his honor, here
in review are posts containing the phrase Space Race
and, more generally, the two words Galois + Space.
Thursday, November 22, 2012
Finite Relativity
(Continued from 1986)
|
S. H. Cullinane This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.
— H. Weyl, The Classical Groups , In finite geometry "points" are often defined as ordered n-tuples of a finite (i.e., Galois) field GF(q). What geometric structures ("frames of reference," in Weyl's terms) are coordinatized by such n-tuples? Weyl's use of "objectively" seems to mean that such structures should have certain objective— i.e., purely geometric— properties invariant under each S. This note suggests such a frame of reference for the affine 4-space over GF(2), and a class of 322,560 equivalent coordinatizations of the frame. The frame: A 4×4 array. The invariant structure: The following set of 15 partitions of the frame into two 8-sets.
A representative coordinatization:
0000 0001 0010 0011
The group: The group AGL(4,2) of 322,560 regular affine transformations of the ordered 4-tuples over GF(2). |
S. H. Cullinane This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.
— H. Weyl, The Classical Groups , In finite geometry "points" are often defined as ordered n-tuples of a finite (i.e., Galois) field GF(q). What geometric structures ("frames of reference," in Weyl's terms) are coordinatized by such n-tuples? Weyl's use of "objectively" seems to mean that such structures should have certain objective— i.e., purely geometric— properties invariant under each S. This note suggests such a frame of reference for the affine 4-space over GF(2), and a class of 322,560 equivalent coordinatizations of the frame. The frame: An array of 16 congruent equilateral subtriangles that make up a larger equilateral triangle. The invariant structure: The following set of 15 partitions of the frame into two 8-sets.
The group: The group AGL(4,2) of 322,560 regular affine transformations of the ordered 4-tuples over GF(2). |
For some background on the triangular version,
see the Square-Triangle Theorem,
noting particularly the linked-to coordinatization picture.
Tuesday, October 16, 2012
Cube Review
Last Wednesday's 11 PM post mentioned the
adjacency-isomorphism relating the 4-dimensional
hypercube over the 2-element Galois field GF(2) to
the 4×4 array made up of 16 square cells, with
opposite edges of the 4×4 array identified.
A web page illustrates this property with diagrams that
enjoy the Karnaugh property— adjacent vertices, or cells,
differ in exactly one coordinate. A brief paper by two German
authors relates the Karnaugh property to the construction
of a magic square like that of Dürer (see last Wednesday).
In a similar way (search the Web for Karnaugh + cube ),
vertex adjacency in the 6-dimensional hypercube over GF(2)
is isomorphic to cell adjacency in the 4x4x4 cube, with
opposite faces of the 4x4x4 cube identified.
The above cube may be used to illustrate some properties
of the 64-point Galois 6-space that are more advanced
than those studied by enthusiasts of "magic" squares
and cubes.
See
- the 4x4x4 cube and An Invariance of Symmetry
- the 4x4x4 cube and the nineteenth-century
geometers' "Solomon's seal" - the 4x4x4 cube and the Kummer surface
- the 4x4x4 cube and the Klein quadric.
Those who prefer narrative to mathematics may
consult posts in this journal containing the word "Cuber."
Wednesday, August 1, 2012
Elementary Finite Geometry
I. General finite geometry (without coordinates):
A finite affine plane of order n has n^2 points.
A finite projective plane of order n has n^2 + n + 1
points because it is formed from an order-n finite affine
plane by adding a line at infinity that contains n + 1 points.
Examples—
|
|
|
II. Galois finite geometry (with coordinates over a Galois field):
A finite projective Galois plane of order n has n^2 + n + 1
points because it is formed from a finite affine Galois 3-space
of order n with n^3 points by discarding the point (0,0,0) and
identifying the points whose coordinates are multiples of the
(n-1) nonzero scalars.
Note: The resulting Galois plane of order n has
(n^3-1)/(n-1)= (n^2 + n + 1) points because
(n^2 + n + 1)(n – 1) =
(n^3 + n^2 + n – n^2 – n – 1) = (n^3 – 1) .
III. Related art:
Another version of a 1994 picture that accompanied a New Yorker
article, "Atheists with Attitude," in the issue dated May 21, 2007:

The Four Gods of Borofsky correspond to the four axes of
symmetry of a square and to the four points on a line at infinity
in an order-3 projective plane as described in Part I above.
Those who prefer literature to mathematics may, if they like,
view the Borofsky work as depicting
"Blake's Four Zoas, which represent four aspects
of the Almighty God" —Wikipedia
Wednesday, July 11, 2012
Cuber
For Pete Rustan, space recon expert, who died on June 28—
See also Galois vs. Rubik and Group Theory Template.
Thursday, May 3, 2012
Everybody Comes to Rick’s

“The key is the cocktail that begins the proceedings.”
– Brian Harley, Mate in Two Moves
See also yesterday's Endgame , as well as Play and Interplay
from April 28… and, as a key, the following passage from
an earlier April 28 post—
| Euclidean geometry has long been applied to physics; Galois geometry has not. The cited webpage describes the interplay of both sorts of geometry— Euclidean and Galois, continuous and discrete— within physical space— if not within the space of physics . |
Saturday, April 28, 2012
Sprechen Sie Deutsch?
A Log24 post, "Bridal Birthday," one year ago today linked to
"The Discrete and the Continuous," a brief essay by David Deutsch.
From that essay—
"The idea of quantization—
the discreteness of physical quantities—
turned out to be immensely fruitful."
Deutsch's "idea of quantization" also appears in
the April 12 Log24 post Mythopoetic—
|
"Is Space Digital?" — Cover story, Scientific American "The idea that space may be digital — Physicist Sabine Hossenfelder "A quantization of space/time — Peter Woit in a comment |
It seems some clarification is in order.
Hossenfelder's "The idea that space may be digital"
and Woit's "a quantization of space/time" may not
refer to the same thing.
Scientific American on the concept of digital space—
"Space may not be smooth and continuous.
Instead it may be digital, composed of tiny bits."
Wikipedia on the concept of quantization—
Causal sets, loop quantum gravity, string theory,
and black hole thermodynamics all predict
a quantized spacetime….
For a purely mathematical approach to the
continuous-vs.-discrete issue, see
Finite Geometry and Physical Space.
The physics there is somewhat tongue-in-cheek,
but the geometry is serious.The issue there is not
continuous-vs.-discrete physics , but rather
Euclidean-vs.-Galois geometry .
Both sorts of geometry are of course valid.
Euclidean geometry has long been applied to
physics; Galois geometry has not. The cited
webpage describes the interplay of both sorts
of geometry— Euclidean and Galois, continuous
and discrete— within physical space— if not
within the space of physics.
Thursday, April 12, 2012
Mythopoetic*
"Is Space Digital?"
— Cover story, Scientific American magazine, February 2012
"The idea that space may be digital
is a fringe idea of a fringe idea
of a speculative subfield of a subfield."
— Physicist Sabine Hossenfelder
at her weblog on Feb. 5, 2012
"A quantization of space/time
is a holy grail for many theorists…."
— Peter Woit in a comment at his physics weblog today
See also
* See yesterday's Steiner's Systems.
Monday, February 20, 2012
Coxeter and the Relativity Problem
In the Beginning…
"As is well known, the Aleph is the first letter of the Hebrew alphabet."
– Borges, "The Aleph" (1945)
From some 1949 remarks of Weyl—
"The relativity problem is one of central significance throughout geometry and algebra and has been recognized as such by the mathematicians at an early time."
— Hermann Weyl, "Relativity Theory as a Stimulus in Mathematical Research," Proceedings of the American Philosophical Society , Vol. 93, No. 7, Theory of Relativity in Contemporary Science: Papers Read at the Celebration of the Seventieth Birthday of Professor Albert Einstein in Princeton, March 19, 1949 (Dec. 30, 1949), pp. 535-541
Weyl in 1946—:
"This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them."
— Hermann Weyl, The Classical Groups , Princeton University Press, 1946, p. 16
Coxeter in 1950 described the elements of the Galois field GF(9) as powers of a primitive root and as ordered pairs of the field of residue-classes modulo 3—
"… the successive powers of the primitive root λ or 10 are
λ = 10, λ2 = 21, λ3 = 22, λ4 = 02,
λ5 = 20, λ6 = 12, λ7 = 11, λ8 = 01.
These are the proper coordinate symbols….
(See Fig. 10, where the points are represented in the Euclidean plane as if the coordinate residue 2 were the ordinary number -1. This representation naturally obscures the collinearity of such points as λ4, λ5, λ7.)"
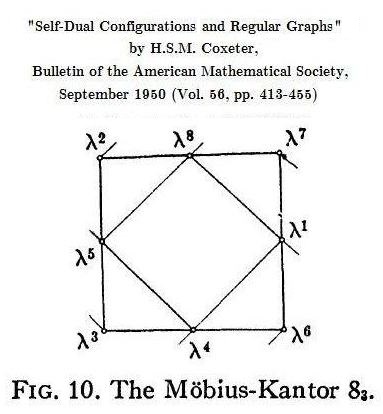
Coxeter's Figure 10 yields...

The Aleph
The details:
Coxeter's phrase "in the Euclidean plane" obscures the noncontinuous nature of the transformations that are automorphisms of the above linear 2-space over GF(3).
Saturday, February 18, 2012
Symmetry
From the current Wikipedia article "Symmetry (physics)"—
"In physics, symmetry includes all features of a physical system that exhibit the property of symmetry—that is, under certain transformations, aspects of these systems are 'unchanged', according to a particular observation. A symmetry of a physical system is a physical or mathematical feature of the system (observed or intrinsic) that is 'preserved' under some change.
A family of particular transformations may be continuous (such as rotation of a circle) or discrete (e.g., reflection of a bilaterally symmetric figure, or rotation of a regular polygon). Continuous and discrete transformations give rise to corresponding types of symmetries. Continuous symmetries can be described by Lie groups while discrete symmetries are described by finite groups (see Symmetry group)."….
"A discrete symmetry is a symmetry that describes non-continuous changes in a system. For example, a square possesses discrete rotational symmetry, as only rotations by multiples of right angles will preserve the square's original appearance."
Note the confusion here between continuous (or discontinuous) transformations and "continuous" (or "discontinuous," i.e. "discrete") groups .
This confusion may impede efforts to think clearly about some pure mathematics related to current physics— in particular, about the geometry of spaces made up of individual units ("points") that are not joined together in a continuous manifold.
For an attempt to forestall such confusion, see Noncontinuous Groups.
For related material, see Erlanger and Galois as well as the opening paragraphs of Diamond Theory—
Symmetry is often described as invariance under a group of transformations. An unspoken assumption about symmetry in Euclidean 3-space is that the transformations involved are continuous.
Diamond theory rejects this assumption, and in so doing reveals that Euclidean symmetry may itself be invariant under rather interesting groups of non-continuous (and a-symmetric) transformations. (These might be called noncontinuous groups, as opposed to so-called discontinuous (or discrete ) symmetry groups. See Weyl's Symmetry .)
For example, the affine group A on the 4-space over the 2-element field has a natural noncontinuous and asymmetric but symmetry-preserving action on the elements of a 4×4 array. (Details)
(Version first archived on March 27, 2002)
Update of Sunday, February 19, 2012—
The abuse of language by the anonymous authors
of the above Wikipedia article occurs also in more
reputable sources. For instance—

Some transformations referred to by Brading and Castellani
and their editees as "discrete symmetries" are, in fact, as
linear transformations of continuous spaces, themselves
continuous transformations.
This unfortunate abuse of language is at least made explicit
in a 2003 text, Mathematical Perspectives on Theoretical
Physics (Nirmala Prakash, Imperial College Press)—
"… associated[*] with any given symmetry there always exists
a continuous or a discrete group of transformations….
A symmetry whose associated group is continuous (discrete)
is called a continuous (discrete ) symmetry ." — Pp. 235, 236
[* Associated how?]
Logo
Pentagram design agency on the new Windows 8 logo—
"… the logo re-imagines the familiar four-color symbol
as a modern geometric shape"—

Sam Moreau, Principal Director of User Experience for Windows,
yesterday—
On Redesigning the Windows Logo—
"To see what is in front of one's nose
needs a constant struggle." —George Orwell
That is the feeling we had when Paula Scher
(from the renowned Pentagram design agency)
showed us her sketches for the new Windows logo.
Related material:
- A Four-Color Theorem,
- The Galois Window, and
-
An illustration from
Finite Geometry of the Square and Cube—

Monday, January 23, 2012
How It Works
J. H. Conway in 1971 discussed the role of an elementary abelian group
of order 16 in the Mathieu group M24. His approach at that time was
purely algebraic, not geometric—

For earlier (and later) discussions of the geometry (not the algebra )
of that order-16 group (i.e., the group of translations of the affine space
of 4 dimensions over the 2-element field), see The Galois Tesseract.
Sunday, August 28, 2011
The Cosmic Part
Yesterday’s midday post, borrowing a phrase from the theology of Marvel Comics,
offered Rubik’s mechanical contrivance as a rather absurd “Cosmic Cube.”
A simpler candidate for the “Cube” part of that phrase:

The Eightfold Cube
As noted elsewhere, a simple reflection group* of order 168 acts naturally on this structure.
“Because of their truly fundamental role in mathematics,
even the simplest diagrams concerning finite reflection groups
(or finite mirror systems, or root systems—
the languages are equivalent) have interpretations
of cosmological proportions.”
— Alexandre V. Borovik in “Coxeter Theory: The Cognitive Aspects“
Borovik has a such a diagram—

The planes in Borovik’s figure are those separating the parts of the eightfold cube above.
In Coxeter theory, these are Euclidean hyperplanes. In the eightfold cube, they represent three of seven projective points that are permuted by the above group of order 168.
In light of Borovik’s remarks, the eightfold cube might serve to illustrate the “Cosmic” part of the Marvel Comics phrase.
For some related theological remarks, see Cube Trinity in this journal.
Happy St. Augustine’s Day.
* I.e., one generated by reflections : group actions that fix a hyperplane pointwise. In the eightfold cube, viewed as a vector space of 3 dimensions over the 2-element Galois field, these hyperplanes are certain sets of four subcubes.
Wednesday, April 27, 2011
Tuesday, April 19, 2011
Romancing the Omega
Today's news from Oslo suggests a review—
The circular sculpture in the foreground
is called by the artist "The Omega Point."
This has been described as
"a portal that leads in or out of time and space."
Some related philosophical remarks—
Sunday, April 17, 2011
Annals of Search
The following has rather mysteriously appeared in a search at Google Scholar for "Steven H. Cullinane."
|
[HTML] Romancing the Non-Euclidean Hyperspace |
This turns out to be a link to a search within this weblog. I do not know why Google Scholar attributes the resulting web page to a journal article by "AB Story" or why it drew the title from a post within the search and applied it to the entire list of posts found. I am, however, happy with the result— a Palm Sunday surprise with an eclectic mixture of styles that might please the late Robert de Marrais.
I hope the late George Temple would also be pleased. He appears in "Romancing" as a resident of Quarr Abbey, a Benedictine monastery.
The remarks by Martin Hyland quoted in connection with Temple's work are of particular interest in light of the Pope's Christmas remark on mathematics quoted here yesterday.
Thursday, March 10, 2011
Paradigms Lost
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—

This journal on January 19, 2011—

If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
Sunday, February 20, 2011
Sermon
From Galleri MGM in Oslo —
A connection to today's earlier post, Sunday School— The Oslo Version, from Friday, May 21, 2010.
Lyche's "Omega Point" portal, together with her last name, suggested three posts from the following Saturday morning— which later proved to be the date of Martin Gardner's death—
Art Space, Through the Lyche Gate and The Lyche Gate Asterisk.
For some further religious remarks, see November 9th, 2010— A Theory of Pure Design.
Wednesday, January 19, 2011
Intermediate Cubism
The following is a new illustration for Cubist Geometries—

(For elementary cubism, see Pilate Goes to Kindergarten and The Eightfold Cube.
For advanced, see Solomon's Cube and Geometry of the I Ching .)
Thursday, January 6, 2011
Epiphany Riddle
"Spaces and geometries, those which we perceive,
which we can’t perceive, or which only some of us perceive,
are a recurring theme in Against the Day ."
"大哉大哉 宇宙之谜
美哉美哉 真理之源"
"Great indeed is the riddle of the universe.
Beautiful indeed is the source of truth."
— Shing-Tung Yau, Chairman,
Department of Mathematics, Harvard University
"Always keep a diamond in your mind."
— King Solomon at the Paradiso
Image from stoneship.org
Monday, December 13, 2010
Mathematics and Narrative continued…
Apollo's 13: A Group Theory Narrative —
I. At Wikipedia —

II. Here —
See Cube Spaces and Cubist Geometries.
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the 27-part (Galois) 3×3×3 cube–
A note from 1985 describing group actions on a 3×3 plane array—

Undated software by Ed Pegg Jr. displays
group actions on a 3×3×3 cube that extend the
3×3 group actions from 1985 described above—
Pegg gives no reference to the 1985 work on group actions.
Friday, October 22, 2010
Moreover
Google News this afternoon—
Related material:
- Celebration of Mind (Oct. 20)
- Problem Picture (Oct. 21)
- Hereafter (Oct. 22)
- The Gypsy in The French Mathematician
Thursday, July 22, 2010
Pilate Goes to Kindergarten, continued
Barnes & Noble has an informative new review today of the recent Galois book Duel at Dawn.
It begins…
"In 1820, the Hungarian noble Farkas Bolyai wrote an impassioned cautionary letter to his son Janos:
'I know this way to the very end. I have traversed this bottomless night, which extinguished all light and joy in my life… It can deprive you of your leisure, your health, your peace of mind, and your entire happiness… I turned back when I saw that no man can reach the bottom of this night. I turned back unconsoled, pitying myself and all mankind. Learn from my example…'
Bolyai wasn't warning his son off gambling, or poetry, or a poorly chosen love affair. He was trying to keep him away from non-Euclidean geometry."
For a less dark view (obtained by simply redefining "non-Euclidean" in a more logical way*) see Non-Euclidean Blocks and Finite Geometry and Physical Space.
* Finite geometry is not Euclidean geometry— and is, therefore, non-Euclidean
in the strictest sense (though not according to popular usage), simply because
Euclidean geometry has infinitely many points, and a finite geometry does not.
(This more logical definition of "non-Euclidean" seems to be shared by
at least one other person.)
And some finite geometries are non-Euclidean in the popular-usage sense,
related to Euclid's parallel postulate.
The seven-point Fano plane has, for instance, been called
"a non-Euclidean geometry" not because it is finite
(though that reason would suffice), but because it has no parallel lines.
(See the finite geometry page at the Centre for the Mathematics
of Symmetry and Computation at the University of Western Australia.)
Thursday, June 24, 2010
Midsummer Noon
Geometry Simplified

(a projective space)
The above finite projective space
is the simplest nontrivial example
of a Galois geometry (i.e., a finite
geometry with coordinates in a
finite (that is, Galois) field.)
The vertical (Euclidean) line represents a
(Galois) point, as does the horizontal line
and also the vertical-and-horizontal
cross that represents the first two points'
binary sum (i.e., symmetric difference,
if the lines are regarded as sets).
Homogeneous coordinates for the
points of this line —
(1,0), (0,1), (1,1).
Here 0 and 1 stand for the elements
of the two-element Galois field GF(2).
The 3-point line is the projective space
corresponding to the affine space
(a plane, not a line) with four points —

(an affine space)
The (Galois) points of this affine plane are
not the single and combined (Euclidean)
line segments that play the role of
points in the 3-point projective line,
but rather the four subsquares
that the line segments separate.
For further details, see Galois Geometry.
There are, of course, also the trivial
two-point affine space and the corresponding
trivial one-point projective space —

Here again, the points of the affine space are
represented by squares, and the point of the
projective space is represented by a line segment
separating the affine-space squares.
Wednesday, June 16, 2010
Geometry of Language
(Continued from April 23, 2009, and February 13, 2010.)
Paul Valéry as quoted in yesterday’s post:
“The S[elf] is invariant, origin, locus or field, it’s a functional property of consciousness” (Cahiers, 15:170 [2: 315])
The geometric example discussed here yesterday as a Self symbol may seem too small to be really impressive. Here is a larger example from the Chinese, rather than European, tradition. It may be regarded as a way of representing the Galois field GF(64). (“Field” is a rather ambiguous term; here it does not, of course, mean what it did in the Valéry quotation.)
From Geometry of the I Ching—

The above 64 hexagrams may also be regarded as
the finite affine space AG(6,2)— a larger version
of the finite affine space AG(4,2) in yesterday’s post.
That smaller space has a group of 322,560 symmetries.
The larger hexagram space has a group of
1,290,157,424,640 affine symmetries.
From a paper on GL(6,2), the symmetry group
of the corresponding projective space PG(5,2),*
which has 1/64 as many symmetries—
For some narrative in the European tradition
related to this geometry, see Solomon’s Cube.
* Update of July 29, 2011: The “PG(5,2)” above is a correction from an earlier error.
Monday, May 10, 2010
Requiem for Georgia Brown
Paul Robeson in
"King Solomon's Mines," 1937—
The image above is an illustration from
"Romancing the Hyperspace," May 4, 2010.
This illustration, along with Georgia Brown's
song from "Cabin in the Sky"—
"There's honey in the honeycomb"—
suggests the following picture.
"What might have been and what has been
Point to one end, which is always present."
— Four Quartets
Tuesday, May 4, 2010
Mathematics and Narrative, continued
Romancing the
Non-Euclidean Hyperspace
Backstory —
Mere Geometry, Types of Ambiguity,
Dream Time, and Diamond Theory, 1937

For the 1937 grid, see Diamond Theory, 1937.
The grid is, as Mere Geometry points out, a non-Euclidean hyperspace.
For the diamonds of 2010, see Galois Geometry and Solomon’s Cube.
Monday, April 26, 2010
Types of Ambiguity
From Ursula K. Le Guin’s novel
The Dispossessed: An Ambiguous Utopia (1974)—
Chapter One
“There was a wall. It did not look important. It was built of uncut rocks roughly mortared. An adult could look right over it, and even a child could climb it. Where it crossed the roadway, instead of having a gate it degenerated into mere geometry, a line, an idea of boundary. But the idea was real. It was important. For seven generations there had been nothing in the world more important than that wall.
Like all walls it was ambiguous, two-faced. What was inside it and what was outside it depended upon which side of it you were on.”
Note—
“We note that the phrase ‘instead of having a gate it degenerated into mere geometry’ is mere fatuousness. If there is an idea here, degenerate, mere, and geometry in concert do not fix it. They bat at it like a kitten at a piece of loose thread.”
— Samuel R. Delany, The Jewel-Hinged Jaw: Notes on the Language of Science Fiction (Dragon Press, 1977), page 110 of revised edition, Wesleyan University Press, 2009
(For the phrase mere geometry elsewhere, see a note of April 22. The apparently flat figures in that note’s illustration “Galois Affine Geometry” may be regarded as degenerate views of cubes.)
Later in the Le Guin novel—
“… The Terrans had been intellectual imperialists, jealous wall builders. Even Ainsetain, the originator of the theory, had felt compelled to give warning that his physics embraced no mode but the physical and should not be taken as implying the metaphysical, the philosophical, or the ethical. Which, of course, was superficially true; and yet he had used number, the bridge between the rational and the perceived, between psyche and matter, ‘Number the Indisputable,’ as the ancient founders of the Noble Science had called it. To employ mathematics in this sense was to employ the mode that preceded and led to all other modes. Ainsetain had known that; with endearing caution he had admitted that he believed his physics did, indeed, describe reality.
Strangeness and familiarity: in every movement of the Terran’s thought Shevek caught this combination, was constantly intrigued. And sympathetic: for Ainsetain, too, had been after a unifying field theory. Having explained the force of gravity as a function of the geometry of spacetime, he had sought to extend the synthesis to include electromagnetic forces. He had not succeeded. Even during his lifetime, and for many decades after his death, the physicists of his own world had turned away from his effort and its failure, pursuing the magnificent incoherences of quantum theory with its high technological yields, at last concentrating on the technological mode so exclusively as to arrive at a dead end, a catastrophic failure of imagination. Yet their original intuition had been sound: at the point where they had been, progress had lain in the indeterminacy which old Ainsetain had refused to accept. And his refusal had been equally correct– in the long run. Only he had lacked the tools to prove it– the Saeba variables and the theories of infinite velocity and complex cause. His unified field existed, in Cetian physics, but it existed on terms which he might not have been willing to accept; for the velocity of light as a limiting factor had been essential to his great theories. Both his Theories of Relativity were as beautiful, as valid, and as useful as ever after these centuries, and yet both depended upon a hypothesis that could not be proved true and that could be and had been proved, in certain circumstances, false.
But was not a theory of which all the elements were provably true a simple tautology? In the region of the unprovable, or even the disprovable, lay the only chance for breaking out of the circle and going ahead.
In which case, did the unprovability of the hypothesis of real coexistence– the problem which Shevek had been pounding his head against desperately for these last three days. and indeed these last ten years– really matter?
He had been groping and grabbing after certainty, as if it were something he could possess. He had been demanding a security, a guarantee, which is not granted, and which, if granted, would become a prison. By simply assuming the validity of real coexistence he was left free to use the lovely geometries of relativity; and then it would be possible to go ahead. The next step was perfectly clear. The coexistence of succession could be handled by a Saeban transformation series; thus approached, successivity and presence offered no antithesis at all. The fundamental unity of the Sequency and Simultaneity points of view became plain; the concept of interval served to connect the static and the dynamic aspect of the universe. How could he have stared at reality for ten years and not seen it? There would be no trouble at all in going on. Indeed he had already gone on. He was there. He saw all that was to come in this first, seemingly casual glimpse of the method, given him by his understanding of a failure in the distant past. The wall was down. The vision was both clear and whole. What he saw was simple, simpler than anything else. It was simplicity: and contained in it all complexity, all promise. It was revelation. It was the way clear, the way home, the light.”
Related material—
Time Fold, Halloween 2005, and May and Zan.
See also The Devil and Wallace Stevens—
“In a letter to Harriet Monroe, written December 23, 1926, Stevens refers to the Sapphic fragment that invokes the genius of evening: ‘Evening star that bringest back all that lightsome Dawn hath scattered afar, thou bringest the sheep, thou bringest the goat, thou bringest the child home to the mother.’ Christmas, writes Stevens, ‘is like Sappho’s evening: it brings us all home to the fold’ (Letters of Wallace Stevens, 248).”
— “The Archangel of Evening,” Chapter 5 of Wallace Stevens: The Intensest Rendezvous, by Barbara M. Fisher, The University Press of Virginia, 1990
Thursday, April 22, 2010
Mere Geometry

Stanford Encyclopedia of Philosophy —
“Mereology (from the Greek μερος, ‘part’) is the theory of parthood relations: of the relations of part to whole and the relations of part to part within a whole. Its roots can be traced back to the early days of philosophy, beginning with the Presocratics….”
A non-Euclidean* approach to parts–
Corresponding non-Euclidean*
projective points —

Richard J. Trudeau in The Non-Euclidean Revolution, chapter on “Geometry and the Diamond Theory of Truth”–
“… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions:
(1) Diamonds– informative, certain truths about the world– exist.
(2) The theorems of Euclidean geometry are diamonds.
Presumption (1) is what I referred to earlier as the ‘Diamond Theory’ of truth. It is far, far older than deductive geometry.”
Trudeau’s book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called “Diamond Theory.”
Although non-Euclidean,* the theorems of the 1976 “Diamond Theory” are also, in Trudeau’s terminology, diamonds.
* “Non-Euclidean” here means merely “other than Euclidean.” No violation of Euclid’s parallel postulate is implied.
Saturday, February 27, 2010
Cubist Geometries
"The cube has…13 axes of symmetry:
6 C2 (axes joining midpoints of opposite edges),
4 C3 (space diagonals), and
3C4 (axes joining opposite face centroids)."
–Wolfram MathWorld article on the cube
These 13 symmetry axes can be used to illustrate the interplay between Euclidean and Galois geometry in a cubic model of the 13-point Galois plane.
The geometer's 3×3×3 cube–
27 separate subcubes unconnected
by any Rubik-like mechanism–

The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
A closely related structure–
the finite projective plane
with 13 points and 13 lines–
A later version of the 13-point plane
by Ed Pegg Jr.–
A group action on the 3×3×3 cube
as illustrated by a Wolfram program
by Ed Pegg Jr. (undated, but closely
related to a March 26, 1985 note
by Steven H. Cullinane)–
The above images tell a story of sorts.
The moral of the story–
Galois projective geometries can be viewed
in the context of the larger affine geometries
from which they are derived.
The standard definition of points in a Galois projective plane is that they are lines through the (arbitrarily chosen) origin in a corresponding affine 3-space converted to a vector 3-space.
If we choose the origin as the center cube in coordinatizing the 3×3×3 cube (See Weyl's relativity problem ), then the cube's 13 axes of symmetry can, if the other 26 cubes have properly (Weyl's "objectively") chosen coordinates, illustrate nicely the 13 projective points derived from the 27 affine points in the cube model.
The 13 lines of the resulting Galois projective plane may be derived from Euclidean planes through the cube's center point that are perpendicular to the cube's 13 Euclidean symmetry axes.
The above standard definition of points in a Galois projective plane may of course also be used in a simpler structure– the eightfold cube.
(The eightfold cube also allows a less standard way to picture projective points that is related to the symmetries of "diamond" patterns formed by group actions on graphic designs.)
See also Ed Pegg Jr. on finite geometry on May 30, 2006
at the Mathematical Association of America.
Wednesday, January 6, 2010
Brightness at Noon, continued
The Galois Quaternion
|
I had foreseen it all in precise detail. i = an imaginary being Here, on this complex space, |
Related material:
The Galois Quaternion
Click for context.
(See also Nativity and the end
of this morning's post.)
Tuesday, September 8, 2009
Tuesday September 8, 2009
Froebel's
Magic Box

Continued from Dec. 7, 2008,
and from yesterday.
|
Non-Euclidean
Blocks
Passages from a classic story:
… he took from his pocket a gadget he had found in the box, and began to unfold it. The result resembled a tesseract, strung with beads….  Tesseract
"Your mind has been conditioned to Euclid," Holloway said. "So this– thing– bores us, and seems pointless. But a child knows nothing of Euclid. A different sort of geometry from ours wouldn't impress him as being illogical. He believes what he sees."
"Are you trying to tell me that this gadget's got a fourth dimensional extension?" Paradine demanded. "Hardening of the thought-arteries," Jane interjected. Paradine was not convinced. "Then a baby could work calculus better than Einstein? No, I don't mean that. I can see your point, more or less clearly. Only–" "Well, look. Let's suppose there are two kinds of geometry– we'll limit it, for the sake of the example. Our kind, Euclidean, and another, which we'll call x. X hasn't much relationship to Euclid. It's based on different theorems. Two and two needn't equal four in it; they could equal y, or they might not even equal. A baby's mind is not yet conditioned, except by certain questionable factors of heredity and environment. Start the infant on Euclid–" "Poor kid," Jane said. Holloway shot her a quick glance. "The basis of Euclid. Alphabet blocks. Math, geometry, algebra– they come much later. We're familiar with that development. On the other hand, start the baby with the basic principles of our x logic–" "Blocks? What kind?" Holloway looked at the abacus. "It wouldn't make much sense to us. But we've been conditioned to Euclid." — "Mimsy Were the Borogoves," Lewis Padgett, 1943 |
Padgett (pseudonym of a husband-and-wife writing team) says that alphabet blocks are the intuitive "basis of Euclid." Au contraire; they are the basis of Gutenberg.
For the intuitive basis of one type of non-Euclidean* geometry– finite geometry over the two-element Galois field– see the work of…
Friedrich Froebel
(1782-1852), who
invented kindergarten.
His "third gift" —

fom the Inventing Kindergarten
exhibit at The Institute for Figuring
Tuesday, August 18, 2009
Tuesday August 18, 2009
Prima Materia
(Background: Art Humor: Sein Feld (March 11, 2009) and Ides of March Sermon, 2009)
From Cardinal Manning's review of Kirkman's Philosophy Without Assumptions—
"And here I must confess… that between something and nothing I can find no intermediate except potentia, which does not mean force but possibility."
— Contemporary Review, Vol. 28 (June-November, 1876), page 1017
Furthermore….
Cardinal Manning, Contemporary Review, Vol. 28, pages 1026-1027:
The following will be, I believe, a correct statement of the Scholastic teaching:–
1. By strict process of reason we demonstrate a First Existence, a First Cause, a First Mover; and that this Existence, Cause, and Mover is Intelligence and Power.
2. This Power is eternal, and from all eternity has been in its fullest amplitude; nothing in it is latent, dormant, or in germ: but its whole existence is in actu, that is, in actual perfection, and in complete expansion or actuality. In other words God is Actus Purus, in whose being nothing is potential, in potentia, but in Him all things potentially exist.
3. In the power of God, therefore, exists the original matter (prima materia) of all things; but that prima materia is pura potentia, a nihilo distincta, a mere potentiality or possibility; nevertheless, it is not a nothing, but a possible existence. When it is said that the prima materia of all things exists in the power of God, it does not mean that it is of the existence of God, which would involve Pantheism, but that its actual existence is possible.
4. Of things possible by the power of God, some come into actual existence, and their existence is determined by the impression of a form upon this materia prima. The form is the first act which determines the existence and the species of each, and this act is wrought by the will and power of God. By this union of form with the materia prima, the materia secunda or the materia signata is constituted.
5. This form is called forma substantialis because it determines the being of each existence, and is the root of all its properties and the cause of all its operations.
6. And yet the materia prima has no actual existence before the form is impressed. They come into existence simultaneously;
[p. 1027 begins]
as the voice and articulation, to use St. Augustine's illustration, are simultaneous in speech.
7. In all existing things there are, therefore, two principles; the one active, which is the form– the other passive, which is the matter; but when united, they have a unity which determines the existence of the species. The form is that by which each is what it is.
8. It is the form that gives to each its unity of cohesion, its law, and its specific nature.*
When, therefore, we are asked whether matter exists or no, we answer, It is as certain that matter exists as that form exists; but all the phenomena which fall under sense prove the existence of the unity, cohesion, species, that is, of the form of each, and this is a proof that what was once in mere possibility is now in actual existence. It is, and that is both form and matter.
When we are further asked what is matter, we answer readily, It is not God, nor the substance of God; nor the presence of God arrayed in phenomena; nor the uncreated will of God veiled in a world of illusions, deluding us with shadows into the belief of substance: much less is it catter [pejorative term in the book under review], and still less is it nothing. It is a reality, the physical kind or nature of which is as unknown in its quiddity or quality as its existence is certainly known to the reason of man.
* "… its specific nature"
(Click to enlarge) —
For a more modern treatment of these topics, see Werner Heisenberg's Physics and Philosophy. For instance:
"The probability wave of Bohr, Kramers, Slater, however, meant… a tendency for something. It was a quantitative version of the old concept of 'potentia' in Aristotelian philosophy. It introduced something standing in the middle between the idea of an event and the actual event, a strange kind of physical reality just in the middle between possibility and reality."
Compare to Cardinal Manning's statement above:
"… between something and nothing I can find no intermediate except potentia…"
To the mathematician, the cardinal's statement suggests the set of real numbers between 1 and 0, inclusive, by which probabilities are measured. Mappings of purely physical events to this set of numbers are perhaps better described by applied mathematicians and physicists than by philosophers, theologians, or storytellers. (Cf. Voltaire's mockery of possible-worlds philosophy and, more recently, The Onion's mockery of the fictional storyteller Fournier's quantum flux. See also Mathematics and Narrative.)
Regarding events that are not purely physical– those that have meaning for mankind, and perhaps for God– events affecting conception, birth, life, and death– the remarks of applied mathematicians and physicists are often ignorant and obnoxious, and very often do more harm than good. For such meaningful events, the philosophers, theologians, and storytellers are better guides. See, for instance, the works of Jung and those of his school. Meaningful events sometimes (perhaps, to God, always) exhibit striking correspondences. For the study of such correspondences, the compact topological space [0, 1] discussed above is perhaps less helpful than the finite Galois field GF(64)– in its guise as the I Ching. Those who insist on dragging God into the picture may consult St. Augustine's Day, 2006, and Hitler's Still Point.
Monday, July 27, 2009
Monday July 27, 2009
The New York Times
on June 17, 2007:
Design Meets Dance,
and Rules Are Broken
Yesterday's evening entry was
on the fictional sins of a fictional
mathematician and also (via a link
to St. Augustine's Day, 2006), on
the geometry of the I Ching* —
The eternal
combined with
the temporal:

The fictional mathematician's
name, noted here (with the Augustine-
I Ching link as a gloss) in yesterday's
evening entry, was Summerfield.
From the above Times article–
"Summerspace," a work by
choreographer Merce Cunningham
and artist Robert Rauschenberg
that offers a competing
vision of summer:


From left, composer John Cage,
choreographer Merce Cunningham,
and artist Robert Rauschenberg
in the 1960's
"When shall we three meet again?"
Saturday, April 25, 2009
Saturday April 25, 2009

|
Hotel Puzzle by John Tierney "Russell Crowe arrives at the Hotel Infinity looking tired and ornery. He demands a room. The clerk informs him that there are no vacancies…."
|
Footprints from California today
(all by a person or persons using Firefox browsers):
7:10 AM
http://m759.xanga.com/679142359/concepts-of-space/?
Concepts of Space: Euclid vs. Galois
8:51 AM
http://m759.xanga.com/689601851/art-wars-continued/?
Art Wars continued: Behind the Picture
1:33 PM
http://m759.xanga.com/678995132/a-riff-for-dave/?
A Riff for Dave: Me and My Shadow
2:11 PM
http://m759.xanga.com/638308002/a-death-of-kings/?
A Death of Kings: In Memory of Bobby Fischer
2:48 PM
http://m759.xanga.com/691644175/art-wars-in-review–/?
Art Wars in review– Through the Looking Glass: A Sort of Eternity
3:28 PM and
http://m759.xanga.com/684680406/annals-of-philosophy/?
Annals of Philosophy: The Dormouse of Perception
4:28 PM
http://m759.xanga.com/641536988/epiphany-for-roy-part-i/?
Epiphany for Roy, Part I
6:03 PM
http://m759.xanga.com/641949564/art-wars-continued/?
At the Still Point: All That Jazz
6:22 PM
http://m759.xanga.com/644330798/where-entertainment-is-not-god/?
Where Entertainment is Not God: The Just Word
7:14 PM
http://m759.xanga.com/643490468/happy-new-yorker-day/?
Happy New Yorker Day– Class Galore
7:16 PM
http://m759.xanga.com/643812753/the-politics-of-change/?
The Politics of Change: Jumpers
"We are programmed to receive."
— Hotel California
Tuesday, February 24, 2009
Tuesday February 24, 2009
Meets
Pantheistic Solipsism
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."

|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)

over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Cara:

Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Thursday, February 5, 2009
Thursday February 5, 2009
Through the
Looking Glass:
A Sort of Eternity
From the new president’s inaugural address:
“… in the words of Scripture, the time has come to set aside childish things.”
The words of Scripture:
“through a glass”—
[di’ esoptrou].
By means of
a mirror [esoptron].
Childish things:

fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
Not-so-childish:
Three planes through
the center of a cube
that split it into
eight subcubes:

Through a glass, darkly:
A group of 8 transformations is
generated by affine reflections
in the above three planes.
Shown below is a pattern on
the faces of the 2x2x2 cube
that is symmetric under one of
these 8 transformations–
a 180-degree rotation:
(Click on image
for further details.)
But then face to face:
A larger group of 1344,
rather than 8, transformations
of the 2x2x2 cube
is generated by a different
sort of affine reflections– not
in the infinite Euclidean 3-space
over the field of real numbers,
but rather in the finite Galois
3-space over the 2-element field.

Galois age fifteen,
drawn by a classmate.
These transformations
in the Galois space with
finitely many points
produce a set of 168 patterns
like the one above.
For each such pattern,
at least one nontrivial
transformation in the group of 8
described above is a symmetry
in the Euclidean space with
infinitely many points.
For some generalizations,
see Galois Geometry.
Related material:
The central aim of Western religion–
"Each of us has something to offer the Creator... the bridging of masculine and feminine, life and death. It's redemption.... nothing else matters." -- Martha Cooley in The Archivist (1998) The central aim of Western philosophy– Dualities of Pythagoras as reconstructed by Aristotle: Limited Unlimited Odd Even Male Female Light Dark Straight Curved ... and so on .... “Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy.” — Jamie James in The Music of the Spheres (1993) “In the garden of Adding — The Midrash Jazz Quartet in City of God, by E. L. Doctorow (2000) A quotation today at art critic Carol Kino’s website, slightly expanded: “Art inherited from the old religion — Octavio Paz,”Seeing and Using: Art and Craftsmanship,” in Convergences: Essays on Art and Literature (New York: Harcourt Brace Jovanovich 1987), 52 From Brian O’Doherty’s 1976 Artforum essays– not on museums, but rather on gallery space: “We have now reached
“Space: what you — James Joyce, Ulysses |