Eight is a Gate (continued)
|
Tom Stoppard, Jumpers:
"Heaven, how can I believe in Heaven?" she sings at the finale. "Just a lying rhyme for seven!"
"To begin at the beginning: Is God?…" [very long pause]
|
|
From "Space," by Salomon Bochner
Makom. Our term “space” derives from the Latin, and is thus relatively late. The nearest to it among earlier terms in the West are the Hebrew makom and the Greek topos (τόπος). The literal meaning of these two terms is the same, namely “place,” and even the scope of connotations is virtually the same (Theol. Wörterbuch…, 1966). Either term denotes: area, region, province; the room occupied by a person or an object, or by a community of persons or arrangements of objects. But by first occurrences in extant sources, makom seems to be the earlier term and concept. Apparently, topos is attested for the first time in the early fifth century B.C., in plays of Aeschylus and fragments of Parmenides, and its meaning there is a rather literal one, even in Parmenides. Now, the Hebrew book Job is more or less contemporary with these Greek sources, but in chapter 16:18 occurs in a rather figurative sense:
Late antiquity was already debating whether this makom is meant to be a “hiding place” or a “resting place” (Dhorme, p. 217), and there have even been suggestions that it might have the logical meaning of “occasion,” “opportunity.” Long before it appears in Job, makom occurs in the very first chapter of Genesis, in:
This biblical account is more or less contemporary with Hesiod's Theogony, but the makom of the biblical account has a cosmological nuance as no corresponding term in Hesiod. Elsewhere in Genesis (for instance, 22:3; 28:11; 28:19), makom usually refers to a place of cultic significance, where God might be worshipped, eventually if not immediately. Similarly, in the Arabic language, which however has been a written one only since the seventh century A.D., the term makām designates the place of a saint or of a holy tomb (Jammer, p. 27). In post-biblical Hebrew and Aramaic, in the first centuries A.D., makom became a theological synonym for God, as expressed in the Talmudic sayings: “He is the place of His world,” and “His world is His place” (Jammer, p. 26). Pagan Hellenism of the same era did not identify God with place, not noticeably so; except that the One (τὸ ἕν) of Plotinus (third century A.D.) was conceived as something very comprehensive (see for instance J. M. Rist, pp. 21-27) and thus may have been intended to subsume God and place, among other concepts. In the much older One of Parmenides (early fifth century B.C.), from which the Plotinian One ultimately descended, the theological aspect was only faintly discernible. But the spatial aspect was clearly visible, even emphasized (Diels, frag. 8, lines 42-49). BIBLIOGRAPHY Paul Dhorme, Le livre de Job (Paris, 1926). H. Diels and W. Kranz, Die Fragmente der Vorsokratiker, 6th ed. (Berlin, 1938). Max Jammer, Concepts of Space… (Cambridge, Mass., 1954). J. M. Rist, Plotinus: The Road to Reality (Cambridge, 1967). Theologisches Wörterbuch zum Neuen Testament (1966), 8, 187-208, esp. 199ff.
|
Related material: In the previous entry — "Father Clark seizes at one place (page eight)
upon the fact that…."
Father Clark's reviewer (previous entry) called a remark by Father Clark "far fetched."
This use of "place" by the reviewer is, one might say, "near fetched."
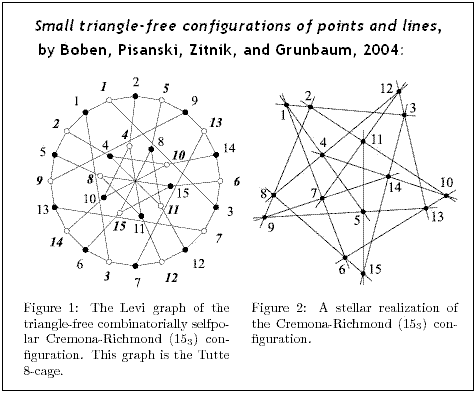
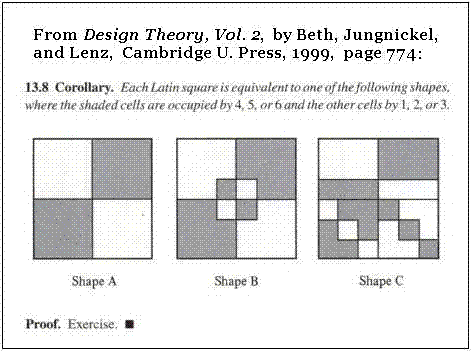
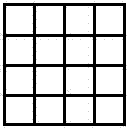
lets us visualize the projective space PG(3,2) as well as the affine space AG(4,2). For 
“Imaginary time is a relatively simple concept that is rather difficult to visualize or conceptualize. In essence, it is another direction of time moving at right angles to ordinary time. In the image at right, the light gray lines represent ordinary time flowing from left to right – past to future. The dark gray lines depict imaginary time, moving at right angles to ordinary time.”
